NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle

NCERT Exemplar Solutions For Class 10 Maths Chapter 6  Triangles

Introduction:

• To see the above pairs of figures, what do you think?
• Figures in a pair are alike, i.e., their shapes are the same.
• Shapes are the same but do not mean that they are necessarily equal in size.
• Shapes the same mean that their curvature is the same, i.e., figures are proportionately (not necessarily exactly) the same insides as well as angles.

NCERT Exemplar Solutions For Class 10 Maths Chapter 6  Difference Between Proportional And Equal

1. In Terms of Sides :

⇒ \(\frac{A B}{P Q}=\frac{2 x}{2 y}=\frac{x}{y}\)

⇒ \(\frac{B C}{Q R}=\frac{3 x}{3 y}=\frac{x}{y}\)

⇒ \(\frac{A C}{P R}=\frac{4 x}{4 y}=\frac{x}{y}\)

⇒  \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A C}{P R}\), i.e, corresponding angles are proportional.

Read and Learn More Class 10 Maths Solutions Exemplar

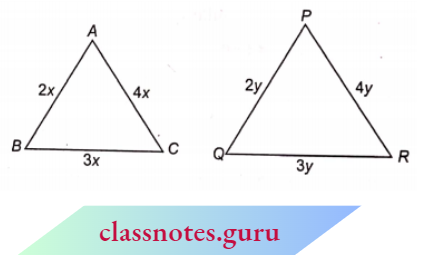
Suppose, the value of x = 2 sandy = 3.5 then the sides of the first triangle are 2 x 2, 3 x 2, 4 x 2, i.e., 4 cm, 6 cm, and 8 cm, and the sides of the second triangle are 2 x 3.5, 3 x 3.5, 4 x 3.5, i.e., 7 cm, 10.5 cm, and 14 cm. So, we can say that the corresponding sides are proportional, but that does not mean that their corresponding sides are equal.

2. In terms of Angles :

Here,\(\frac{\angle A}{\angle P}=\frac{2 x}{2 y}=\frac{x}{y}\)

⇒ \(\frac{\angle B}{\angle Q}=\frac{3 x}{3 y}=\frac{x}{y}\)

⇒ \(\frac{\angle C}{\angle R}=\frac{4 x}{4 y}=\frac{x}{y}\)

⇒ \(\frac{\angle A}{\angle P}=\frac{\angle B}{\angle Q}=\frac{\angle C}{\angle R}\)

i.e., corresponding angles are proportional.

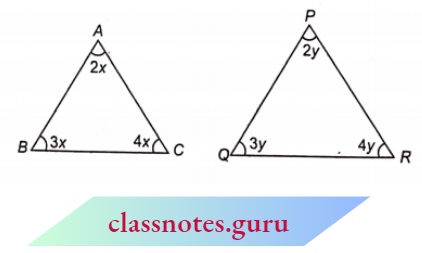
Now, see whether x and y are equal or they will be different.

We know that,

⇒ \(\angle A+\angle B+\angle C =180^{\circ}\)

⇒ \(2 r+3 x+4 r =180^{\circ}\)

⇒ \(9 x =180^{\circ} \Rightarrow x=20^{\circ}\)

⇒ \(\angle A=2 \times 20^{\circ}=40^{\circ}, \angle B =3 \times 20^{\circ}=60^{\circ}, \angle C=4 \times 20^{\circ}=80^{\circ}\)

⇒ \(\angle P+\angle Q+\angle R =180^{\circ}\)

⇒ \(2 y+3 y+4 y =180^{\circ}\)

⇒ \(9 y =180^{\circ} \Rightarrow y=20^{\circ}\)

P=2 \(\times 20^{\circ}=40^{\circ}, \angle Q =3 \times 20^{\circ}=60^{\circ}, \angle R=4 \times 20^{\circ}=80^{\circ}\)

⇒ \(\angle A=\angle P, \angle B=\angle Q\) and \(\angle C=\angle R\) .

From (1) and (2) we conclude that:

• Corresponding sides are proportional does not mean that their corresponding sides are equal.
• Corresponding angles are proportional means that their corresponding angles are necessarily equal.
• So, corresponding parts are proportional means
• Two triangles are similar if their shapes are the same.

NCERT Exemplar Solutions For Class 10 Maths Chapter 6  Definition Of Similar Triangles

Two triangles are similar if their corresponding parts are proportional.

1. their corresponding sides are proportional.
2.  their corresponding angles are equal.

From the above figures, two triangles ABC and PQR are Bi similar, i.e.\(\triangle\)ABC ~ \(\triangle\)PQR, if any one of the following properties occurs :

(1) Their corresponding sides are proportional (or the ratio of their corresponding sides are equal)

⇒ \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A C}{P R}\) (SSS similarity)

(2) Their corresponding angles are equal

⇒ \(\angle A=\angle P, \angle B=\angle Q, \angle C=\angle R\) (AAA similarity)

If any two corresponding angles are equal

⇒ \(\left.\begin{array}{ll}\text { i.e., } & \angle A=\angle P \text { and } \angle B=\angle Q \\ \text { or } & \angle A=\angle P \text { and } \angle C=\angle R \\ \text { or } & \angle B=\angle Q \text { and } \angle C=\angle R\end{array}\right\}\) (AA similarity)

⇒ Any two corresponding sides are proportional and their included angles are the same i.e., or

⇒ \(\left.\begin{array}{l}
\frac{A B}{P Q}=\frac{B C}{Q R} \text { and } \angle B=\angle Q \\
\frac{A B}{P Q}=\frac{A C}{P R} \text { and } \angle A=\angle P \\
\frac{B C}{Q R}=\frac{A C}{P R} \text { and } \angle C=\angle R
\end{array}\right\}\)

The above three postulates are the postulates of the similarity of triangles

Remark:

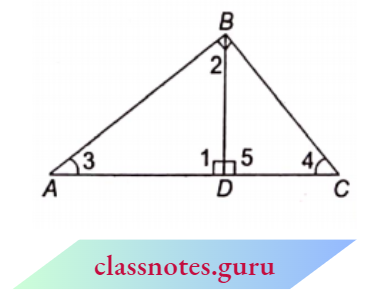
1. In an obtuse-angled triangle,

⇒  \(ar(\triangle A B C) =\frac{1}{2} \times \text { Base } \times \text { Corresponding altitude }\)[/latex]

= \(\frac{1}{2} \times B C \times A D\)

In an obtuse triangle, the perpendicular will be inside the triangle.

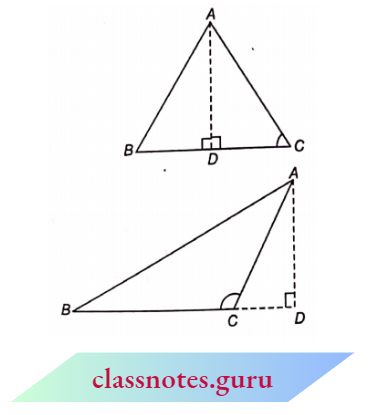
2. In an obtuse-angled triangle,

⇒ \(ar(\triangle A B C) =\frac{1}{2} \times \text { Base } \times \text { Corresponding altitude }\)

=\(\frac{1}{2} \times B C \times A D\)

In an obtuse triangle, the perpendicular will be outside the triangle.

Note: In \(\triangle\)ABC, when the base is taken as BC, then perpendicular will be drawn from the angle opposite to the base, i.e. \(\angle\)A on the base BC (whether it lies inside the BC or outside the BC).

NCERT Exemplar Solutions For Class 10 Maths Chapter 6  Difference Between Congruency And Similarity

• Two things are said to be congruent if they have the same shape as well as the same size, i.e., the two figures coincide with each other.
• Two things are said to be similar to each other if they have the same shapes but their sizes may be different.

Remark:

1. Congruent triangles are necessarily similar triangles but the converse is not always true.
2. If two triangles are similar to the third triangle, then they are similar to each other.

Basic Proportionality Theorem Or Thale’s Theorem

Theorem 1: If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points then it divides the other two sides in the same ratio.

Given: \(\triangle\)ABC in which DE || BC and DE intersects AB and AC at D and E respectively.

To Prove: \(\frac{A D}{D B}=\frac{A E}{E C}\)

Construction: Join BE and CD. Draw EL \(\perp AB\) and \(DM \perp AC\)

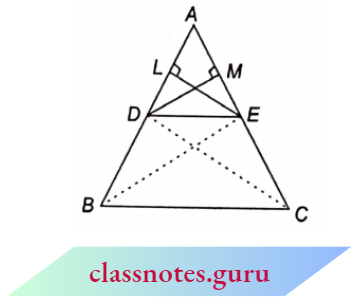
Proof:

Area of \((\triangle A D E) =\frac{1}{2} \times A D \times E L\)

Area of \((\triangle D B E) =\frac{1}{2} \times D B \times E L \)

⇒ \(\frac{{ar}(\triangle A D E)}{{ar}(\triangle D B E)} =\frac{\frac{1}{2} \times A D \times E L}{\frac{1}{2} \times D B \times E L}=\frac{A D}{D B}\)

Again Area of \(\triangle M D E =\frac{1}{2} \times A E \times D M\)

Area of \(\triangle E C D =\frac{1}{2} \times E C \times D M\)

⇒\(\frac{{ar}(\triangle A D E)}{{ar}(\triangle E C D)} =\frac{\frac{1}{2} \times A E \times D M}{\frac{1}{2} \times E C \times D M}=\frac{A E}{E C}\)

But since \(\triangle\)DBE and \(\triangle\)ECD are on the same base DE and between the same parallels DE and BC, we have

⇒ \({ar}(\triangle D B E)={ar}(\triangle E C D)\)

From (1), (2), and (3), we get \(\frac{A D}{D B}=\frac{A E}{E C}\)

Corollary: In a \(\triangle\)ABC, a line DE || BC intersects AB in D and AC in E, then prove that:

⇒ \(\frac{A B}{D B}=\frac{A C}{E C}\)

⇒ \(\frac{A D}{A B}=\frac{A E}{A C}\)

Proof: (1) From basic proportionality theorem

⇒ \(\frac{A D}{D B}=\frac{A E}{E C}\)

Adding 1 on both sides, we have

⇒ \(\frac{A D}{D B}+1 =\frac{A E}{E C}+1\)

⇒ \(\frac{A D+D B}{D B} =\frac{A E+E C}{E C} \Rightarrow \quad \frac{A B}{D B}=\frac{A C}{E C}\)

(2) From the basic proportionality theorem, we have

⇒ \(\frac{A D}{D B}=\frac{A E}{E C} \quad \Rightarrow \quad \frac{D B}{A D}=\frac{E C}{A E}\)

Adding 1 on both sides, we have

⇒ \(\frac{D B}{A D}+1 =\frac{E C}{A E}+1\)

⇒ \(\frac{D B+A D}{A D}=\frac{E C+A E}{A E}\)

⇒ \(\frac{A B}{A D}=\frac{A C}{A E} \quad \Rightarrow \quad \frac{A D}{A B}=\frac{A E}{A C}\)

Now, we can conclude

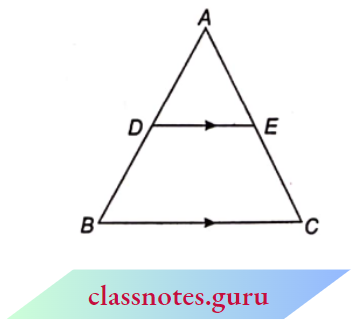
In \(\triangle A B C\) if D E || BC

1.  \(\quad \frac{A D}{D B}=\frac{A E}{E C}\) (B.P.T.)
2. \(\frac{A B}{D B}=\frac{A C}{E C}\)
3.  \(\frac{A D}{A B}=\frac{A E}{A C}\)

These Are the Same operations in R.H.S, as in the L.H.S.

NCERT Exemplar Solutions For Class 10 Maths Chapter 6  Converse of Basic Proportionality Theorem

Theorem 2: If a line divides any two sides of a triangle in the same ratio then the line must be parallel to the third side.

Given: \(\triangle\)ABC in which a line l intersects AB at D and AC at E, such that 12

To Prove: DE \\BC

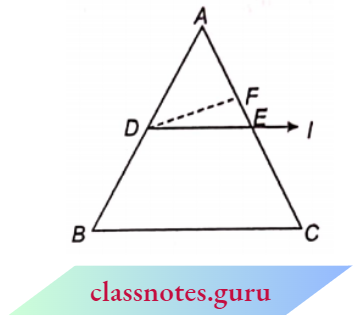
Proof: If possible, let DE is not parallel to BC, then there must be another line through D, which is parallel to BC. Let DE || BC.

Then by the basic proportionality theorem, we have

⇒ \(\frac{A D}{D B} =\frac{A F}{F C}\)

But \(\frac{A D}{D B}=\frac{A E}{E C}\)

From (1) and (2) we have \(\frac{A F}{F C}=\frac{A E}{E C}\)

Now, adding 1 on both sides

⇒ \(\frac{A F}{F C}+1=\frac{A E}{E C}+1 \quad \Rightarrow \quad \frac{A F+F C}{F C}=\frac{A E+E C}{E C}\)

⇒ \(\frac{A C}{F C}=\frac{A C}{E C} \quad \Rightarrow \quad F C=E C\)

This is possible only, when E and F coincide.

Hence D E || B C (D F || BC, by construction)

NCERT Exemplar Solutions For Class 10 Maths Chapter 6  Solved Examples

Example 1. In the adjoining figure DE || BC and D divide AB in the ratio 2 : 3. Find.

1. \(\frac{A E}{E C}\)
2. \(\frac{A E}{A C}\)

Solution:

Given

In the adjoining figure DE || BC and D divide AB in the ratio 2 : 3.

(1) Since D E || BC, hence by Thale’s theorem

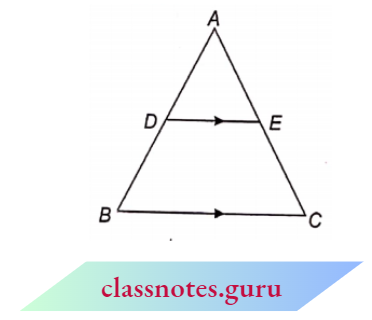
⇒ \(\frac{A D}{D B}=\frac{A E}{E C}\)

Also, \(\frac{A D}{D B}=\frac{2}{3}\) (given)

Therefore, from (1) and (2), \(\frac{A D}{D B}=\frac{A E}{E C}=\frac{2}{3}\)

(2) \(\frac{AE}{AC}\)=\(\frac{A E}{A E+E C}\)

= \(\frac{A E / E C}{A E / E C+E C / E C}\)

= \(\frac{\frac{2}{3}}{\frac{2}{3}+1}=\frac{\frac{2}{3}}{\frac{5}{3}}=\frac{2}{5}\)

Example 2. In the figure, PQ is parallel to MN, if \(\frac{K P}{P M}=\frac{4}{13}\) and KN = 20.4. Find KQ

Solution:

Given In \(\triangle\)KMN, PQ || MN

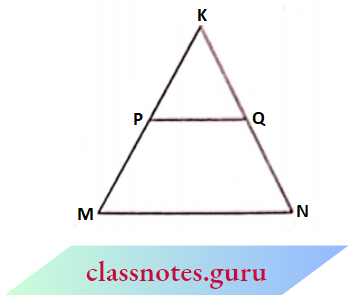
⇒ \(\frac{K P}{P M}=\frac{K Q}{Q N}\) (by B.P.T. or Thale’s theorem)

Let K Q=x, then Q N=20.4-x

Now, from (1) \(\frac{4}{13} =\frac{x}{20.4-x}\)

81.6-4 x =13 x

17 x =81.6

x =4.8 cm

Therefore K Q=4.8 cm

Example 3. In the figure, DE || BC. If DB = 10.8 cm, AE = 2.7 cm and£C =8.1 cm, find AD. DE || BC

Solution:

Given

In the figure, DE || BC. If DB = 10.8 cm, AE = 2.7 cm and£C =8.1 cm

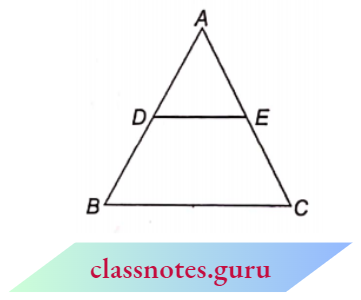
⇒ \(\frac{A D}{D B}=\frac{A E}{E C}\)

⇒ \(\frac{A D}{10.8}=\frac{2.7}{8.1}\)

A D=\(\frac{1}{3} \times 10.8 \mathrm{~cm}=3.6 \mathrm{~cm}\)

Example 4. In the given figure, in \(\triangle\)ABC, DE || BC so that AD = (4x – 3) cm, AE = (8x – 7) cm. BD = (3x – 1) cm and CE = (5x – 3) cm. Find the value of x.

Solution:

Given

In the given figure, in \(\triangle\)ABC, DE || BC so that AD = (4x – 3) cm, AE = (8x – 7) cm. BD = (3x – 1) cm and CE = (5x – 3) cm.

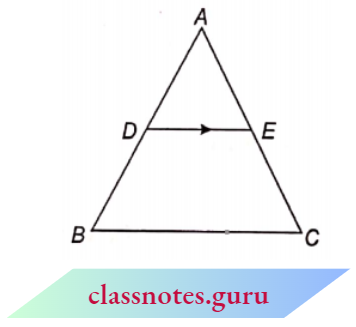
⇒ \(\triangle\)ABC, DE || BC

⇒ \(\frac{A D}{B D}=\frac{A E}{C E}\)

⇒ \(\frac{4 x-3}{3 x-1}=\frac{8 x-7}{5 x-3}\)

(4 x-3)(5 x-3)=(8 x-7)(3 x-1)

20 x²-27 x+9=24 x²-29 x+7

4 x²-2 x-2=0

2 x²-x-1=0

2 x²-2 x+x-1=0

2 x(x-1)+1(x-1)=0

(2 x+1)(x-1)=0

x=1 or x=-\(\frac{1}{2}\) (by Thale’s theorem)

But when x=-\(\frac{1}{2}\)

A D=\(\left[4 \times\left(-\frac{1}{2}\right)-3\right]\)=-5

Since distance cannot be negative so x \(\neq-\frac{1}{2}\)

Here X = 1

The value of x = 1

Example 5. The bisector (internal or external) of an angle of a triangle divides the opposite side (internally or externally) in the ratio of the sides containing the angle.

Solution:

Given: \(\triangle\)ABC in which the bisector AD of \(\angle\)A meets BC (or BC produced) in D.

To Prove : \(\frac{B D}{D C}=\frac{A B}{A C}\)

Construction: Draw CE || DA meeting BA (produced if necessary) in E.

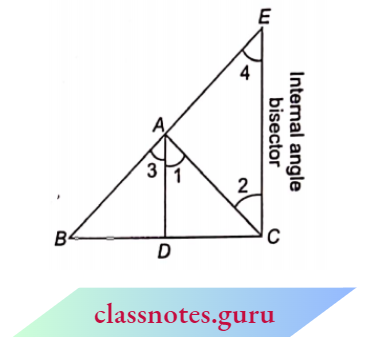
Proof: Since CE || DA

⇒ \(\angle 1=\angle 2\) (alternate angles)

and \(\angle 3 =\angle 4\) (corresponding angles) (2)

But \(\angle 1=\angle 3\) (given)…(3)

⇒ \(\angle 2=\angle 4\) [from (1), (2) and (3)]

An E=A C (sides opposite to equal angles are equal) …(4)

Now, since CE || DA (construction)

⇒ \(\frac{B D}{D C}=\frac{B A}{A E}\)

⇒ \(\frac{B D}{D C}=\frac{B A}{A C}\) [from (4) and (5)]

Example 6. Prove that any line parallel to parallel sides of a trapezium divides the non-parallel sides proportionally.

Solution:

Given: A trapezium ABCD in which AB || DC.

Also a line EF || AB || DC.

To Prove: \(\frac{A E}{E D}=\frac{B F}{F C}\)

Construction: Join DB which intersects E F at G,

Proof: In \(\triangle A D B\), since E G || A B. ( E F || A B)

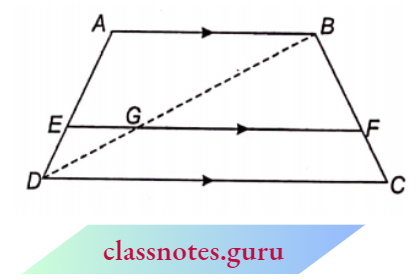
⇒ \(\frac{A E}{E D}=\frac{B G}{G D}\) (by B.P. theorem)

Also, in \(\triangle B D C, since G F || DC (E F || D C)\)

⇒ \(\frac{B F}{F C}=\frac{B G}{G D}\) (by B.P. theorem)

From (1) and (2), we have

⇒ \(\frac{A E}{E D}=\frac{B F}{F C}\)

Hence Proved.

Example 7. The side BC of a triangle ABC is bisected at D. O is any point in Al). BO and CO produced meet AC and AB at E and F respectively and AD is produced to X so that D is the mid-point of OX. Prove that AO: AX = AF: AB and show that FE || BC.

Solution:

Given

The side BC of a triangle ABC is bisected at D. O is any point in Al). BO and CO produced meet AC and AB at E and F respectively and AD is produced to X so that D is the mid-point of OX.

Join BX and CX.

Since D is the mid-point of BC (given)

BD = DC …(1)

Also, D is the mid-point of OX (given)

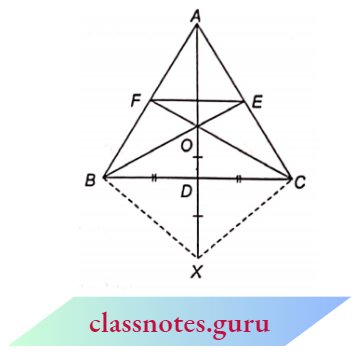
OD = DX …(2)

From (1) and (2), we have

⇒ [Square OBXC is a parallelogram (diagonals bisect each other) B

OC || BX (opposite sides of a parallelogram)

FC || BX ⇒ OE || XC

In \(\triangle A X C\),

⇒ \(\frac{A O}{O X}=\frac{A E}{E C}\) (by B.P. theorem)

From (3) and (4), we have

⇒ \(\frac{A F}{F B}=\frac{A E}{E C}\)

In \(\triangle A B C\), F E || B C. (converse of B.P. theorem)

Hence Proved.

Example 8. In \(\triangle\)ABC, D, and E are two points on AB such that AD = BE. If DP || BC and EQ || AC, prove that PQ || AB.

Solution:

Given: \(\triangle\)ABC in which D and E are two points such that AD = BE.

Also DP || BC and EQ || AC.

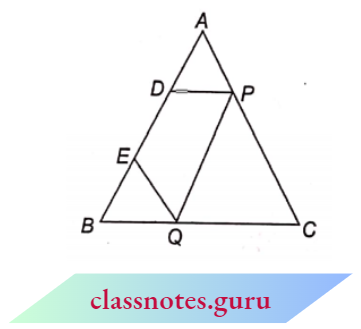
To Prove: PQ || AB

Proof: In ΔABC, Since DP || BC

⇒ \(\frac{A D}{D B}=\frac{A P}{P C}\) (by B.P. theorem) …. 1

In \(\triangle A B C\), since E Q || A C

⇒ \(\frac{B E}{E A}=\frac{B Q}{Q C}\) (by B.P. theorem) …. 2

Now, as A D = B E (given) …. 3

A D+D E=B E+D E (adding DE on both sides)

AE = BD ….. 4

From (1), (2), (3) and (4),

⇒ \(\frac{A P}{P C}=\frac{B Q}{Q C}\)

P Q || A B (by the converse of B.P. theroem)

Hence Proved.

Criteria For Similarity Of Two Triangles

• Two triangles are said to be similar if their corresponding angles are equal and corresponding sides are proportional (i.e., the ratios between the lengths of corresponding sides are equal).
• For example if in \(\triangle\)ABC and \(\triangle\)PQR
•  \(\angle A=\angle P, \angle B=\angle Q, \angle C=\angle R\)
• and, \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A C}{P R}\)
• then \(\triangle\)ABC is similar to \(\triangle\)PQR.
• Symbol ~ is used for “is similar to”

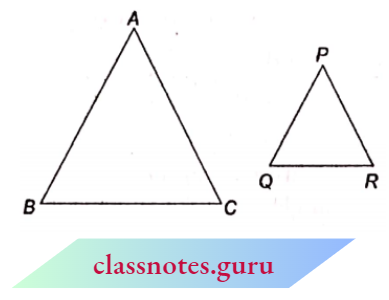
Conversely: If \(\triangle\)ABC is similar to \(\triangle\)PQR then

⇒ \(\angle A=\angle P, \angle B=\angle Q, \angle C=\angle R\)

⇒ \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A C}{P R}\)

Theorem 1 (AAA Similarity): If in two triangles the corresponding angles are equal, then their corresponding sides are proportional and hence the triangles are similar.

Given: \(\triangle\)ABC and \(\triangle\)DEF such that

⇒ \(\angle A=\angle D, \angle B=\angle E \text {, and } \angle C=\angle F\)

To Prove: \(\triangle\)ABC ~ \(\triangle\)DEF

Construction: Cut DP =AB and DQ = AC. Join PQ.

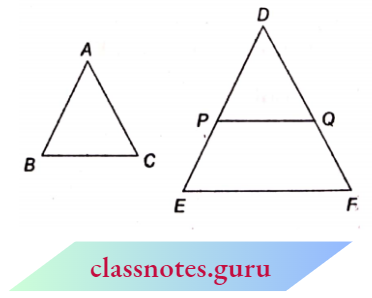
Proof: In \(\triangle\)ABC and \(\triangle\)DPQ, we have

A B =D P

A C =D Q (by construction)

⇒ \(\angle A =\angle D\)

⇒ \(\triangle A B C \cong \triangle D P Q\) (by SAS congruence)

⇒ \(\angle B =\angle P\)

But it is given that \(\angle B=\angle E\)

Therefore, \(\angle E=\angle P\)

P Q || E F

⇒ \(\frac{D P}{D E}=\frac{D Q}{D F}\)

⇒ \(\frac{A B}{D E}=\frac{C A}{F D}\)

Similarly \(\frac{A B}{D E}=\frac{B C}{E F}\)

⇒ \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{C A}{F D}\)

Thus \(\angle A=\angle D, \angle B=\angle E, \angle C=\angle F\) and

⇒ \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{C A}{F D}\)

Hence, \(\triangle A B C \sim \triangle D E F\).

Corollary (AA Similarity): If two angles of a triangle are respectively equal to two angles of another triangle then the two triangles are similar.

Proof: In \(\triangle A B C\) and \(\triangle D E F\) , let \(\angle A=\angle D\) and \(\angle B=\angle E\) , then 3rd \(\angle C= 3rd \angle F\)

Thus, the two triangles are equal.

Hence, the two triangles are similar.

Thus, we can see that two triangles can be proved similar if two angles of 1st triangle are equal to two angles of the other triangle respectively.

Theorem 2 (SSS Similarity): If the corresponding sides of two triangles are proportional then their corresponding angles are equal, and hence the two triangles are similar.

Given: \(\triangle\)ABC and \(\triangle\)DFF in which

To Prove: \(\triangle A B C \sim \triangle D E F\)

Construction: Let us take \(\triangle\) A B C and \(\triangle\) D E F such that

⇒ \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{A C}{D F}\)

Cut D P=A B and D Q=A C. Join PQ

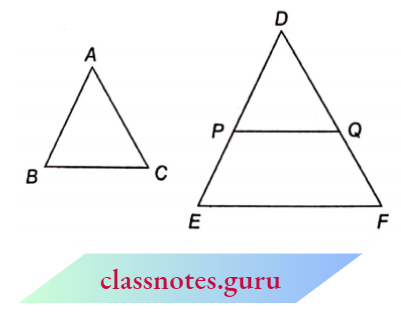
Proof: \(\frac{A B}{D E}=\frac{A C}{D F}\)

⇒ \(\frac{D P}{D E}=\frac{D Q}{D F}\)

Thus, the given triangles are equiangular and hence similar.

Theorem 3 (SAS Similarity): If one angle of a triangle is equal to one angle of the other triangle and the sides including these angles are proportional then the two triangles are similar.

Given: \(\triangle\)ABC and \(\triangle\)DPP in which

⇒ \(\angle A=\angle D and \frac{A B}{D E}=\frac{A C}{D F}\)

To Prove: \(\triangle \triangle B C \sim \triangle D E F\)

Construction: Let us take \(\triangle A B C\) and \(\triangle D E F\) such that

⇒ \(\frac{A B}{D E}=\frac{A C}{D F}\) and \(\angle A=\angle D\)

Cut D P=A B and D Q=A C. Join P Q.

Proof: In \(\triangle A B C\) and \(\triangle D P Q\)

A B=D P (by construction)

⇒ \(\angle A=\angle D\) (given)

A C=D Q (by construction)

⇒ \(\triangle A B C \cong \triangle D P Q\) (by SAS axiom)

⇒ \(\angle A=\angle D, \angle B=\angle P and \angle C=\angle Q \)

Now \(\frac{A B}{D E}=\frac{A C}{D F}\) (given)

⇒ \(\frac{D P}{D E}=\frac{D Q}{D F}\) (AB = DP and AC = DQ)

P Q || E F (by the converse of Thale’s theorem)

⇒ \(\angle P=\angle E and \angle Q=\angle F )\)

⇒ \(\angle P=\angle E\) and \(\angle Q=\angle F \)(corresponding angles)

⇒ \(\angle A=\angle D, \angle B=\angle P=\angle E \angle C=\angle Q=\angle F\)

Thus, \(\angle A=\angle D, \angle B=\angle E and \angle C=\angle F\)

So, the given triangles are equiangular and hence similar.

Some More Results

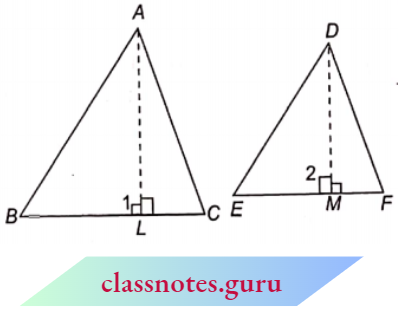
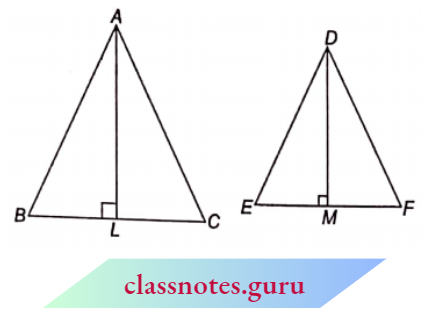
Result 1: If two triangles are equiangular, prove that the ratio of their corresponding sides is the same as the ratio of their corresponding altitudes.

Given : \(\triangle M B C\) and \(\triangle D E F \)in which \(\angle A=\angle D\), \(\angle B=\angle E\) and \(\angle C=\angle F\) and \(A L \perp B C\) and \(D M \perp E F\) :

To Prove : \(\frac{B C}{E F}=\frac{A L}{D M}\)

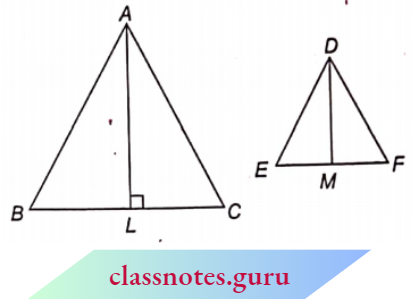
Proof : Since \(\triangle A B C\) and \(\triangle D E F\) are equiangular, therefore, \(\triangle A B C \sim \triangle D E F\)

⇒  \(\frac{A B}{D E}=\frac{B C}{E F}\)

In \(\triangle A L B\) and \(\triangle D M E\), we have

⇒ \(\angle A L B=\angle D M E=90^{\circ}\) and \(\angle B=\angle E\)

⇒ \(\triangle A L B \sim \triangle D M E\)

⇒ \(\frac{A B}{D E}=\frac{A L}{D M}\)

From (1), and (2) we get

⇒ \(\frac{B C}{E F}=\frac{A L}{D M}\)

Result 2: If two triangles are equal, prove that the ratio of their corresponding sides is the same as the ratio of the corresponding medians.

Given : \(\triangle\)ABC and \(\triangle\)DEF in which \(\angle\)A = \(\angle\)D, \(\angle\)B = \(\angle\)E and \(\angle\)C = \(\angle\)F and \(\angle\)L and \(\angle\)M are medians.

To Prove : \(\frac{B C}{E F}=\frac{A L}{D M}\)

Proof : Since \(\triangle A B C\) and \(\triangle D E F\) are equiangular, we have \(\triangle A B C \sim \triangle D E F\)

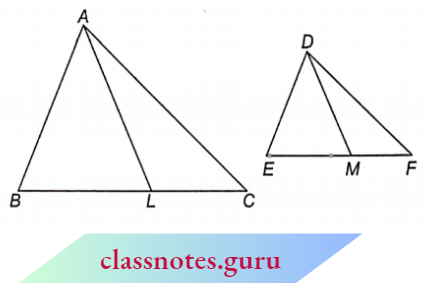
⇒ \(\frac{A B}{D E}=\frac{B C}{E F}\)

⇒ \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{2 B L}{2 E M}=\frac{B L}{E M}\)

But, \(\frac{A B}{D E} =\frac{B L}{E M}\) and \(\angle B=\angle E\)

Now, in \(\triangle A B L\) and \(\triangle D E M\), we have

⇒ \(\frac{A B}{D E}=\frac{B L}{E M}\) and \(\angle B=\angle E\) (given)

⇒ \(\triangle A B L \sim \triangle D E M\) (by SAS similarity)

⇒ \(\frac{A B}{D E} =\frac{A L}{D M}\) … 2

From 1 And 2, we get

∴ \(\frac{B C}{E F}=\frac{A L}{D M}\)

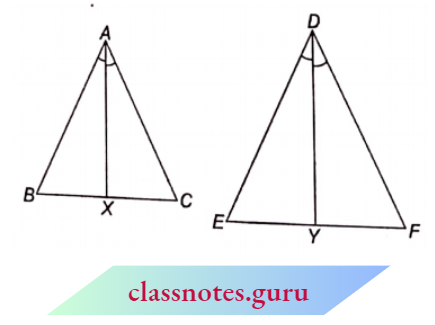
Result 3: If two triangles are equiangular, show that the ratio of the corresponding sides is the same as the ratio of the corresponding angle bisector segments.

Given : \(\triangle\)ABC and \(\triangle\)DEF in which \(\angle\)A = \(\angle\)D, \(\angle\)B = \(\angle\)E and A

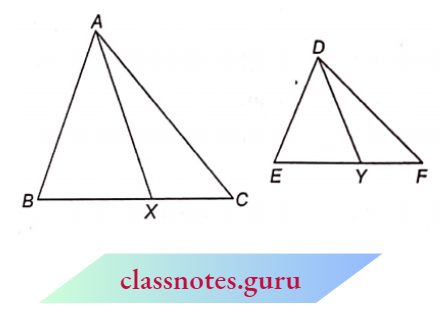
⇒ \(\angle\)C = \(\angle\)F and AX and DY are the bisectors of \(\angle\)A and \(\angle\)D
respectively.

To Prove: \(\frac{B C}{E F}=\frac{A X}{D Y}\)

Proof : Since \(\triangle\)ABC and \(\triangle\)DEF are equiangular, we have \(\triangle\)ABC ~ \(\triangle\)DEF

⇒ \(\frac{A B}{D E}=\frac{B C}{E F}\) …. 1

Now, \(\angle A=\angle D \Rightarrow \frac{1}{2} \angle A=\frac{1}{2} \angle D\)

⇒ \(\angle B A X=\angle E D Y\)

Thus in \(\triangle A B X\) and \(\triangle D E Y\), we have

⇒ \(\angle B A X =\angle E D Y\) (proved)

⇒ \(\angle B =\angle E\) (given)

⇒ \(\triangle A B X \sim \triangle D E Y\) (by AA similarity)

⇒ \(\frac{A B}{D E}=\frac{A X}{D Y}\) ….. 2

From (1) and (2), we get

⇒ \(\frac{B C}{E F}=\frac{A X}{D Y}\)

Remark:

1. If two triangles ABC and DEF are similar, then
2. \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{A C}{D F}\)
3. \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{A C}{D F}=\frac{A B+B C+A C}{D E+E F+D F}\) (using ratio and proportion)
4. \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{A C}{D F}=\frac{\text { Perimeter of } \triangle A B C}{\text { Perimeter of } \triangle D E F}\)
5. Thus, if two triangles are similar, then their corresponding sides are proportional and they are proportional to the corresponding perimeters.

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Traingle Importance Of Correct Namings

Then which of the following is correct?

•  \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A C}{P R}\).
• \(\frac{A B}{P R}=\frac{B C}{P Q}=\frac{A C}{Q R}\).
• \(\frac{A B}{Q R}=\frac{B C}{P Q}=\frac{A C}{P R}\)

You will say option (a) is correct, but your answer is wrong.

Until we do not know which part of one triangle is equal to which part of another triangle, how can we give the correct answer? We should give the name of the other triangle according to the first triangle. We must see the corresponding parts.

For Example,

Here , \(\angle A=\angle Q\)

⇒ \(\angle C=\angle P\)

⇒ \(\angle B=\angle R\)

Since two angles of \(\triangle\)ABC, are equal to two angles of \(\triangle\)PQR, therefore two triangles are similar to each other. For this, write the name of the first triangle say \(\triangle\)CAB. Now we have to write the name of the other triangle correspondingly, (according to \(\triangle\)CAB)

As C comes in 1st place and C = P, So  P will come in 1st place also

As A comes in 2nd place and A = Q, So Q will come in 2nd place

As the remaining place of first, A is for B so the remaining place of second A will be for R.

Corresponding sides are proportional.

⇒ \(\frac{\text { first two of } \triangle C A B}{\text { first two of } \Delta P Q R} =\frac{\text { last two of } \triangle C A B}{\text { last two of } \triangle P Q R}=\frac{\text { first and last of } \triangle C A B}{\text { first and last of } \triangle P Q R}\)

⇒ \(\frac{C A}{P Q} =\frac{A B}{Q R}=\frac{C B}{P R}\) (no need to see the figures, you can confuse there) …(1)

Also, you can write

1st angle of first \(\Delta\)=I angle of other \(\Delta\) i.e., \(\angle C=\angle P\)

2nd angle of first \(\Delta\)= 2nd angle of other \(\Delta\) i.e., \(\angle A=\angle Q \)

and 3rd angle of first \(\Delta\)= 3rd angle of other \(\Delta\) i.e. \(\angle B=\angle R\)

If we write the name of first \(\triangle\) as BCA then the name of other \(\triangle\) will be as follows (As \(\angle\)A = \(\angle\)Q => if A comes in 3rd place, so Q will also come in 3rd place and as \(\angle\)C = \(\angle\)P if C comes in 2nd places, so P will also come in 2nd place, etc.)

So, now for \(\triangle\)BCA.

(2) Then put

(3) Then finally (remaining place)

Hence, \(\triangle B C A-\triangle R P Q\)

The corresponding sides are proportional

⇒ \(\frac{\text { first two of } \triangle B C A}{\text { first two of } \triangle R P Q} =\frac{\text { last two of } \triangle B C A}{\text { last two of } \triangle R P Q}\)

= \(\frac{\text { first and last of } \triangle B C A}{\text { first and last of } \triangle R P Q}\)

B C =\(\frac{C A}{P Q}=\frac{B A}{R Q}\)

This is also the same as in (1)

Now, we shall see one example based on the above discussion

Example : D is a point on the side BC of a \(\triangle\)ABC, such that \(\angle\)ADC = \(\angle\)BAC.

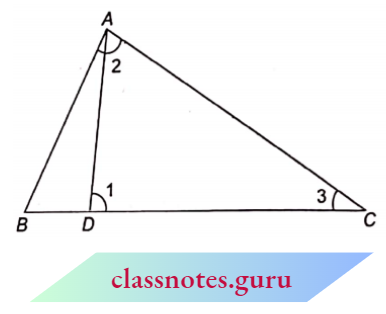
Prove that: \(\frac{C A}{C D}=\frac{C B}{C A}\)

Solution:

Don’t bother to write the correct namings of two triangles. We know that as \(\angle\)1 = \(\angle\)2 (given)

So we can take the As in which \(\angle\)1 and \(\angle\)2 occur i.e., \(tri\angle\)ADC and \(tri\angle\)ABC

Now, in \(tri\angle\)s ADC and ABC

⇒ \(\angle\)1 = \(\angle\)2

Now From We Can Write,

⇒ \(\triangle \mathrm{A} D \mathrm{~A} \sim \triangle B A C\)

Now, from this, we can write

⇒ \(\frac{\text { first two of } \triangle A D C}{\text { first two of } \triangle B A C}=\frac{\text { last two of } \triangle A D C}{\text { last two of } \triangle B A C}\)

=\(\frac{\text { first and last of } \triangle A D C}{\text { first and last of } \triangle B A C}\)

i.e., \(\frac{A D}{B A}=\underbrace{\frac{D C}{A C}=\frac{A C}{B C}}\)

or \(\frac{C A}{C D}=\frac{C B}{C A}\)

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Traingle Importance Of Correct Namings Solved Examples

Example 1. In the given figure \(\triangle\)ACB ~ \(\triangle\)APQ. If BC = 8 cm, PQ = 4 cm, BA = 6.5 cm, AP = 2.8 cm, find C/1 and AQ.

Solution:

Given

In the given figure \(\triangle\)ACB ~ \(\triangle\)APQ. If BC = 8 cm, PQ = 4 cm, BA = 6.5 cm, AP = 2.8 cm

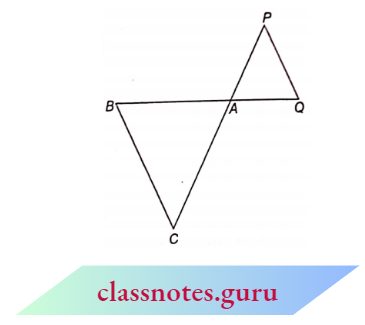
Since, \(\triangle\)ACB ~ \(\triangle\)APQ (given)

⇒ \(\frac{A C}{A P}=\frac{C B}{P Q}=\frac{A B}{A Q}\)

(don’t see the figure) write \(\frac{\text { first two }}{\text { first two }}=\frac{\text { last two }}{\text { last two }}=\frac{\text { first and last }}{\text { first and last }}\)

⇒ \(\frac{A C}{2.8}=\frac{8}{4}=\frac{6.5}{A Q}\)

Consider, \(\frac{A C}{2.8}=\frac{8}{4}\)

4 A C=8 \(\times 2.8 \quad \Rightarrow \quad A C=5.6 \mathrm{~cm}\)

Consider, \(\frac{8}{4}=\frac{6.5}{A Q}\)

2 A Q=6.5 or A Q=3.25 cm

Example 2. The triangles shown in adjoining figures are similar. Find the values of a and b.

Solution:

Given

The triangles shown in adjoining figures are similar.

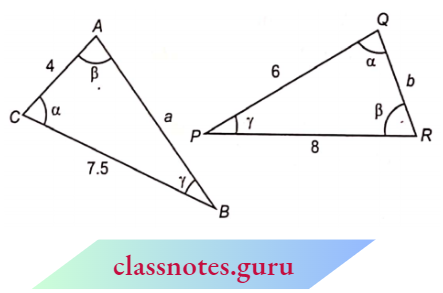
⇒ \(\angle A=\angle R, \angle B=\angle P\)and \(\angle C=\angle Q\)

⇒ \(\triangle A B C \sim \triangle R P Q\) (AAA similarity)

⇒ \(\frac{A B}{R P}\) … First Two

= \(\frac{B C}{P Q}\) ……. Last Two

= \(\frac{A C}{R Q}\) ….. First And Last

⇒ \(\frac{a}{8} =\frac{7.5}{6}=\frac{4}{b}\)

⇒ \(\frac{a}{8} =\frac{7.5}{6}\) and \(\frac{4}{b}=\frac{7.5}{6}\)

a =\(\frac{8 \times 7.5}{6}\) and \(b=\frac{4 \times 6}{7.5}\)

a =10 and b=3.2

Example 3. In the given figure if AD || BC, find the value of x.

Solution:

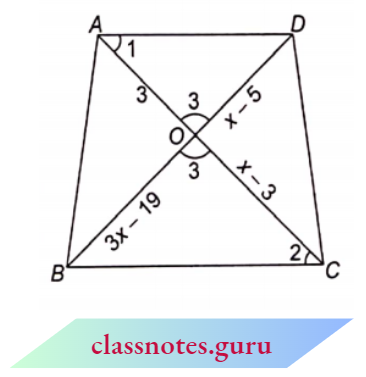
Given: AD || BC

Proof: In \(\triangle\)AOD and \(\triangle\)BOC

⇒ \(\angle 1=\angle 2\) (alternate angles)

⇒ \(\angle 3=\angle 3\) (vertically opposite angles)

i.e., \(\triangle A O D \sim \triangle C O B\) [AA corollary]

⇒ \(\frac{A O}{O C}=\frac{O D}{O B}\)

⇒ \(\frac{3}{x-3}=\frac{x-5}{3 x-19}\)

(x-3)(x-5)=3(3 x-19) \(\quad \Rightarrow \quad x^2-5 x-3 x+15=9 x-57\)

⇒ \(x^2-17 x+72=0 \quad \Rightarrow \quad x^2-9 x-8 x+72=0\)

x(x-9)-8(x-9)=0 or (x-9)(x-8)=0

x-9=0 or x=9

x-8=0 or x=8

Hence x=9,8

Example 4. Find ∠P in the figure below

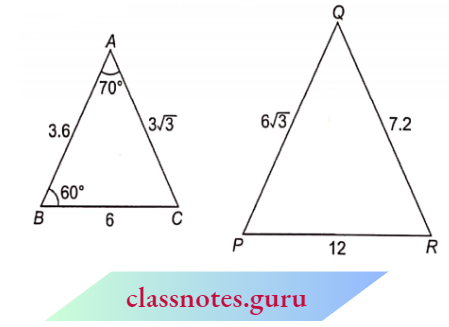
Solution:

In \(\triangle A B C\) and \(\triangle Q R P\), we have

⇒ \(\frac{A B}{Q R}=\frac{3.6}{7.2}=\frac{1}{2}, \frac{B C}{R P}=\frac{6}{12}=\frac{1}{2}\)

and \(\frac{C A}{P Q}=\frac{3 \sqrt{3}}{6 \sqrt{3}}=\frac{1}{2}\)

Thus,\(\frac{A B}{Q R}=\frac{B C}{R P}=\frac{C A}{P Q}\)

Hence, by SSS criterion,

⇒ \(\triangle A B C \sim \triangle Q R \quad\left(\quad \frac{\text { first two }}{\text { first two }}=\frac{\text { last two }}{\text { last two }}=\frac{\text { first and last }}{\text { first and last }}\right)\)

⇒ \(\angle C =\angle P\) (third angle = third angle)

But \(\angle C=180-(\angle A+\angle B)=180^{\circ}-\left(70^{\circ}+60^{\circ}\right)=50^{\circ}\)

⇒ \(\angle P=50^{\circ}\)

Example 5. In the figure AC || BD, prove that:

1. \(\triangle A C E \sim \triangle B D E\)
2. \(\frac{A E}{C E}=\frac{B E}{D E}\)

Solution:

(1) A C || B D (given)

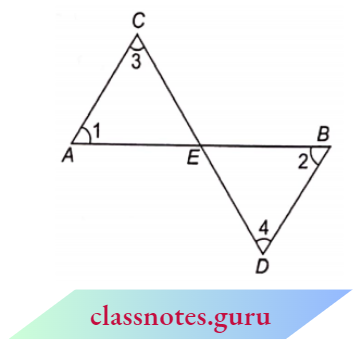
and, \(\left.\begin{array}{l}\angle 1=\angle 2 \\ \angle 3=\angle 4\end{array}\right\}\)

∴ \(\triangle \widehat{A C E \sim \triangle B D E}\) (by AA criterion)

(2) Now, since \(\triangle A C E \sim \triangle B D E\)

⇒ \(\frac{A E}{B E}=\frac{C E}{D E}\) (corresponding sides of similar \(\Delta\) s are proportional)

∴ \(\frac{A E}{C E}=\frac{B E}{D E}\) (by alternendo)

Example 6. \(\triangle\)ABC is an isosceles triangle with Ab = AC And D is a point on AC such that \(B C^2=A C \times C D\), Prove that BD = BC

Solution:

Given

\(\triangle\)ABC is an isosceles triangle with Ab = AC And D is a point on AC such that \(B C^2=A C \times C D\),

We have

⇒ \(B C^2 =A C \times C D\) and A B=A C

⇒ \(B C \times B C =A C \times C D\) and \(\angle B =\angle C\)

There may be 3 possibilities:

Possibility 1

⇒ \(\frac{B C}{A C}=\frac{C D}{B C}\)

and \(\angle C=\angle B\)

⇒ \(\triangle B \overline{D C} \sim \triangle A \overline{B C}\)

But in this case, \(\angle C \neq \angle B\)

So, the position will not suit.

Therefore, not possible.

Possibility 2.

⇒ \(\frac{B C}{C D}=\frac{A C}{B C}\)

⇒ \(\angle B=\angle C\)

⇒ \(\triangle \widehat{A B C} \sim \triangle \widehat{B D C}\)

But in this case, \(\angle B \neq \angle C\)

So, the position will not suit. Therefore, not possible.

Possibility 3.

⇒ \(\frac{B C}{A B} =\frac{C D}{B C}\)( A B=A C)

⇒ \(\angle C =\angle B \)

⇒ \(\triangle \overline{B C} D \sim \Delta \overline{A B} C\)

All positions are satisfied.

So, possible.

⇒ \(\triangle B C D \sim \triangle A B C\) (by SAS criterion)

⇒ \(\frac{B C}{A B} =\frac{C D}{B C}=\frac{B D}{A C}\)

⇒ \(\frac{B C}{A B} =\frac{B D}{A C}\)

BC =B D (A B=A C, given)

Hence Proved.

We could take directly the 3rd possibility and the solution seems to be much easier but our main aim is to make you understand why we take the 3rd one.

Example 7. In the given figure if \(\angle B=90^{\circ}\) and B D is perpendicular to A C then prove that :

1.  \(\triangle A D B \sim \triangle B D C\)
2. \(\triangle A D B \sim \triangle A B C\)
3.  \(\triangle B D C \sim \triangle A B C\)
4. \(B D^2=A D \times D C\)
5.  \(A B^2=A D \times A C\)
6. \(B C^2=C D \times A C\)
7.  \(A B^2+B C^2=A C^2\)

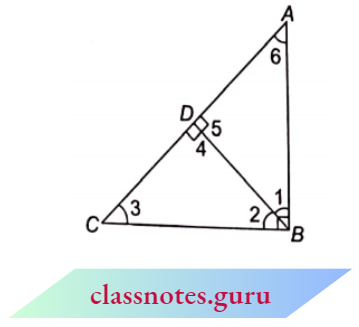
Solution:

(1) In \(\triangle A D B\) and \(\triangle B D C\),

⇒ \(\angle 5=\angle 4 (each 90^{\circ}\) )

Searching for Second Angle :

We know that \(\angle 1+\angle 2=90^{\circ}\)

Also, since \(\angle 4=90^{\circ}\)

⇒ \(\angle 2+\angle 3=90^{\circ}\) (angle sum property)

From (1) and (2), we get

⇒ \(\angle 1+\angle 2=\angle 2+\angle 3 \quad\left(\text { each } 90^{\circ}\right)\)

⇒ \(\angle 1=\angle 3\)

Now, in \(\triangle A D B\) and \(\triangle B D C\),

⇒ \(\angle 5=\angle 4\) (each \(90^{\circ}\) )

⇒ \(\angle 1=\angle 3\) (just proved)

⇒ \(\triangle A D B \sim \triangle B D C\) (AA corollary)

Hence Proved.

(2) In \(\triangle A D B\) and \(\triangle A B C\),

⇒\(\angle 5=\angle 1+\angle 2\)

\(\angle 6=\angle 6\)

⇒ \(\left(\right. each \left.90^{\circ}\right)\)

∴ \(\triangle \widehat{D B \sim \triangle A B C}\) (common)

(3) In \(\triangle B D C\) and \(\triangle A B C\),

⇒ \(\angle 4 =\angle 1+\angle 2 (each 90^{\circ} )\)

⇒ \(\angle 3 =\angle 3\) (common)

⇒ \(\triangle B D C \sim \triangle A B C\) (AA corollary)

Hence Proved.

(4) For \(B D^2=A D \times D C\), we need to prove two \(\Delta s\) similar which contain \(B D, A D\) and D C as sides.

Obviously, these are \(\triangle A B D\) and \(\triangle B D C\), we have proved these \(\triangle s\) as similar in part (1).

So, \(\Delta \underline{A \overline{D B}} \sim \Delta \underline{B} \bar{D} C\) [from part (1)]

⇒ \(\underbrace{\frac{A D}{B D}=\frac{D B}{D C}}_{\text {Taking first two }}=\frac{A B}{B C}\) (corresponding sides of similar triangles are proportional)

⇒ \(B D^2=A D \times D C\)

Hence Proved.

(5) For \(A B^2=A D \times A C\), we need to prove two triangles similar which contain AB, AD and AC as sides. Obviously, these are AABD and AABC. We have already proved these As as similar in part (2)

⇒ \(\triangle A D B \sim \triangle A B C\) [from part (2)]

⇒ \(\frac{A D}{A B}=\frac{D B}{B C}=\frac{A B}{A C}\) (corresponding sides of similar }

⇒ \(A B^2=A D \times A C\) …(1) Hence Proved.

So,\(\Delta \widehat{B D C} \sim \triangle \widehat{A B C}\) [from part (3)]

⇒ \(\frac{B D}{A B}=\frac{D C}{B C}=\frac{B C}{A C}\) (corresponding sides of similar triangles are proportional)

⇒ \(B C^2=C D \times\) A C … 2

Hence Proved.

(7) Adding results (1) and (2), we get

⇒ \(A B^2+B C^2 =A D \times A C+C D \times A C\)

= A C(A D+C D)

= \(A C \times A C=A C^2\)

Hence Proved.

Example 8. In the given figure, DEFG is a square, and ABAC = 90°. Prove that

1. \(\triangle\)AGF ~ \(\triangle\)DBG
2.  \(\triangle\)AGF-\(\triangle\)EFC
3.  \(\triangle\)DBG – \(\triangle\)EFC
4.  \(DE^2\) = BD x EC

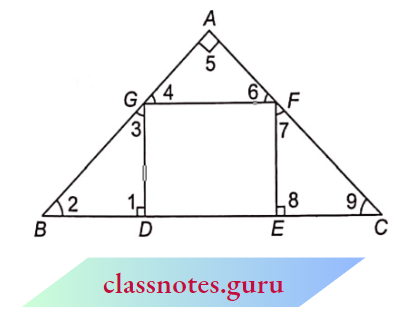
Solution:

(1) Since Square DEFG is a square

GF || BC

⇒ \(\angle 2=\angle 4\) and \(\angle 6=\angle 9\) (corresponding \(\angle s\) )

Now, in \(\triangle A G F\) and \(\triangle D B G\),

⇒ \(\angle 5\) = \(\angle 1\)

⇒ \(\angle 4 =\angle 2\)

⇒ \(\triangle A G F \sim \triangle D B G\) (each \(90^{\circ}\) )

⇒ \(\angle 5=\angle 8\)

⇒ \(\angle 4=\angle 2\) (corresponding \(\angle \mathrm{s}\) )

⇒ \(\triangle A G F \sim \triangle D B G\) (AA corollary)

Hence Proved.

(2) In \(\triangle A G F\) and \(\triangle E F C\),

⇒ \(\angle 5=\angle 8\) (each \(90^{\circ}\) )

⇒ \(\angle 6=\angle 9\) (corresponding \(\angle s\) )

⇒ \(\triangle A G F \sim \triangle E F C\)(AA corollary)

Since \(\triangle A G F \sim \triangle D B G\) [proved in (1)]

and \(\triangle A G F \sim \triangle E F C\) [proved in (2)]

⇒ \(\triangle A G F \sim \triangle D B G \sim \triangle E F C\)

Hence Proved.

(4) Now, since \(\triangle \widehat{D B G} \sim \triangle \overparen{E F C}\)

⇒ \(\frac{D B}{E F}=\frac{D G}{E C}\) (corresponding \(\Delta s\) are proportional)

DG \(\times E F=B D \times E C\)

D E \(\times D E=B D \times E C\)( DG = FE = DE being the sides of square)

⇒ \(D E^2=B D \times E C\)

If we have to prove only the fourth part i.e., prove that \(DE^2\) = BD x EC, then no need to prove the first two parts.

For \(DE^2\) = BD x EC, we need to prove two As similar which contain DE, BD, and EC.

Obviously, these are \(\triangle G B D\) and \(\triangle F E C\).

So, \(\angle I=\angle 8 (each .90^{\circ})\)

Searching for Second Angle :

As we know that \(\angle 5=90^{\circ}\)

⇒ \(\angle 2+\angle 9=90^{\circ}\) (angle sum property)

Also since \(\angle 1=90^{\circ}\)

⇒ \(\angle 2+\angle 3=90^{\circ}\) (angle sum property)

From (1) and (2),

⇒ \(\angle 2+\angle 3=\angle 2+\angle 9\)

⇒ \(\angle 3=\angle 9\)

Now, in \(\triangle G B\) and \(\triangle F E C\),

⇒ \(\angle 1=\angle 8\) (each \(90^{\circ}\) )

⇒ \(\angle 3=\angle 9\) (just proved)

⇒ \(\frac{D B}{E F}=\frac{B G}{F C}=\frac{D G}{E C}\)

Taking the first and last

⇒ \(\triangle D B G \sim \triangle E F C\) (AA corollary)

⇒ \(\frac{D B}{E F}=\frac{B G}{F C}=\frac{D G}{E C}\) (corresponding sides of similar)

⇒ \(D G \times E F=B D \times E C\)

⇒ \(D E \times D E=B D \times E C\) ( D G=E F=D E, sides of a square)

⇒ \(D E^2=B D \times E C\)

Hence Proved.

Example 9. In the adjoining Figure, if a = 18,b = 12, c = 14, and d = 8, what is the measure of x?

Solution:

Given

In the adjoining Figure, if a = 18,b = 12, c = 14, and d = 8

We have, \(\frac{P R}{Q R}=\frac{12}{18}=\frac{2}{3}\) …… 1

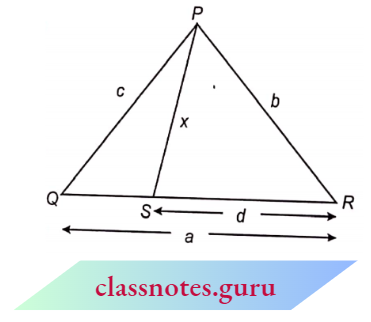
⇒ \(\frac{S R}{P R}=\frac{8}{12}=\frac{2}{3}\) …… 2

Now in \(\triangle P R S\) and \(\triangle Q R P\),

Since, \(\frac{P R}{Q R}=\frac{S R}{P R}\) [from (1) and (2)]

⇒ \(\angle R=\angle R\)

⇒ \(\Delta P \widehat{R S}-\triangle Q \overparen{R P}\) (common)

⇒ \(\frac{P R}{Q R}=\frac{S R}{P R}=\frac{P S}{Q P}\) (SAS similarity)

⇒ \(\frac{2}{3}=\frac{2}{3}=\frac{x}{14}\)

∴\(\frac{x}{14}=\frac{2}{3} \quad \Rightarrow \quad x=\frac{28}{3}\)

Example 10. In the adjoining figure, AB || CD || EF. Prove that \(\frac{1}{x}+\frac{1}{y}=\frac{1}{z}\).

Solution:

In \(triangle\) BFE and \(\triangle\) BDC

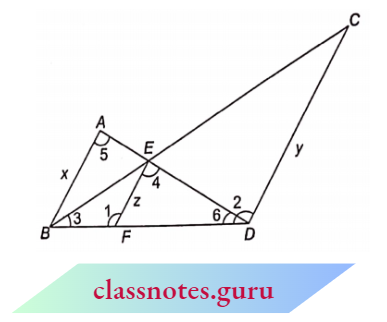
⇒ \(\angle 1=\angle 2\) (corresponding \(\angle\) s as E F || C D )

⇒ \(\angle 3=\angle 3\) (common)

⇒ \(\underline{B F E} \sim \triangle B C\) (AA corollary)

⇒ \(\frac{B F}{B D}=\frac{F E}{D C}\)

⇒ \(\frac{B F}{B D}=\frac{z}{y}\) (corresponding sides of similar triangles are proportional)

In \(\triangle D F E\) and \(\triangle D B A\),

⇒ \(\angle 4=\angle 5\) (corresponding \(\angle \)s as E F || A B )

⇒ \(\angle 6=\angle 6\) (common)

⇒ \(\triangle D F E \sim \triangle D B A\) (AA corollary)

⇒ \(\frac{D F}{D D}=\frac{F E}{D A}\) (Corresponding Sides Of Similar Triangles Are Proportional)

⇒ \(\frac{D F}{D B}=\frac{z}{x}\)

Remember, the common part in (1) and (2) should be in the denominator so that we can add easily) Adding (1) and (2), we get

⇒ \(\frac{B F}{B D}+\frac{D F}{D B} =\frac{z}{y}+\frac{z}{x}\)

⇒ \(\frac{B F+D F}{B D} =z\left(\frac{1}{y}+\frac{1}{x}\right)\)

⇒ \(\frac{B D}{B D} =z\left(\frac{1}{x}+\frac{1}{y}\right) \quad \Rightarrow \quad 1=z\left(\frac{1}{x}+\frac{1}{y}\right)\)

∴  \(\frac{1}{z} =\frac{1}{x}+\frac{1}{y}\)

Example 11. Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn, intersecting AC in L and AD produced in E. Prove that EL = 2BL.

Solution:

Given

Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn, intersecting AC in L and AD produced in E.

In \(\triangle\)s BMC and DME,

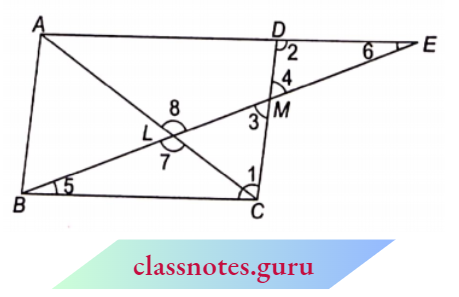
⇒ \(\angle 1 =\angle 2\) (alternate \(\angle\)s as B C || D E)

C M =D M (M is the mid-point of DC)

⇒ \(\angle 3 =\angle 4\) (vertically opposite angles)

⇒ \(\triangle B M C \simeq \triangle E M D)\)

BC = E D (corresponding parts of the congruent triangle)….. 1

But, BC = A D (opposite sides of a parallelogram)…… 2

Adding (1) and (2), we get

2 BC = D E+A D \(\Rightarrow 2 B C = A E\)

⇒ \(\frac{B C}{A E} =\frac{1}{2}\)

Now in \(\triangle B C L\) and \(\triangle E A L\)

⇒\(\angle 5 =\angle 6\)

⇒ \(\angle 7 =\angle 8\) (vertically opposite angles)

⇒ (\(\angle\)s as B C || A E )

⇒ \(\triangle B C L \sim \triangle E A L\) (AA Collary)

⇒ \(\frac{B C}{E A}=\frac{B L}{E L}\) (corresponding sides of similar triangles are proportional) ….(4)

⇒ \(\frac{1}{2}=\frac{B L}{E L}\) [ from (3) and (4)]

EL = 2BL

Hence Proved.

Example 12. The perimeters of two similar triangles are 25 cm and 15 cm respectively. If one side of the first triangle is 9 cm. Find the corresponding side of the second triangle.

Solution:

Given

The perimeters of two similar triangles are 25 cm and 15 cm respectively. If one side of the first triangle is 9 cm.

Let AB = 9cm

Since \(\triangle A B C \sim \triangle P Q R\) (given)

⇒ \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A C}{P R}=k\) corresponding sides of similar , triangles are proportional

AB= k. PQ, BC = k. QR, AC = k. PR

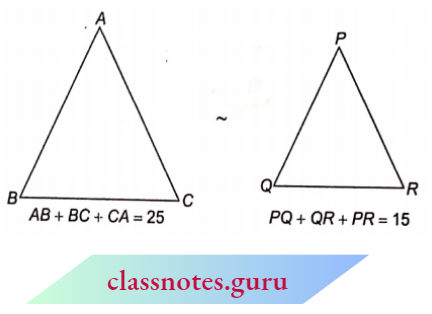
⇒ \(\frac{\text { Perimeter of } \triangle A B C}{\text { Perimeter of } \triangle P Q R} =\frac{A B+B C+C A}{P Q+Q R+R P}\)

=\(\frac{k \cdot P Q+k \cdot Q R+k \cdot P R}{P Q+Q R+R P}\)

=\(\frac{k(P Q+Q R+R P)}{(P Q+Q R+R P)}\) = k

From (1) and (2), we get

⇒ \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A C}{P R}=\frac{\text { Perimeter }(\triangle A B C)}{\text { Perimeter }(\triangle P Q R)}\)

⇒ \(\frac{9}{P Q}=\frac{25}{15} \Rightarrow P Q=\frac{9 \times 15}{25}\)=5.4

Hence, the corresponding side of the second triangle is 5.4 cm.

Example 13. A lamp is 3.3 m above ground. A boy I Hi em tall walks away from (be base of (bis lamp post at a speed of 0.8 m/s. Find (be the length of (be a shadow of the boy after 4 seconds.

Solution:

Given

A lamp is 3.3 m above ground. A boy I Hi em tall walks away from (be base of (bis lamp post at a speed of 0.8 m/s.

Let AB = 3.3 m be the lamp post and CD = 1.1 m be the position of the boy after 4 seconds. Also, let the shadow of the boy after 4 see = x m

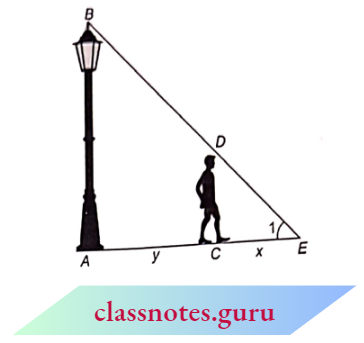
Distance travelled by boy in 4 sec =y = 0.8 x 4 = 3.2 m

Now, in \(\triangle\)AEB and \(\triangle\)CED

⇒\(\angle E A B=\angle E C D=90^{\circ}\) (obvious)

⇒ \(\angle 1=\angle 1\) (common)

⇒ \(\triangle A E B \sim \triangle C E D\) (AA corollary)

⇒ \(\triangle A E B \sim \triangle C E D\)

⇒  –\(\frac{A E}{C E}=\frac{A B}{C D}\) (corresponding sides of similar triangles are proportional)

⇒ \(\frac{x+y}{x}=\frac{3.3}{1.1}\) (110 cm =1.1 m)

x+y=3 x \(\quad \Rightarrow y=2 x\)

x=\(\frac{y}{2}=\frac{3.2}{2}=1.6\)

Hence, the length of the shadow of the boy after 4 seconds is 1.6 m

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Ratio Of The Areas Of Two Similar Triangles

Theorem: The ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.

Given : \(\triangle A B C \sim \triangle D E F\)

To Prove : \(\frac{{ar}(\triangle A B C)}{{ar}(\triangle D E F)}=\frac{A B^2}{D E^2}=\frac{A C^2}{\dot{D F}}=\frac{B C^2}{E F^2}\)

Construction : Draw A L \(\perp B C\) and D M \(\perp E F\)

Proof : Consider,\(\frac{{ar}(\triangle A B C)}{{ar}(\triangle D E F)}=\frac{\frac{1}{2} \times B C \times A L}{\frac{1}{2} \times E F \times D M}=\left(\frac{B C}{E F}\right) \cdot\left(\frac{A L}{D M}\right)\) Equation 1

Now, since \(\triangle A B C \sim \triangle D E F\)

⇒ \(\angle B=\angle E\)(corresponding angles of similar triangles are equal)

In \(\triangle A L B\) and \(\triangle D M E\),

⇒ \(\angle B=\angle E\) [ from (2)]

⇒ \(\angle 1=\angle 2\) (each \(90^{\circ}\), by construction)

⇒ \(\triangle A L B \sim \triangle D M E\)(AA corollary)

⇒ \(\frac{A B}{D E}=\frac{A L}{D M}=\frac{B L}{E M}\) (corresponding sides of similar triangles are proportional)

But \(\frac{A B}{D E}=\frac{B C}{E F}=\frac{A C}{D F}(\triangle A B C \sim \triangle D E F)\)

From (3) and (4), we have

⇒ \(\frac{A L}{D M}=\frac{B C}{E F}\)

From (1) and (5), we have

⇒ \(\frac{{ar}(\triangle A B C)}{{ar}(\triangle D E F)}=\frac{B C}{E F} \times \frac{B C}{E F}=\frac{B C^2}{E F^2}\)

=\(\frac{A B^2}{D E^2}\)

=\(\frac{A C^2}{D F^2}\) From 4

Hence, \(\frac{{ar}(\triangle A B C)}{{ar}(\triangle D E F)}=\frac{A B^2}{D E^2}=\frac{B C^2}{E F^2}=\frac{A C^2}{D F^2}\)

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Ratio Of The Areas Of Two Similar Triangles Solved Examples

Example 1. In figures \(\triangle\)ABC and \(\triangle\)DEF are similar, the areas of 4 MBC is 9 sq. cm and that of ADEF is 16 sq. cm. If EF = 4.2 cm, find BC.

Solution:

Given

In figures \(\triangle\)ABC and \(\triangle\)DEF are similar, the areas of 4 MBC is 9 sq. cm and that of ADEF is 16 sq. cm. If EF = 4.2 cm

ar(\(\triangle\)ABC) = 9 sq. cm

ar(\(\triangle\)DEF) = 16 sq. cm

EF= 4.2 cm

BC= ?

Since the ratio of areas of two similar triangles is equal to the ratio of squares on their corresponding sides

⇒ \(\frac{{ar}(\triangle A B C)}{{ar}(\triangle D E F)}=\frac{B C^2}{E F^2} \quad \Rightarrow \quad \frac{9}{16}=\left(\frac{B C}{E F}\right)^2 \)

⇒ \(\frac{B C}{4.2}=\frac{3}{4} \quad \Rightarrow \quad B C=\frac{3 \times 4.2}{4}=3.15 \mathrm{~cm}\)

Example 2. In a trapezium ABCD, O is the point of intersection of AC and BD, AB || CD, and AB = 2 x CD. If the area of \(\triangle\)AOB = 84 cm², find the area of \(\triangle\)COD.

Solution:

Given

In a trapezium ABCD, O is the point of intersection of AC and BD, AB || CD, and AB = 2 x CD. If the area of \(\triangle\)AOB = 84 cm²,

In \(\triangle AOB\) and \(\triangle COD\),

⇒ \(\angle 1 =\angle 2\) (alternate angles)

⇒ \(\angle 3 =\angle 4\) (alternate angles)

⇒ \(\Delta A O B \sim \triangle C O D\) (AA corollary)

⇒ \(\frac{{ar}(\triangle A O B)}{{ar}(\triangle C O D)} =\frac{A B^2}{C D^2}\)

(The ratio of the area of two similar triangles is equal to the ratio of the square of the corresponding sides)

= \(\frac{(2 C D)^2}{C D^2}\)

= \(\frac{4}{1}\) ( A B=2 C D, given )

∴ \(\frac{84}{{ar}(\triangle C O D)}=\frac{4}{1} \quad \Rightarrow \quad {ar}(\triangle C O D)=\frac{84}{4} \mathrm{~cm}^2=21 \mathrm{~cm}^2\)

Example 3. In the figure, DE || BC and the ratio of the areas of \(\triangle\) and trapezium BDEC is 4: 5. Find the ratio of DE: BC. If BD=2cm, Then find AD

Solution:

Given

In the figure, DE || BC and the ratio of the areas of \(\triangle\) and trapezium BDEC is 4: 5. Find the ratio of DE: BC. If BD=2cm

In \(\triangle A D E\) and \(\triangle A B C\).

⇒ \(\angle 1=\angle 2\) (corresponding angles as } \(D E \mid B C \)

⇒ \(\angle A=\angle A\) (common)

⇒ \(\triangle A D E-\triangle A B C\)

⇒ \(\frac{a r \Delta A D E}{{ar}(A B C}=\frac{A D^2}{A B^2}=\frac{D E^2}{B C^2}=\frac{A E^2}{A C^2}\) (AA corollary)(ratio of areas of two similar triangles is equal to the ratio of squares of their corresponding sides)

Now;\(\frac{{ar}(\text { trapezium } B D E C)}{{ar}(\Delta+D E)}=\frac{5}{4}\)

⇒ \(\frac{{ar}(\Delta+B C)-{ar}(\Delta+D E)}{{ar}(\Delta+D E)}=\frac{5}{4}\)

⇒ \(\frac{{ar}(\Delta+B C)}{{ar}(\Delta+D E)}-1=\frac{5}{4} \quad \Rightarrow \quad \frac{{ar}(\Delta A B C)}{{ar}(\Delta A D E)}=\frac{9}{4}\)

⇒ \(\frac{{ar}(\Delta+D E)}{{ar}(\Delta+B C)}=\frac{4}{9}\)

⇒ \(\frac{D E^2}{B C^2} =\frac{4}{9}\)

⇒ \(\frac{D E}{B C} =\frac{2}{3}\)

D E: B C =2: 3 from (1) and (2)

Now, from (1) and (2), we get

⇒ \(\frac{{ar}(\Delta A D E)}{{ar}(\Delta A B C)} =\frac{A D^2}{A B^2} =\frac{4}{9}\)

⇒ \(\frac{A D}{A B} =\frac{2}{3}\Rightarrow \frac{A D}{A D+B D}=\frac{2}{3}\)

⇒ \(\frac{A D+B D}{A D} =\frac{3}{2} \Rightarrow 1+\frac{B D}{A D}=\frac{3}{2}\)

⇒ \(\frac{B D}{A D} =\frac{3}{2}-1 =\frac{1}{2}\)

∴ \(\frac{2}{A D} =\frac{1}{2} \Rightarrow A D=4 \mathrm{~cm}\)

Example 4. X and Y are points on the sides AB and BC respectively of \(\triangle\) ABC such that XY || AC and XY divides AABC into two parts equal in area. Find \(\frac{A X}{A B}\)

Solution:

Given

X and Y are points on the sides AB and BC respectively of \(\triangle\) ABC such that XY || AC and XY divides AABC into two parts equal in area.

Since X Y || A C

In \(\triangle\) BXY and \(\triangle BAC\),

⇒ \(\angle 1=\angle 2\) (corresponding angles)

⇒ \(\angle B=\angle B\) (common)

⇒ \(\triangle B X Y \sim \triangle B A C\) (AA corollary)

⇒ \(\frac{{ar}(\Delta B X Y)}{{ar}(\triangle B A C)}=\frac{B X^2}{B A^2}\) (area ratio theorem)

But \({ar}(\triangle B X Y)=\frac{1}{2} {ar}(\triangle A B C)\) (given)

⇒ \(\frac{{ar}(\triangle B X Y)}{{ar}(\triangle A B C)}=\frac{1}{2}\)

From (1) and (2), we get

⇒ \(\frac{B X^2}{A B^2}=\frac{1}{2} \Rightarrow \frac{B X}{A B}=\frac{1}{\sqrt{2}}\)

⇒ \(\frac{A B-A X}{A B}=\frac{1}{\sqrt{2}} \Rightarrow \frac{A B}{A B}-\frac{A X}{A B}=\frac{1}{\sqrt{2}}\)

⇒ \(1-\frac{A X}{A B}=\frac{1}{\sqrt{2}} \Rightarrow \frac{A X}{A B}=1-\frac{1}{\sqrt{2}}=\frac{\sqrt{2}-1}{\sqrt{2}}\)

Hence, \(\frac{A X}{A B}=\frac{2-\sqrt{2}}{2}\)

Example 5. CE and DE are equal chords of a circle with centre O. If \(\angle A O B\) = 90°, find \(\text{ar}(\triangle C E D): \text{ar}(\triangle A O B)\).

Solution:

Given

CE and DE are equal chords of a circle with centre O. If \(\angle A O B\) = 90°

Since O is the centre of the circle.

⇒ \(\angle\)E = 90° (angle in a semicircle is right angle)

\(\angle\)I = \(\angle\)2 = 45°

(angles opposite to equal sides are equal as CE = DE)

Also, since Z\(\angle\)AOB = 90° (given)

⇒ \(\angle\)3 = \(\angle\)4 = 45°

(angles opposite to equal sides are equal as OA = OB, each radius) Now, in \(\triangle\)CED and \(\triangle\)AOB

⇒ \(\angle E =\angle A O B\)

⇒ \(\angle \mathrm{l}=\angle 3\)

⇒ \(\triangle C E D \sim \triangle A O B\)

⇒ \(\frac{\text{ar}(\triangle C E D)}{\text{ar}(\triangle A O B)}=\frac{C D^2}{A B^2}\)

= \(\frac{(2 O B)^2}{O A^2+O B^2}\)

(CD = diameter and OB = radius so, CD = 2 • OB and using Pythagoras theorem)

= \(\frac{4 O B^2}{O B^2+O B^2}\) (OA = OB, each radius)

= \(\frac{4 O B^2}{2 O B^2}=\frac{2}{1}\)

Hence, \(\frac{\text{ar}(\triangle C E D)}{\text{ar}(\triangle \triangle O B)}=\frac{2}{1}\)

Example 6. Prove that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding altitudes.

Solution:

Given: \(\triangle A B C \sim \triangle D E F, A L \perp B C\) and \(D M \perp E F\)

To Prove: \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle D E F)}=\frac{A L^2}{D M^2}\)

Proof: \(\ln \triangle A L B\) and \(\triangle D M E\),

(because the ratio of areas of two similar triangles is equal to the ratio of squares of the corresponding sides

From (1) and (2) we have \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle D E F)}=\frac{A L^2}{D M^2}\)

Hence Proved.

Example 7. Prove that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding angle bisector segments.
Solution:

Given: \(\triangle\)ABC ~ \(\triangle\)DEF in which AX and DY are the bisectors of \(\angle\)A and \(\angle\)D respectively.

To Prove: \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle D E F)}=\frac{A B^2}{D E^2}\)

Proof: Given \(\triangle A B C \sim \triangle D E F\)

Therefore, \(\angle A =\angle D\)

⇒ \(\frac{1}{2} \angle A=\frac{1}{2} \angle D\)

⇒ \(\angle B A X=\angle E D Y\)

Now, in \(\triangle A B X\) and \(\triangle D E Y\), we have

⇒ \(\angle B A X=\angle E D Y\)

and \(\angle B =\angle E\)

⇒ \(\triangle A B X \sim \triangle D E Y\)

Hence \(\frac{A B}{D E}=\frac{A X}{D Y}\)…..(1) given

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Pythagoras Theorem

Theorem 1: In a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

Given: A right-angled triangle ABC in which \(\angle\)B = 90°.

To Prove: \((Hypotenuse^2=(\text { Base })^2+ Perpendicular)^2\)

i.e., \(A C^2=A B^2+B C^2\)

Construction: From B, draw \(B D \perp A C\)

Proof: In \(\triangle A D B\) and \(\triangle A B C\)

⇒ \(\angle 1=\angle 2\)

and \(\angle A =\angle A\) (common)

Hence, \(\triangle M D B \sim \triangle A B C\) (by AA corollary)

⇒ \(\frac{A D}{A B} =\frac{A B}{A C}\) (corresponding sides of similar triangle are proportional)

⇒ \(A B^2=A D \times A C\)……(1)

Now in \(\triangle B D C\) and \(\triangle A B C\)

⇒ \(\angle 5=\angle 2\) (each 90^{\circ}[/latex])

and \(\angle C=\angle C\) (common)

Hence, \(\triangle B D C \sim \triangle A B C\) (by AA corollary)

⇒ \(\frac{D C}{B C}=\frac{B C}{A C}\) (corresponding sides of similar triangles are proportional)

⇒ \(B C^2=A C \times D C\)…… (2)

Adding (1) and (2), we get

⇒ \(A B^2+B C^2=A D \times A C+A C \times D C\)

⇒ \(A B^2+B C^2=A C(A D+D C)\)

⇒ \(A B^2+B C^2=A C \times A C\)(because A D+D C=A C)

⇒ \(A B^2+B C^2=A C^2\)

Theorem 2 (Converse of Pythagoras Theorem): In a triangle, if the square of one side is equal to the sum of squares of the other two sides, then the angle opposite to the longest side is a right angle.

Given: A triangle ABC such that \(A C^2=A B^2+B C^2\)

To Prove: \(\angle\)B = 90°

Construction: Construct a triangle DEF such that DE =AB, EF = BC and \(\angle\)E = 90°. Join DF.

Proof: In right \(\triangle\)DEF

⇒ \(D F^2=D E^2+E F^2\) (by Pythagoras theorem)

⇒ \(D F^2=A B^2+B C^2\) (given DE = AB and Ef = BC)

⇒ \(D F^2=A C^2\) (given \(A C^2=A B^2+B C^2\))

DF = AC

Now, in \(\triangle A B C\) and \(\triangle D E F\)

AB = DE (by construction)

BC=EF (by construction)

AC = DF from (1)

⇒ \(\triangle A B C \cong \triangle D E F\)

Hence \(\angle E=\angle B=90^{\circ}\)

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Some Important Results Based Uponpythagoras Theorem

Result 1: In an obtuse-angled triangle ABC, obtuse-angled at B, if \(A D \perp C B\) (produced), prove that \(A C^2=A B^2+B C^2\) + 2BC x BD.

Given: An obtuse triangle ABC, obtuse-angled at B and AD is perpendicular to CB produced.

To Prove: \(A C^2=A B^2+B C^2\) + 2BC x BD

Proof: Since \(\triangle\)ADB is right angled triangle therefore by Pythagoras’ theorem in \(\triangle\)ADC

⇒ \(A C^2=A D^2+D C^2\)

⇒ \(A C^2=A D^2+(D B+B C)^2\) (because DC=D B+BC)

⇒ \(A C^2=A D^2+D B^2+B C^2+2 D B \cdot B C\)

⇒ \(A C^2=\left(A D^2+D B^2\right)+B C^2+2 B C \cdot B D\)

⇒ \(A C^2=A B^2+B C^2+2 B C \cdot B D\)

Result 2: In an acute-angled triangle ABC, if \(A D \perp B C\), prove that: \(A C^2=A B^2+B C^2-2 B C \times B D\)

Given: \(\triangle A B C\) which is an acute-angled triangle and \(A D \perp B C\)

To Prove: \(A C^2=A B^2+B C^2-2 B C \times B D\)

Proof: In \(\triangle A D C, \angle D=90^{\circ}\), hence by Pythagoras theorem

⇒ \(A C^2=A D^2+D C^2\)

⇒ \(A C^2=A D^2+(B C-B D)^2\) ( D C=B C-B D)

⇒ \(A C^2=A D^2+B C^2+B D^2-2 B C \times B D\)

⇒ \(A C^2=\left(A D^2+B D^2\right)+B C^2-2 B C \times B D\)

⇒ \(A C^2=A B^2+B C^2-2 B C \times B D\) (because \(A D^2+B D^2=A B^2\))

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Pythagoras Theorem Solved Examples

Example 1. The sides of a triangle are 5 cm, 8 cm and 11 cm respectively. Determine whether it is a right-angled triangle or not.
Solution:

Given

The sides of a triangle are 5 cm, 8 cm and 11 cm respectively.

5 = 25

8 = 64

and 11 = 121

We find that 1 = 5 + 8

Hence, it is not a right-angled triangle.

Example 2. A ladder 25 m long reaches a window of a building 20 m above the ground. Determine the distance of the foot of the ladder from the building.
Solution:

Given

A ladder 25 m long reaches a window of a building 20 m above the ground.

Let AC = 20 m be the wall of a building. A is the window and AB = 25 m be the ladder.

In right \(\triangle\)ABC

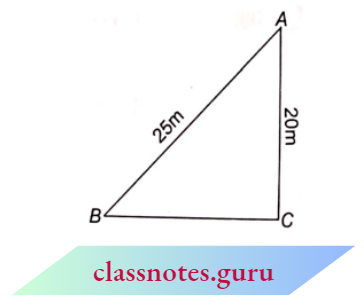
⇒ \(A B^2=B C^2+A C^2\) (by Pythagoras theorem)

⇒ \(25^2=B C^2+20^2\)

625 = \(B C^2+400\)

⇒ \(B C^2\) = 225

BC = 15

The distance of the foot of the ladder from the building = 15 cm

Example 3. P and Q are the mid-points of the sides C A and CB respectively of a \(\triangle\)ABC, oght anglers at C, prove that:

1. \(4 A Q^2=4 A C^2+B C^2\)
2. \(4 B P^2=4 B C^2+A C^2\)
3. \(4\left(A Q^2+B P^2\right)=5 A B^2\)

Solution:

Given

P and Q are the mid-points of the sides C A and CB respectively of a \(\triangle\)ABC, oght anglers at C,

1. In \(\triangle A Q C, \angle C=90^{\circ}\)

Using Pythagoras theorem. \(A Q^2=A C^2+Q C^2\)

⇒ \(A Q^2=A C^2+\left(\frac{B C}{2}\right)^2\) (because Q is the mid-point of BC)

⇒ \(A Q^2=A C^2+\frac{B C^2}{4}\)

⇒ \(4 A Q^2=4 A C^2+B C^2\)……(1) Hence proved.

2. ln \(\triangle B P C, \angle C=90^{\circ}\)

Using Pythagoras theorem, we have \(B P^2=B C^2+P C^2\)

⇒ \(B P^2=B C^2+\left(\frac{A C}{2}\right)^2\) (since P is the mid-point of AC)

⇒ \(B P^2=B C^2+\frac{A C^2}{4}\)

∴ \(4 B P^2=4 B C^2+A C^2\)……(2) Hence Proved.

3. Adding (1) and (2), we get

⇒ \(4 A Q^2+4 B P^2=4 A C^2+B C^2+4 B C^2+A C^2\)

⇒ \(4\left(A Q^2+B P^2\right)=5 A C^2+5 B C^2\)

⇒ \(4\left(A Q^2+B P^2\right)=5\left(A C^2+B C^2\right)\)

⇒ \(4\left(A Q^2+B P^2\right)=5 A B^2\) (because \(A C^2+B C^2=A B^2\))

Hence Proved.

Example 4. ABC is a right triangle, right angled at C. If p is the length of the perpendicular from C to AB and AB = c, BC = a and CA = b, then prove that

1. pc = ab
2. \(\frac{1}{p^2}=\frac{1}{a^2}+\frac{1}{b^2}\)

Solution:

Given: ABC is a right triangle, right angled at C, and p is the length of the perpendicular from C to AB.

Proof:

1. \(\text{ar}(\triangle A B C)=\frac{1}{2} \times A B \times C D\)

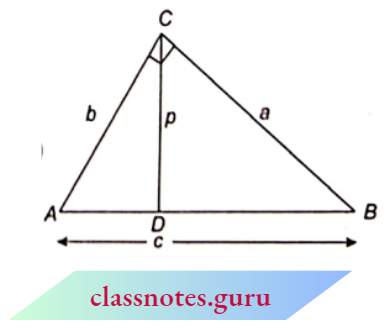
= \(\frac{1}{2} c p\)

Also, \(\text{ar}(\triangle M B C)=\frac{1}{2} \times \Lambda C \times B C\)

= \(\frac{1}{2} b a\)……(2)

From (1) and (2), we get, \(\frac{1}{2} p c=\frac{1}{2} a b\)

pc = ab……(3)

Hence Proved.

2. From (3) we get c = \(\frac{a b}{p}\)

In \(\triangle A B C\) \(c^2=a^2+b^2\) (by Pythagoras theorem)

⇒ \(\left(\frac{a b}{p}\right)^2=a^2+b^2 \quad \Rightarrow \quad \frac{a^2 b^2}{p^2}=a^2+b^2 \)

⇒ \(\frac{1}{p^2}=\frac{a^2+b^2}{a^2 b^2} \quad \Rightarrow \quad \frac{1}{p^2}=\frac{a^2}{a^2 b^2}+\frac{b^2}{a^2 b^2}\)

⇒ \(\frac{1}{p^2}=\frac{1}{b^2}+\frac{1}{a^2}\)

Hence Proved.

Alternative proof (Using trigonometry)

Let \(\angle C A B=\theta\)

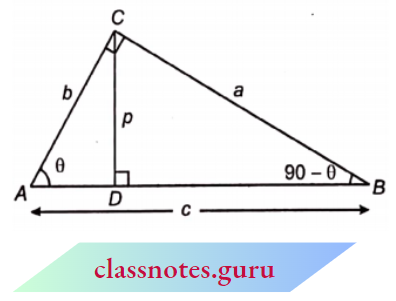
⇒ \(\angle C B A=90^{\circ}-\theta\) (\(\angle ACB\) = 90°)

In right \(\triangle C D A\), \(\sin \theta=\frac{p}{b}\)…….(1)

In right \(\triangle C D B\), \(\sin (90-\theta)=\frac{p}{a}\)

⇒ \(\sin (90-\theta)=\frac{p}{a}\) (because \(\sin (90-\theta)=\cos \theta\))……(2)

Squaring and adding equations (1) and (2), we get \(\sin ^2 \theta+\cos ^2 \theta=\frac{p^2}{b^2}+\frac{p^2}{a^2}\)

1 = \(p^2\left(\frac{1}{a^2}+\frac{1}{b^2}\right)\)

∴ \(\frac{1}{p^2}=\frac{1}{a^2}+\frac{1}{b^2}\) (using identity \(\sin ^2 \theta+\cos ^2 \theta=1\))

Hence Proved.

Example 5. O is any point in the Interior of rectangle ABCD. Prove that \(O B^2+O D^2=O C^2+O A^2\).
Solution:

ABCD is a rectangle and O is a point interior to the rectangle. Through O, draw a line parallel to BC meeting AB and DC at F and E respectively.

Then EC = PB and DE = AF

L.H.S. = \(O B^2+O I^2\)

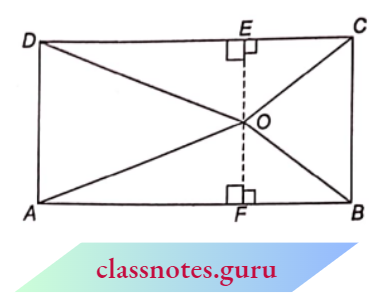
= \(\left(O F^2+F B^2\right)+\left(O E^2+D E^2\right)\)

= \(O F^2+E C^2+O E^2+A F^2\) (by Pythagoras theorem)

= \(\left(O R^2+E C^2\right)+\left(O F^2+A F^2\right)\)

= \(O C^2+O A^2\) FB and \(D E=A F\) (by Pythagoras theorem)

= R.H.S.

Hence, \(O B^2+O D^2=O C^2+O \Lambda^2\)

Hence Proved.

Example 6. In the given figure, S and T trisect QR of a right-angled triangle PQR, right-angled at Q. Prove that 8 PT2 = 3PR² + 5PS².
Solution:

Given

In the given figure, S and T trisect QR of a right-angled triangle PQR, right-angled at Q.

Let QS = ST=TR =x

Now by Pythagoras theorem Prove that \(8 P T^2=3 P R^2+5 P S^2\).

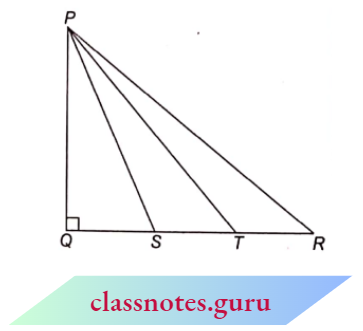
Let QS = ST = TR = x

Now by Pythagoras theorem \(P R^2=P Q^2+Q R^2=P Q^2+(3 x)^2\)

= \(P Q^2+9 x^2\)

⇒ \(3 P R^2=3 P Q^2+27 x^2\) ……(1)

Also, \(P S^2=P Q^2+Q S^2\) (by Pythagoras theorem)

= \(P Q^2+x^2\)

⇒ \(5 P S^2=5 P Q^2+5 x^2\)…..(2)

Adding (1) and (2), we get

R.H.S. = \(3 P R^2+5 P S^2=8 P Q^2+32 x^2=8(P Q^2+ Q T^2)\)

= \(8\left(P Q^2+Q T^2\right)=8 P T^2\)

= L.H.S (by Pythagoras theorem)

Hence Proved.

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle Exercise 6.1

Question 1. Fill in the blanks using the correct word given in brackets:

1. All circles are……… (congruent, similar)
Solution: Similar

2. All squares are……… (similar, congruent)
Solution: Similar

3. All……..triangles are similar. (isosceles, equilateral)
Solution:  equilateral

4. Two polygons of the same number of sides are similar, if (1) their corresponding angles are … (2) their corresponding sides are
Solution: equal, proportional

Question 2. Give two different examples of

1. Similar
2. Non-similar

Solution:

1. The two circles are similar to each other.

Two squares are similar to each other.

2. A circle and a square are not similar.

A parallelogram and a rhombus are not similar.

Question 3. State whether the following quadrilaterals are similar or not
Answer:

Here the sides are proportional but the corresponding angles are not equal.

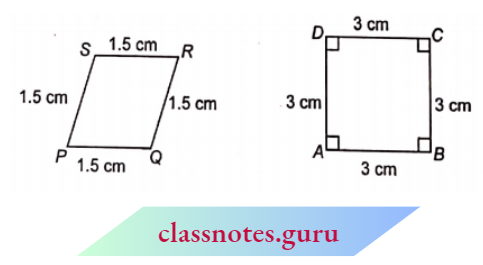
Figures are not similar

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle Exercise 6.2

Question 1. In figures, (1) and (2), DE || BC. Find EC in (1) and AD in (2).
Solution:

(1) In \(\triangle A B C: D E || B C\)

⇒ \(\frac{A D}{B D}=\frac{A E}{E C}\)

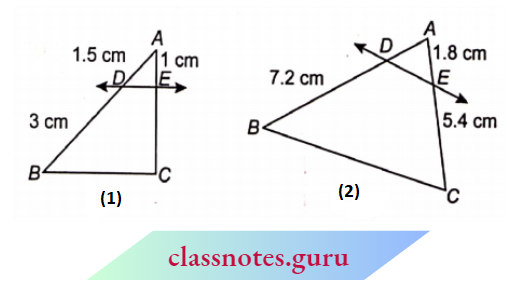
⇒ \(\frac{1.5}{3}=\frac{1}{E C}\)

EC = \(\frac{1 \times 3}{1.5}=2 \mathrm{~cm}\)

(2) ln MBC: DE || BC

⇒ \(\frac{A D}{B D}=\frac{A E}{E C} \Rightarrow \frac{A D}{7.2}=\frac{1.8}{5.4}\)

⇒ \(\frac{A D}{7.2}=\frac{1}{3} \Rightarrow A D=\frac{7.2}{3}=2.4 \mathrm{~cm}\)

Question 2. E and F are points on the sides of PQ and PR respectively of an \(\triangle\)PQR. For each of the following cases, state whether EF || QR:

1. PE = 3.9 cm. EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm
2. PE = 4 cm, QE = 4.5 cm, PF = 8 cm and RF = 9 cm
3. PQ = 1 .28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm

Solution:

Given

E and F are points on the sides of PQ and PR respectively of an \(\triangle\)PQR.

1. Here PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm, FR = 2.4 cm

⇒ \(\frac{P E}{E Q}=\frac{3.9}{3}=\frac{1.3}{1}\)

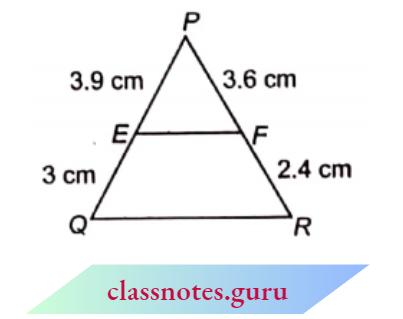
and \(\frac{P F}{F R}=\frac{3.6}{2.4}=\frac{1.5}{1}\)

because \(\frac{P E}{E Q} \neq \frac{P F}{F R}\)

EF is not parallel to QR.

2. Here PE = 4 cm, QE = 4.5 cm, PF = 8 cm, FR = 9 cm

\(\frac{P F}{E Q}=\frac{4}{4.5}=\frac{40}{45}=\frac{8}{9}=\frac{P F}{F R}\)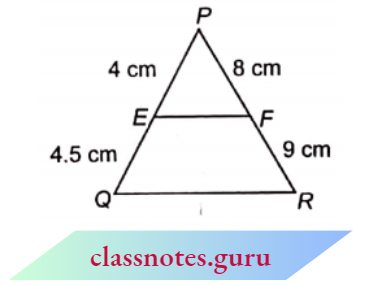

EF || QR

3. Here, PE = 0.18 cm, PQ – 1.28 cm, PF = 0.36 cm, PR = 2.56 cm

⇒ \(\frac{P E}{P Q}=\frac{0.18}{1.28}=\frac{18}{128}=\frac{9}{64}\)

and \(\frac{P F}{P R}=\frac{0.36}{2.56}=\frac{36}{256}=\frac{9}{64}\)

⇒ \(\frac{P E}{P Q}=\frac{P F}{P R}\)

EF || Q R

Question 3. in Figure, if LM || CB and LN || CD, prove that = \(\frac{A M}{A B}=\frac{A N}{A D}\)

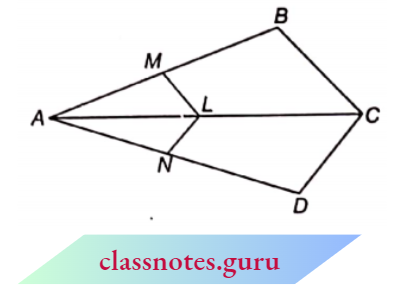
Solution:

In \(\triangle A B C, L M \| C B\)

⇒ \(\frac{A M}{A B}=\frac{A L}{A C}\)……..(1)

In \(\triangle A C D, L N \| C D\)

⇒ \(\frac{A L}{A C}=\frac{A N}{A D}\)….(2)

From equations (1) and (2), \(\frac{A M}{A B}=\frac{A N}{A D}\)

Hence Proved.

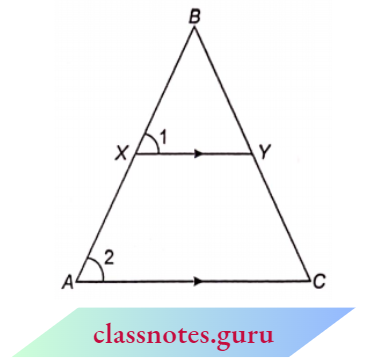
Question 4. In the figure, DE || AC and DF || AE. Prove that \(\frac{B F}{F E}=\frac{B E}{E C}\).

Solution:

Here D E || A C

In \(\triangle A B C, \quad \frac{B E}{E C}=\frac{B D}{D A}\)……(1)

Again D F }| AE,

In \(\triangle A B E, \quad \frac{B D}{D A}=\frac{B F}{F E}\)……(2)

From equations (1) and (2), \(\frac{3 F}{E E}=\frac{B E}{E C}\)

Hence proved.

Question 5. In the figure, DE || OQ and DF || OR. Show that EF || QR.

Solution:

In the figure, DE || OQ and DF || OR.

Now, from B.P.T.,

In \(\triangle P Q O\), \(\frac{P E}{E Q}=\frac{P D}{D O}\)….(1)

and, in \(\triangle P O R\), \(\frac{P F}{F R}=\frac{P D}{D O}\)…..(2)

From equations (1) and (2), \(\frac{P E}{E Q}=\frac{P F}{F R}\)

Now, in \(\triangle P Q R, \quad \frac{P E}{E Q}=\frac{P F}{F R}\)

E F || QR (from the converse of B.P.T)

Hence Proved.

Question 6. In the figure, A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.

Solution:

In the figure, AB || PQ (given)

⇒ \(\frac{O A}{A P}=\frac{O B}{B Q}\) (from B.PT.)…..(1)

In the figure, \(A C \| P R\)(given)

⇒ \(\frac{O A}{A P}=\frac{O C}{C R}\) from B.P.T. …(2)

From equations (1) and (2), \(\frac{O B}{B Q}=\frac{O C}{C R} \Rightarrow B C \| Q R\) (from the converse of B.P.T.)

Hence Proved.

Question 7. Prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side.
Solution:

Given: \(\triangle\)ABC in which D is the mid-point of AB and DE is parallel to BC, meets AC at E

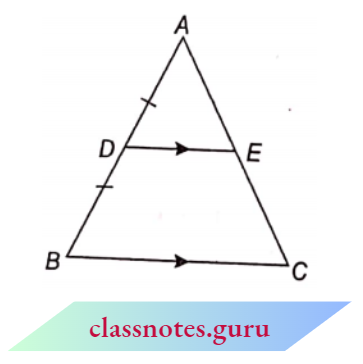
To prove: AE = EC

Proof: DE || BC

From B.P.T., \(\frac{A D}{D B}=\frac{A E}{E C}\)

but AD = D B (because D is midpoint)

⇒ \(\frac{A D}{D B}=\frac{A D}{A D}=1\)

From equations (1) and (2), \(\frac{A E}{E C}=1 \Rightarrow A E=E C\)

Hence Proved.

Question 8. Using Theorem 2, prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side.
Solution:

Given: In \(\triangle\)ABC, D is the midpoint ofAB and E is the mid-point of AC.

To prove: DE || BC

Proof: D and E are the mid-points of AB and AC respectively.

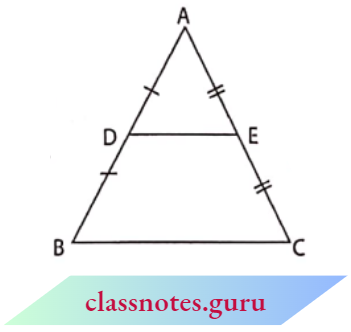
AD = DB

and AE = EC

⇒ \(\frac{A D}{D B}=1\) and \(\frac{A E}{E C}=1\)

⇒ \(\frac{A D}{D B}=\frac{A E}{E C}\)

From the converse of B.P.T., DE || BC.

Hence Proved

Question 9. ABCD is a trapezium in which AB || DC and its diagonals intersect each other at the point O. Show that \(\frac{A O}{B O}=\frac{C O}{D O}\)

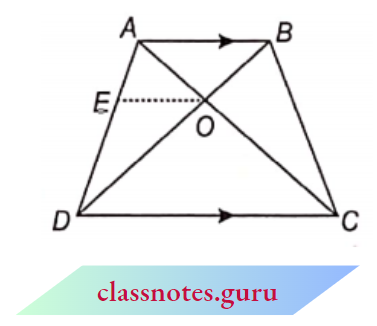
Solution:

ABCD is a trapezium whose diagonals intersect each other at point O.

Draw EO || AB || DC.

In \(\triangle\) ADC, EO || DC

⇒ \(\frac{A E}{E D}=\frac{A O}{O C}\)…..(1)

In \(\triangle D A B, E O \| A B\)

⇒ \(\frac{D E}{E A}=\frac{D O}{O B} \Rightarrow \frac{A E}{E D}=\frac{O B}{O D}\)……(2)

From equations (1) and (2), \(\frac{A O}{O C}=\frac{O B}{O D}\)

Hence Proved.

Question10. The diagonals of a quadrilateral ABCD intersect each other at point O such that \(\frac{A O}{B O}=\frac{C O}{D O}\). Show that ABCD is a trapezium.
Solution:

Given

The diagonals of a quadrilateral ABCD intersect each other at point O such that \(\frac{A O}{B O}=\frac{C O}{D O}\).

Draw EO || DC which meets AD at E

Now, in \(\triangle A D C, E O \| D C\)

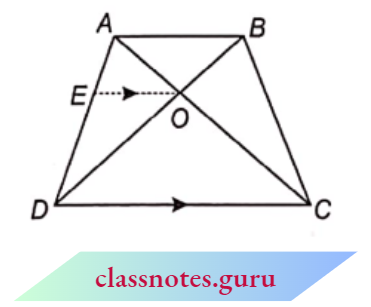
⇒ \(\frac{A E}{E D}=\frac{A O}{O C}\)…..(1)

Given, \(\frac{A O}{O C}=\frac{B O}{O D}\)…..(2)

From equations (1) and (2) \(\frac{A E}{E D}=\frac{B O}{O D} \Rightarrow E O \| A B(\ln \triangle D A B)\)

but E O || DC

AB || DC

⇒ square A B C Dis a trapezium.

Hence Proved.

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle Exercise 6.3

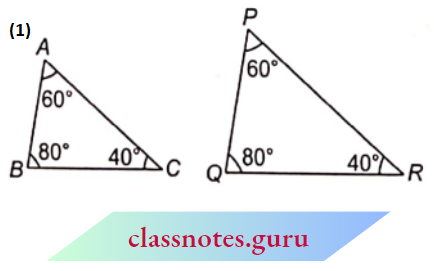
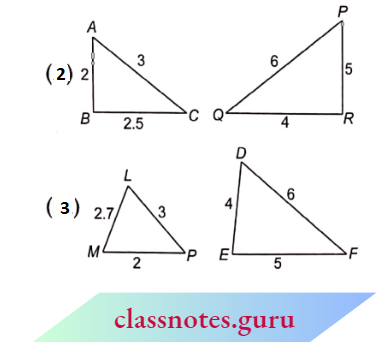
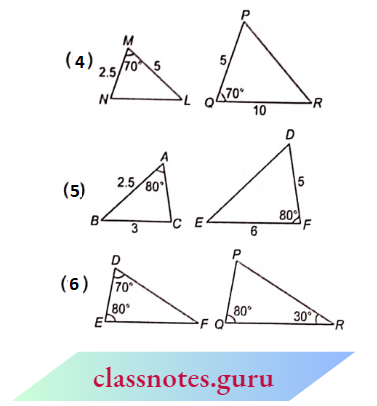
Question 1. State which pairs of triangles in figures are similar. Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form:

Solution:

1. In \(\triangle M B C\) and \(\triangle P Q R\),

⇒ \(\angle A=\angle P=60^{\circ}, \angle B=\angle Q=80^{\circ},\), \(\angle C=\angle R=40^{\circ}\)

⇒ \(\triangle A B C-\triangle P Q R\)

⇒ \(\triangle A B C-\triangle P Q R\) (from A.A.A. similarity)

2. Here \(\frac{A B}{Q R}=\frac{2}{4}=\frac{1}{2}, \frac{B C}{R P}=\frac{2.5}{5}=\frac{1}{2}\)

and \(\frac{A C}{Q P}=\frac{3}{6}=\frac{1}{2}\)

⇒ \(\frac{A B}{Q R}=\frac{B C}{R P}=\frac{A C}{Q P}\)

⇒ \(\triangle M B C-\triangle Q R P\) (from S.S.S. similarity)

3. Here \(\frac{M P}{E D}=\frac{2}{4}=\frac{1}{2}, \frac{P L}{D F}=\frac{3}{6}=\frac{1}{2}\),

⇒ \(\frac{L M}{F E}=\frac{2.7}{5}=\frac{27}{50}\)

⇒ \(\frac{M P}{E D}=\frac{P L}{D F} \neq \frac{L M}{F E}\)

∴ \(\triangle L M P\) and \(\triangle D E F\) are not similar.

4. Here \(\frac{M P}{Q P}=\frac{2.5}{5}=\frac{1}{2}, \frac{M L}{Q R}=\frac{5}{10}=\frac{1}{2}\)

⇒ \(\frac{M P}{Q P}=\frac{M L}{Q R}\) and \(\angle M=\angle Q\)

⇒ \(\triangle M N L-\triangle Q P R\) (from S.S.A. similarity)

5.Here \(\frac{A B}{F D}=\frac{2.5}{5}=\frac{1}{2}, \angle A=\angle F=80^{\circ}\)

but AC and DE are unknown.

∴ \(\triangle A B C\) and \(\triangle D E F\) are not similar.

6. ln \(\triangle D E F\), \(\angle F=180^{\circ}-\angle D-\angle E\)

= \(180^{\circ}-70^{\circ}-80^{\circ}=30^{\circ}\)

In \(\triangle P Q R\),

⇒ \(\angle P =180^{\circ}-\angle Q-\angle R\)

= \(180^{\circ}-80^{\circ}-30^{\circ}=70^{\circ}\)

Now, \(\angle D=\angle P, \angle E=\angle Q, \angle F=\angle R\)

⇒ \(\triangle D E F \sim \triangle P Q R\) (from AAA similarity)

Question 2. In figure, \(\triangle O D C \sim \triangle O B A\), \(\angle B O C=125^{\circ}\) and \(\angle C D O=70^{\circ}\). Find \(\angle D O C\), \(\angle D C O\) and \(\angle O A B\).

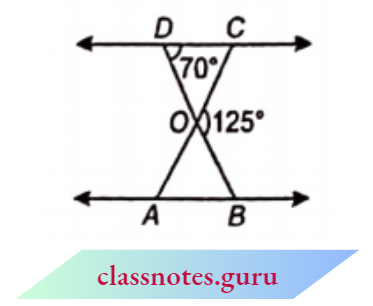
Solution:

Here \(\angle B O C=125^{\circ}\)

and \(\angle C D O=70^{\circ}\)

⇒ \(\angle D O C+\angle B O C=180^{\circ}\) (linear pair)

⇒ \(\angle D O C=180^{\circ}-\angle B O C\)

= \(180^{\circ}-125^{\circ}=55^{\circ}\)

In \(\triangle D O C\),

⇒ \(\angle D C O=180^{\circ}-\angle C O D-\angle O D C\)

= \(180^{\circ}-55^{\circ}-70^{\circ}=55^{\circ}\)

⇒ \(\triangle O D C-\triangle O B A\)

⇒ \(\angle O C D=\angle O A B\)

∴ \(\angle O A B=55^{\circ}\)

Question 3. Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O. Using a similarity criterion for two triangles, show that \(\frac{O A}{O C}=\frac{O B}{O D}\)
Solution:

Given

Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O.

Draw EO || AB || CD

Now, in \(\triangle\)ADC,

EO || DC

⇒ \(\frac{A E}{E D}=\frac{A O}{O C}\) (from B.P.T.)…(1)

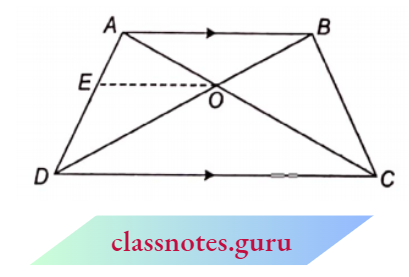
ln \(\triangle A B D\)

EO || AB

⇒ \(\frac{A E}{E D}=\frac{B O}{O D}\) (from B.P.T.)…(2)

From equations (1) and (2) \(\frac{A O}{O C}=\frac{B O}{O D}\)

Hence proved.

Question 4. In figure, \(\frac{Q R}{Q S}=\frac{Q T}{P R}\) and \(\angle 1=\angle 2\). Show that \(\triangle P Q S \sim \triangle T Q R\).

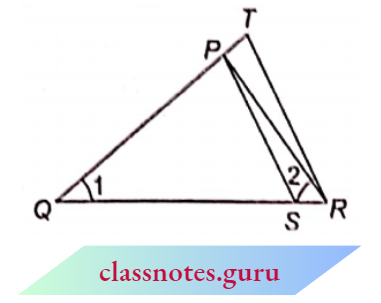
Solution:

Given

In figure, \(\frac{Q R}{Q S}=\frac{Q T}{P R}\) and \(\angle 1=\angle 2\).

In \(\triangle P Q R, \angle 1=\angle 2\) (given)

PR = PQ….(1) (sides opposite to equal angles in \(\Delta\) are equal)

Given, \(\frac{Q R}{Q S}=\frac{Q T}{P R} \Rightarrow \frac{Q R}{Q S}=\frac{Q T}{Q P}\) [from equation (1)]

⇒ \(\frac{Q S}{Q R}=\frac{Q P}{Q T}\)

In \(\triangle P Q S\) and \(\triangle T Q R\),

⇒ \(\frac{Q S}{Q R}=\frac{Q P}{Q T}\) and \(\angle S Q P=\angle R Q T\) (each \(\angle 1\))

⇒ \(\triangle P Q S \sim \triangle T Q R\) (from S.A.S. similarity)

Hence Proved.

Question 5. S and T are points on sides PR and QR of \(\triangle P Q R\) such that \(\angle P=\angle R T S\). Show that \(\triangle R P Q \sim \triangle R T S\).
Solution:

Given: \(\triangle R P Q\) and \(\triangle R T S\) in which \(\angle P=\angle R T S\)

To show: \(\triangle R P Q \sim \triangle R T S\)

Proof: \(\ln \triangle R P Q\) and \(\triangle R T S\), \(\angle 1=\angle 2\) (given)

⇒ \(\angle 3=\angle 3\) (common)

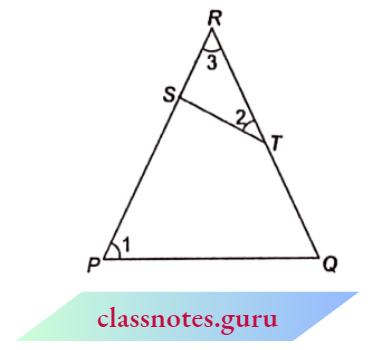
⇒ \(\triangle R P Q \sim \Delta R T S\) (from A.A. corollary)

Hence Proved.

Question 6. In the figure, if \(\triangle A B E \cong \triangle A C D\) shows that \(\triangle A D E \sim \triangle A B C\).
Solution:

Given, \(\triangle A B E \cong \triangle A C D\)

AE=AD….(1)

and AB=AC….(2)

⇒ \(\frac{A D}{A B}=\frac{A I}{A C}\) and \(\angle A=\angle A\) (common)

⇒ \(\triangle\) ADE \(\triangle\) ABC (from S.A.S. similarity)

Hence Proved.

Question 7. In the figure, altitudes AD and CE of \(\triangle\) ABC Intersect each other at the point Show that:

1. \(\triangle A E P-\triangle C D P\)
2. \(\triangle A B D-\triangle C B E\)
3. \(\triangle A E P \sim \triangle A D B\)
4. \(\triangle P D C-D B E C\)

Solution:

1. In the figure, \(\angle A E P=\angle C D P\) (each \(90^{\circ}\))

and \(\angle A P E=\angle C P D\) (vertically opposite angles)

⇒ \(\triangle A E P \sim \triangle C D P\) (from A.A.A. similarity)

Hence Proved.

2. In figure, \(\angle A D B=\angle C E B\) (each \(90^{\circ}\))

and \(\angle A B D=\angle C B E\) (each = \(\angle B\))

⇒ \(\triangle A B D \sim \triangle C B E\) (from A.A.A. similarity)

Hence Proved.

3.  In figure, \(\angle A E P=\angle A D B\) (each \(90^{\circ}\))

and \(\angle P A E=\angle B A D\) (common angle)

\(\triangle A E P \sim \triangle A D B\) (from A.A.A. similarity)

Hence Proved.

4. In figure, \(\angle P D C=\angle B E C\) (each \(90^{\circ}\))

and \(\angle P C D=\angle B C E\) (common angle)

⇒ \(\triangle P D C \sim \triangle B E C\) (from A.A.A. similarity)

Hence Proved.

Question 8. E is a point on the side AD produced of parallelogramABCD and BE intersects CD at F. Show that \(\triangle A B E \sim \triangle C F B\)
Solution:

Given

E is a point on the side AD produced of parallelogramABCD and BE intersects CD at F.

AE || BC and BE is a transversal.

⇒ \(\angle\) 1 = \(\angle\) 2(alternate angles)

⇒ \(\angle\) 3 = \(\angle\) 4(opposite angles of parallelogram)

⇒ \(\triangle A B E \sim \triangle C F B\) (A. A similarly)

Question 9. In the figure, ABC and AMP are two right. triangles, right-angled at B and M respectively. Prove that

1. \(\triangle A B C \sim \triangle A M P\)
2. \(\frac{C A}{P A}=\frac{B C}{M P}\)

Solution:

Given

In the figure, ABC and AMP are two right. triangles, right-angled at B and M respectively.

1. In \(\triangle A B C\) and \(\triangle A M P\)

⇒ \(\angle A B C=\angle A M P\) (each \(90^{\circ}\))

⇒ \(\angle B A C=\angle M A P\) (common)

⇒ \(\triangle A B C \sim \triangle A M P\) (from A.A. similarity)

2. Divide (2) by (1)

⇒ \(\triangle A B C \sim \triangle A M P\)

⇒ \(\frac{C A}{P A}=\frac{B C}{M P}\) (ratio of corresponding sides)

Hence Proved.

Question 10. CD and GH are respectively the bisectors of \(\angle\) ACB and \(\angle\) EGF such that D and H lie on sides AB and FE of \(\triangle\) ABC and \(\triangle\) EFG respectively. If latex]\triangle[/latex] ABC ~ \(\triangle\) FEG.

1. \(\frac{C D}{G H}=\frac{A C}{F G}\)
2. \(\triangle D C B \sim \triangle H G E\)
3. \(\triangle D C A \sim \triangle H G F\)

Solution:

1. In \(\triangle A C D\) and \(\triangle F G H\)

⇒ \(\angle C A D=\angle G F H\)

(because \(\triangle A B C \sim \triangle F E G\))

but \(\triangle A B C \sim \triangle F E G\) (given)

⇒ \(\angle C=\angle G\)

⇒ \(\angle A C D=\angle F G H\)

From equations (1) and (2)

⇒ \(\triangle A C D \sim \triangle F G H\) (from A.A. similarity)

⇒ \(\frac{C D}{G H}=\frac{A C}{F G}\)

2. \(\triangle A B C \sim \triangle F E G\)

⇒ \(\angle A C B=\angle F G E\)

⇒ \(\frac{1}{2} \angle A C B=\frac{1}{2} \angle F G E\)

⇒ \(\angle D C B=\angle H G E\) and \(\angle D B C=\angle H E G\)

⇒ (because \(\triangle A B C \sim \triangle F E G \Rightarrow \angle B=\angle E\))

⇒ \(\triangle D C B \sim \triangle H G E\) (A.A. similarity)

3. \(\triangle A B C \sim \triangle F E G\)

⇒ \(\angle C A B=\angle G F E\)

⇒ \(\angle C A D=\angle G F H\)

⇒ \(\angle D A C=\angle H F G\)

and \(\triangle A B C \sim \triangle F E G\)

⇒ \(\angle A C B=\angle F G E\)

⇒ \(\frac{1}{2} \angle A C B=\frac{1}{2} \angle F G E\)

⇒ \(\angle D C A=\angle H G F\)

Now \(\angle D A C=\angle H F G\)

and \(\angle D C A=\angle H G F\)

⇒ \(\triangle D C A \sim \triangle H G F\) (A.A. similarity)

Hence Proved.

Question 11. In figure, E is a point on side CB produced of an isosceles triangle ABC with AB =AC. If \(A D \perp B C\) and \(E F \perp A C\), prove that \(\triangle A B D \sim \triangle E C F\).

Solution:

Given

In figure, E is a point on side CB produced of an isosceles triangle ABC with AB =AC. If \(A D \perp B C\) and \(E F \perp A C\)

⇒ \(\triangle A B C\) is isosceles in which,

AB = AC

⇒ \(\angle A C B=\angle A B C\) (angles opposite to equal sides)

⇒ \(\angle E C F=\angle A B D\)

and \(\angle E F C=\angle A D B\) (each \(90^{\circ}\))

⇒ \(\triangle E C F \sim \triangle A B D\) (from A.A. similarity)

Hence Proved.

Question 12. Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of \(\triangle\)PQR. Show that \(\triangle A B C \sim \triangle P Q R \text {. }\).

Solution:

Given: \(\triangle A B C\) and \(\triangle P Q R\), in AD \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A D}{P M}\)

To show: \(\triangle A B C \sim \triangle P Q R\)

Proof: \(\frac{A B}{P Q}=\frac{B C}{Q R}=\frac{A D}{P M}\) (given)

⇒ \(\frac{A B}{P Q}=\frac{2 B D}{2 Q M}=\frac{A D}{P M}\) (AD and PM are medians)

⇒ \(\frac{A B}{P Q}=\frac{B D}{Q M}=\frac{A D}{P M}\)

⇒ \(\triangle A B D \sim \triangle P Q M\) (from S.S.S. similarity)

⇒ \(\angle B=\angle Q\) (corresponding angles are equal of similar triangles)

Now, in \(\triangle A B C\) and \(\triangle P Q R\), \(\frac{A B}{P Q}=\frac{B C}{Q R}\) (given)

and \(\angle B=\angle Q\) (proved above)

⇒ \(\triangle A B C \sim \triangle P Q R\) (from S.A.S. similarity)

Hence Proved.

Question 13. D is a point on the side BC of a triangle ABC such that triangle ABC such that \(\angle A D C=\angle B A C\). Show that \(C A^2=C B \cdot C D\).
Solution:

Given

D is a point on the side BC of a triangle ABC such that triangle ABC such that \(\angle A D C=\angle B A C\).

In \(\triangle A B C\) and \(\triangle D A C\),

⇒ \(\angle B A C=\angle A D C\) (given)

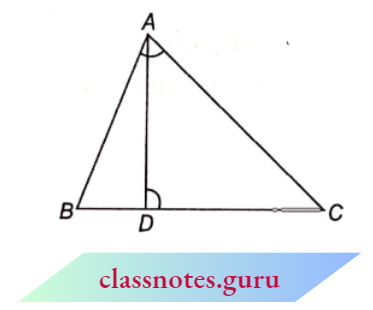
⇒ \(\angle A C B=\angle D C A\) (common)

⇒ \(\triangle A B C \sim \triangle D A C\)

⇒ \(\frac{C D}{C A}=\frac{C A}{C B}\) (A.A. similarity)

⇒ \(C A^2=C B \times C D\)

Hence Proved.

Question 14. Sides AB and AC and median AD of a triangle ABC are respectively proportional
to sides PQ and PR and median PM of another triangle PQR. Show that triangle PQR. Show that \(\triangle A B C \sim \triangle P Q R\).
Solution:

Given: \(\triangle A B C\) and \(\triangle P Q R\) in which AD and PS are the median such that

⇒ \(\frac{A B}{P Q}=\frac{A C}{P R}=\frac{A D}{P S}\)

To show: \(\triangle A B C \sim \triangle P Q R\)

Construction: Produce AD to E such that AD=DE. Join EC, produce PS to T such that PS=ST.

Join TR.

Proof: In \(\triangle A B D\) and \(\triangle E C D\),

BD = DC (Dis mid-point of BC)

⇒ \(\angle\)5 = \(\angle\)6 (vertically opposite angle)

AD = DE (from construction)

⇒ \(\triangle A B D \cong \triangle E C D\) (S.A.S. congruency)

AB=EC (congruent parts of congruent triangles are equal)…(1)

Similarly, we can prove that \(\triangle P Q S \cong \triangle T R S\)

PQ=TR…..(2)

Now, \(\frac{A B}{P Q}=\frac{A C}{P R}=\frac{A D}{P S}\)

⇒ \(\frac{E C}{T R}=\frac{A C}{P R}=\frac{2 A D}{2 P S}\) (from equations (1) and (2))

⇒ \(\frac{E C}{T R}=\frac{A C}{P R}=\frac{A E}{P T}\)

⇒ \(\triangle A E C \sim \triangle P T R\) (S.S.S. similarity)

⇒ \(\angle 1=\angle 2\) (corresponding angles of similar triangles are equal)…..(3)

Similarly, we can prove that \(\angle 3=\angle 4\)…..(4)

Adding equations (3) and (4).

⇒ \(\angle 1+\angle 3=\angle 2+\angle 4 \Rightarrow \angle A=\angle P\)

Now, in \(\triangle A B C\) and \(\triangle P Q R\).

⇒ \(\frac{A B}{P Q}=\frac{A C}{P R}\) (given)

and \(\angle A=\angle P\)(proved above)

∴ \(\Delta B C-\triangle P Q R\) (S.A.S. similarity)

Question 15. A vertical pole of length 6 m casts a shadow 4 m long on the ground and at the same time, a tower casts a shadow 28 m long. Find the height of the tower.
Solution:

Given

A vertical pole of length 6 m casts a shadow 4 m long on the ground and at the same time, a tower casts a shadow 28 m long.

Let MC be a pole of 6m in height and its shadow is DC = 4m. At the same time, tower AB, whose height is h metre, has its shadow BD = 28 m.

Now, \(\triangle D C M \sim \triangle D B A\)

⇒ \(\frac{D C}{D B}=\frac{C M}{B A}\)

⇒ \(\frac{4}{28}=\frac{6}{h} \quad \Rightarrow \quad h=\frac{28 \times 6}{4}=42\)

Height of tower = 42 ~m

Question 16. If AD and PM are medians of triangles ABC and PQR respectively where \(\triangle A B C – [latex]\triangle P Q R\) prove that \(\frac{A B}{P Q}=\frac{A D}{P M} \text {. }\)
Solution:

Given: AD and PM are the medians of \(\triangle A B C\) and \(\triangle P Q R\) respectively.

and \(\triangle A B C \sim \triangle P Q R\)

To prove: \(\frac{A B}{P Q}=\frac{A D}{P M}\)

Proof: In \(\triangle A B D\) and \(\triangle P Q M\),

⇒ \(\angle B=\angle Q\) (because \(\triangle A B C \sim \triangle P Q R\))

⇒ \(\frac{A B}{P Q}=\frac{\frac{1}{2} B C}{\frac{1}{2} Q R}\) (because \(\frac{A B}{P Q}=\frac{B C}{Q R}\))

⇒ \(\frac{A B}{P Q}=\frac{B D}{Q M}\)

⇒ \(\triangle A B D \sim \triangle P Q M\) (from S.A.S. similarity)

∴ \(\frac{A B}{P Q}=\frac{A D}{P M}\) Hence Proved.

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle Exercise 6.4

Question 1. Let \(\triangle\)ABC ~ \(\triangle\)DEF areas be, respectively, 64 cm EF = 15.4 cm, find BC.
Solution:

Given

Let \(\triangle\)ABC ~ \(\triangle\)DEF areas be, respectively, 64 cm EF = 15.4 cm

⇒ \(\triangle A B C \sim \triangle D E F\)

⇒ \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle D E F)}=\frac{B C^2}{E F^2} \Rightarrow \frac{64}{121}=\frac{B C^2}{E F^2}\)

⇒ \(\frac{8}{11}=\frac{B C}{E F}\)

∴ \(BC=\frac{8}{11} \times E F=\frac{8}{11} \times 15.4=11.2 \mathrm{~cm}\)

Question 2. Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2CD, find the ratio of the areas of triangles AOB and COD.
Solution:

Given

Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2CD

In \(\triangle\)AOB and \(\triangle\)COD, \(\angle\)1 = \(\angle\)2 (vertically opposite angles)

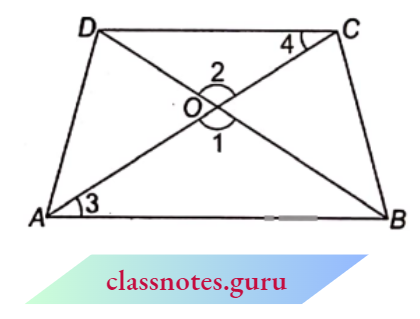
⇒  \(\angle\)3 = \(\angle\)4 (alternate angles, AB || DC)

⇒ \(\triangle A O B \sim \triangle C O D\) (A.A. corollary)

⇒ \(\frac{\text{ar}(\triangle A O B)}{\text{ar}(\triangle C O D)}=\frac{A B^2}{C D^2}\)

(because the ratio of the areas of two similar triangles is equal to the ratio of the square of their corresponding sides)

= \(\frac{(2 C D)^2}{C D^2}\) (because AB=2CD, given)

= \(\frac{4 C D^2}{C D^2}=\frac{4}{1}\)

∴ \(\text{ar}(\triangle A O B):\text{ar}(\triangle C O D)=4: 1\)

Question 3. In the figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that \(\frac{\text{ar}(A B C)}{\text{ar}(D B C)}=\frac{A O}{D O}\).

Solution:

Given

In the figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O

Draw \(A L \perp B C\) and \(D M \perp B C\)

In \(\triangle O L A\) and \(\triangle O M D\),

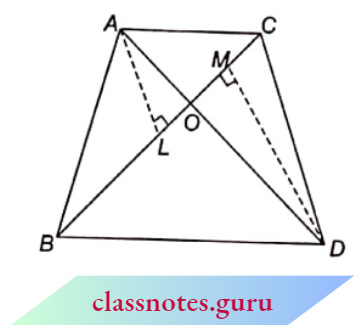
⇒ \(\angle A L O=\angle D M O=90^{\circ}\)

and \(\angle A O L=\angle D O M\) (vertically opposite angles)

⇒ \(\triangle O L A \sim \triangle O M D\) (from A.A.A. similarity)

⇒ \(\frac{A L}{D M}=\frac{A O}{D O}\)

= \(\frac{A L}{D M}=\frac{A O}{D O}\)

Now \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle D B C)}=\frac{\frac{1}{2} \times(B C) \times(A L)}{\frac{1}{2} \times(B C) \times(D M)}\) [from equation (1)]

Therefore, \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle D B C)}=\frac{A O}{D O}\) Hence Proved.

Question 4. If the areas of two similar triangles are equal, prove that they are congruent.
Solution:

Given, \(\triangle A B C \sim \triangle P Q R\)

⇒ \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle P Q R)}=\frac{A B^2}{P Q^2}=\frac{B C^2}{Q R^2}=\frac{C A^2}{R P^2}\)

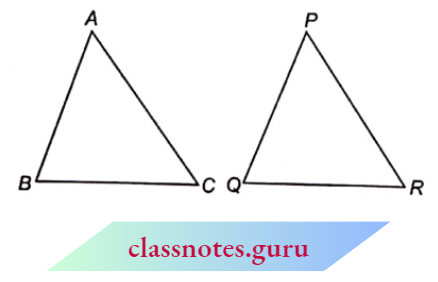
But \(\text{ar}(\triangle A B C)=\text{ar}(\triangle P Q R)\)

⇒ \(\frac{A B^2}{P Q^2}=\frac{B C^2}{Q R^2}=\frac{C A^2}{R P^2}=1\)

AB = PQ, BC = QR

CA = RP

⇒ \(\triangle A B C \cong \triangle P Q R\)(from S.S.S.)

Hence Proved.

Question 5. D, E and F are respectively the mid-points of sides AB, BC and CA of \(\triangle\) ABC. Find the ratio of the areas of \(\triangle\)DEF and \(\triangle\)ABC.
Solution:

Given: \(\triangle\)ABC in which D, E and F are the mid-points of sides BC, CA and AB respectively.

To find: The ratio of the areas of \(\triangle\)DEF and \(\triangle\)ABC.

Proof: D, E, and F are mid-points.

⇒  \(E F\| B C\) and \(E F=\frac{1}{2} B C\)

EF = BD

Similarly, \(D E=B F\) (because \(D E \| A B\) and \(\frac{1}{2} A B\))

So, \(E F B D\) is a parallelogram.

⇒ \(\angle B=\angle 1\)

Similarly, AFDE and EFDC are parallelograms.

⇒ \(\angle A=\angle 2\) and \(\angle C=\angle 3\)

i.e., \(\triangle A B C\) and \(\triangle D E F\) are equiangular.

⇒ \(\triangle D E F \sim \triangle A B C\)

⇒ \(\frac{\text{ar}(\triangle D E F)}{\text{ar}(\triangle A B C)}=\frac{D E^2}{A B^2}=\frac{F B^2}{A B^2}=\frac{(A B / 2)^2}{A B^2}=\frac{1}{4}\)

Therefore, \(\frac{\text{ar}(\triangle D E F)}{\text{ar}(\triangle A B C)}=\frac{1}{4}\)

Question 6. Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
Solution:

Given: \(\triangle\)ABC ~ \(\triangle\)DEF, AP and DQ are the medians of AABC and ADEF respectively.

To prove: \(\frac{\text{ar}(\triangle M B C)}{\text{ar}(\triangle D E F)}=\frac{A P^2}{D Q^2}\)

Proof: AP and DQ are medians.

So, BP=PC and EQ=QF

and given that \(\triangle A B C \sim \triangle D E F\)

So, \(\frac{A B}{D E}=\frac{A C}{D F}=\frac{B C}{E F}\) and \(\angle A=\angle D, \angle B=\angle E\) and \(\angle C=\angle F\)

Now, \(\frac{A B}{D E}=\frac{B C}{E F}\)

⇒ \(\frac{A B}{D E}=\frac{2 B P}{2 E Q} \Rightarrow \frac{A B}{D E}=\frac{B P}{E Q}\)

and \(\angle B=\angle E\)

⇒ \(\triangle A B P \sim \triangle D E Q\)(S.A.S. similarity)

Now, in \(\triangle A B C\) and \(\triangle D E F\), \(\frac{\text{ar}(\triangle \triangle B C)}{\text{ar}(\triangle D E F)}=\frac{A B^2}{D E^2}\)

(because the ratio of the areas of two similar triangles is equal to the ratio of the squares of its corresponding sides)

From equations (1) and (2), \(\frac{\text{ar}(\triangle A B C)}{text{ar}(\triangle D E F)}=\frac{A P^2}{D Q^2}\)

Hence Proved.

Question 7. Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the equilateral triangle described on one of its diagonals.
Solution:

Given: A square ABCD and an equilateral triangle BCE, formed on side BC and \(\triangle\) ACF formed on side AC.

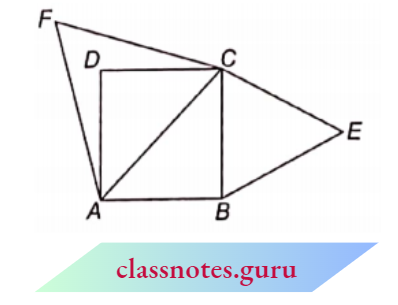
To prove: \(\text{ar}(\triangle B C E)=\frac{1}{2} \text{ar}(\triangle A C F)\)

Proof: \(\triangle B C E\) and \(\triangle A C F\) are equilateral triangles, so each angle of both is \(60^{\circ}\).

So, \(\triangle B C E \sim \triangle A C F\)

⇒ \(\frac{\text{ar}(\triangle B C E)}{\text{ar}(\triangle A C F)}=\frac{B C^2}{A C^2}=\frac{B C^2}{2(B C)^2}=\frac{1}{2}\)

Therefore, \(\text{ar}(\triangle B C E)=\frac{1}{2} \times \text{ar}(\triangle A C F)\)

Hence Proved.

Question 8. ABC and BDE are two equilateral triangles such that D is the mid-point of BC. The ratio of the areas of triangles ABC and BDE is:

1. 2: 1
2. 1: 2
3. 4: 1
4. 1: 4

Solution: 3. 4: 1

⇒ \(\triangle A B C\) and \(\triangle B D E\) are two equilateral triangles such that

BD = \(\frac{1}{2} B C\)

BD = \(\frac{1}{2} A B\) (because BC=A B)

⇒ \(\frac{A B}{B D}=\frac{2}{1}\)

⇒ \(\triangle A B C \sim \triangle B D E\)(triangles are equilateral)

⇒ \(\frac{\text{ar}(\triangle A B C)}{\text{ar}(\triangle B D E)}=\frac{A B^2}{B D^2}=\left(\frac{2}{1}\right)^2=4: 1\)

Question 9. The sides of two similar triangles are in

1. 2 : 3
2. 4: 9
3. 81: 16
4. 16: 81

Solution: 4. 16: 81

The ratio of the areas of two similar triangles = (ratio of the sides of triangles)2

= \(\left(\frac{4}{9}\right)^2=\frac{16}{81}=16: 81\)

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle Exercise 6.5

Question 1. The sides of the triangles are given below. Determine which of them are right triangles. In the case of a right triangle, write the length of its hypotenuse.

1. 7 cm, 24 cm, 25 cm
2. 3 cm, 8 cm, 6 cm
3. 50 cm, 80 cm, 100 cm
4. 13 cm, 12 cm, 5 cm

Solution:

Here

1. 7² + 24² = 49 + 576 = 625 = 252

The triangle is a right-angled hypotenuse = 25 cm

2. 3² + 6² = 9 + 36 = 45 82

The triangle is not right-angled.

3. 50² + 80² = 2500 + 6400 = 8900* 1002

The triangle is not right-angled.

4. 5²+ 12² = 25 + 144= 169 = 132

∴  Triangle is right-angled

hypotenuse = 13 cm

Question 2. PQR is a triangle right angled at P and M is a point on QR such that \(P M \perp Q R\). Show that \(P M^2=Q M \cdot M R\).
Solution:

Given

PQR is a triangle right angled at P and M is a point on QR such that \(P M \perp Q R\).

In \(\triangle P Q R, \angle P=90^{\circ}\)

and \(\angle 2+\angle 4=90^{\circ}\)

⇒ \(\angle 1+\angle 2=\angle 2+\angle 4 \Rightarrow \angle 1=\angle 4\)

Similarly, \(\angle 3=\angle 2\)

⇒ \(\triangle P Q M \sim \triangle R P M\) (from A.A. similarity)

⇒ \(\frac{Q M}{P M}=\frac{P M}{R M}\)

∴ \(P M^2=Q M \cdot M R\) Hence Proved

Question 3. In the figure, ABD is a triangle right-angled at A and AC¹ BD. Show that

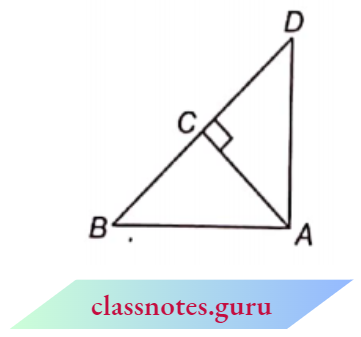
1. AB² = BC • BD
2. AC² =BC- DC
3. AD = BD CD

Solution:

1. In \(\triangle A B C\) and \(\triangle D B A\),

⇒ \(\angle A B C=\angle D B A\) (common)

⇒ \(\angle A C B=\angle D A B\) (each \(90^{\circ}\))

⇒ \(\triangle A B C \sim \triangle D B A\)(A.A. similarity)

⇒ \(\frac{A B}{D B}=\frac{B C}{B A} \Rightarrow A B^2=B C \cdot B D\)

Hence Proved.

2. In \(\triangle A B C\) and \(\triangle D A C\),

⇒ \(\angle A B C=180^{\circ}-\angle A C B-\angle B A C\)

= \(180^{\circ}-90^{\circ}-\angle B A C\)

= \(90^{\circ}-\angle B A C=\angle D A C\)

and \(\angle A C B=\angle D C A\) (each \(90^{\circ}\))

⇒ \(\triangle A B C \sim \triangle D A C\) (A.A. similarity)

⇒ \(\frac{A C}{D C}=\frac{B C}{A C} \Rightarrow A C^2=B C \cdot D C\)

Hence Proved.

3.In \(\triangle D A C\) and \(\triangle D B A\),

⇒ \(\angle A D C=\angle B D A\) (common)

⇒ \(\angle D C A=\angle D A B\) (each \(90^{\circ}\))

⇒ \(\triangle D A C \sim \triangle D B A\) (A.A. similarity)

⇒ \(\frac{D A}{D B}=\frac{D C}{D A} \Rightarrow A D^2=B D \cdot C D\)

Hence Proved.

Question 4.ABC is an isosceles triangle right angled at C. Prove that \(A B^2=2 A C^2\).
Solution:

Given

ABC is an isosceles triangle right angled at C.

In \(\triangle A B C\),

AC = BC

and \(\angle C=90^{\circ}\)

⇒ \(A B^2=A C^2+B C^2\)

⇒ \(A B^2=A C^2+A C^2\) [from equation (1)]

⇒ \(A B^2=2 A C^2\)

Hence Proved.

Question 5. ABC is an isosceles triangle with AC = BC. If \(A B^2=2 A C^2\), prove that ABC is a right triangle.
Solution:

Given

ABC is an isosceles triangle with AC = BC. If \(A B^2=2 A C^2\)

In \(\triangle A B C\),

and AC = BC

⇒ \(A B^2\) =2 A C^2[/latex]

⇒ \(A B^2\) = \(A C^2+A C^2\)

⇒ \(A B^2\) and \(=A C^2+B C^2\)

⇒ \(\angle C\) and \(=90^{\circ}\) (from equation (1))

⇒ \(\angle C=90^{\circ}\)

⇒ \(\triangle A B C\) is a right-angled triangle.

Hence Proved.

Question 6. ABC is an equilateral triangle of side 2a. Find each of its altitudes.
Solution:

Given

ABC is an equilateral triangle of side 2a.

Let ABC be an equilateral triangle in which,

AB = BC = CA = 2a

AB = BC = CA = 2a

AP \(\perp\) BC

BP = \(\frac{1}{2} B C\)

= \(\frac{1}{2}(2 a)=a\)

In \(\triangle A B P\),

⇒ \(A B^2=A P^2+B P^2\)

⇒ \(A P^2=A B^2-B P^2=(2 a)^2-a^2=3 a^2\)

⇒ \(A P=a \sqrt{3}\)

Length of each altitude = \(a \sqrt{3}\) units.

Question 7. Prove that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals.
Solution:

Let ABCD be a rhombus whose side is ‘a’

The diagonals of the rhombus intersect each other at point O at right angle.

In right \(\triangle\) A O B,

⇒ \(O A^2+O B^2=a^2\) (from Pythagoras theorem)

⇒ \((\frac{1}{2} A C)^2+\left(\frac{1}{2} B D\right)^2=a^2\) (diagonals bisect each other)

⇒ \(\frac{1}{4} A C^2+\frac{1}{4} B D^2=a^2\)

⇒ \(A C^2+B D^2=4 a^2\)

⇒ \(A C^2+B D^2=a^2+a^2+a^2+a^2\)

⇒ \(A C^2+B D^2=A B^2+B C^2+C D^2+D A^2\)

Hence Proved

Question 8. In the figure, O is A a point in the interior of a triangle ABC, OD \(\perp\) BC, OE \(\perp\) AC and OF \(\perp\) AB. Show that :

1.  \(O A^2+O B^2+O C^2= O D^2-O E^2-O F^2=A F^2 +B D^2+C E^2 \)
2.  \(A F^2+B D^2+C E^2=A E^2+C D^2+B F^2\)

Solution:

Given

In the figure, O is A a point in the interior of a triangle ABC, OD \(\perp\) BC, OE \(\perp\) AC and OF \(\perp\) AB.

(1) In \(\triangle A O F\),

⇒ \(A F^2=O A^2-O F^2\)

In \(\triangle B O D\),

⇒ \(B D^2=O B^2-O D^2\)

In \(\triangle C O E\),

⇒ \(C E^2=O C^2-O E^2\)

Adding equations (1), (2) and (3),

⇒ \(A F^2 +B D^2+C E^2\)

=\(O A^2+O B^2+O C^2-O F^2-O D^2-O E^2\)

Hence Proved.

(2) From part 1

\(A F^2+B D^2+C E^2\)

= \(O A^2+O B^2+O C^2-O F^2-O D^2-O E^2\)

= \(\left(O A^2-O E^2\right)+\left(O B^2-O F^2\right)+\left(O C^2-O D^2\right)\)

=\(A E^2+B F^2+C D^2\)

Hence Proved.

Question 9. A ladder 10 m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from the base of the wall.

Solution:

Given

A ladder 10 m long reaches a window 8 m above the ground.

Let PR is a ladder of length 10 m which reaches at a window of height 8 m from the ground. The distance of the lower end of the ladder from the base of the wall is QR.

Now, \(\angle P Q R=90^{\circ}\)

In \(\triangle P Q R\),

⇒ \(P R^2=P Q^2+Q R^2\)

⇒ \(Q R^2=P R^2-P Q^2\)

=\(10^2-8^2\)

=100-64=36

Distance of the lower end of the ladder from the base of the wall = 6 m

Question 10. A guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?

Solution:

Given

A guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end.

Let PQ be a vertical pole of height 18 m. A wire whose length is 24 m, is tied at the upper-end P of the pole. Its other end is tied at point R on the ground.

In \(\triangle P Q R\),

⇒ \(Q R^2+P Q^2 =P R^2\)

⇒ \(Q R^2 =P R^2-P Q^2=24^2-18^2\)

=576-324=252

Q R =\(\sqrt{252}=6 \sqrt{7} \mathrm{~m}\)

Question 11. An aeroplane leaves an airport and flies due north at a speed of 1000 km per hour. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 same airport and flies due west at a speed of 1200 km per hour. How far apart will be the two planes after \(1 \frac{1}{2}\) hours?

Solution:

Given

An aeroplane leaves an airport and flies due north at a speed of 1000 km per hour. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 same airport and flies due west at a speed of 1200 km per hour.

Distance covered by aeroplane in North in 1 \(\frac{1}{2}=\frac{3}{2}\) hrs is A B=1000 \(\times \frac{3}{2}=1500 \mathrm{~km}\).

Similarly, the distance covered by aeroplane in the west in \(\frac{3}{2} \mathrm{hrs}\).

⇒ \(B C=1200 \times \frac{3}{2}=1800 \mathrm{~km}\)

In \(\triangle A B C, A C^2=A B^2+B C^2\)

=\((1500)^2+(1800)^2\)

= 2250000+3240000 = 5490000

∴ \(A C=\sqrt{5490000}=300 \sqrt{61} \mathrm{~km}\)

Question 12. Two poles of heights 6 m and 1 1 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.

Solution :

Given

Two poles of heights 6 m and 1 1 m stand on a plane ground. If the distance between the feet of the poles is 12 m,

Let AB and CD be two vertical poles, and then

AB = 6 m, CD = 11m

and AC = 12m

Draw BE || AC, then

CE = AB = 6 m,

BE = AC = 12 m

DE = CD- CE = 11m – 6m = 5m

Now in right \(\triangle B E D\),

⇒ \(B D^2 =B E^2+D E^2\)

=\(12^2+5^2\)

=144+25

=169 \(\mathrm{~m}^2 \Rightarrow B D=13 m\)

Therefore, the distance between poles =13 m.

Question 13. D and E are points on the sides CA and CB respectively of a triangle ABC right angled at C . Prove that \( A E^2+B D^2=A B^2+D E^2\).

Solution :

Given

D and E are points on the sides CA and CB respectively of a triangle ABC right angled at C .

In \(\triangle A C E, A E^2=A C^2+C E^2\) …..(1)

In \(\triangle A B C, \quad A B^2=A C^2+B C^2\) …. (2)

In \(\triangle C D E, \quad D E^2=C D^2+C E^2\) ….(3)

In \(\triangle D C B, \quad B D^2=C D^2+B C^2\) …..(4)

Adding equations (1) and (4),

⇒ \(A E^2+B D^2= \left(A C^2+C E^2\right)+\left(C D^2+B C^2\right)\)

= \(\left(A C^2+B C^2\right)+\left(C D^2+C E^2\right)\)

= \(A B^2+D E^2\) [from equations (2) and (3)]

Hence Proved.

Question 14. The perpendicular from A on side BC of a \(\triangle\)ABC intersects BC at D such that DB = 3 CD. Prove that 2AB² = 2AC² + BC²

Solution:

Given, In \(\triangle A B C\), A D \(\perp B C\) and D B=3 C D

To prove : \(2 A B^2=2 A C^2+B C^2\)

Proof: \(D B=3 C D \Rightarrow \frac{D B}{C D}=\frac{3}{1}\)

Let \(D B=3 x \quad \Rightarrow \quad C D\)=x

⇒ \(\frac{D B}{B C}=\frac{3 x}{4 x}=\frac{3}{4} \quad \Rightarrow \quad D B=\frac{3}{4} B C\)

and \(\frac{D C}{B C}=\frac{x}{4 x}=\frac{1}{4} \quad \Rightarrow \quad D C=\frac{1}{4} B C\)…

Now, from Pythagoras theorem,

⇒ \(A B^2=A D^2+B D^2=\left(A C^2-D C^2\right)+B D^2\) (from Pythagoras theorem)

=\(A C^2-\frac{1}{16} B C^2+\frac{9}{16} B C^2\)

=\(A C^2+\frac{8}{16} B C^2=A C^2+\frac{1}{2} B C^2\)

⇒ \(2 A B^2=2 A C^2+B C^2\)

Hence Proved.

Question 15. In an equilateral triangle ABC, D is a point on side BC Such That B D=\(\frac{1}{3} B C\). Prove that \(9 A D^2=7 A B^2\).

Solution :

Given

In an equilateral triangle ABC, D is a point on side BC Such That B D=\(\frac{1}{3} B C\).

Let \(A B=B C=A C=6 x\)

and \(B D=\frac{1}{3} B C=\frac{1}{3} \times 6 x=2 x\)

and B E=E C=\(\frac{B C}{2}\)=3 x ( In equilateral triangle, perpendicular bisects the base)

D E=B E-B D=3 x-2 x=x

Now, from Pythagoras theorem,

⇒ \(A B^2= A E^2+B E^2\)

= \(A D^2-D E^2+B E^2\) (from Pythagoras theorem)

⇒ \((6 x)^2 =A D^2-x^2+(3 x)^2\)

⇒ \(A D^2 =36 x^2+x^2-9 x^2=28 x^2\)

⇒ \(9 A D^2= 9 \times 28 x^2=9 \times 7 \times 4 x^2\)

=\(v7 \times 36 x^2=7(A B)^2\)

⇒ \(9 A D^2 =7 A B^2\)

Hence Proved.

Question 16. In an equilateral triangle, prove that three times the square of one, side is equal to four times the square of one of its altitudes.

Solution:

Let AB=BC = CA=a

In \(\triangle\)ADB and \(\triangle\)ADC,

⇒ \({\begin{array}{lr}
A B=A C \text { (sides of equilateral triangle) } \\
\angle 1=\angle 2 & \text { (each } 90^{\circ} \text { ) } \\
A D=A D & \text { (common) }
\end{array}}. \)

⇒ \(\triangle A D B \cong \triangle A D C\) (R.H.S.)

BD = DC =\(\frac{B C}{2}=\frac{a}{2}\) (c.p.c.t.)

Now, in \(\triangle A D B\) , from Pythagoras theorem

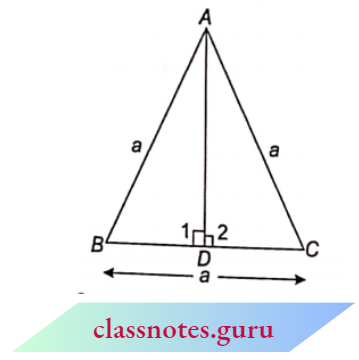
⇒ \(A B^2=A D^2+B D^2\)

⇒ \(a^2=A D^2+\left(\frac{a}{2}\right)^2\)

⇒ \(A D^2=a^2-\frac{a^2}{4} \Rightarrow A D^2=\frac{3 a^2}{4}\)

⇒ \(4 A D^2=3 a^2\)

⇒ \(4 A D^2=3 A B^2\)

Therefore, three times the square of the side of an equilateral triangle is equal to 4 times the square of its altitude.

Hence Proved

Question 17. Tick the correct answer and justify: In \(\triangle A B C\), A B=6 \(\sqrt{3} \mathrm{~cm}\), A C=12 cm and B C=6 \cm. The angle B is :

1. \(120^{\circ}\)
2. \(60^{\circ}\)
3. \(90^{\circ}\)
4. \(45^{\circ}\)

Solution :

⇒ \(A B^2+B C^2 =(6 \sqrt{3})^2+6^2\)

=\(108+36=144=12^2=A C^2\)

⇒ \(\angle B =90^{\circ}\)

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle Exercise 6.6

Question 1. n figure, PS is the bisector of \(\angle\)QPR of \(\triangle\)PQR. Prove that \(\frac{Q S}{S R}=\frac{P Q}{P R}\)

Solution:

Solution: Draw R T \\ S P which intersects the produced QP at T.

Now, R T \\ S P and R P is a transversal.

⇒ \(\angle S P R=\angle P R T\) (alternate angles) …(1)

Again, SP \\ RT and QT is a transversal.

⇒ \(\angle Q P S=\angle P T R\)

Given, \(\angle Q P S=\angle S P R \Rightarrow \angle P T R=\angle P R T\) [from equations (1) and (2)]

P R=P T

In \(\triangle Q R T\), S P \\ R T

⇒ \(\frac{Q S}{S R}=\frac{Q P}{P T} \quad \Rightarrow \quad \frac{Q S}{S R}=\frac{P Q}{P R}\) [from equation (3)]

Hence proved

Question 2. In the figure, D is a point on hypotenuse AC of \(\triangle\) ABC, such that BD \( \perp\) AC, DM \( \perp\) BC and DN \( \perp\) AB. Prove that :

1. \(D M^2=D N \cdot M C\)
2. \(D N^2=D M \cdot A N\)

Solution :

In right \(\triangle A B C\), BD is perpendicular to A-C.

⇒ \(\triangle B D C \sim \triangle A B C \sim \triangle A D B\)

(1) In \(\triangle B D C\)

⇒ \(\triangle D M \perp \sim B C\)

⇒ \(\frac{M C}{D M}=\frac{M D}{B M}\)

⇒ \(D M^2=B M \cdot M C\)

In square B M D N, \(\quad \angle D=\angle M=\angle N=90^{\circ}\)

⇒ In square B M D N is a rectangle. From equation (1),

⇒ \(D M^2=D N \cdot M C\)

Hence Proved.

(2) In \(\triangle A D B, D N \perp A B\)

In \(\triangle A N D \sim \triangle D N B, \frac{D N}{B N}=\frac{A N}{D N}\)

⇒ \(D N^2=B N \cdot A N\)

but B N=D M,

⇒ \(D N^2=D M \cdot A N\)

Hence Proved.

Question 3. Figure A B C is a triangle in which \(\angle A B C>90^{\circ}\) and \(A D \perp C B\) are produced. Prove that \(A C^2=A B^2+B C^2+2 B C \cdot B D\).

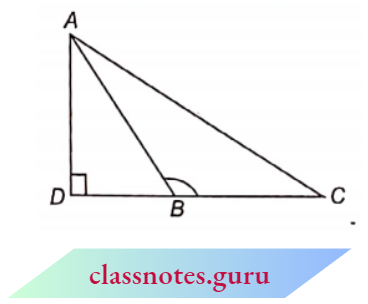
Solution :

An obtuse-angled triangle A B C in which \(\angle B\) is an obtuse angle and AD is perpendicular to produce C B,

To prove : \(A C^2=A B^2+B C^2+2 B C \times B D\)

Proof : \(\triangle A D C\) is the right triangle.

In \(\triangle A D C\), from Pythagoras theorem,

⇒ \(A C^2=A D^2+D C^2\)

⇒ \(A C^2=A D^2+(D B+B C)^2\) (D C=D B+B C)

⇒ \(A C^2=A D^2+D B^2+B C^2+2 D B \cdot B C\)

⇒ \(A C^2=\left(A D^2+D B^2\right)+B C^2+2 B C \cdot B D\)

⇒ \(A C^2=A B^2+B C^2+2 B C \cdot B D\)

Hence Proved.

Question 4. In figure, \(\triangle\)ABC is a triangle in which \(\angle\)ABC < 90° and AD \(\perp\) BC. Prove that AC² = AB² + BC² – 2BC • BD.

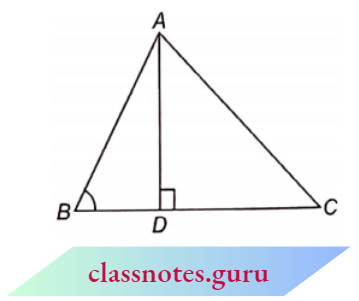
Solution:

Given: \(\triangle A B C\) is an acute-angled triangle and \(A D \perp B C\).

To prove: \(A C^2=A B^2+B C^2-2 B C \times B D\)

Proof: In \(\triangle A D C, \angle D=90^{\circ}\). So from Pythagoras theorem,

⇒ \(A C^2=\left(A D^2+B D^2\right)+B C^2-2 B C \times B D\)

⇒\(A C^2=A B^2+B C^2-2 B C \times B D\) (\(A D^2+B D^2=A B^2\))

Hence Proved.

Question 5. Figure AD is a median of a triangle ABC and AM \(\perp\) BC. Prove that

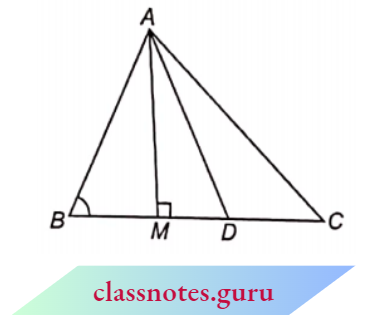
1. \(A C^2=A D^2+B C \cdot D M+\left(\frac{B C}{2}\right)^2 \)
2. \(A B^2=A D^2-B C \cdot D M+\left(\frac{B C}{2}\right)^2\)
3. \(A C^2+A B^2=2 A D^2+\frac{1}{2} B C^2\)

Solution:

⇒\(\triangle A B C\) is acute angled.

AD is a median.

B D=C D=\(\frac{1}{2} B C\)

AM is the altitude.

(1) In \(\triangle A C M\) ,

⇒ \(A C^2=A M^2+M C^2\)

⇒ \(A C^2=A M^2+(M D+C D)^2\)

= \(A M^2+M D^2+2 M D \cdot C D+C D^2\)

= \(\left(A M^2+D M^2\right)+(2 \cdot C D) \cdot D M+C D^2\)

= \(A D^2+(B C) \cdot D M+\left(\frac{1}{2} B C\right)^2\)

∴ \(A C^2=A D^2+B C \times D M+\frac{1}{4} B C^2\)

(2) In \(\triangle A B M\),

⇒ \(A B^2=A M^2+B M^2\)

= \(A M^2+(B D-D M)^2\)

= \(A M^2+B D^2+D M^2-2 B D \cdot D M\)

= \(A M^2+D M^2+\left(\frac{1}{2} B C\right)^2-B C \cdot D M\)

∴ \(A B^2 =A D^2-B C \times D M+\frac{1}{4} B C^2\)

Hence Proved.

(3) Adding equations (2) and (3),

⇒ \(A C^2+A B^2=2 A D^2+\frac{1}{2} B C^2\)

Hence Proved.

Question 6. Prove that the sum of the squares of the diagonals of a parallelogram is equal to the sum of the squares of its sides

Solution:

Draw DE \(\perp\) AB and CF perpendicular to produce AB.

In \(\triangle A E D\) and \(\triangle B F C\),

AD = B C (opposite sides of the parallelogram)

⇒ \(\angle D E A =\angle C F B\) (each \(90^{\circ}\))

D E =C F (perpendicular distance between two parallel lines)

⇒ \(\triangle A E D \cong \triangle B F C\)

An E=B F (corresponding parts of congruent triangles)

= \((A F^2+C F^2)+\left(D E^2+B E^2\right)\) (from Pythagoras theorem)

= \((A B+B F)^2+\left(B C^2-B F^2\right)+\left(A D^2-A E^2\right)+(A B-A E)\) (from Pythagoras theorem)

= \(A B^2+B F^2+2 A B \cdot B F+B C^2 -B F^2+A D^2-A E^2+A B^2+A E^2 -2 A B \cdot A E\)

= \(A B^2+2 A B \cdot A E+B C^2 +A D^2+C D^2-2 A B \cdot A E\)(A E=B F and A B=C D)

= \(A B^2+B C^2+C D^2+D A^2\)

Now, L.H.S. = \(A C^2+B D^2\)

Hence Proved.

Question 7. In the figure, two chords AB and CD intersect each other at point p. Prove that :

1. \(\triangle A P C=\triangle D P B\)
2. \(A P \cdot P B=C P \cdot D P\)

Solution:

(1) In \(\triangle P^P C\) and \(\triangle D P B\),

⇒ \(\angle A P C=\angle D P B\) (vertically opposite angles)

⇒ \(\angle C A P=\angle B D P\) (angles of same segment)

⇒ \(\triangle A P C \sim \triangle D P B\) (from A.A. similarity)

Hence Proved.

(2) From part (1),

⇒ \(\triangle A P C \sim \triangle D P B \Rightarrow \frac{A P}{D P}=\frac{C P}{P B}\)

⇒ \(A P \cdot P B=C P \cdot D P\)

Hence Proved.

Question 8. In the figure, two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that

1. \(\triangle\)PAC – \(\triangle\)PDB
2. PA. PB = PC. PD

Solution:

(1) In \(\triangle P A C\) and \(\triangle P D B\),

⇒ \(\angle A P C =\angle D P B\) (common)

⇒ \(\angle P A C =180^{\circ}-\angle B A C\)

=\(\angle P D B\)

⇒ \(\Delta P A C \sim \triangle P D B\) (from A.A. similarity)

(2) \(\Delta P A C \sim \triangle P D B\)

⇒ \(\frac{A P}{D P} =\frac{P C}{P B}\)

⇒ \(P A \cdot P B =P C \cdot P D \)

Hence Proved.

Question 9. In the figure, D is a point on side BC of \(\triangle\) ABC such that \(\frac{B D}{C D}=\frac{A B}{A C}\). Prove that AD is the bisector of \(\angle\)BAC

Solution:

Produce BA to M such that

A M=A C

Join CM.

In \(\triangle M C\),

A M =A C

A C M =\(\angle A M C\) .

and \(\frac{B D}{C D}=\frac{A B}{A C}\)

⇒ \(\frac{B D}{C D}=\frac{B A}{A M}\) (given) from equation ( 1 ]

D A \\ C M

Now, \(\angle B A D=\angle A M C\) (corresponding angles)

and \(\angle D A C=\angle A C M\) (alternate angles)

From equations (2), (3) and (4)

⇒ \(\angle D A C=\angle B A D\)

A D bisects \(\angle B A C\).

Hence Proved.

Question 10. Nazima is fly Fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the rod. Assuming that her string (from the tip of her rod to the fly) is taut, how much string does she have out (common) (see figure)? If she pulls in the string at the rate of 5 cm per second, what will be the horizontal distance of the fly from her after 12 seconds?

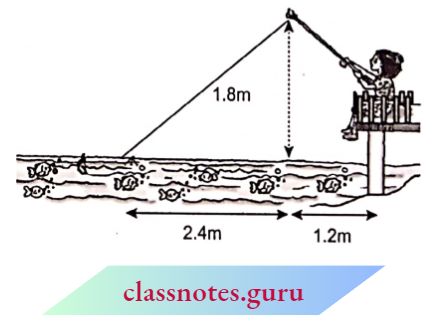
Solution:

Description of figure :

C → tip of fishing rod DC

AC string, AF → fly

The initial length of the string

A C=\(\sqrt{(180)^2+(240)^2}\) (from Pythagoras theorem)

A C=\(\sqrt{32400+57600}\)

=300 cm

But, When Nazima pulls the string at the rate of 5 cm/sec, she pulls it 5 x 12 cm in 12 seconds.

So she pulls a 60 cm string from C to D. The remaining string CA will now CG – 300 – 60 = 240 cm because when the string pulls then its tip and fly both will move from A to G. So the horizontal distance of fly G from Nazima E will be EG.

Now, \([latex]G B^2= C G^2-C B^2\) (from Pythagoras theorem)

=\((240)^2-(180)^2\)

=57600-32400=25200

60 \(\times 2.64575\)=158.745 cm

Required distance =E G=G B+B E

=158.745+120

= 278.745 cm =2.79 m (approximately)

NCERT Exemplar Solutions For Class 10 Maths Chapter 6 Triangle Multiple Choice Question And Answers

Question 1. The length of the diagonals of a rhombus is 16 cm and 12 cm. The length of its side is :

1. 9 cm
2. 10 cm
3. 12 cm
4. 8 cm

Answer: 2. 10cm

Question 2. In \(\triangle A B C\) and \(\triangle P Q R\), \(\frac{A B}{Q R}=\frac{B C}{P R}=\frac{C A}{P Q}\), then

1. \(\triangle P Q R \sim \triangle C A B\)
2. \(\triangle P Q R \sim \triangle A B C\)
3. \(\triangle P Q R \sim \triangle C B A\)
4. \(\triangle P Q R \sim \triangle B C A\)

Answer: 1. \(\triangle P Q R \sim \triangle C A B\)

Question 3. Points D and E are on the sides AB and AC respectively of an \(\triangle\)ABC such that AD = 2 cm, BD = 3 cm, BC = 7.5 cm and DE || BC, then the length of DE is :

1. 2.5 cm
2. 3 cm
3. 5 cm
4. 6 cm

Answer: 2. 3cm

Question 4. In \(\triangle\)ABC and \(\triangle\)DEF, \(\angle\)A = \(\angle\)D, \(\angle\)F = \(\angle\)C and AB = 3DE then two triangles are :

1. congruent but not similar
2. similar but not congruent
3. neither congruent nor similar
4. congruent and similar both

Answer: 2. Similar but not congruent

Question 5. In \(\triangle\)ABC and \(\triangle\)DEF, \(\frac{A B}{D E}=\frac{B C}{F D}\)then these triangles will be similar if:

1. \(\angle B=\angle E\)
2. \(\angle A=\angle D\)
3. \(\angle B=\angle D\)
4. \(\angle A=\angle\)

Answer: 3. \(\angle B=\angle D\)

Question 6. S is a point on side PQ of \(\triangle P Q R\) such that P S=Q S=R S, then :

1. \(P Q \cdot Q R=R S^2\)
2. \(P R^2+Q R^2=P Q^2\)
3. \(Q S^2+R S^2=Q R^2\)
4. \(P S^2+R S^2=P R^2\)

Answer: 3. \(Q S^2+R S^2=Q R^2\)

Question 7. If \(\triangle\)ABC ~ \(\triangle\)PQR and BC = 5QR then ar(\(\triangle\)ABC) : ar(\(\triangle\)PQR) is :

1. 1: 25
2. 25: 1
3. 1: 5
4. 5: 1

Answer: 1. 1: 25

Question 8. The areas of two similar triangles are in the ratio 4: 9. The ratio on the sides of the triangle will be :

1. 2 : 3
2. 4: 9
3. 81: 16
4. 16: 81

Answer: 1. 2 : 3

Question 9. The side of an equilateral triangle is 2a. The length of each altitude will be :

1. \(a \sqrt{2}\)
2. \(2 a \sqrt{3}\)
3. \(a \sqrt{3}\)
4. 3a

Answer: 3. \(a \sqrt{3}\)

Question 10. The corresponding sides of two similar triangles are in the ratio 9:4. The ratio of the areas of these triangles will be :

1. 2 : 3
2. 4: 9
3. 81: 16
4. 16:81

Answer: 3. 81: 16

Question 11. the following figure, a line segment PQ is drawn parallel to base BC of \(\triangle\)ABC. If PQ: BC = 1 : 3 then the ratio ofAP and PB will be :

1. 1: 4
2. 1 : 3
3. 1: 2
4. 2 : 3

Answer: 3. 1: 2