Class 12 Maths

• Algebra
• Binomial Distribution Formula in Probability with Examples
• Relations And Functions
• Probability
• Probability Distribution
• Differential Calculus
• Bayes Theorem And Its Applications

Differential Calculus

Differential Calculus Exercise 1 Multiple Choice Questions

Question 1. If y = 2^x,then \(\frac{d y}{d x}\)= ?

1. \(x\left(2^{x-1}\right)\)
2. \(\frac{2^x}{(\log 2)}\)
3. \(2^x(\log 2)\)
4. None of these

Answer:  3. \(2^x(\log 2)\)

⇒ \(\frac{d y}{d x}=2^x(\log 2)\)

Question 2. If y = \(\log _{10} x, \text { then } \frac{d y}{d x}=?\)

1. \(\frac{1}{x}\)
2. \(\frac{1}{x}\)(log 10)
3. \(\frac{1}{x(\log 10)}\)
4. None of these

Answer: 3. \(\frac{1}{x(\log 10)}\)

⇒ \(y=\frac{\log x}{\log 10}\)

Read and Learn More WBCHSE Solutions For Class 12 Maths

Question 3. \(\text { If } y=e^{1 / x} \text {, then } \frac{d y}{d x}=?\)

1. \(\frac{1}{x} \cdot e^{(1 / x-1)}\)
2. \(\frac{-e^{1 / x}}{x^2}\)
3. \(e^{1 / x} \log x\)
4. None of these

Answer: 2. \(\frac{-e^{1 / x}}{x^2}\)

⇒ \(\frac{d y}{d x}=\frac{e^{1 / x}}{-x^2}\)

Question 4. \(\text { If } y=x^x \text {, then } \frac{d y}{d x}=?\)

1. x^x log x*
2. x^x (1+log x)
3. x (1+log x)
4. None of these

Answer: 2. x^x (1+log x)

log y= x^x log x. Now differentiate w.r.t. x.

Question 5. \(\text { If } y=x^{\sin x}, \text { then } \frac{d y}{d x}=?\)

1. \((\sin x) \cdot x^{(\sin x-1)}\)
2. \((\sin x \cos x) \cdot x^{(\sin x-1)}\)
3. \(x^{\sin x}\left\{\frac{\sin x+x \log x \cdot \cos x}{x}\right\}\)
4. None of these

Answer: 3. \(x^{\sin x}\left\{\frac{\sin x+x \log x \cdot \cos x}{x}\right\}\)

logy= sin x(logx)

Question 6. \(\text { If } y=x^{\sqrt{x}} \text {, then } \frac{d y}{d x}=?\)

1. \(\sqrt{ } x \cdot x^{(\sqrt{ } x-1)}\)
2. \(\frac{x^{\sqrt{x}} \log x}{2 \sqrt{x}}\)
3. \(x^{\sqrt{x}}\left\{\frac{2+\log x}{2 \sqrt{ } x}\right\}\)
4. None of these

Answer: 3. \(x^{\sqrt{x}}\left\{\frac{2+\log x}{2 \sqrt{ } x}\right\}\)

log y= \(\sqrt{ } x(\log x)\)

Question 7. \(\text { If } y=e^{\sin \sqrt{x}} \text {, then } \frac{d y}{d x}=?\)

1. \(e^{\sin \sqrt{ } x} \cdot \cos \sqrt{x}\)
2. \(\frac{e^{\sin \sqrt{x}} \cos \sqrt{x}}{2 \sqrt{x}}\)
3. \(\)
4. None of these

Answer: 2. \(\frac{e^{\sin \sqrt{x}} \cos \sqrt{x}}{2 \sqrt{x}}\)

log y= sin \(\sqrt{ } x\)

Question 8. If y (tanx)^{cot x}, then \(\frac{d y}{d x}\)=?

1. cot x. (tan x)^{cot x-1}.sec²x
2. -(tan x)^{cot x} . cosec²x
3. (tan x)^{cot x}. cosec²x(l- log tan x)
4. None of these

Answer: 3. (tan x)^{cot x}. cosec²x(l- log tan x)

log y= cot x .log (tan x)

Question 9. If y= (sin x)log^x, then\(\frac{d y}{d x}\) =?

1. (logx). (sinx)^(logx-1). cos x
2. (sin x)^logx . \(\left\{\frac{x \log x+\log \sin x}{x}\right\}\)
3. (sin x)^logx . \(\left\{\frac{(x \log x) \cot x+\log \sin x}{x}\right\}\)
4. None of these

Answer: 3. (sin x)^logx . \(\left\{\frac{(x \log x) \cot x+\log \sin x}{x}\right\}\)

log y= (log x). (log sin x)

Question 10. If y = sin(x^x),then \(\frac{d y}{d x}\) = ?

1. x^xcos (x^x)
2. x^x cos x^x (1+logx)
3. x^xcos x^xlog ^x
4. None of these

Answer: 2. x^x cos x^x (1+logx)

Let x^x = z. Then, y= sin z. Then, \(\frac{d y}{d x}=\left(\frac{d y}{d z} \times \frac{d z}{d x}\right)\)

Question 11. If y= \(\sqrt{x \sin x}\) then \(\frac{d y}{d x}\) = ?

1. \(\frac{(x \cos x+\sin x)}{2 \sqrt{x \sin x}}\)
2. \(\frac{1}{2}(x \cos x+\sin x) \cdot \sqrt{x \sin x}\)
3. \(\frac{1}{2 \sqrt{x \sin x}}\)
4. None of these

Answer: 1. \(\frac{(x \cos x+\sin x)}{2 \sqrt{x \sin x}}\)

y² = x sin x

= 2y . \(\frac{d y}{d x}\)

= (xcos + sinx).

Question 12. If e^x+y= xy, then \(\frac{d y}{d x}\) = ?

1. \(\frac{x(1-y)}{y(x-1)}\)
2. \(\frac{y(1-x)}{x(y-1)}\)
3. \(\frac{(x-x y)}{(x y-y)}\)
4. None of these

Answer: 2. \(\frac{y(1-x)}{x(y-1)}\)

(x+ y)= log x + logy

= \(1+\frac{d y}{d x}=\frac{1}{x}+\frac{1}{y} \cdot \frac{d y}{d x}\)

Question 13. If (x + y) = sin (x+ y), then \(\frac{d y}{d x}\) = ?

1. -1
2. 1
3. \(\frac{1-\cos (x+y)}{\cos ^2(x+y)}\)
4. None of these

Answer: 1. -1

(x + y) = sin(x + y) ⇒ \(1+\frac{d y}{d x}=\cos (x+y) \cdot\left[1+\frac{d y}{d x}\right]\)

Question 14. If \(\sqrt{ } x+\sqrt{ } y=\sqrt{ } a\),then \(\frac{d y}{d x}\) = ?

1. \(\frac{-\sqrt{x}}{\sqrt{y}}\)
2. \(-\frac{1}{2} \cdot \frac{\sqrt{y}}{\sqrt{x}}\)
3. \(\frac{-\sqrt{y}}{\sqrt{x}}\)
4. None of these

Answer: 3. \(\frac{-\sqrt{y}}{\sqrt{x}}\)

⇒ \(\sqrt{ } x+\sqrt{ } y=\sqrt{ } a \Rightarrow \frac{1}{2 \sqrt{ } x}+\frac{1}{2 \sqrt{ } y} \cdot \frac{d y}{d x}\)= 0

Question 15. x^y= y^x,then \(\frac{d y}{d x}\) =?

1. \(\frac{(y-x \log y)}{(x-y \log x)}\)
2. \(\frac{y(y-x \log y)}{x(x-y \log x)}\)
3. \(\frac{y(y+x \log y)}{x(x+y \log x)}\)
4. None of these

Answer: 2. \(\frac{y(y-x \log y)}{x(x-y \log x)}\)

x^y = y^x ⇒ y log x= x logy. Now, differentiate both sides w.r.t. x.

Question 16. If x^py^q= (x + y)^y(p+q) ,then \(\frac{d y}{d x}\) = ?

1. \(\frac{x}{y}\)
2. \(\frac{y}{x}\)
3. \(\frac{x^{p-1}}{y^{q-1}}\)
4. None of these

Answer:  2. \(\frac{y}{x}\)

plog x+ qlogy= (p + q) log(x+ y).

Question 17. If y = \(x^2 \sin \frac{1}{x}\), then\(\frac{d y}{d x}\) = ?

1. \(x \sin \frac{1}{x}-\cos \frac{1}{x}\)
2. \(-\cos \frac{1}{x}+2 x \sin \frac{1}{x}\)
3. \(-x \sin \frac{1}{x}+\cos \frac{1}{x}\)
4. None of these

Answer: 2. \(-\cos \frac{1}{x}+2 x \sin \frac{1}{x}\)

⇒ \(\frac{d y}{d x}=x^2 \cdot\left(\cos \frac{1}{x}\right)\left(\frac{-1}{x^2}\right)+2 x \sin \frac{1}{x}\)

Question 18. If y = cos² x³, then \(\frac{d y}{d x}\) = ?

1. – 3x²sin(2x³)
2. -3x² sin² x³
3. -3x² cos²(2x³)
4. None of these

Answer: 1. – 3x²sin(2x³)

y = \(\left(\cos x^3\right)^2 \Rightarrow \frac{d y}{d x}\)

=2\(\left(\cos x^3\right)\left(-\sin x^3\right)\left(3 x^2\right)\)

=-3x² \(\sin \left(2 x^3\right)\)

Question 19. If y = \(\log \left(x+\sqrt{x^2+a^2}\right)\), then \(\frac{d y}{d x}\) =?

1. \(\frac{1}{2\left(x+\sqrt{x^2+a^2}\right)}\)
2. \(\frac{-1}{\sqrt{x^2+a^2}}\)
3. \(\frac{1}{\sqrt{x^2+a^2}}\)
4. None of these

Answer: 3. \(\frac{1}{\sqrt{x^2+a^2}}\)

⇒ \(\frac{d y}{d x}=\frac{1}{\left(x+\sqrt{x^2+a^2}\right.} \cdot\left\{1+\frac{1}{2 \sqrt{x^2+a^2}} \times 2 x\right\}\)

= \(\frac{1}{\sqrt{x^2+a^2}}\)

Question 20. If y \(=\log \left(\frac{1+\sqrt{x}}{1-\sqrt{x}}\right)\) then\(\frac{d y}{d x}\)= ?

1. \(\frac{1}{\sqrt{x}(1-x)}\)
2. \(\frac{-1}{x(1-\sqrt{x})^2}\)
3. \(\frac{-\sqrt{ } x}{2(1-\sqrt{ } x)}\)
4. None of these

Answer: 1. \(\frac{1}{\sqrt{x}(1-x)}\)

y= \(\log (1+\sqrt{ } x)-\log (1-\sqrt{ } x)\)

= \(\frac{d y}{d x}=\left\{\frac{1}{2 \sqrt{ } x(1+\sqrt{ } x)}+\frac{1}{2 \sqrt{ } x(1-\sqrt{ } x)}\right\}\)

= \(\frac{1}{2 \sqrt{x}} \cdot \frac{2}{(1-x)}=\frac{1}{\sqrt{x(1-x)}}\)

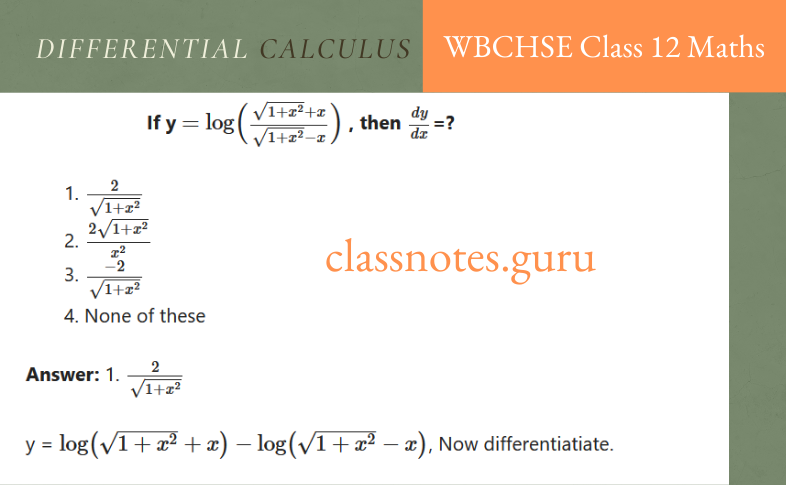
Question 21. If y \(=\log \left(\frac{\sqrt{1+x^2}+x}{\sqrt{1+x^2}-x}\right)\) , then \(\frac{d y}{d x}\)=?

1. \(\frac{2}{\sqrt{1+x^2}}\)
2. \(\frac{2 \sqrt{1+x^2}}{x^2}\)
3. \(\frac{-2}{\sqrt{1+x^2}}\)
4. None of these

Answer: 1. \(\frac{2}{\sqrt{1+x^2}}\)

y = \(\log \left(\sqrt{1+x^2}+x\right)-\log \left(\sqrt{1+x^2}-x\right)\), Now differentiatiate.

Question 22. If y= \(\sqrt{\frac{1+\sin x}{1-\sin x}}\), then \(\frac{d y}{d x}\)=?

1. \(\frac{1}{2} \sec ^2\left(\frac{\pi}{4}-\frac{x}{2}\right)\)
2. \(\frac{1}{2}{cosec}^2\left(\frac{\pi}{4}-\frac{x}{2}\right)\)
3. \(\frac{1}{2}{cosec}\left(\frac{\pi}{4}-\frac{x}{2}\right) \cot \left(\frac{\pi}{4}-\frac{x}{2}\right)\)
4. None of these

Answer: 2. \(\frac{1}{2}{cosec}^2\left(\frac{\pi}{4}-\frac{x}{2}\right)\)

y = \(\left\{\frac{1+\cos \left(\frac{\pi}{2}-x\right)}{1-\cos \left(\frac{\pi}{2}-x\right)}\right\}^{\frac{1}{2}}=\left\{\frac{2 \cos ^2\left(\frac{\pi}{4}-\frac{x}{2}\right)}{2 \sin ^2\left(\frac{\pi}{4}-\frac{x}{2}\right)}\right\}^{\frac{1}{2}}=\)

= \(\cot \left(\frac{\pi}{4}-\frac{x}{2}\right)\)

Question 23. If y= \(\sqrt{\frac{\sec x-1}{\sec x+1}}\) then \(\frac{d y}{d x}\) = ?

1. sec²x
2. \(\frac{1}{2} \sec ^2 \frac{x}{2}\)
3. \(\frac{-1}{2}{cosec}^2 \frac{x}{2}\)
4. None of these

Answer: 2. \(\frac{1}{2} \sec ^2 \frac{x}{2}\)

y = \(\left(\frac{1-\cos x}{1+\cos x}\right)^{\frac{1}{2}}=\left\{\frac{2 \sin ^2(x / 2)}{2 \cos ^2(x / 2)}\right\}^{\frac{1}{2}}\)

= \(\tan \frac{x}{2}\)

Question 24. If y = \(=\sqrt{\frac{1+\tan x}{1-\tan x}}\), then \(\frac{d y}{d x}\) = ?

1. \(\frac{1}{2} \sec ^2 x \cdot \tan \left(x+\frac{\pi}{4}\right)\)
2. \(\frac{\sec ^2\left(x+\frac{\pi}{4}\right)}{2 \sqrt{\tan \left(x+\frac{\pi}{4}\right)}}\)
3. \(\frac{\sec ^2\left(\frac{x}{4}\right)}{\sqrt{\tan \left(x+\frac{\pi}{4}\right)}}\)
4. None of these

Answer: 2. \(\frac{\sec ^2\left(x+\frac{\pi}{4}\right)}{2 \sqrt{\tan \left(x+\frac{\pi}{4}\right)}}\)

y= \(\left\{\tan \left(x+\frac{\pi}{4}\right)\right\}^{\frac{1}{2}}\)

= \(\frac{d y}{d x}=\frac{1}{2}\left\{\tan \left(x+\frac{\pi}{4}\right)\right\}^{-\frac{1}{2}} \cdot \sec ^2\left(x+\frac{\pi}{4}\right)\)

Question 25. If y = \(\tan ^{-1}\left(\frac{1-\cos x}{\sin x}\right)\), then \(\frac{d y}{d x}\) = ?

1. 1
2. -1
3. \(\frac{1}{2}\)
4. \(\frac{-1}{2}\)

Answer: 3. \(\frac{1}{2}\)

y= \(\tan ^{-1}\left(\frac{1-\cos x}{\sin x}\right)=\tan ^{-1}\left\{\frac{2 \sin ^2(x / 2)}{2 \sin (x / 2) \cos (x / 2)}\right\}\)

= \(\tan ^{-1}\left\{\tan \frac{x}{2}\right\}\)

=\(\frac{x}{2}\)

Question 26. If y= \(\tan ^{-1}\left\{\frac{\cos x+\sin x}{\cos x-\sin x}\right\}\) , then \(\frac{d y}{d x}\) = ?

1. 1
2. -1
3. \(\frac{1}{2}\)
4. \(\frac{-1}{2}\)

Answer: 1. 1

y = \(\tan ^{-1}\left\{\frac{\cos x+\sin x}{\cos x-\sin x}\right\}\)

= \(\tan ^{-1}\left\{\frac{1+\tan x}{1-\tan x}\right\}\)

= \(\tan ^{-1}\left\{\tan \left(\frac{\pi}{4}+x\right)\right\}\)

= \(\left(\frac{\pi}{4}+x\right)\)

Question 27. If y= \(\tan ^{-1}\left\{\frac{\cos x+\sin x}{\cos x-\sin x}\right\}\) ,then \(\frac{d y}{d x}\) = ?

1. \(\frac{1}{2}\)
2. \(\frac{-1}{2}\)
3. 1
4. -1

Answer: 2. \(\frac{-1}{2}\)

y \(=\tan ^{-1}\left\{\frac{\sin \left(\frac{\pi}{2}-x\right)}{1+\cos \left(\frac{\pi}{2}-x\right)}\right\}\)

= \(\tan ^{-1}\left\{\tan \left(\frac{\pi}{4}-\frac{x}{2}\right)\right\}\)

= \(\left(\frac{\pi}{4}-\frac{x}{2}\right)\)

Question 28. If y = \(\tan ^{-1} \sqrt{\frac{1-\cos x}{1+\cos x}}\) ,then \(\frac{d y}{d x}\) = ?

1. \(\frac{1}{2}\)
2. \(\frac{-1}{2}\)
3. \(\frac{1}{\left(1+x^2\right)}\)
4. None of these

Answer:  2. \(\frac{-1}{2}\)

y = \(\tan ^{-1}\left\{\frac{2 \sin ^2(x / 2)}{2 \cos ^2(x / 2)}\right\}^{\frac{1}{2}}\)

⇒ \(\tan ^{-1}\left\{\tan \frac{x}{2}\right\}\)

= \(\frac{x}{2}\)

Question 29. If y= \(\tan ^{-1}\left(\frac{a \cos x-b \sin x}{b \cos x+a \sin x}\right)\) , then \(\frac{d y}{d x}\) = ?

1. \(\frac{a}{b}\)
2. \(\frac{-b}{a}\)
3. 1
4. -1

Answer: 4. -1

\(=\tan ^{-1}\left(\frac{\frac{a}{b}-\tan x}{1+\frac{a}{b} \tan x}\right)\)

= \(\tan ^{-1}\left(\frac{\tan \theta-\tan x}{1+\tan \theta \tan x}\right)\)

= \(\tan ^{-1} \tan (\theta-x)=\theta-x=\left(\tan ^{-1} \frac{a}{b}-x\right)\)

= \(\frac{d y}{d x}\)= 1

Question 30. If y= sin^-1(3x- 4X³), then \(\frac{d y}{d x}\) = ?

1. \(\frac{3}{\sqrt{1-x^2}}\)
2. \(\frac{-4}{\sqrt{1-x^2}}\)
3. \(\frac{3}{\sqrt{1+x^2}}\)
4. None of these

Answer: 1. \(\frac{3}{\sqrt{1-x^2}}\)

Putting x= sin θ, we get y= sin 1(sin 3θ) = 3θ = 3sin 1x.

Question 31. If y= cos^-1(4X³– 3x), then \(\frac{d y}{d x}\) = ?

1. \(\frac{3}{\sqrt{1-x^2}}\)
2. \(\frac{-3}{\sqrt{1-x^2}}\)
3. \(\frac{4}{\sqrt{1-x^2}}\)
4. \(\frac{-4}{\left(3 x^2-1\right)}\)

Answer: 2. \(\frac{3}{\sqrt{1-x^2}}\)

Putting x= cos θ, we get y= cos^-1(cos 3θ) = 3θ= 3cos^-1x.

Question 32. If y = \(\tan ^{-1}\left(\frac{\sqrt{ } a+\sqrt{ } x}{1-\sqrt{a x}}\right)\), then \(\frac{d y}{d x}\) = ?

1. \(\frac{1}{(1+x)}\)
2. \(\frac{1}{\sqrt{x}(1+x)}\)
3. \(\frac{2}{\sqrt{x}(1+x)}\)
4. \(\frac{1}{2 \sqrt{x}(1+x)}\)

Answer: 4. \(\frac{1}{2 \sqrt{x}(1+x)}\)

Put \(\sqrt{ } a\)= tan θ and \(\sqrt{ } x\)= tan Φ.

Then

y= tan¹ {tan (θ+ Φ)>)} = θ+ Φ = tan^-1 \(\sqrt{ } a\) + tan^-1 \(\sqrt{ } x\).

Question 33. If y = \(\cos ^{-1}\left(\frac{x^2-1}{x^2+1}\right)\), then \(\frac{d y}{d x}\) = ?

1. \(\frac{2}{\left(1+x^2\right)}\)
2. \(\frac{-2}{\left(1+x^2\right)}\)
3. \(\frac{2x}{\left(1+x^2\right)}\)
4. None of these

Answer: 2. \(\frac{-2}{\left(1+x^2\right)}\)

Putting x= cot θ we get:

y =\(\cos ^{-1}\left(\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta}\right)=\cos ^{-1}(\cos 2 \theta)\)

= 2θ= 2 cot^-1x

Question 34. If y = tan-1\(\frac{2 x}{\left(1+x^4\right)}\), then \(\frac{d y}{d x}\) = ?

1. \(\frac{2 x}{\left(1+x^4\right)}\)
2. \(\frac{-2 x}{\left(1+x^4\right)}\)
3. \(\frac{x}{\left(1+x^4\right)}\)
4. None of these

Answer: 1. \(\frac{2 x}{\left(1+x^4\right)}\)

Putting x²= tan θ, we get:

y= \(y=\tan ^{-1}\left\{\tan \left(\frac{\pi}{4}+\theta\right)\right\}=\left(\frac{\pi}{4}+\theta\right)\)

= \(\left(\frac{\pi}{4}+\tan ^{-1} x^2\right)\)

Question 35. If y = cos^-1 x³, then\(\frac{d y}{d x}\) = ?

1. \(\frac{-1}{(1+x)}\)
2. \(\frac{2}{\sqrt{(1+x)}}\)
3. \(\frac{-1}{2 \sqrt{ } x(1+x)}\)
4. None of these

Answer: 3. \(\frac{-1}{2 \sqrt{ } x(1+x)}\)

y = \(\cot ^{-1} \sqrt{x}\)

⇒ \(\frac{d y}{d x}=\frac{-1}{(1+x)} \cdot \frac{1}{2 \sqrt{ } x}=\frac{-1}{2 \sqrt{ } x(1+x)}\)

Question 36. If y = cos^-1x³, then\(\frac{d y}{d x}\) = ?

1. \(\frac{-1}{\sqrt{1-x^6}}\)
2. \(\frac{-3 x^2}{\sqrt{1-x^6}}\)
3. \(\frac{-3}{x^2 \sqrt{1-x^6}}\)
4. None of these

Answer: 2. \(\frac{-3 x^2}{\sqrt{1-x^6}}\)

y = \(\cos ^{-1} x^3\)

⇒ \(\frac{d y}{d x}=\frac{-3 x^2}{\sqrt{1-x^6}}\)

Question 37. If y= tan^-1(sec x + tan x), then \(\frac{d y}{d x}\) = ?

1. \(\frac{1}{2}\)
2. \(\frac{1}{2}\)
3. 1
4. None of these

Answer: 1. \(\frac{1}{2}\)

y= \(\tan ^{-1}\left(\frac{1+\sin x}{\cos x}\right)=\tan ^{-1}\left[\frac{\{\cos (x / 2)+\sin (x / 2)\}^2}{\cos ^2(x / 2)-\sin ^2(x / 2)}\right]\)

= \(\tan ^{-1}\left\{\frac{\cos (x / 2)+\sin (x / 2)}{\cos (x / 2)-\sin (x / 2)}\right\}\)

= \(\tan ^{-1}\left\{\frac{1+\tan (x / 2)}{1-\tan (x / 2)}\right\}\)

= \(\tan ^{-1}\left\{\tan \left(\frac{\pi}{4}+\frac{x}{2}\right)\right\}\)

= \(\left(\frac{\pi}{4}+\frac{x}{2}\right)\)

Question 38. If y \(\cot ^{-1}\left(\frac{1-x}{1+x}\right)\), then \(\frac{d y}{d x}\)

1. \(\frac{-1}{\left(1+x^2\right)}\)
2. \(\frac{1}{\left(1+x^2\right)}\)
3. \(\frac{1}{\left(1+x^2\right)^{3 / 2}}\)
4. None of these

Answer: 2. \(\frac{1}{\left(1+x^2\right)}\)

Put x= tan θ. Then, y= cot^-1.tan\(\left(\frac{\pi}{4}-\theta\right)\)

= \(\cot ^{-1}\left[\cot \left\{\frac{\pi}{2}-\left(\frac{\pi}{4}-\theta\right)\right\}\right]\)

= \(\left(\frac{\pi}{4}+\theta\right)\)

y = \(\frac{\pi}{4}+\tan ^{-1} x\)

Question 39. If y= \(y\sqrt{\frac{1+x}{1-x}}\), then \(\frac{d y}{d x}\)

1. \(\frac{2}{(1-x)^2}\)
2. \(\frac{x}{(1-x)^{3 / 2}}\)
3. \(\frac{1}{(1-x)^{3 / 2} \cdot(1+x)^{1 / 2}}\)
4. None of these

Answer: 3. \(\frac{1}{(1-x)^{3 / 2} \cdot(1+x)^{1 / 2}}\)

log y = \(\frac{1}{2}\{\log (1+x)-\log (1-x)\}\)

⇒ \(\frac{1}{y} \cdot \frac{d y}{d x}\)

= \(\frac{1}{2}\left\{\frac{1}{(1+x)}+\frac{1}{(1-x)}\right\}\)

Question 40. If y = \(\sec ^{-1}\left(\frac{x^2+1}{x^2-1}\right)\) , then \(\frac{d y}{d x}\)= ?

1. \(\frac{-2}{\left(1+x^2\right)}\)
2. \(\frac{2}{\left(1+x^2\right)}\)
3. \(\frac{-1}{\left(1-x^2\right)}\)
4. None of these

Answer: 1. \(\frac{-2}{\left(1+x^2\right)}\)

Put x= cot θ. Then

y = \(\sec ^{-1}\left(\frac{1+\tan ^2 \theta}{1-\tan ^2 \theta}\right)\)

= \(\sec ^{-1}\left(\frac{1}{\cos 2 \theta}\right)=\sec ^{-1}(\sec 2 \theta)\)

= 2θ = 2 cot x^-1

Question 41. If y = \(\sec ^{-1}\left(\frac{1}{2 x^2-1}\right)\),then \(\frac{d y}{d x}\)= ?

1. \(\frac{-2}{\left(1+x^2\right)}\)
2. \(\frac{-2}{\left(1-x^2\right)}\)
3. \(\frac{-2}{\sqrt{1-x^2}}\)
4. None of these

Answer: 3. \(\frac{-2}{\sqrt{1-x^2}}\)

Put x= cos θ.

Then , y= \(\sec ^{-1}\left(\frac{1}{2 \cos ^2 \theta-1}\right)\)

= sec(sec 2θ) = 2 θ = 2 cos^-1 x

Question 42. If y = \(\tan ^{-1}\left\{\frac{\sqrt{1+x^2}-1}{x}\right\}\), then \(\frac{d y}{d x}\)= ?

1. \(\frac{1}{\left(1+x^2\right)}\)
2. \(\frac{2}{\left(1+x^2\right)}\)
3. \(\frac{1}{2\left(1+x^2\right)}\)
4. None of these

Answer: 3. \(\frac{1}{2\left(1+x^2\right)}\)

Put x = tan θ.

Then, y =\(\frac{1}{2}\) tan^-1 x

Question 43. If \(y=\sin ^{-1}\left\{\frac{\sqrt{1+x}+\sqrt{1-x}}{2}\right\}\) , then \(\frac{d y}{d x}\) = ?

1. \(\frac{-1}{2 \sqrt{1-x^2}}\)
2. \(\frac{1}{2 \sqrt{1-x^2}}\)
3. \(\frac{1}{2\left(1+x^2\right)}\)
4. None of these

Answer: 1. \(\frac{-1}{2 \sqrt{1-x^2}}\)

Put x = cos θ. Then,

y= \(\frac{\pi}{4}+\frac{1}{2} \cos ^{-1} x\)

Question 44. If x= at², y= 2at, then \(\frac{d y}{d x}\) = ?

1. \(\frac{1}{t}\)
2. \(\frac{-1}{t^2}\)
3. \(\frac{-2}{t}\)
4. None of these

Answer: 1. \(\frac{1}{t}\)

⇒ \(\frac{d x}{d t}=2 a t\)

⇒  \(\frac{d y}{d t}=2 a\)

So, \(\frac{d y}{d x}=\frac{(d y / d t)}{(d x / d t)}\)

= \(\frac{1}{t}\)

Question 45. If x= a sec θ, y= b tan θ, then \(\frac{d y}{d x}\) =?

1. \(\frac{b}{a} \sec \theta\)
2. \(\frac{b}{a}{cosec} \theta\)
3. \(\frac{b}{a} \cot \theta\)
4. None of these

Answer: 2. \(\frac{b}{a}{cosec} \theta\)

⇒ \(\frac{d x}{d \theta}=a \sec \theta \tan \theta\)

= \(\frac{d y}{d \theta}=b \sec ^2 \theta\). \(\frac{d y}{d x}=\frac{(d y / d \theta)}{(d x / d \theta)}\)

= \(\frac{b}{a}{cosec} \theta\)

Question 46. If x = a cos 2θ, y= b sin 2θ, then \(\frac{d y}{d x}\) = ?

1. \(\frac{-a}{b}\)
2. \(\frac{a}{b}\) cot θ
3. \(\frac{-b}{a}\)
4. None of these

Answer: 3. \(\frac{-b}{a}\)

⇒ \(\frac{d x}{d \theta}=-2 a \cos \theta \sin \theta\),

=  \(\frac{d y}{d \theta}=2 b \sin \theta \cos \theta\)

= \(\frac{d y}{d x}=\frac{(d y / d \theta)}{(d x / d \theta)}\)

= \(\frac{-b}{a}\)

Question 47. If x= a(cos θ+ θ sin θ) and y = a(sin θ — θ cos θ), then\(\frac{d y}{d x}\) =?

1. cot θ
2. tan θ
3. cot θ
4. a tan θ

Answer:  2. tan θ

⇒ \(\frac{d y}{d x}=\frac{(d y / d \theta)}{(d x / d \theta)}\)

Question 48. If y = \(x^{x^{x \ldots \infty}}\), then \(\frac{d y}{d x}\)= ?

1. \(\frac{y}{x(1-\log x)}\)
2. \(\frac{y^2}{x(1-\log x)}\)
3. \(\frac{y^2}{x(1-y \log x)}\)
4. None of these

Answer: 3. \(\frac{y^2}{x(1-y \log x)}\)

y = \(x^y\)

log y =  y log x

⇒ \(\frac{1}{y} \frac{d y}{d x}\)

⇒ \(\frac{y}{x}+(\log x) \frac{d y}{d x}\)

Question 49. If y = \(\sqrt{x+\sqrt{x+\sqrt{x+}}} \ldots \infty\) , then \(\frac{d y}{d x}\) = ?

1. \(\frac{1}{(2 y-1)}\)
2. \(\frac{1}{\left(y^2-1\right)}\)
3. \(\frac{2 y}{\left(y^2-1\right)}\)
4. None of these

Answer:  1. \(\frac{1}{(2 y-1)}\)

y= \(\sqrt{x+y}\)

y²= \(x+y\)

⇒ \(2 y \frac{d y}{d x}=\cos x+\frac{d y}{d x}\)

Question 50. If y = \(\sqrt{\sin x+\sqrt{\sin x+\sqrt{\sin x+}}} \ldots\), then \(\frac{d y}{d x}\) = ?

1. \(\frac{\sin x}{(2 y-1)}\)
2. \(\frac{\cos x}{(y-1)}\)
3. \(\frac{\cos x}{(2 y-1)}\)
4. None of these

Answer: 3. \(\frac{\cos x}{(2 y-1)}\)

y = \(e^{x+y} \Rightarrow x+y=\log y\)

y = sin x+y

= \(2 y \frac{d y}{d x}\)

= \(\cos x+\frac{d y}{d x}\)

Question 51. If y = \(e^x+e^{x+\ldots}\), then\(\frac{d y}{d x}\)

1. \(\frac{1}{(1-y)}\)
2. \(\frac{y}{(1-y)}\)
3. \(\frac{y}{(y-1)}\)
4. None of these

Answer:  2. \(\frac{y}{(1-y)}\)

y= e^(x+y)

x+y =logy

1+ \(+\frac{d y}{d x}=\frac{1}{y} \cdot \frac{d y}{d x}\)

Question 52.  The value k for which \(f(x)=\left\{\begin{array}{c}
\frac{\sin 5 x}{3 x}, \text { if } x \neq 0 \\
k, \text { if } x=0
\end{array} \text { is continuous at } x=0\right.\) is 

1. \(\frac{1}{3}\)
2. 0
3. \(\frac{3}{5}\)
4. \(\frac{5}{3}\)

Answer: 4. \(\frac{5}{3}\)

For continuity at x= 0, we must have \(\lim _{x \rightarrow 0} f(x)\) = f(0).

⇒ \(\lim _{x \rightarrow 0} f(x)\)

= \(\lim _{x \rightarrow 0} \frac{\sin 5 x}{5 x} \times \frac{5}{3}=\frac{5}{3} \lim _{5 x \rightarrow 0} \frac{\sin 5 x}{5 x}\)

= \(\left(\frac{5}{3} \times 1\right)=\frac{5}{3}\)

∴ We must have,f(0) = \(\frac{5}{3}\)

k = \(\frac{5}{3}\)

Question 53. \(\left\{\begin{array}{l}
x \sin \frac{1}{x}, \text { if } x \neq 0 \\
0, \text { when } x=0 .
\end{array}\right.\) Then, which of the following is the true statement?

1. f(x) is not defined at x= 0
2. \(\lim _{x \rightarrow 0} f(x)\)does not exist
3. f(x) is continuous at x= 0
4. f(x) is discontinuous at x= 0

Answer: 3. f(x) is continuous at x= 0

f(0) = 0.

⇒ \(\lim _{x \rightarrow 0} f(x)\) = \(\lim _{x \rightarrow 0} x \sin \frac{1}{x}=0 \times(\text { a finite quantity })\)

= 0

∴ f(x)is continuous at x= 0

Question 54. The value of k for which f(x) = x is continuous at x= 0, is

1. 7
2. 4
3. 3
4. None of these

Answer: 1.7

f(0)=k.

⇒ \(\lim _{x \rightarrow 0} f(x)\)

= \(=\lim _{x \rightarrow 0} \frac{3 x+4 \tan x}{x}=\)

= \(\lim _{x \rightarrow 0}\left\{3+\frac{4 \tan x}{x}\right\}\)

(3+4) = 7

∴ f(x) is continuous at x= 0 ⇔ f(0) = 7 ⇔ k= 7.

Question 55. Let f(x) =x^3/2 Then, f'(0)= ?

1. \(\frac{3}{2}\)
2. \(\frac{1}{2}\)
3. Does not exist
4. None of these

Answer: 3. Does not exist

\(L f^{\prime}(0)=\lim _{h \rightarrow 0} \frac{f(0-h)-f(0)}{-h}\)

= \(\lim _{h \rightarrow 0} \frac{\left(h^{3 / 2}-0\right)}{-h}=\lim _{h \rightarrow 0}(-h)^{1 / 2}\), which does not exist, since

(-h)^1/2 is imaginary

Question 56. The function f(x) = lx I ∀ x ∈ R is

1. Continuous but not differentiable at x = 0
2. Differentiable but not continuous at x = 0
3. Neither continuous nor differentiable at x = 0
4. None of these

Answer: 1. Continuous but not differentiable at x = 0

f(0+0) = \(\lim _{h \rightarrow 0}|0+h|\)

= \(\lim _{h \rightarrow 0}|h|=0\)

f(0-0)= \(\lim _{h \rightarrow 0}|0-h|\)

= \(\lim _{h \rightarrow 0}|-h|=\lim _{h \rightarrow 0}|h|=0\) and f(0)= 0

∴ f(x) is continuous at x= 0

Rf'(0)= \(\lim _{h \rightarrow 0} \frac{f(0+h)-f(0)}{h}\)

= \(\lim _{h \rightarrow 0} \frac{f(h)-0}{h}=\lim _{h \rightarrow 0} \frac{|h|}{h}\)

= \(\lim _{h \rightarrow 0} \frac{h}{h}=1\)

lf'(0)= \(\lim _{h \rightarrow 0} \frac{f(0-h)-f(0)}{-h}=\lim _{h \rightarrow 0} \frac{f(-h)-0}{-h}\)

= \(\lim _{h \rightarrow 0} \frac{|-h|}{-h}\)

= \(\lim _{h \rightarrow 0} \frac{h}{-h}=-1\)

∴ Rf'(0) ≠ L f'(0), which shows that f(x) is not differentiable at x= 0.

Question 57. \(
\text { The function } f(x)=\left\{\begin{array}{l}
1+x, \text { when } x \leq 2 \\
5-x, \text { when } x>2
\end{array}\right. \text { is }\)

1. Continuous as well as differentiable at x = 2
2. Continuous but not differentiable at x = 2
3. Differentiablebutnot continuous at x = 2
4. None of these

Answer: 2. Continuous but not differentiable at x=2

f(2+ 0) = \(\lim _{h \rightarrow 0} f(2+h)=\lim _{h \rightarrow 0}\{5-(2+h)\}=3\)

f(2 – 0)= \(\lim _{h \rightarrow 0} f(2-h)=\lim _{h \rightarrow 0}\{1+(2-h)\}=3\) and f(2) = 3

∴ f(x) is continuous at x= 2

⇒ Rf'(2) = \(\lim _{h \rightarrow 0} \frac{f(2+h)-f(2)}{h}=\lim _{h \rightarrow 0} \frac{(3-h)-3}{h}\)

= \(\lim _{h \rightarrow 0} \frac{-h}{h}=-1\)

⇒ Lf'(2) = \(\lim _{h \rightarrow 0} \frac{f(2-h)-f(2)}{-h}=\lim _{h \rightarrow 0} \frac{(3-h)-3}{-h}\)

= \(\lim _{h \rightarrow 0} \frac{h}{h}=1\)

∴ f (x) is not differentiable at x= 2

Question 58. \(
\text { If the function } f(x)=\left\{\begin{array}{r}
k x+5, \text { when } x \leq 2 \\
x-1, \text { when } x>2
\end{array}\right. \text { is }\) continuous at x= 2, then k= ?

1. 2
2. -2
3. 3
4. -3

Answer: 2.- 2

f(2) = \(\lim _{x \rightarrow 2} f(x)\)

= \(\lim _{x \rightarrow 2^{+}} f(x)=\lim _{h \rightarrow 0} f(2+h)\)

= \(\lim _{h \rightarrow 0}(2+h-1)=1\)

= \(\lim _{x \rightarrow 2^{-}} f(x)=\lim _{h \rightarrow 0} f(2-h)\)

= \(\lim _{h \rightarrow 0}\{k(2-h)+5\}\)

∴ 2k+5 = 1

k= -2

Also,f(2) = 2k+5 = 1 .Hence, k= -2

Question 59. If the function \(f(x)=\left\{\begin{array}{c}
\frac{1-\cos 4 x}{8 x^2}, x \neq 0 \\
k, x=0
\end{array}\right.\) is continuous at x= 0 , then k= ?

1. 1
2. 2
3. \(\frac{1}{2}\)
4. \(\frac{-1}{2}\)

Answer: 3. \(\frac{1}{2}\)

⇒ \(\lim _{x \rightarrow 0^{+}} f(x)=\lim _{h \rightarrow 0} f(0+h)\)

= \(\lim _{h \rightarrow 0} \frac{1-\cos 4 h}{8 h^2}\)

= \(\lim _{h \rightarrow 0} \frac{2 \sin ^2 2 h}{8 h^2}\)

= \(\frac{1}{2} \lim _{h \rightarrow 0}\left(\frac{\sin 2 h}{2 h}\right)^2\)

= \(\left(\frac{1}{2} \times 1^2\right)=\frac{1}{2}\)

⇒ \(\lim _{x \rightarrow 0} f(x)=\lim _{x \rightarrow 0} \frac{\sin ^2 a x}{a^2 x^2} \times a^2\)

= \(a^2 \cdot \lim _{a x \rightarrow 0}\left(\frac{\sin a x}{a x}\right)^2\)

= \(a^2 \times 1^2=a^2\)

For continuity, we must have f(0) = \(\frac{1}{2}\)

For continuity, we must have f(0) = a²

Question 60. If the function f(x) \(\left\{\begin{array}{r}
\frac{\sin ^2 a x}{x^2}, \text { when } x \neq 0 \\
k, \text { when } x=0
\end{array}\right.\) is continuous at x= 0, then k= ?

1. a
2. a²
3. -2
4. -4

Answer: 2. a²

Question 61. If the function f(x) = \(\left\{\begin{aligned}
\frac{k \cos x}{(\pi-2 x)}, \text { when } x & \neq \frac{\pi}{2} \\
3, \text { when } x & =\frac{\pi}{2}
\end{aligned}\right.\)

1. 3
2. -3
3. -5
4. 6

Answer: 4. 6

⇒ \(\lim _{x \rightarrow 0} f(x)=\lim _{x \rightarrow 0} \frac{\sin ^2 a x}{a^2 x^2} \times a^2\)

= \(a^2 \cdot \lim _{a x \rightarrow 0}\left(\frac{\sin a x}{a x}\right)^2\)

= \(a^2 \times 1^2=a^2\)

For continuity, we must have f(x) = a²

Question 62. At x= 2,f(x) = [x] is

1. Continuous but not differentiable
2. Differentiablebutnot continuous
3. Continuous as well as differentiable
4. None of these

Answer: 4. None of these

f(2) = [2] = 2

f(2 + 0)= \(\lim _{h \rightarrow 0} f(2+h)=\lim _{h \rightarrow 0}[2+h]\)= 2

f(2 – 0)= \(\lim _{h \rightarrow 0} f(2-h)=\lim _{h \rightarrow 0}[2-h]\)= 1

∴ f(x) is not continuous at x= 2

Rf'(2) = \(\lim _{h \rightarrow 0} \frac{f(2+h)-f(2)}{h}=\lim _{h \rightarrow 0} \frac{[2+h]-[2]}{h}\)

\(\lim _{h \rightarrow 0} \frac{(2-2)}{h}\) = 0

Lf'(2)= \(\lim _{h \rightarrow 0} \frac{f(2-h)-f(2)}{-h}=\lim _{h \rightarrow 0} \frac{[2-h]-[2]}{-h}\)

= \(\lim _{h \rightarrow 0} \frac{(1-2)}{-h}=\)

= \(\lim _{h \rightarrow 0} \frac{1}{h}=\infty\)

∴ f(x) is not differentiable at x = 2

Question 63. \(
\text { Let } f(x)=\left\{\begin{array}{r}
\frac{x^2-2 x-3}{x+1^2}, \text { when } x \neq-1 \\
k, \text { when } x=-1
\end{array}\right.\)

1. 4
2. -4
3. -3
4. 2

Answer: 2. -4

⇒ \(\lim _{x \rightarrow-1} f(x)=\lim _{x \rightarrow-1} \frac{(x-3)(x+1)}{(x+1)}\)

= \(\lim _{x \rightarrow-1}(x-3)\) = -4

For continuity, we must have, f(-1) =-4

Question 64. The function f(x) = x³– 6x² + I5x- 12 is

1. Strictly decreasing on R
2. Strictly increasing on R
3. Increasingin (-∞, 2] and decreasing in (2, ∞)
4. None of these

Answer: 2. Strictly increasing on R

f'(x) = 3x² -12x+15 = 3(x² -4x+ 5) = 3[(x-2)² + 1] > 0.

⇒  f'(x) > 0 for all x ∈ R ⇒  f(x) is strictly increasing on R.

Question 65. The function f (x) = 4- 3x + 3X² -x³ is

1. Decreasing on R
2. Increasing on R
3. Strictly decreasing on R
4. Strictly increasing on R

Answer: 1. Decreasing on R

f'(x) =-3 + 6x- 3x²= 3(x²– 2x+ 1) = -3(x- 1)² < 0.

⇒   f'(x) < 0 for all x ∈ R ⇒  f(x) is decreasing on R.

Question 66. The function f(x) = 3x + cos 3x is

1. Increasing on R
2. Strictly increasing on R
3. Decreasing on R
4. Strictly decreasing on R

Answer: 1. Increasing on R

f'(x) = 3- 3sin 3x = 3(1- sin 3x) > 0 since -1 < sin 3x < 1.

f( x) > 0 for all x ∈ R ⇒  f(x) is increasing on R.

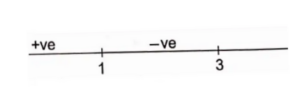
Question 67. The function/ f(x) = x³– 6X²+ 9x+ 3 is decreasing for

1. 1 < x < 3
2. x>1
3. x <1
4. x <1 or x > 3

Answer: 1. 1 < x < 3

f'(x) = 3x²– 12 + 9 = 3Cx²– 4x + 3) = 3(x- 1)(x- 3)

f'(x) = 0 ⇒ x=1 or x= 3.

There are two factors in f'(x), so we start with + ve sign.

∴ f(x) is decreasing for 1 < x < 3.

 

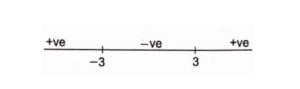
Question 68. The function f(x) -x³– 27x + 8 is increasing when

1. 1 x 1 <3
2. 1 x 1 >3
3. -3 < x < 3
4. None of these

Answer: 2. 1x 1 >3

f'( x) = 3x² -27= 3(x²– 9) = 3(x+ 3)(x- 3)

f'(x)= 0 ⇒ x=-3 or x= 3.

There are two factors in f(x), so we start with +ve sign.

f(x) is increasing when x < -3 or x > 3, i.e., when IxI >3.

 

Question 69. f(x) = sin x is increasing in

1. \(\left(\frac{\pi}{2}, \pi\right)\)
2. \(\left(\pi, \frac{3 \pi}{2}\right)\)
3. [0,π]
4. \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

Answer: 4. \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

∴ f'(x)= cos x > 0 in \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

∴  f(x) is increasing in \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

Question 70. f(x) = \(\frac{2 x}{\log x}\) is increasing in

1. (0,1)
2. (1, e)
3. (e, ∞)
4. (-∞,e)

Answer: 3. (e, ∞)

⇒ \(f^{\prime}(x)=\frac{(\log x) \cdot 2-2 x \cdot \frac{1}{x}}{(\log x)^2}\)

= \(\frac{2(\log x-1)}{(\log x)^2}\)

∴ f'(x)> 0 ⇔ log x-1>0 ⇔ log x>1 ⇔ log x>log e ⇔ x>e.

∴ f(x) is increasing (e, ∞)

Question 71. f(x) = (sin x- cos x) is decreasing

1. \(\left(0, \frac{3 \pi}{4}\right)\)
2. \(\left(\frac{3 \pi}{4}, \frac{7 \pi}{4}\right)\)
3. \(\left(\frac{7 \pi}{4}, 2 \pi\right)\)
4. None of these

Answer: 2. \(\left(\frac{3 \pi}{4}, \frac{7 \pi}{4}\right)\)

f'(x)= (cos x+ sinx) = \(\sqrt{2}\left(\frac{1}{\sqrt{2}} \cos x+\frac{1}{\sqrt{2}} \sin x\right)\)

= \(\sqrt{2} \sin \left(\frac{\pi}{4}+x\right)\)

∴ f(x)’ \(\sin \left(\frac{\pi}{4}+x\right)<0\)

= \(\pi<\frac{\pi}{4}+x<2 \pi\)

= \(\frac{3 \pi}{4}<x<\frac{7 \pi}{4}\)

∴ f (x) is decreasing in \(\left(\frac{3 \pi}{4}, \frac{7 \pi}{4}\right)\)

Question 72. f(x) = \(\frac{x}{\sin x}\) is

1. Increasing in (0, 1)
2. Decreasing in (0, 1)
3. Increasingin \(\left(0, \frac{1}{2}\right)\) and decreasing \(\left(, \frac{1}{2}\right)\)in
4. None of these

Answer: 1. Increasing in (0, 1)

f'(x)= \(\frac{\sin x-x \cos x}{\sin ^2 x}\)

= \(\frac{\cos x(\tan x-x)}{\sin ^2 x}\)

0<x<1 ⇒  tan x >x and cos x >0 ⇒  cos x(tan x- x) > 0

⇒  f'(x)>0

∴ f(x) is increasing (0, 1).

Question 73. f(x) = x^x is decreasing in the interval

1. (0,e)
2. \(\left(0, \frac{1}{e}\right)\)
3. (0,1)
4. None of these

Answer: 2. \(\left(0, \frac{1}{e}\right)\)

f (x) = (1 +log x)

f'(x)< 0 ⇔ (1 +logx)<0 ⇒ logx<-1

= \(\log \frac{1}{e}\)

= x >0 and \(x<\frac{1}{e}\)

f(x) is decreasing in (0, \(\frac{1}{e}\))

Question 74. f(x) = x²e^x is increasing in

1. (-2,0)
2. (0,2)
3. (2,∞)
4. (-∞, ∞)

Answer: 2. (0,2)

f'(x) = 2xe^–x– x²e^–x= xe^–x(2- x)

∴ f'(x) > 0 < t ⇒ > 0 and (2- x) > 0 ⇔ 0< x <2.

∴ f(x) is increasing in (0, 2).

Question 75. f(x) = sin x- kx is decreasing for all x ∈ R, when

1. k<1
2. k≥3
3. k<3
4. k≤3

Answer: 3. k<3

f'(x)- (cos x-k) and therefore,

f(x) is decreasing ⇔ f'(x) <0⇒ cos x- k<0

⇒  cos x<k ⇔ k>cosx ⇒ k>1

Question 76. f(x) = (x + 1)³(x- 3)³ is increasing in

1. (-∞, 1)
2. (-1,3)
3. (3, ∞)
4. (1,∞)

Answer: 4. (1,∞)

f'(x) = (x+1) (x- 3)³

f'(x) = 3(x+ 1)3(x- 3)²+ 3(x+ 1)²(x- 3)³

= 3(x+ 1)²(x- 3)²[(x+1) + (x- 3)] = 3(x+1)²(x- 3)²(x-1)

⇒ f'(x) > 0 when (x- 1) > 0, i.e., when x > 1.

∴ f(x) is increasing in (1, ∞).

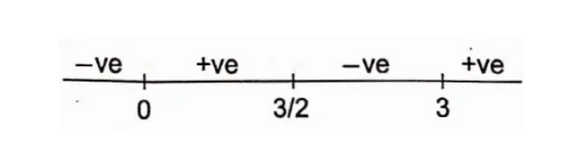
Question 77. f(x) = [x(x-3)]² is increasing in

1. (0,∞)
2. (-,∞,0)
3. (1, 3)
4. (0,\(\frac{1}{3}\)∪(3,∞)

Answer: 4.  (0,\(\frac{1}{3}\))∪(3,∞)

f(x) = [x(x- 3)]² ⇒ f(x)= 2x(x- 3)(2x- 3).

f'(x) = 0 ⇒ x = 0 or x= \(\frac{1}{3}\) or x = \(\frac{1}{3}\) 3.

∴ f (x) is increasing when 0<x <-or x>3.

∴ f(x) is increasing in(0,\(\frac{1}{3}\)).

 

78. If f(x) = kx³– 9x² + 9x + 3 is increasing for every real number x, then

1. k>3
2. k≥3
3. k<3
4. k≤3

Answer: 1. k>3

∴ f(x) = 3kx²– 18x+ 9= 3(kx²– 6x+ 3).

This is positive when k > 0 and (36- 12k) < 0 ⇒ k> 3

Question 79. f(x) = \(\frac{x}{\left(x^2+1\right)}\) is increasing in

1. (-1,1)
2. (-1, ∞)
3. (∞, -1)∪(1,∞)
4. None of these

Answer: 1. (-1,1)

f'(x)= \(\frac{\left(x^2+1\right) \cdot 1-x \cdot 2 x}{\left(x^2+1\right)^2}=\frac{\left(1-x^2\right)}{\left(1+x^2\right)^2}\)

f'(x)> 0 ⇔(1-x²)>0 < ⇒ x²< 1 < ⇒ -1< X <1.

∴ f(x) is increasing in (-1, 1)

Question 80. The least value of k for which f(x) = x² + kx+1 is increasing on (1, 2), is

1. -2
2. -1
4. 1
5. 2

Answer: 1. -2

f'(x)= (2x+ k).

1< x < 2 ⇒ 2< 2x < 4 ⇒ 2+k < 2x + k < 4+ k =  2+k< f(x) <4 + k

f (x) is increasing o (2x +k)>0 ⇔ 2+ k> 0 ⇔ k >-2.

Least value of k is -2.

Question 81. f(x) =IxI has

1. Minimum at x= 0
2. Maximum at x = 0
3. Neither a maximum nor a minimum at x = 0
4. None of these

Answer: 1. Minimum at x= 0

f(x) = IxI > 0 for all x ∈ R.

The least value of I x I is 0 at x = 0.

f(x) = IxI has minima at x= 0

Question 82. When x is positive, the minimum value of x^x is

1. e^e
2. e^1/e
3. e^–1/e
4. (1/e)

Answer: 3. e^–1/e

f(x) = x² ⇒  f'(x) = f(1 +log x) and f”(x) = \(x^x\left[\frac{1}{x}+(1+\log x)^2\right]\)

f'(x) = 0 ⇒ 1 +log x = 0 ⇒ log x =-l \(\log \left(\frac{1}{e}\right) \Rightarrow x=\left(\frac{1}{e}\right)\)

[f”(x)]x= (1/e) = \(e\left(\frac{1}{e}\right)^{1 / e}\)>0.

x= \(\frac{1}{e}\)is apoint ofminima.

Minimum value of x is \(\left(\frac{1}{e}\right)^{1 / e}=e^{-1 / e}\)

Question 83. The maximum value of \(\left(\frac{\log x}{x}\right)\) is

1. \(\left(\frac{1}{e}\right)\)
2. \(\left(\frac{2}{e}\right)\)
3. e
4. 1

Answer: 1. \(\left(\frac{1}{e}\right)\)

f(x) = \(\frac{\log x}{x}\)

f'(x)= \(\frac{x \cdot \frac{1}{x}-\log x \cdot 1}{x^2}=\frac{(1-\log x)}{x^2}\)

f'(x) = \(\frac{x^2 \cdot \frac{1}{x}-(1-\log x) \cdot 2 x}{x^4}=\frac{(-3+2 \log x)}{x^3}\)

f'(x) = 0 ⇒ 1-logx= 0 ⇒ log x=1 = log e ⇒ x= e.

f”(e)= \(\left(\frac{-3+2}{e^3}\right)=\frac{-1}{e^3}\)<0

x= e is a point of maxima

Maximum value of f(x) is \(\frac{1}{e}(\log e)=\frac{1}{e}\)

Question 84. f(x) = cosec x in (-π, 0) has a maxima at

1. x = 0
2. x =\(\frac{-\pi}{4}\)
3. x =\(\frac{-\pi}{3}\)
4. x =\(\frac{-\pi}{2}\)

Answer: 4. x =\(\frac{-\pi}{2}\)

f(x) = cosec x ⇒ f'(x) = -cosec x cot x’

= f”(x) = cosec³x+ cosec x (cot²x) = cosec x (cosec²x+ cot²x)

= cosec x (2cosec²x-1)

f(x) = 0 ⇒ cot x= 0 ⇒ X= \(\frac{-\pi}{2}\)

⇒ \(f^{\prime \prime}\left(\frac{-\pi}{2}\right)=\operatorname{cosec}\left(\frac{-\pi}{2}\right)\left[2 \operatorname{cosec}^2\left(\frac{-\pi}{2}\right)-1\right]\)

= (-1)(2-1)= -1 < 0.

x = \(\frac{-\pi}{2}\)is a point of maxima

Question 85. If x > 0 and xy=1, the minimum value of (x+ y) is

1. -2
2. 1
3. 2
4. None of these

Answer: 3. 2

xy = 1

y = \(\frac{1}{x}\)

Lest S = x+y = x+ \(\frac{1}{x}\)

Then , \(\frac{d s}{d x}=\left(1-\frac{1}{x^2}\right)=\frac{\left(x^2-1\right)}{x^2}\) and \(\frac{d^2 s}{d x^2}=\frac{2}{x^3}\)

\(\frac{d s}{d x}\) = 0

x²-1= 0 x = ± 1

⇒ \(\left.\left.\frac{d^2 s}{d x^2}\right]_{(x=-1)}=-2<0 \text { and } \frac{d^2 s}{d x^2}\right]_{(x=1)}\)

= 2>0

S is minimum at x=1 and minimum value of S = (1 + 1) = 2

Question 86. The minimum value of \(\left(x^2+\frac{250}{x}\right)\)

1. 0
2. 25
3. -39
4. None of these

Answer: 4. None of these

Let f(x) \(\left(x^2+\frac{250}{x}\right)\)

Then f'(x)= \(\left(2 x-\frac{250}{x^2}\right)and\) f”(x} = \(\left(2+\frac{500}{x^3}\right)\)

f'(x) = 0 ⇒ 2x³ -250= 0

x³ = \(\frac{250}{2}\)

x³ = 125

x³ = 5³

⇒ x= 5.

f”(5)= \(\left(2+\frac{500}{125}\right)\)

= 6 > 0

f(x) is minimum at x= 5 and minimum value = \(\left(25+\frac{250}{5}\right)\)= 75

Question 87. The minimum value of f(x) = 3x⁴– 8X³– 48x+ 25 on [0, 3] is

1. 16
2. 25
3. 50
4. 75

Answer: 3. 50

f(x) = 12x³– 24x²+ 24x- 48 = 12(x- 2)(x²+ 2)

f”(x)= 36x²– 48x + 24=12(3x²– 4x+ 2).

f'(x) = 0 ⇒ x= 2 and f”(2) = 12(3x⁴-4x² + 2) = 72>0

x= 2 is a point of minima.

Minimum value=min {f(0),f(2),f(3)}

= min {25, -39, 16} =-39.

Question 88. The maximum value of f(x) = (x- 2)(x- 3)² is

1. \(\frac{7}{3}\)
2. 3
3. \(\frac{4}{27}\)
4. 27

Answer: 3. \(\frac{4}{27}\)

f(x) = (x- 2)(x- 3)² ⇒ f(x)= (x- 3)(3x- 7) and f”(x) = (6x- 16).

f'(x) = 0 ⇒ x= 3 or x= \(\frac{7}{3}\)

f”(3)= 2>0 and f” (\(\frac{7}{3}\))= – 2>0.

x = \(\frac{7}{3}\) is a point of maxima.

Maximum value = (\(\frac{7}{3}\)– 2)(\(\frac{7}{3}\)-3) = \(\frac{4}{27}\)

Question 89. The least value off (x) = (e^x+ e^–x) is

1. -2
2. 0
3. 2
4. None of these

Answer: 3. 0

f'(x) = e^x– e^–x and f”(x) = e^x+ e^–x

f'(x) = 0  ⇒ e^x– e^–x = 0

⇒  e^x= e^–x

⇒ e^2x= e⁰

⇒ x = 0.

f”(0) = e°+ \(\frac{1}{e^0}\)= (1 + 1) = 2 > 0.

f(x) is minimum at x= 0 and minimum value of f(x) is 2

Algebra

Algebra Exercise 1A Review Of Facts And Formulae

1. Results on transposition of matrices

(A +B)’=(A’+B’)

(AB)’= (BA)’

(KB)’= (K.A’)

(A’)’= (A)

2.

A is symmetric ⇔  A’= A

A is skew-symmetric ⇔ A’=-A

Read and Learn More WBCHSE Solutions For Class 12 Maths

3.

A is idempotent ⇔ A²= A

A is nilpotent of order n = Aⁿ= 0

A is orthogonal ⇔ AA’ = A’A =I

A is involutory ⇔ A²=I

4.

A is singular ⇔ I A I = 0

A is non-singular ⇔ I A I # 0

A^-1 exists ⇔ I A I # 0

(AB)^-1 = B^-1 A^-1

(kA)^-11= \(\frac{1}{k}\)A^-1

(A’)^-1 = (A^-1)’

I A^-1I = \(\frac{1}{|A|}\)

5.

A(adj . A) = I AI I

I adj. A I = I AI^n-1

adj . AB = (adj . B) . (adj – A)

6. For square matrices A and B of the same order, we have

1. (A + B)²= A²+AB +BA + B²
2. (A- B)² = A²– AB- BA + B²
3. (A + B) . (A- B) = A²– AB +BA- B²
4. A and B anticommute AB= -BA

Class 12 Algebra Multiple Choice Questions

Question 1.  If A and B are 2 – 2-rowed square matrices such that   

Answer: 2. \(\left[\begin{array}{rr}
7 & -5 \\
1 & -5
\end{array}\right]\)

⇒ 2A= (A + B) + (A- B) and 2B = (A + B)- (A- B)

Question  2. \(\text { If }\left[\begin{array}{rr}
3 & -2 \\
5 & 6
\end{array}\right]+2 A=\left[\begin{array}{rr}
5 & 6 \\
-7 & 10
\end{array}\right], \text { then } A=?\)

1. \(\left[\begin{array}{rr}
1 & 3 \\
-5 & 4
\end{array}\right]\)

2. \(\left[\begin{array}{rr}
-1 & 5 \\
-3 & 4
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
1 & 4 \\
-6 & 2
\end{array}\right]\)

4. None of these

Answer: 3. \(\left[\begin{array}{rr}
1 & 4 \\
-6 & 2
\end{array}\right]\)

⇒ 2A= \(\left[\begin{array}{rr}
5 & 6 \\
-7 & 10
\end{array}\right]\)– \(\left[\begin{array}{rr}
3 & -2 \\
5 & 6
\end{array}\right]\)= \(\left[\begin{array}{rr}
2 & 8 \\
-12 & 4
\end{array}\right]\)

Question 3. \(A=\left[\begin{array}{rr}
2 & 0 \\
-3 & 1
\end{array}\right] \text { and } B=\left[\begin{array}{rr}
4 & -3 \\
-6 & 2
\end{array}\right] \text { are such that } 4 A+3 X=5 B \text {, then } X=\text { ? }\)

1. \(\left[\begin{array}{rr}
4 & -5\\
-6 & 2
\end{array}\right]\)

2. \(\left[\begin{array}{rr}
4 & 5 \\
-6 & -2
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
-4 & 5 \\
-6 & -2
\end{array}\right]\)

4. None of these

Answer: 1. \(\left[\begin{array}{rr}
4 & -5\\
-6 & 2
\end{array}\right]\)

⇒ 4A + 3X= 5B => 3X= (5B-4A) => X = \(\frac{1}{3}\)(5B-4A).

Question 4. \(\text { If }(A-2 B)=\left[\begin{array}{rr}
1 & -2 \\
3 & 0
\end{array}\right] \text { and }(2 A-3 B)=\left[\begin{array}{rr}
-2 & 2 \\
3 & -3
\end{array}\right] \text {, then } B=\text { ? }\)

1. \(\left[\begin{array}{rr}
6 & -4 \\
-3 & 3
\end{array}\right]\)

2. \(\left[\begin{array}{rr}
-4 & 6 \\
-3 & -3
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
4 & -6 \\
-3 & -3
\end{array}\right]\)

4. None of these

Answer: 2. \(\left[\begin{array}{rr}
-4 & 6 \\
-3 & -3
\end{array}\right]\)

⇒ B = (2A-3B)-2(A-2B).

Question 5. \(\text { If }(2 A-B)=\left[\begin{array}{rrr}
6 & -6 & 0 \\
-4 & 2 & 1
\end{array}\right] \text { and }(2 B+A)=\left[\begin{array}{rrr}
3 & 2 & 5 \\
-2 & 1 & -7
\end{array}\right] \text {, then } A=\text { ? }\)

1. \(\left[\begin{array}{rrr}
-3 & 2 & 1 \\
2 & 1 & -1
\end{array}\right]\)

2. \(\left[\begin{array}{rrr}
3 & 2 & -1 \\
2 & -1 & -1
\end{array}\right]\)

3. \(\left[\begin{array}{rrr}
3 & -2 & 1 \\
-2 & 1 & -1
\end{array}\right]\)

4. None of these

Answer: 3. \(\left[\begin{array}{rrr}
3 & -2 & 1 \\
-2 & 1 & -1
\end{array}\right]\)

⇒ 5A = 2(2A- B) + (2B + A). Then, A = \(\frac{1}{5}\)(5A)

Question 6. \(\text { If } 2\left[\begin{array}{ll}
3 & 4 \\
5 & x
\end{array}\right]+\left[\begin{array}{ll}
1 & y \\
0 & 1
\end{array}\right]=\left[\begin{array}{rr}
7 & 0 \\
10 & 5
\end{array}\right] \text {, then }\)

1. (x = -2,y = 8)

2. x =  2y = 8

3. x = 3,y = -6

4. x =-3,y = 6

Answer: 2. x =  2y = 8

⇒ [2x+1 = 5 ⇒ x= 2] and [8 + y= 0 => y= -8].

Question 7. \(\text { If }\left[\begin{array}{cc}
x-y & 2 x-y \\
2 x+z & 3 z+w
\end{array}\right]=\left[\begin{array}{rr}
-1 & 0 \\
5 & 13
\end{array}\right] \text {, then }\)

1. z = 3,w = 4

2. z = 4,w = 3

3. z =1,w = 2

2. z = 2,w =-1

Answer: 1. z = 3,w = 4

⇒ (x- y=-1 and 2x- y= 0) => (x=1, y= 2)

⇒  (2x+ z = 5 => z = 3) and (3z+ w= 13 => w= 4).

Question 8. \(\text { If }\left[\begin{array}{cc}
x & y \\
3 y & x
\end{array}\right]\left[\begin{array}{l}
1 \\
2
\end{array}\right]=\left[\begin{array}{l}
3 \\
5
\end{array}\right] \text {, then }\)

1. x = 1,y = 2

2. x = 2,y = 1

3. x =1,y = 1

4. None of these

Answer: 3. x =1,y = 1

⇒  Solve x+ 2y= 3 and 3y+ 2x = 5.

Question 9. \(\text { If the matrix } A=\left[\begin{array}{cc}
3-2 x & x+1 \\
2 & 4
\end{array}\right] \text { is singular, then } x=\text { ? }\)

1. 0

2. 1

3. -1

4. -2

Answer:  2. 1

⇒  A is singular ⇔ IAI = 0.

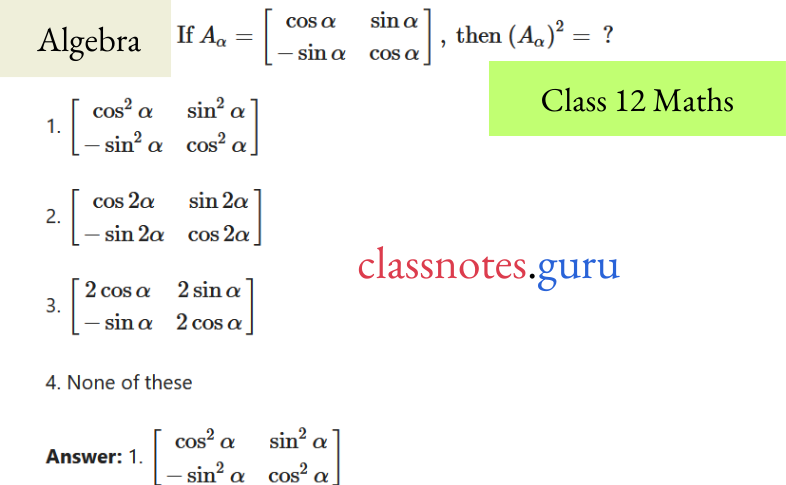
Question 10. \(\text { If } A_\alpha=\left[\begin{array}{cc}
\cos \alpha & \sin \alpha \\
-\sin \alpha & \cos \alpha
\end{array}\right], \text { then }\left(A_\alpha\right)^2=\text { ? }\)

1. \(\left[\begin{array}{cc}
\cos ^2 \alpha & \sin ^2 \alpha \\
-\sin ^2 \alpha & \cos ^2 \alpha
\end{array}\right]\)

2. \(\left[\begin{array}{cc}
\cos 2 \alpha & \sin 2 \alpha \\
-\sin 2 \alpha & \cos 2 \alpha
\end{array}\right]\)

3. \(\left[\begin{array}{cc}
2 \cos \alpha & 2 \sin \alpha \\
-\sin \alpha & 2 \cos \alpha
\end{array}\right]\)

4. None of these

Answer: 1. \(\left[\begin{array}{cc}
\cos ^2 \alpha & \sin ^2 \alpha \\
-\sin ^2 \alpha & \cos ^2 \alpha
\end{array}\right]\)

Question 11. \(\text { If } A=\left[\begin{array}{cc}
\cos \alpha & \sin \alpha \\
-\sin \alpha & \cos \alpha
\end{array}\right] \text { be such that } A+A^{\prime}=I \text {, then } \alpha=\text { ? }\)

1. π

2. \(\frac{\pi}{3}\)

3. 2π

4. \(\frac{2 \pi}{3}\)

Answer: 2. \(\frac{\pi}{3}\)

Question 12. \(\text { If } A=\left[\begin{array}{rrr}
1 & k & 3 \\
3 & k & -2 \\
2 & 3 & -4
\end{array}\right] \text { is singular, then } k=\text { ? }\)

1. \(\frac{16}{3}\)

2. \(\frac{34}{5}\)

3. \(\frac{33}{2}\)

4. None of these

Answer: 3. \(\frac{33}{2}\)

⇒  A is singular ⇔ IAI = 0.

Question 13. \(\text { If } A=\left[\begin{array}{ll}
a & b \\
c & d
\end{array}\right]\), then adj. A= ?

1. \(\left[\begin{array}{ll}
d & -c \\
-b & a
\end{array}\right]\)

2. \(\left[\begin{array}{ll}
-d & b \\
c & -a
\end{array}\right]\)

3. \(\left[\begin{array}{ll}
d & -b \\
-c & a
\end{array}\right]\)

4. \(\left[\begin{array}{ll}
-d & -b \\
c & a
\end{array}\right]\)

Answer: 3. \(\left[\begin{array}{ll}
d & -b \\
-c & a
\end{array}\right]\)

Question 14. \(\text { If } A=\left[\begin{array}{cc}
2 x & 0 \\
x & x
\end{array}\right] \text { and } A^{-1}=\left[\begin{array}{rr}
1 & 0 \\
-1 & 2
\end{array}\right], \text { then } x=?\)

1. 1

2. 2

3. \(\frac{1}{2}\)

4. -2

Answer: 3. \(\frac{1}{2}\)

Use AA^-1 =I.

Question 15. If A and B are square matrices of the same order, then (A + B)(A — B) = ?

1. (A²– B²)

2. A² + AB + BA + B²

3. A²-AB + BA-B²

4. None of these

Answer: 3. A²-AB + BA-B²

⇒ Using distributive law, we have

(A +B)-(A-B) = A(A-B) +B(A-B) = (A²-AB +BA-B²).

Question 16. If A and B are square matrices of the same order, then (A + B)²=?

1. A²+ 2AB + B²

2.  A²-AB-BA + B²

3. A² + 2BA + B²

4. None of these

Answer: 2.  A²-AB-BA + B²

⇒ (A + B)²= (A + B)-(A + B)=A(A + B) + B(A + B) = (A²+ AB + BA + B²).

Question 17. If A and B are square matrices of the same order, then (A- B)² = ?

1. A²– 2AB + B²

2. A²+ AB-BA-B²

3. A²– 2BA + B²

4. None of these

Answer: 2. A²+ AB-BA-B²

⇒ (A- B)² = (A-B) . (A-B)= A(A- B)- B(A- B) = (A2- AB- BA + B2).

Question 18. If A and B are symmetric matrices of the same order, then (AB-BA) is
always

1. A symmetric matrix

2. A skew-symmetric matrix

3. A zero matrix

4. An identity matrix

Answer: 2. A skew-symmetric matrix

Given A’= A and B’ = B.

∴ (AB- BA)’= (AB)’- (BA)’= (B’A’- A’B’) = (BA- AB) = -(AB- BA)

∴ (AB- BA) is skew-symmetric.

Question 19. Matrices A and B are inverses of each other only when

1. AB = BA

2. AB = BA = O

3. AB = O,BA =I

4. AB = BA = I

Answer: 4. AB = BA = I

A and B are inverses of each other only when AB = BA =I.

Question 20. For square matrices A and B of the same order, we have adj (AB) = ?

1. (adj A)(adj B)

2.  (adj A)(adj B)

3. I ABI

4. None of these

Answer: 2.  (adj A)(adj B)

⇒ adj (AB)= (adj B) (adj A).

Question 21. If A is a 3-rowed square matrix and I A I =4, then adj (adj A) =?

1. 4A

2. 16A

3. 64A

4. None of these

Answer: 1. 4A

adj(adj A) = IAI^(n-1)=  IAI^(3-2).A= IAI -A = 4A.

Question 22. If A is a 3-rowed square matrix and I A I =5, then I adj AI =?

1. 5

2. 25

3. 125

4. None of these

Answer: 2. 25

I adj AI = IA^(n-1)I= I A 1²= 5²= 25.

Question 23. For any two matrices A and B

1. AB = BA is always true

2. AB = BA is never true

3. Sometimes AB = BA and sometimes AB BA

4. Whenever AB exists, then BA exists

Answer: 3. Sometimes AB = BA and sometimes AB BA

Question 24.  \(\text { If } A \cdot\left[\begin{array}{rr}
3 & 2 \\
1 & -1
\end{array}\right]=\left[\begin{array}{ll}
4 & 1 \\
2 & 3
\end{array}\right] \text {, then } A=\text { ? }\)

1. \(\left[\begin{array}{rr}
1 & -1 \\
1 & 1
\end{array}\right]\)

2. \(\left[\begin{array}{rr}
1 & 1 \\
-1 & 1
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
1 & 1 \\
1 & -1
\end{array}\right]\)

4. None of these

Answer: 3. \(\left[\begin{array}{rr}
1 & 1 \\
1 & -1
\end{array}\right]\)

⇒ Let \(\left[\begin{array}{ll}
a & b \\
c & d
\end{array}\right]\left[\begin{array}{rr}
3 & 2 \\
1 & -1
\end{array}\right]=\left[\begin{array}{ll}
4 & 1 \\
2 & 3
\end{array}\right]\), find a,b,c and d

Question 25. If A is an invertible square matrix, then 1A^-1I =?

1. |A|

2. \(\frac{1}{|A|}\)

3. 1

4. 0

Answer: 2. \(\frac{1}{|A|}\)

AA^-1 = ⇒  IAA^-1 I = III =1

⇒  IAI. I A^-1 I  =1 = \(\frac{1}{|A|}\)

Question 26. If A and B are invertible matrices of the same order, then (AB)^-1 =?

1. (A^-1 x B^-1)

2. (A x B^-1)

3. (A^-1x B)

4. (B^-1x A^-1)

Answer: 4. (B^-1x A^-1)

⇒  (AB)^-1= B^-1A^-1

Question 27. If A and B are two non-zero square matrices of the same order such that AB = 0, then

1.  IAI =0 or IBI =0

2. IAI = 0 and IBI =0

3.  IAI ≠ 0and IB I ≠ 0

4. None of these

Answer: 2. IAI = 0 and IBI =0

⇒ [AB= 0 and A ≠ 0, B≠ 0] => IAI =0 and IBI =0.

Question 28. If A is a square matrix such that IAI ≠ 0 and A²– A + 2I = 0, then A^-1 =?

1. (I-A)

2. (I+A)

3. \(\frac{1}{2}\)(I-A)

4. \(\frac{1}{2}\)(I+A)

Answer:  3. \(\frac{1}{2}\)(I-A)

⇒ 21=(A- A² ) => 2A^-1 = A^-1 A-A^-1AA = I-IA = (I-A)

A^-1 = \(\frac{1}{2}\) (I-A)

Question 29.  \(
\text { If } A=\left[\begin{array}{lll}
1 & \lambda & 2 \\
1 & 2 & 5 \\
2 & 1 & 1
\end{array}\right] \text { is not invertible, then } \lambda=\text { ? }\)

1. 2

2. 1

3. -1

4. 0

Answer: 2. 1

⇒ A is not invertible ⇒ I A I = 0.

Question 30. \(\text { If } A=\left[\begin{array}{rr}
\cos \theta & -\sin \theta \\
\sin \theta & \cos \theta
\end{array}\right] \text {, then } A^{-1}=\text { ? }\)

1. A

2. -A

3. adj A-

4. -adj A

Answer: 3. adj A-

IAI =1 ⇒ A^-1 =\(
\frac{1}{|A|}
\) adj A= (adj A).

Question 31. \(
\text { The matrix } A=\left[\begin{array}{cc}
a b & b^2 \\
-a^2 & -a b
\end{array}\right] \text { is }\)

1. Idempotent

2. Orthogonal

3. Nilpotent

4. None of these

Answer:  3. Nilpotent

A²= 0  ⇒ A is nilpotent.

Question 32. \(
\text { The matrix } A=\left[\begin{array}{rrr}
2 & -2 & -4 \\
-1 & 3 & 4 \\
1 & -2 & -3
\end{array}\right] \text { is }\)

1. Non-singular

2. Idempotent

3. Nilpotent

4. Orthogonal

Answer: 2. Idempotent

A²= A ⇒ A is idempotent.

Question 33. If A is singular, then A (adj A) =?

1. A unit matrix

2. A null matrix

3. A symmetric matrix

4. None of these

Answer: 2. A null matrix

Given IAI = 0. So, A(adj A) = IAI.I = 0-I = 0.

∴ A(adj A) is a null matrix.

Question 34. \(
\text { For any 2-rowed square matrix } A, \text { if } A \cdot({adj} A)=\left[\begin{array}{ll}
8 & 0 \\
0 & 8
\end{array}\right] \text {, then the value of }\)

1. 0

2. 8

3. 64

4. 4

Answer: 2. 8

A . adj  A = IAI. I= 8 \(\left[\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right]\) = 8I= |A|

Question 35. \(\text { If } A=\left[\begin{array}{rr}
-2 & 3 \\
1 & 1
\end{array}\right] \text {, then }\left|A^{-1}\right|=\text { ? }\)

1. -5

2. \(\frac{-1}{5}\)

3. \(\frac{1}{25}\)

4. 25

Answer: 2. \(\frac{-1}{5}\)

AA^-1  = I ⇒  I AA^-1 I = 1I1 => IAI .I A^-1 I =1 ⇒ IA^-1 I = \(\frac{1}{|A|}\)

IAI = \(\left|\begin{array}{rr}
-2 & 3 \\
1 & 1
\end{array}\right|\)=(-2- 3) = (-5)⇒I A^-1I= \(\frac{-1}{5}\)

Question 36. \(
\text { If } A=\left[\begin{array}{ll}
3 & 1 \\
7 & 5
\end{array}\right] \text { and } A^2+x I=y A \text {, then the values of } x \text { and } y \text { are }\)

1.  x = 6, y = 6

2.  x = 8, y = 8

3. x = 5, y – 8

4. x = 6, y = 8

Answer: 2.  x = 8, y = 8

⇒ \(\left[\begin{array}{ll}
3 & 1 \\
7 & 5
\end{array}\right]\left[\begin{array}{ll}
3 & 1 \\
7 & 5
\end{array}\right]+x\left[\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right]=y\left[\begin{array}{ll}
3 & 1 \\
7 & 5
\end{array}\right]\)

⇒ \(\left[\begin{array}{rr}
16 & 8 \\
56 & 32
\end{array}\right]+\left[\begin{array}{ll}
x & 0 \\
0 & x
\end{array}\right]=\left[\begin{array}{rr}
3 y & y \\
7 y & 5 y
\end{array}\right]\)

⇒ \(\left[\begin{array}{cc}
16+x & 8 \\
56 & 32+x
\end{array}\right]=\left[\begin{array}{cc}
3 y & y \\
7 y & 5 y
\end{array}\right]\)

y= 8 and (16 + x= 3y= 3 x 8= 24) => x= 8.

Question 37. If matrices A and B anticommuting, then

1. AB = BA

2. AB = -BA

3. (AB) = (BA)^-1

4. None of these

Answer: 2. AB = -BA

A and B anticommute ⇔ AB= -BA.

Question 38. \(
\text { If } A=\left[\begin{array}{ll}
2 & 5 \\
1 & 3
\end{array}\right], \text { then adj } A=\text { ? }\)

1. \(\left[\begin{array}{rr}
3 & -5 \\
-1 & 2
\end{array}\right]\)

2. \(\left[\begin{array}{rr}
3 & -1 \\
-5 & 2
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
-1 & 2 \\
3 & -5
\end{array}\right]\)

4. None of these

Answer: 1. \(\left[\begin{array}{rr}
3 & -5 \\
-1 & 2
\end{array}\right]\)

Question 39. \(
\text { If } A=\left[\begin{array}{rr}
3 & -4 \\
-1 & 2
\end{array}\right] \text { and } B \text { is a square matrix of order } 2 \text { such that } A B=I \text {, then }\) B= ?

1. \(\left[\begin{array}{ll}
1 & 2 \\
2 & 3
\end{array}\right]\)

2. \(\left[\begin{array}{ll}
1 & 2 \\
2 & 3
\end{array}\right]\)

3. \(\left[\begin{array}{ll}
1 & 2 \\
2 & 3
\end{array}\right]\)

4. None of these

Answer: 3. \(\left[\begin{array}{ll}
1 & 2 \\
2 & 3
\end{array}\right]\)

AB =7 ⇒ B = A^-1

Question 40. If A and B are invertible square matrices of the same order, then (AB)^-1 = ?

1. AB^-1

2. A^-1B

3. A^-1B^-1

4. B^-1A^-1

Answer: 4. B^-1A^-1

(AB)^-1 = B^-1 A^-1

Question 41. \(
\text { If } A=\left[\begin{array}{rr}
2 & -1 \\
1 & 3
\end{array}\right] \text {, then } A^{-1}=\text { ? }\)

1. \(\left[\begin{array}{cc}
\frac{3}{7} & \frac{-1}{7} \\
\frac{1}{7} & \frac{2}{7}
\end{array}\right]\)

2. \(\left[\begin{array}{cc}
\frac{3}{7} & \frac{-1}{7} \\
\frac{1}{7} & \frac{2}{7}
\end{array}\right]\)

3. \(\left[\begin{array}{cc}
\frac{3}{7} & \frac{-1}{7} \\
\frac{1}{7} & \frac{2}{7}
\end{array}\right]\)

4. None of these

Answer: 2. \(\left[\begin{array}{cc}
\frac{3}{7} & \frac{-1}{7} \\
\frac{1}{7} & \frac{2}{7}
\end{array}\right]\)

⇒  \(|A|=\left|\begin{array}{rr}
2 & -1 \\
1 & 3
\end{array}\right|=(6+1)=7 \neq 0\)

M₁₁ = 3, M₁₂= 1, M₂₁ = -1 and M₂₂= 2

∴ C₁₁= 3, C₁₂= -1, C₂₁ =1 and C₂₂= 2

⇒ A \(=\left[\begin{array}{rr}
3 & -1 \\
1 & 2
\end{array}\right]^{\prime}=\left[\begin{array}{rr}
3 & 1 \\
-1 & 2
\end{array}\right.]\)

Question 42. \(
\text { If }|A|=3 \text { and } A^{-1}=\left[\begin{array}{rr}
3 & -1 \\
\frac{-5}{3} & \frac{2}{3}
\end{array}\right], \text { then adj } A=?\)

1. \(\left[\begin{array}{rr}
9 & 3 \\
-5 & -2
\end{array}\right]\)

2. \(\left[\begin{array}{rr}
9 & -3 \\
-5 & 2
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
-9 & 3 \\
5 & -2
\end{array}\right]\)

4. \(\left[\begin{array}{rr}
9 & -3 \\
5 & -2
\end{array}\right]\)

Answer: 2. \(\left[\begin{array}{rr}
9 & -3 \\
-5 & 2
\end{array}\right]\)

⇒ \(
A^{-1}=\frac{1}{|A|} \cdot {adj} A \Rightarrow {adj} A=|A| \cdot A^{-1}=3 A^{-1}=\left[\begin{array}{rr}
9 & -3 \\
-5 & 2
\end{array}\right]\)

Question 43. \(
\text { If } A \text { is an invertible matrix and } A^{-1}=\left[\begin{array}{ll}
3 & 4 \\
5 & 6
\end{array}\right] \text {, then } A=\text { ? }\)

1. \(\left[\begin{array}{rr}
6 & -4 \\
-5 & 3
\end{array}\right]\)

2. \(\left[\begin{array}{ll}
\frac{1}{3} & \frac{1}{4} \\
\frac{1}{5} & \frac{1}{6}
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
-3 & 2 \\
\frac{5}{2} & \frac{-3}{2}
\end{array}\right]\)

4. None of these

Answer: 3. \(\left[\begin{array}{rr}
-3 & 2 \\
\frac{5}{2} & \frac{-3}{2}
\end{array}\right]\)

⇒ A = (A^-1)^-1. So, find the inverse of A^-1.

Question 44. \(
\text { If } A=\left[\begin{array}{rr}
1 & 2 \\
4 & -3
\end{array}\right] \text { and } f(x)=2 x^2-4 x+5, \text { then } f(A)=?\)

1. \(\left[\begin{array}{rr}
19 & 2 \\
4 & -3
\end{array}\right]\)

2. \(\left[\begin{array}{rr}
19 & -16 \\
-32 & 51
\end{array}\right]\)

3. \(\left[\begin{array}{rr}
19 & -11 \\
-27 & 51
\end{array}\right]\)

4. None of these

Answer: 2. \(\left[\begin{array}{rr}
19 & -16 \\
-32 & 51
\end{array}\right]\)

⇒ (A) = 2A²-4A+5I.

Question 45. \(
\text { If } A=\left[\begin{array}{ll}
1 & 4 \\
2 & 3
\end{array}\right], \text { then } A^2-4 A=\text { ? }\)

1. I

2. 5I

3. 3I

4. 0

Answer: 2. 5I

⇒ \(\left[\begin{array}{ll}
1 & 4 \\
2 & 3
\end{array}\right]\left[\begin{array}{ll}
1 & 4 \\
2 & 3
\end{array}\right]-\left[\begin{array}{ll}
4 & 16 \\
8 & 12
\end{array}\right]=\left[\begin{array}{ll}
9 & 16 \\
8 & 17
\end{array}\right]-\left[\begin{array}{ll}
4 & 16 \\
8 & 12
\end{array}\right]=\left[\begin{array}{ll}
5 & 0 \\
0 & 5
\end{array}\right]\)=5I

Question 46. If A is a 2-rowed square matrix and I A I =6, then A . adj A = ?

1. \(\left[\begin{array}{ll}
6 & 0 \\
0 & 6
\end{array}\right]\)

2. \(\left[\begin{array}{ll}
3 & 0 \\
0 & 3
\end{array}\right]\)

3. \(\left[\begin{array}{ll}
\frac{1}{6} & 0 \\
0 & \frac{1}{6}
\end{array}\right]\)

4. None of these

Answer: 1. \(\left[\begin{array}{ll}
6 & 0 \\
0 & 6
\end{array}\right]\)

⇒ A.(adj A) = IAI.

⇒ \(
I=6 \cdot\left[\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right]=\left[\begin{array}{ll}
6 & 0 \\
0 & 6
\end{array}\right]\)

Question 47. If A is an invertible square matrix and A:is a non-negative real number, (kA^-1) = ?

1. k. A^-1

2. \(\frac{1}{k} \cdot A^{-1}\)

3. – K. A^-1

4. None of these

Answer: 2. \(\frac{1}{k} \cdot A^{-1}\)

⇒ \((k A)^{-1}=\frac{1}{k} \cdot A^{-1}\) is true.

Question 48. \(
\text { If } A=\left[\begin{array}{rrr}
3 & 4 & 1 \\
1 & 0 & -2 \\
-2 & -1 & 2
\end{array}\right], \text { then } A^{-1}=\text { ? }\)

1. \(\left[\begin{array}{rrr}
2 & 9 & -8 \\
-2 & 8 & 7 \\
-1 & 5 & -4
\end{array}\right]\)

2. \(\left[\begin{array}{rrr}
2 & 9 & -8 \\
2 & 8 & 7 \\
-1 & -5 & -4
\end{array}\right]\)

3. \(\left[\begin{array}{rrr}
2 & -9 & -8 \\
2 & 8 & 7 \\
-1 & -5 & -4
\end{array}\right]\)

4. None of these

Answer: 3. \(\left[\begin{array}{rrr}
2 & -9 & -8 \\
2 & 8 & 7 \\
-1 & -5 & -4
\end{array}\right]\)

⇒ \(A^{-1}=\frac{1}{|A|}\).adj A.

Question 49. If A is a square matrix, then (A + A’) is

1. Anull matrix

2. An identity matrix

3. A symmetric matrix

4. A skew-symmetric matrix

Answer: 3. A symmetric matrix

⇒ A is a square matrix ⇒ (A + A’) is symmetric.

Question 50. If A is a square matrix, then (A- A’) is

1. Anull matrix

2. An identity matrix

3. A symmetric matrix

4. A skew-symmetric matrix

Answer: 4. A skew-symmetric matrix

A is a square matrix ⇒(A -A’) is skew-symmetric.

Question 51. If A is a 3-rowed square matrix and I 3 AI = k I  A I, then K=?

1. 3

2. 9

3. 27

4. 1

Answer: 3. 27

⇒ I3AI =( 3 x 3 x 3) IAI =27. I A I.

Question 52. Which one of the following is a scalar matrix?

1. \(\left[\begin{array}{ll}
1 & 1 \\
1 & 1
\end{array}\right]\)

2. \(\left[\begin{array}{ll}
6 & 0 \\
0 & 3
\end{array}\right]\)

3. \(\left[\begin{array}{ll}
8 & 0 \\
0 & -8
\end{array}\right]\)

4. None of these

Answer: 3. \(\left[\begin{array}{ll}
8 & 0 \\
0 & -8
\end{array}\right]\)

⇒ A scalar matrix is a square matrix each of whose non-diagonal elements is 0 and all diagonal elements are equal.

Question 53. \(
\text { If } A=\left[\begin{array}{ll}
1 & -1 \\
2 & -1
\end{array}\right] \text { and } B=\left[\begin{array}{rr}
a & 1 \\
b & -1
\end{array}\right] \text { and }(A+B)^2=\left(A^2+B^2\right) \text {, then }\)

1. a= 2, b= -3

2. a= -2, b= 3

3. a= 1, b= 4

4. None of these

Answer:  3. a= 1, b= 4

A+ B)² = (A²+ B²) ⇒ A²+ B²+AB+ BA = (A²+ B²) ⇒ AB =-BA

⇒ \(\left[\begin{array}{cc}
a-b & 2 \\
2 a-b & 3
\end{array}\right]=\left[\begin{array}{ll}
-a-2 & a+1 \\
-b+2 & b-1
\end{array}\right]\)

Now, (a +1 = 2 and b-1 = 3)⇒ (a =1 and 6 = 4).

Algebra Exercise 1B Review Of Facts And Formulae

1.

1.\(\left|\begin{array}{ll}
a_{11} & a_{12} \\
a_{21} & a_{22}
\end{array}\right|=\left(a_{11} a_{22}-a_{12} a_{21}\right)\)

2. \(\left|\begin{array}{lll}
a_{11} & a_{12} & a_{13} \\
a_{21} & a_{22} & a_{23} \\
a_{31} & a_{32} & a_{33}
\end{array}\right|\)

= \(a_{11} \cdot\left|\begin{array}{ll}
a_{22} & a_{23} \\
a_{32} & a_{33}
\end{array}\right|-a_{12} \cdot\left|\begin{array}{ll}
a_{21} & a_{23} \\
a_{31} & a_{33}
\end{array}\right|+a_{13} \cdot\left|\begin{array}{ll}
a_{21} & a_{22} \\
a_{31} & a_{32}
\end{array}\right|\)

\(=a_{11}\left(a_{22} a_{33}-a_{32} a_{23}\right)-a_{12}\left(a_{21} a_{33}-a_{31} a_{23}\right)+a_{13}\left(a_{21} a_{32}-a_{31} a_{22}\right)\)

2. 

1. Minor of a_ij is given by

M_ij= det. obtained after deleting ith row and jth column.

2. Co-factor of a_ij is given by C_ij= (-1)^i+j.M_ij

Δ= sum of the products of the elements of any row or column with their
corresponding co-factors.

Δ= a₁₁C₁₁+ a₁₂C₁₂ + a₁₃C₁₃

= a₁₁C₁₁+ a₂₁C₂₁ + a₃₁C₃₁

3. Properties of determinants

If the rows and columns of a determinant are interchange the value of the determinant remains unchanged.

⇒ R₁ ↔ R₃ shows the interchange of first and 3rd rows

If any two adjacent rows (or columns) of a determinant are interchanged, the value of the new determinant is the negative of the value of original determinant.

If all the elements of one row (or column) of a determinant are multiplied by k, the value of the original determinant is multiplied by k.

⇒ R₂—> R₂ shows the multiplication of each element of the second rowby k.

If the elements of a row (or a column) of a determinant are added k times the corresponding elements of another row (or column), the value of the determinant remains unchanged.

⇒ R_i —>R_j+ kR_j shows that k times the elements of jth row are added to the
corresponding elements of ith row.

If two rows (or columns) of a determinant are identical, the value of the
the determinant is zero.

If each element of a row (or a column) of a determinant is 0, the value of
the determinant is 0.

4.  Area of Δ A ABC with vertices A(x₁,y₁) B(x₂, y₂) and C(x₃, y₃) is given by

⇒ \(\Delta=\frac{1}{2}\left|\begin{array}{lll}
x_1 & y_1 & 1 \\
x_2 & y_2 & 1 \\
x_3 & y_3 & 1
\end{array}\right|\)

Points A(x₁,y₁) B(x₂, y₂) and C(x₃, y₃) are collinear ⇔ ar(Δ ABC) = 0 ⇔ Δ = 0.

Algebra Exercise 1B Multiple Choice Questions

Question 1. \(\left|\begin{array}{ll}
\cos 70^{\circ} & \sin 20^{\circ} \\
\sin 70^{\circ} & \cos 20^{\circ}
\end{array}\right|\)

1.  1
2.  0
3.  cos 50°
4. sin 50°

Answer: 2. 0

Question 2. \(\left|\begin{array}{ll}
\cos 15^{\circ} & \sin 15^{\circ} \\
\sin 15^{\circ} & \cos 15^{\circ}
\end{array}\right|\)

1. 1
2. \(\frac{1}{2}\)
3. \(\frac{\sqrt{3}}{2}\)
4. None of these

Answer:  3. \(\frac{\sqrt{3}}{2}\)

Δ = \(\left(\cos ^2 15^{\circ}-\sin ^2 15^{\circ}\right)\)

= cos (2×15° )= cos 30°

= \(\frac{\sqrt{3}}{2}\)

Question 3. \(\left|\begin{array}{rr}
\sin 23^{\circ} & -\sin 7^{\circ} \\
\cos 23^{\circ} & \cos 7^{\circ}
\end{array}\right|=?\)

1. \(\frac{\sqrt{3}}{2}\)
2. \(\frac{1}{2}\)
3. sin 16
4. cos 16

Answer: 2. \(\frac{1}{2}\)

Question 4. \(\left|\begin{array}{cc}
a+i b & c+i d \\
-c+i d & a-i b
\end{array}\right|\) = ?

1.  (a²+ b²– c²– d2²
2. (a²-b²+ c²-d²)
3. (a²+ b²+ c²+ d²)
4.  None of these

Answer: 3.  (a²+ b²+ c²+ d²)

Δ = \(\left|\begin{array}{cc}
a+i b & c+i d \\
-(c-i d) & a-i b
\end{array}\right|=(a+i b)(a-i b)+(c-i d)(c+i d)\)

= (a²+b²+c²+d²)

Question 5. \(\left|\begin{array}{lll}
a-b & b-c & c-a \\
b-c & c-a & a-b \\
c-a & a-b & b-c
\end{array}\right|\)=?

1.  (a + b + c)
2.  3[a+ b+ c)
3.  3abc
4.  0

Answer: 4. 0

R₁ -+ (R₁ + R₂+ R₃) gives all zeros in R₁ and this gives Δ= 0

Question 6. \(\left|\begin{array}{ccc}
1 & 1+p & 1+p+q \\
2 & 3+2 p & 1+3 p+2 q \\
3 & 6+3 p & 1+6 p+3 q
\end{array}\right|\) =?

1. 0
2. 1
3. -1
4. None of these

Answer: 2. 1

Applying R₂→ (R₂– 2R₁) and R₃ -+ (R₃– 3R₁) and expandingby C₁ we get Δ=1.

Question 7.  \(\left|\begin{array}{ccc}
1 & 1 & 1 \\
a & b & c \\
a^3 & b^3 & c^3
\end{array}\right|\) =?

1. (a-b)(b-c)(c-a)
2.  -(a-b)(b-c)(c-a)
3. (a- b)(b- c)(c- a)(a + b + c)
4. abc (a- b)(b- c){c- a)

Answer: 3. (a- b)(b- c)(c- a)(a + b + c)

Question 8. \(\left|\begin{array}{ccc}
1+a^2-b^2 & 2 a b & -2 b \\
2 a b & 1-a^2+b^2 & 2 a \\
2 b & -2 a & 1-a^2-b^2
\end{array}\right|=?\)

1. (1 + a²+ b²)
2. (1 + a2+ b²)²
3. (1 + a² + b²)³
4. None of these

Answer:  3. (1 + a² + b²)³

Apply R₁ → R₁ + bR₃ and R₂ → R₂ — aR₃

Question 9. \(\left|\begin{array}{lll}
\sin \alpha & \cos \alpha & \sin (\alpha+\delta) \\
\sin \beta & \cos \beta & \sin (\beta+\delta) \\
\sin \gamma & \cos \gamma & \sin (\gamma+\delta)
\end{array}\right|\)= ?

1. 0
2. 1
3. sin (a+ 8) + sin (P + 8) + sin (Y+ 8)
4.  None of these

Answer: 1. 0

Apply C₃→ C₃– (cos δ)C₁– (sinδ )C₂

Question 10. \(\left|\begin{array}{ccc}
x+y & x & x \\
5 x+4 y & 4 x & 2 x \\
10 x+8 y & 8 x & 3 x
\end{array}\right|\)

1. 0
2.  x³
3. y³
4. None of these

Answer: 2. x³

Take x common from C₂ and x common from C₃.

Apply R₃→R₃– 2R₂.

Question 11. \(\left|\begin{array}{lll}
b+c & c+a & a+b \\
c+a & a+b & b+c \\
a+b & b+c & c+a
\end{array}\right|=?\)

1. (a + b + c)
2. (a + b + c)²
3.  0
4. None of these

Answer: 4. None of these

Apply C₁ → (C₁ + C₁₂+ C₃).

Question 12. \(\left|\begin{array}{ccc}
a & b & c \\
a-b & b-c & c-a \\
b+c & c+a & a+b
\end{array}\right|=?\)

1. (a³ + b³ + c³)
2.  (a+b+c)³
3. 3abc(a + b + c)
4. (a³ + b³ + c³– 3abc

Answer: 4. (a³ + b³ + c³– 3abc

Apply C₁ →(C₁ + C₂+ C₃)

Question 13. \(\left|\begin{array}{ccc}
0 & a-b & a-c \\
b-a & 0 & b-c \\
c-a & c-b & 0
\end{array}\right|=?\)

1. (a+b+c)
2. (a+b-c)
3. 1
4. 0

Answer:  4. 0

Apply R₁ → (R₁ − R₁₂) and → (R₃−R₂)

Question 14. \(\left|\begin{array}{ccc}
a+b & a & b \\
a & a+c & c \\
b & c & b+c
\end{array}\right|=?\)

1. a+b+c
2. abc
3. 4abc
4. a²b²c²

Answer: 3. 4abc

Apply R₁ →  R₁– (R₂+ R₃).

Question 15.  \(\left|\begin{array}{lll}
a & 1 & b+c \\
b & 1 & c+a \\
c & 1 & a+b
\end{array}\right|=?\)

1. (a+b+c)
2. 2(a+b+c)
3. 0
4. None of these

Answer: 3. 0

Apply C₁ →  (C₁ + C₃) and take (a+ b+ c) common from C₁

Question 16.  \(\left|\begin{array}{ccc}
a-b-c & 2 b & 2 c \\
2 a & b-c-a & 2 c \\
2 a & 2 b & c-a-b
\end{array}\right|=?\)

1. (a+b+c)
2. (a+b+c)²
3. (a+b+c)³
4. None of these

Answer: 3. (a+b+c)³

Apply Cx₁→  C₁+ C₂+ C₃

Question 17. \(\left|\begin{array}{ccc}
a^2+1 & a b & a c \\
a b & b^2+1 & b c \\
c a & c b & c^2+1
\end{array}\right|=?\)

1.  (a²+b²+c²)
2. ( 1+a²+b²+c²)
3. ( 3+a²+b²+c²)
4. None of these

Answer: 2. ( 1+a²+b²+c²)

⇒ \(\Delta=\left|\begin{array}{ccc}
a\left(a+\frac{1}{a}\right) & a b & a c \\
a b & \left(b+\frac{1}{b}\right) b & b c \\
a c & b c & \left(c+\frac{1}{c}\right) c
\end{array}\right|=(a b c) \cdot\left|\begin{array}{ccc}
a+\frac{a}{a} & a & a \\
b & b+\frac{1}{b} & b \\
c & c & c+\frac{1}{c}
\end{array}\right|\)

⇒ \(=(a b c)\left|\begin{array}{ccc}
\frac{\left(a^2+1\right)}{a} & a & a \\
b & \frac{b^2+1}{b} & b \\
c & c & \frac{c^2+1}{c}
\end{array}\right|=\left(\frac{a b c}{a b c}\right) \cdot\left|\begin{array}{ccc}
a^2+1 & a^2 & a^2 \\
b^2 & b^2+1 & b^2 \\
c^2 & c^2 & c^2+1
\end{array}\right|\)

Now apply, R₁→ R₁+R₂+R₃

Question 18.  \(\left|\begin{array}{lll}
265 & 240 & 219 \\
240 & 225 & 198 \\
219 & 198 & 181
\end{array}\right|=?\)

1.  0
2.  78
3. -39
4. 108

Answer: 1. 0

Applying C₁→(C₁-C₃) and C₂→(C₂-C₃) we get:

⇒ \(\Delta=\left|\begin{array}{lll}
46 & 21 & 219 \\
42 & 27 & 198 \\
38 & 17 & 181
\end{array}\right|=\left|\begin{array}{rrr}
4 & 21 & 9 \\
-12 & 27 & -72 \\
4 & 17 & 11
\end{array}\right|\)

C₁→(C₁-2C₂)

C₃→(C₃-10C₂)

Now , apply R₁→(R₁-R₃) and R₂→(R₂-3R₃)

Question 19. \(\left|\begin{array}{lll}
1^2 & 2^2 & 3^2 \\
2^2 & 3^2 & 4^2 \\
3^2 & 4^2 & 5^2
\end{array}\right|=?\)

1. 8
2. -8
3. 16
4. 142

Answer: 2. -8

Question 20.\(\left|\begin{array}{lll}
1 ! & 2 ! & 3 ! \\
2 ! & 3 ! & 4 ! \\
3 ! & 4 ! & 5 !
\end{array}\right|=?\)

1. 2
2. 6
3. 24
4. 120

Answer: 3. 24

Question 21.\(\left|\begin{array}{ccc}
1 & 1 & 1 \\
1 & 1+x & 1 \\
1 & 1 & 1+y
\end{array}\right|\)

1.  (x+y)
2. (x-y)
3. xy
4.  None of these

Answer: 3. xy

Question 22. \(\left|\begin{array}{lll}
a & b & c \\
b & c & a \\
c & a & b
\end{array}\right|=?\)

1. abc (a+b+c)
2. (a³ -b³-c³ + 3abc)
3. (-a³ -b³-c³ + 3abc)
4. None of these

Answer: 3. (-a³ -b³-c³ + 3abc)

Question 23. If a, b, cbe distinct positive real numbers, then the value of \(\left|\begin{array}{lll}
a & b & c \\
b & c & a \\
c & a & b
\end{array}\right|\) is

1. Positive
2. Negative
3. A perfect square
4. 0

Answer: 2. Negative

Δ= -(a+ b+ c)(a²+ b²+ c²-ab-bc-ca)

=- \(\frac{1}{2}\) (a+ b + c)[(a- b)²+ (b- c)²+ (c- a)²], which is negative.

Question 24. \(\left|\begin{array}{ccc}
-a^2 & a b & a c \\
a b & -b^2 & b c \\
a c & b c & -c^2
\end{array}\right|=?\)

1. 0
2. abc
3. 4 a²b²c²
4. None of these

Answer: 3. 4 a²b²c²

Question 25. \(\left|\begin{array}{lll}
b c & b+c & 1 \\
c a & c+a & 1 \\
a b & a+b & 1
\end{array}\right|=?\)

1. (a-b)(b-c)(c-a)
2. – (a-b)(b-c)(c-a)
3. (a+b)(b+c)(c+a)
4.  None of these

Answer: 1. (a-b)(b-c)(c-a)

Question 26. \(\left|\begin{array}{ccc}
a^2+2 a & 2 a+1 & 1 \\
2 a+1 & a+2 & 1 \\
3 & 3 & 1
\end{array}\right|=?\)

1. (a-1)²
2. (a-1)³
3. (a-1)
4. None of these

Answer: 2. (a-1)³

Apply R₁→ (R₁ — R₂) and R₃ → (R₃ — R₂)

Question 27. \(\left|\begin{array}{lll}
\frac{1}{a} & a^2 & b c \\
\frac{1}{b} & b^2 & a c \\
\frac{1}{c} & c^2 & a b
\end{array}\right|=?\)

1. 0
2. 1
3. -1
4. None of these

Answer: 1.0

⇒ \(\Delta=\frac{1}{a b c}\left|\begin{array}{ccc}
1 & a^3 & a b c \\
1 & b^3 & a b c \\
1 & c^3 & a b c
\end{array}\right|=\left(\frac{a b c}{a b c}\right) \cdot\left|\begin{array}{ccc}
1 & a^3 & 1 \\
1 & b^3 & 1 \\
1 & c^3 & 1
\end{array}\right|=0\)

Question 28.\(\left|\begin{array}{ccc}
x+1 & x+2 & x+4 \\
x+3 & x+5 & x+8 \\
x+7 & x+10 & x+14
\end{array}\right|=?\)

1.  -2
2. 2
3. x²– 2
4. x + 2

Answer: 1. -2

Apply C₂→(C₂– C₁) and C₃ → (C₃ — C₁).

Question 29.\(\left|\begin{array}{ccc}
b+c & a & a \\
b & c+a & b \\
c & c & a+b
\end{array}\right|=?\)

1.  4abc
2.  2(a + b + c)
3.  (ab + bc + ca)
4.  None of these

Answer: 1. 4abc

Apply R₁→R₁– (R₂+R₃) and then apply R₂ → (R₂-R₁) and R₃ → (R₃-R₁).

Question 30. \(\left|\begin{array}{ccc}
a & a+2 b & a+2 b+3 c \\
3 a & 4 a+6 b & 5 a+7 b+9 c \\
6 a & 9 a+12 b & 11 a+15 b+18 c
\end{array}\right|=?\)

1.  a³
2. -a³
3. 0
4. None of these

Answer: 2. -a³

Apply R₂ → R₂ − 3R₁ and R₃→ R₃ − 6R₁.

Question 31. \(\left|\begin{array}{lll}
1 & b c & b c(b+c) \\
1 & c a & c a(c+a) \\
1 & a b & a b(a+b)
\end{array}\right|=?\)

1.  abc
2.  2 abc
3. abc(a+b+c)
4. 0

Answer: 4. 0

Apply R₂ → R₂– R₁ and R₃ → R₃-R₁.

Question 32. The value of \(\left|\begin{array}{ccc}
\cos (\theta+\phi) & -\sin (\theta+\phi) & \cos 2 \phi \\
\sin \theta & \cos \theta & \sin \phi \\
-\cos \theta & \sin \theta & \cos \phi
\end{array}\right|\) is

1.  Independent of o only
2.  Independent of o only
3.  Independent of o only
4.  Dependent of o only

Answer: 1. Independent of o only

Apply R₁ → R₁+ (sin Φ)R₂ − (cos Φ)R₃.

Take (2cosΦ) common from R₁. Now, apply R₁ →4 (R₁ +R₃).

Question 33. \(\left|\begin{array}{lll}
b+c & a & b \\
c+a & c & a \\
a+b & b & c
\end{array}\right|=?\)

1. (a + b+ c)(a- c)
2.  (a + b + c)(b- c)
3.  (a + b + c)(a-cf
4.  (a + b + c)(b- c)

Answer: 3. (a + b + c)(a-cf)

Express A as the sum of two determinants and simplify each.

Question 34. If co is a complex root of unity, then \(\left|\begin{array}{ccc}
1 & \omega & \omega^2 \\
\omega & \omega^2 & 1 \\
\omega^2 & 1 & \omega
\end{array}\right|=?\)

1. 1
2. -1
3.  0
4. None of these

Answer: 3. 0

Apply R₁→R₁+ R₂+ R₃ and use the result (1 + ω+ ω²) = 0.

Question 35. If ω is a complex cube root of unity, then the value of \(\left|\begin{array}{ccc}
1 & \omega & 1+\omega \\
1+\omega & 1 & \omega \\
\omega & 1+\omega & 1
\end{array}\right|\)

1. 2
2.  4
3.  0
4. -3

Answer: 2. 4

1 +ω+ ω² => (1 + ω) = – ω². Put (1 + ω) = -ω² and expand.

Question 36. If \(\left|\begin{array}{lll}
a+b & b+c & c+a \\
b+c & c+a & a+b \\
c+a & a+b & b+c
\end{array}\right|=k\left|\begin{array}{lll}
a & b & c \\
b & c & a \\
c & a & b
\end{array}\right|, \text { then } k=?\)

1. 0
2.  1
3.  2
4. -2

Answer:  3. 2

Question 37. \(\text { The solution set of the equation }\left|\begin{array}{lll}
x & 3 & 7 \\
2 & x & 2 \\
7 & 6 & x
\end{array}\right|=0 \text { is }\)

1. {2,-3,7}
2.  {2,7,-9}
3. {-2,3,-7}
4.  None of these

Answer: 2. {2,7,-9}

Apply C₁ → C₁  −C₃ and take (x-7) common from C₁

Question 38. The solution set of the equation \(\left|\begin{array}{ccc}
x-2 & 2 x-3 & 3 x-4 \\
x-4 & 2 x-9 & 3 x-16 \\
x-8 & 2 x-27 & 3 x-64
\end{array}\right|\) is

1.  {4}
2.  {2,4}
3.  {2,8}
4.  {4,8}

Answer: 1. {4}

Apply C₂ → C₂ – 2C₁ and C₃→ C₃– 3C₁

Then, apply R₂ → R₂− R₁ and R₃→ R₃− R₁

Question 39. The solution set of the equation \(\left|\begin{array}{lll}
a+x & a-x & a-x \\
a-x & a+x & a-x \\
a-x & a-x & a+x
\end{array}\right|=0 \text { is }\) = 0 is

1. {1,0}
2.  {3a,0}
3.  {a,3a}
4.  None of these

Answer: 2. {3a,0}

Apply C₁ → (C₁ + C₂+ C₃) and take (3a- x) common from C₁

Question 40. The solution set of the equation \(\left|\begin{array}{rrr}
5 & 3 & -1 \\
-7 & x & 2 \\
9 & 6 & -2
\end{array}\right|=\) =0 is

1.  {0}
2.  {6}
3.  {-6}
4.  {0,9}

Answer: 3. {-6}

Question 41. The solution set of the equation \(\left|\begin{array}{ccc}
3 x-8 & 3 & 3 \\
3 & 3 x-8 & 3 \\
3 & 3 & 3 x-8
\end{array}\right|\) = 0 is

1.  \(\left\{\frac{2}{3}, \frac{8}{3}\right\}\)
2.  \(\left\{\frac{2}{3}, \frac{11}{3}\right\}\)
3.  \(\left\{\frac{3}{2}, \frac{8}{3}\right\}\)
4. None of these

Answer: 2. \(\left\{\frac{2}{3}, \frac{11}{3}\right\}\)

Question 42. The vertices of a A ABC are A(-2, 4), B(2, -6) and C(5, 4). The area of Δ ABC is

1.  17.5 sq units
2.  35 sq units
3. 32 sq units
4. 28 squats

Answer: 2. 35 sq units

⇒ \(\Delta=\frac{1}{2}\left|\begin{array}{rrr}
-2 & 4 & 1 \\
2 & -6 & 1 \\
5 & 4 & 1
\end{array}\right|=\frac{1}{2}\left|\begin{array}{rrr}
-2 & 4 & 1 \\
4 & -10 & 0 \\
7 & 0 & 0
\end{array}\right|\)

= 35 sq units

Question 43. If the points A(3, -2), B(k, 2) and C(8, 8) are collinear, then the value of k is

1. 2
2.  -3
3. 5
4. -4

Answer: 3. 5

⇒ \(\text { If } \Delta=\left|\begin{array}{rrr}
3 & -2 & 1 \\
k & 2 & 1 \\
8 & 8 & 1
\end{array}\right| \text {, then we must have } \Delta=0 \text {. }\)

Relations And Functions

Relations And Functions Exercise 1A Review Of Facts And Formulae

1. A relation R in a set A is a subset of A x A

If (a, b) ∈ R, then a R b.

If (a, b) ∉ R, then a is not related to b.

Domain (R) = {a: (a, b) ∈ R] and Range (R) = {b: (a, b) ∈ R}.

2. Let R be a relation on a non-empty set A. Then, R is said to be:

1. Reflexive, if (a, a) ∈ R for each a ∈ A

i.e., if a R a for each a ∈ R.

2. Symmetric, if (a, b) ∈ R ⇒ (b, a) ∈ R

i.e., a R b ⇒ b Ra

3. Transitive, if (a, b) e R and (b, c)e R ⇒ (a, c) ∈ R

Read and Learn More WBCHSE Solutions For Class 12 Maths

i.e., a R b, b R c ⇒  a R c.

3. A relation R on a set A is said to be an equivalence relation if it is reflexive, symmetric, and transitive.

4. Equivalence class, determined by an element a is defined as a

⇒ [a] = [b ∈ A : (a, b) ∈ R}

Relations And Functions Exercise 1A Multiple Choice Questions

Question 1. Let A = {1, 2, 3} and let R = {(1, 1), (2, 2), (3, 3), (1, 3), (3, 2), (1, 2)}. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 2. Reflexive and transitive but not symmetric

R is reflexive and transitive but not symmetric

 

Question 2. Let A = {a, b, c} and let R= {(a, a), (a, b), (b, a)}. Then, Ris

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 3. Symmetric and transitive but not reflexive

R is symmetric and transitive but not reflexive

Question 3. Let A = {1,2, 3} and let R= {(1,1), (2, 2), (3, 3), (1, 2), (2, 1), (2, 3), (3, 2)}. Then, R is

1. Reflexive and symmetric but not transitive
2. Symmetric and transitive but not reflexive
3. Reflexive and transitive but not symmetric
4. An equivalence relation

Answer: 1. Reflexive and symmetric but not transitive

(1,2)∈ R and (2,3) ∈ R, But, (1,3) ∉ R So, R is not transitive.

Question 4. Let S be the set of all straight lines in a plane. Let R be a relation on S Defined by a R b ⇔ a ⊥ b. Then, R is

1. Reflexive but neither symmetric nor transitive
2. Symmetric but neither reflexive nor transitive
3. Transitive but neither reflexive nor symmetric
4. An equivalence relation

Answer: 2. Symmetric but neither reflexive nor transitive

a ⊥ b is not true. So R is not reflexive

a ⊥ b and b ⇒ b ⊥ a is always true.

Question 5. Let S be the set of all straight lines in a plane. Let R be a relation on S Defined by a R b ⇔ a || b. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 4. An equivalence relation

Question 6. Let Z be the set of all integers and let R be a relation on Z defined by a R b o (a-b) is divisible by 3. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 4. An equivalence relation

Question 7. Let R be a relation on the set N of all natural numbers, defined by a R b ⇔  a is a factor of b. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 2. Reflexive and transitive but not symmetric

Question 8. Let Z be the set of all integers and let R be a relation on Z defined by a R b ⇔  a>b. Then, R is

1. Symmetric and transitive but not reflexive
2. Reflexive and symmetric but not transitive
3. Reflexive and transitive but not symmetric
4. An equivalence relation

Answer: 3. Reflexive and transitive but not symmetric

Question 9. Let S be the set of all real numbers and let R be a relation on S defined by a R b ⇔ IaI ≤  b. Then, R is

1. Reflexive but neither symmetric nor transitive
2. Symmetric but neither reflexive nor transitive
3. Transitive but neither reflexive nor symmetric
4. None of these

Answer: 3. Transitive but neither reflexive nor symmetric

⇒  I-3I ≤ -3 is not true. So, R is not reflexive.

⇒ I-1I ≤ 1 ⇒ (-1) R1. But I-1I ≤ 1 is not true.

∴ R is not symmetric

3. a R b, b R c ⇒ IaI ≤  b and IbI ≤  c ⇒ IaI ≤  c.

Question 10. Let S be the set of all real numbers and let R be a relation on S, defined by a R b ⇔ Ia-bI ≤ 1. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 1. Reflexive and symmetric but not transitive

⇒  Ia- aI = 0 ≤ 1 is always true.

⇒  a R b ⇒ Ia- b I≤1 ⇒ I-(a-b)I<1 ⇒ Ib-aI <1 ⇒  b R a.

⇒  2 R 1 and 1 R \(\frac{1}{2}\).

But, 2 is not related to \(\frac{1}{2}\).  So, R is not transitive.

Question 11. Let S be the set of all real numbers and let R be a relation on S, defined by a R b ⇔(1 + ab) > 0. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. None of these

Answer: 1. Reflexive and symmetric but not transitive

a R a, since (1 + a²) > 0.

a R b ⇒ (1 + ab) > 0 ⇒ (1 + ba) > 0 ⇒b R a.

Let a = \(\frac{-1}{2}\), b = \(\frac{-1}{2}\) and c = R.

Then, a R b and b R c. But, a is not related to c.

Question 12. Let S be the set of all triangles in a plane and let R be a relation on S defined by Δ₁S Δ₂ ⇔ Δ₁ ≡ Δ₂. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 4. An equivalence relation

Question 13. Let S be the set of all real numbers and let R be a relation on S defined by a R b ⇔ a² + b² = 1. Then, R is

1. Symmetric but neither reflexive nor transitive
2. Reflexive but neither symmetric nor transitive
3. Transitive but neither reflexive nor symmetric
4. None of these

Answer: 1. Symmetric but neither reflexive nor transitive

(l² + 1²) ≠ 1. So,1 R 1 is not true.

a R b ⇒ a²+ b² =1 ⇒ b² + a² =1 ⇒  b R a.

1 R 0 and 0 R 1. But,1 is not related to 1.

Question 14. Let JR be a relation on N x N, defined by (a, b) R (c, d)⇔ a+d= b+ c. Then, R is 

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 4. An equivalence relation

Question 15. Let A be the set of all points in a plane and let O be the origin. Let R = {(P, Q): OP= OQ}. Then, R is

1. Reflexive and symmetric but not transitive
2. Reflexive and transitive but not symmetric
3. Symmetric and transitive but not reflexive
4. An equivalence relation

Answer: 4. An equivalence relation

Question 16. Let Q be the set of all rational numbers and * be the binary operation, defined by a * b = a + 2b, then

1. * is commutative but not associative
2. * is associative but not commutative
3. * is neither commutative nor associative
4. * is both commutative and associative

Answer: 3. * is neither commutative nor associative

Question 17. Let a * b = a + ab for all a, b ∈ Q. Then,

1. * Is not a binary composition
2. * Is not commutative
3. * Is commutative but not associative
4. * Is both commutative and associative

Answer: 2. * Is not commutative

Question 18. Let Q⁺ be the set of all positive rationals. Then, the operation * on Q⁺ defined by a * b \(=\frac{a b}{2}\) for all a, b ∈ Q⁺ is

1. Commutative but not associative
2. Associative but not commutative
3. Neither commutative nor associative
4. Both commutative and associative

Answer: 4. Both commutative and associative

Question 19. Let Z be the set of all integers and let a*b = a – b + ab. Then, * is

1. Commutative but not associative
2. Associative but not commutative
3. Neither commutative nor associative
4. Both commutative and associative

Answer: 3. Neither commutative nor associative

Question 20. Let Z be the set of all integers. Then, the operation * on Z defined by a*b= a + b – ab is

1. Commutative but not associative
2. Associative but not commutative
3. Neither commutative nor associative
4. Both commutative and associative

Answer: 4. Both commutative and associative

Question 21. Let Z+be the set of all positive integers. Then, the operation * on Z+ defined by a * b = a^b is

1. Commutative but not associative
2. Associative but not commutative
3. Neither commutative nor associative
4. Both commutative and associative

Answer: 3. Neither commutative nor associative

Question 22. Define * on Q- {-1} by a * a+ b+ ab. Then, * on Q- {-1} is

1. Commutative but not associative
2. Associative but not commutative
3. Neither commutative nor associative
4. Both commutative and associative

Answer: 4. Both commutative and associative

Relations And Functions Exercise 1B Review Of Facts And Formulae

1. Function: Let A and B be two non-empty sets. Then, a rule / which associates to each x e A, a unique element fix) of B, is called a function from A to B, and we write, \(f\): A → B.

Dom (f) = {x ∈ A :f(x) ∈ B}, range  (f)= {f(x): x ⇒ A}.

2. Let f: A→ B. Then

⇒ f is one-one ⇔ {f(x₁) =f(x₂) ⇒ x₁ = x₂}

⇒  f is onto ⇔ range (f) = B.

3. Two functions/ and g are said to be equal if they have the same domain and f(x)=g(x) ∀ x.

4. Invertible functions: A function is said to be invertible if/is one-one and

onto.f{x) = y ⇒  f^-1(y) = x.

5. Composite of two functions:

⇒ Let/: A → B and g: B→ C, then g o f: A →  C is called the composite of f and g.

⇒ Dom (g o f) = dom (f)

g o f is defined only when range (f) ⊆ dom (g)

⇒ Dom (f o g) = dom(g).

f o g is defined only when range (g) ⊆ dom (f)

Relations And Functions Exercise 1B Multiple Choice Questions

Question 1. f : N → N : f(x) = 2x is

1. One-one and onto
2. One-one and into
3. Many-one and onto
4. Many-one and into

Answer: 2. One-one and into

⇒ 2x =  3

⇒ x =  \(\frac{3}{2}\)

∴ f is into.

Question 2. f : N → N : f(x) = x²+ x+1 is

1. One-one and onto
2. One-one and into
3. Many-one and onto
4. Many-one and into

Answer: 2. One-one and into

⇒  f(x₁) = f(x₂)

= x²₁+x₁ +1

= x²₂+x₂ +1

= (x²₁– x²₂) + (x₁-x₂) = 0

⇒ (x₁-x₂)(x₁+x₂+1) = 0

= x₁– x₂= 0

=  x₁ = x₂.

∴ f is one-one.

f(x) =1 ⇒  x²+ x+1 =1 ⇒  x(x+ 1) = 0 ⇒ x= 0 or x=-1.

And, none of 0 and -1 is in N. So, f is into.

Question 3. f: R→ R : f(x) = x² is

1. One-one and onto
2. One-one and into
3. Many-one and onto
4. Many-one and into

Answer: 4. Many-one and into

Question 4. f: R→ R : f(x) = x³ is

1. One-one and onto
2. One-one and into
3. Many-one and onto
4. Many-one and into

Answer: 1. Many-one and into

Question 5. f : R⁺→+R⁺: f(x) = e^x is

1. Many-one and into
2. Many-one and onto
3. One-one and into
4. One-one and onto

Answer: 4. One-one and onto

⇒ f(x₁) = f(x₂) => e^x₁ = e^x₂

⇒ x₁ = x₂

∴ f is one-one.

For each x ∈ R⁺ ∃ log x ∈ R⁺ s.t. f(log x)= x.

So,/is onto.

Question 6. \(f:\left[\frac{-\pi}{2}, \frac{\pi}{2}\right] \rightarrow[-1,1]: \) f(x) = sin x is

1. One-one and into
2. One-one and onto
3. Many-one and into
4. Many-one and onto

Answer: 2. One-one and onto

Question 7. f : R → R :f(x) = cos x is

1. One-one and into
2. One-one and onto
3. Many-one and into
4. Many-one and onto

Answer: 3. Many-one and into

Cos (2π- θ) = cos θ ⇒ f is Many-one.

Range (f) = [-1, 1] ⊂ R =>  f is into.

Question 8. f : C→ R : f(z) = IzI is

1. One-one and into
2. One-one and onto
3. Many-one and into
4. Many-one and onto

Answer: 3. Many-one and into

i ≠-i. But f(i) = f(-i)=1. So, fis many-one.

-1 ∈ R having no pre-image in C. So, fis into.

Question 9. Let A = R- {3} and B =R- {1}. Then f: A → B : f(x) = \(\frac{(x-2)}{(x-3)}\) is 

1. One-one and into
2. One-one and onto
3. Many-one and into
4. Many-one and onto

Answer: 2. One-one and onto

⇒ f(x₁)= f(x₂)

⇒ \(\frac{\left(x_1-2\right)}{\left(x_1-3\right)}=\frac{\left(x_2-2\right)}{\left(x_3-3\right)}\)

⇒ x₁= x₂, So f is one one.

Let \(\frac{x-2}{x-3}\). Then, x\(=\frac{3 y-2}{y-1}\). Clearly y ≠1 and x≠3

Question 10.  Let f: N → N: f(n)=

\(\frac{1}[2}\)(n+1) when n is odd

\(\frac{n}{2}\),when n is even

1. One-one and into
2. One-one and onto
3. Many-one and onto
4. Many-one and into

Answer: 4. Many-one and into

f (1) = f(2) shows that/is many-one.

If n is odd, then (2n- 1) is odd, and f(2n- 1) =n.

If n is even, then 2n is even, and f (2n) = n.

∴ f is onto.

Question 11. Let A and B be two non-empty sets and let f: (A x B) → (B x A): f(a, b) = (b, a). Then, f is

1. One-one and onto
2. One-one and into
3. Many-one and onto
4. Many-one and into

Answer: 1. One-one and onto

Question 12. Let f: Q→ Q: f(x) = (2x + 3). Then,f^-1(y) = ?

1. (2y- 3)
2. \(\\)
3. \(\frac{1}{2}\)(y-3)
4. None of these

Answer: 3. \(\frac{1}{2}\)(y-3)

y = 2x+3

x = \(\frac{1}{2}(y-3)\)

⇒ \(f^{-1}(y)=\frac{4 y}{(4-3 y)}\)

Question 13.\(\text { Let } f: R-\left\{\frac{-4}{3}\right\} \rightarrow R-\left\{\frac{4}{3}\right\}: f(x)=\frac{4 x}{(3 x+4)} \text {. Then, } f^{-1}(y)=\text { ? }\)

1. \(\frac{4 y}{(4-3 y)}\)
2. \(\frac{4 y}{(4+3 y)}\)
3. \(\frac{4 y}{(3 y-4)}\)
4. None of these

Answer: 1. \(\frac{4 y}{(4-3 y)}\)

⇒ \(y\frac{4 x}{3 x+4}\)

⇒ \(x\frac{4 y}{(4-3 y)}\)

⇒ \(f^{-1}(y)=\frac{4 y}{(4-3 y)}\)

Question 14. Let f : N→X : f(x) = 4x² + 12x+ 15. Then f^-1(y) =?

1. \(\frac{1}{2}(\sqrt{y-4}+3)\)
2. \(\frac{1}{2}(\sqrt{y-6}-3)\)
3. \(\frac{1}{2}(\sqrt{y-4}+5)\)
4. None of these

Answer: 2. \(\frac{1}{2}(\sqrt{y-6}-3)\)

y = 4x² +12x+15

= (2x+3)² +6

⇒ \(x\frac{1}{2}(\sqrt{y-6}-3)\)

⇒ \(f^{-1}(y)=\frac{1}{2}(\sqrt{y-6}-3)\)

Question 15. \(\text { If } f(x)=\frac{(4 x+3)}{(6 x-4)}, x \neq \frac{2}{3} ; \text { then }(f \circ f)(x)=\text { ? }\)

1. x
2. (2x-3)
3. \(\frac{4 x-6}{3 x+4}\)
4. None of these

Answer: 1. x

f(x)= \(\frac{4 x+3}{6 x-4}\) = y(say)

Then f(y)= \(\frac{4 y+3}{6 y-4}\)

= \(=\frac{4\left(\frac{4 x+3}{6 x-4}\right)+3}{6\left(\frac{4 x+3}{6 x-4}\right)-4}\)

= \(=\frac{34 x}{34}\)

= x

⇒ f[f(x)] = x ⇒ (f o f) (x) = x

Question 16. If f(x) = (x² — 1) and g(x) = (2x + 3), then (g o f)(x) = ?

1. (2X²+ 3)
2. (3X² + 2)
3. (2x² + 1)
4. None of these

Answer: 3. (2x²+ 1)

(g o f )(x) = g [f(x)] = g(x²-1)

= 2(x²-1)+3 = (2x²+1)

Question 17. \(\text { If } f\left(x+\frac{1}{x}\right)=x^2+\frac{1}{x^2}, \text { then } f(x)=?\)

1. x²
2. x-1
3. x-2
4. None of these

Answer: 3. x-2

Let  \(x+\frac{1}{x}\)= z Then,

f(z) = \(f\left(x+\frac{1}{x}\right)=\left(x^2+\frac{1}{x^2}\right)=\left(x+\frac{1}{x}\right)^2-2\)= (z-2)

f(z)= (x²-2)

Question 18. \(\text { If } f(x)=\frac{1}{(1-x)^{\prime}} \text {, then }(f \circ f \circ f)(x)=\text { ? }\)

1. \(\frac{1}{(1-3 x)}\)
2. \(\frac{x}{(1+3 x)}\)
3. x
4. None of these

Answer: 3. x

(f o f)(x) = f{f(x)}

= \(f\left(\frac{1}{1-x}\right)=\frac{1}{\left(1-\frac{1}{1-x}\right)}\)

= \(\frac{1-x}{-x}=\frac{x-1}{x}\)

{f o(f o f)}(x) ={f o(f o f)(x)}]= \(=f\left(\frac{x-1}{x}\right)=\frac{1}{1-\frac{x-1}{x}}=x^1/3\)

Question 19. \(\text { If } f(x)=\sqrt[3]{3-x^3}, \text { then }(f \circ f)(x)=\text { ? }\)

1. x1/3
2. x
3. (1-x1/3)
4. None of these

Answer: 2. x

(fof)(x) =f{f(x)}- {(3- x3) ^1/3} = f/(y), where y= (3- x³) ^1/3

= (3- y³)^1/3= [3- {3- x³}]^1/3= (x³)^1/3= x

Question 20. If f(x) = x²– 3x + 2, then (f o f)(x) = ?

1. x
2. x⁴-6x³
3. x⁴– 6x³+10x²
4. None of these

Answer: 4. None of these

(fof)(x) =f{f(x)} =f{x²– 3x + 2) =f(y), where y= (x²– 3x+ 2)

= y²– 3y+ 2= (x²– 3x+ 2)²– 3(x²– 3x+ 2) + 2= (x⁴– 6x³ + 10x²– 3x).

Question 21. If f(x) = 8x³ and g(x) = x^1/3, then (g o f)(x) = ?

1. x
2. 2x
3. \(\frac{x}{2}\)
4. 3×2

Answer: 2. 2x

(g of)(x) = g{f(x)] = g(8x³) = (8x³)^1/3= 2x.

Question 22. If f (x) = x², g(x) = tan x and h(x) = log x, then {ho(gof}}\(\left(\sqrt{\frac{\pi}{4}}\right)\)

1. 0
2. 1
3. \(\frac{1}{x}\)
4. \(\frac{1}{2} \log \frac{\pi}{4}\)

Answer: 1. 0

{ho(g o f)}(x) = (ho g){f(x)} = (h o g)(x²)

= h{g(x²)} = h(tan x²) = log(tan x²)

∴ \(\{h \circ(g \circ f)\} \sqrt{\frac{\pi}{4}}=\log \left(\tan \frac{\pi}{4}\right)\)

= log 1

= 0

Question 23. If/= {(1, 2), (3, 5), (4, 1)} andg= {(2, 3), (5, 1), (1, 3)}, then (g o f) = ?

1. {(3,1), (1,3), (3, 4)}
2. {(1,3), (3, 1), (4, 3)}
3. {(3,1), (1,3), (3, 4)}
4. {(2, 5), (5, 2), (1,5)}

Answer: 2. {(1,3), (3, 1), (4, 3)}

⇒ Dom (gof) = Dom (f) = {1, 3, 4}

⇒ (g o f )(l) =g{f(l)} = g(2) = 3,(g of)(3) =g{f(3)} =g(5) =1

⇒ (g o f)(4) = g[f(4)) =g(1) = 3

⇒ g o f= {(1/3), (3, 1), (4, 3)}.

Question 24. Let/(x) = \(\sqrt{9-x^2}\). Then, dom (f) =?

1. [-3, 3]
2. (-∞, -3]
3. [3, ∞)
4. (-∞, -3] u (4, ∞)

Answer: 1. [-3, 3]

f(x) is defined only when 9- x² > 0 => x² <9 =» -3 < x < 3.

∴ Dom (f) =  [-3, 3].

Question 25. Let f(x) \(\sqrt{\frac{x-1}{x-4}}\). Then, dom (f) = ?

1. [1,4)
2. [1,4]
3. (-∞,4]
4. (-∞,1]∪ (4,∞)

Answer: 4. (-∞,1]∪ (4,∞)

f(x) is defined when-4≠ 0 and \(\frac{x-1}{x-4}\)

⇒ x ≠ 4 and (x ≥ 4 or x<l) => (x>4 or x<l)

⇒  Dom (f) = (-∞, 1]u (4, ∞).

Question 26. Let f(x) \(f(x)=e^{\sqrt{x^2-1}}\). Then, dom (f) =?

1. (∞-,1]
2. [-1,∞)
3. (1,∞)
4. (-∞,-1](1,∞)

Answer: 3. (1,∞)

f(x) is defined only when (x²-1) > 0 and (x- 1) > 0

⇒ (x – l)(x+ 1) ≥ 0 and (x- 1) > 0 => x+1 > 0 and x-1 > 0⇒ x >1

∴ dom (f) = (1, ∞).

Question 27. Let f(x) = \(\frac{x}{\left(x^2-1\right)}\) . Then, dom (f) =?

1. R
2. R-1
3. R- {-1}
4. R- {-1,1}

Answer: 4. R- {-1,1}

f(x) is not defined when (x- 1) = 0, i.e., when (x- l)(x+ 1) = 0,

i.e., when x=1 or x= -1.

dom (f) = R—{1, -1}.

Question 28. Let f(x)= \(\). Then ,dom(f) = ?

1. (-1,1)
2. [-1/ 1]
3. [-l, 1]- {0}
4. None of these

Answer: 3. [-l, 1]- {0}

⇒ \(\frac{\sin ^{-1} x}{x}\) is defined only when x≠ 0 and x ∈ [-1,1].

∴ dom (f)= [-1,1]- {0}.

Question 29. Let f(x) = cos^-12x. then, dom (f) =?

1. [-1,1]
2. \(\left[\frac{-1}{2}, \frac{1}{2}\right]\)
3. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)
4. \(\left[\frac{-\pi}{4}, \frac{\pi}{4}\right]\)

Answer: 2. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)

sin^-12x is defined only when 2x ∈ [-1, 1] => x \(\left[\frac{-1}{2}, \frac{1}{2}\right]\)

Question 30. Let f(x) = Cos^-1(3x-1) . Then, dom (f) =?

1. \(\left(0, \frac{2}{3}\right)\)
2. \(\left[0, \frac{2}{3}\right]\)
3. \(\left[\frac{-2}{3}, \frac{2}{3}\right]\)
4. None of these

Answer: 2. \(\left[0, \frac{2}{3}\right]\)

cos^-1 (3x- 1) is defined only when (3x-1) ∈ [-1, 1]

⇒  3x ∈ [0, 2] => x ∈ \(\left[0, \frac{2}{3}\right]\)

⇒ dom(f)= \(\left[0, \frac{2}{3}\right]\)

Question 31. Let f(x)= \(\sqrt{\cos x}\). Then, dom (f) = ?

1. \(\left[0, \frac{\pi}{2}\right]\)
2. \(\left[\frac{3 \pi}{2}, 2 \pi\right]\)
3. \(\left[0, \frac{\pi}{2}\right] \cup\left[\frac{3 \pi}{2}, 2 \pi\right]\)
4. None of These

Answer: 3. \(\left[0, \frac{\pi}{2}\right] \cup\left[\frac{3 \pi}{2}, 2 \pi\right]\)

f(x) is defined only when cos x > 0.

⇒ x liesin 1st or 4th quadrant.

⇒  \(=\left[0, \frac{\pi}{2}\right] \cup\left[\frac{3 \pi}{2}, 2 \pi\right]\)

Question 32. Let f(x) = \(\sqrt{\log \left(2 x-x^2\right)}\). then, dom (f) = ?

1. (0,2)
2. [1,2]
3. (-,1)
4. None of these

Answer: 4. None of these

f (x) is defined only when log (2x- x²) > 0

⇒  (2x- x²) >1 => (1 + x²– 2x) < 0

⇒  (1- x)² < 0

⇒  (1- x) = 0

⇒   x=1.

⇒ dom (f) = {1}.

Question 33. Let f(x) = x². Then, dom (/) and range (/) are respectively

1. R and R
2. R⁺ and R⁺
3. R and R⁺
4. R and R- {0}

Answer: 3. R and R⁺

f(x) = x² is clearly defined for each x ∈ R.

So, dom (f)=R. y= x² x=± \(x= \pm \sqrt{ } y .\)

When y < 0, there is no real value of x. So, y ≥ 0.

∴Range (f) = R.

Question 34. Let f(x) = x³. Then, dom (f) and range (f) are respectively.

1. R and R
2. R⁺ and R⁺
3. R and R⁺
4. R⁺ and R

Answer: 1. R and R

f (x) = x³ is defined for each x ∈ R.

So, dom (f)=R.

For each y∈ R,y^1/3 ∈ R and so x= is real.

∴ range (f) =R.

Question 35. Let f(x)= \(\log (1-x)+\sqrt{x^2-1}\).Then ,dom(f) = ?

1. (1,∞)
2. (-∞,-1)
3. [-1,1]
4. (0,1)

Answer: 2. (-∞,-1)

Let f (x) = g{x) + h(x), where g(x) = log (1- x) and h(x) = \(\sqrt{x^2-1}\)

g(x) is defined only whenl-x>0 => x<l.So, dom (g) = (∞, 1)

h(x) is defined only when x2-1 > 0 => x >1 or x <-1

∴ dom (h) = (-∞, -1]∪[1, ∞)

∴ dom (f) = dom (g)∩ dom(h) = (-∞, -1].

Question 36. Let \(=\frac{1}{\left(1-x^2\right)}\). Then ,range(f)= ?

1. [-∞,1)
2. [1,∞)
3. [-1,1]
4. None of these

Answer: 2. [0,1)

y =  \(\frac{1}{\left(1-x^2\right)}\)

x = \(\sqrt{1-\frac{1}{y}}\)

Clearly, x is not defined when y = 0 or 1\(-\frac{1}{y}\) < 0, i.e., y = 0 or y < 1.

∴ range (f) = [1, ∞).

Question 37. Let f(x)\(=\frac{x^2}{\left(1+x^2\right)}\)

1. [1,∞]
2. [0,1)
3. [-1,1]
4. (0,1)

Answer: 2. [0,1)

y = \(\frac{x^2}{\left(1+x^2\right)} \Rightarrow x=\sqrt{\frac{y}{1-y}}\)

Clearly, x is defined only when \(\frac{y}{(1-y)}\) and(1-y)≠ 0, i.e., when 0 ≤ y ≤ 1.

So, range (f) = [0, 1).

Question 38. The range of f(x) = \(x+\frac{1}{x}\) is

1. [-2,2]
2. [2,∞)
3. (-∞,-2)
4. None of these

Answer: 4. None of these

y\(=\frac{x^2+1}{x}\)

⇒ x²– xy + 1= 0

x= \(\frac{y \pm \sqrt{y^2-4}}{2}\)

Question 39. The range of f(x) = a^x, Where a> 0 is

1. ]-∞,0]
2. ]∞-,0)
3. [0,∞)
4. (0,∞)

Answer: 4. (0,∞)

Clearly, a^x> 0 whatever may be the value of x.

∴ Range (f) = (0, ∞)

Relations And Functions Exercise 1C Results At A Glance

1. sin^-1x = θ => x = sin θ

cos^-1x = θ => x = cos θ

tan^-1x = θ =» x = tan θ

2. Domain and range of inverse functions

 

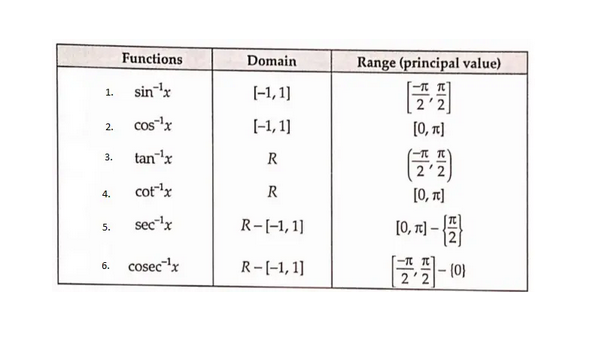
3. sin (sin^-1x) = x, if-1 ≤ x ≤ 1

cos (cos^-1x) = x, if-1 ≤ x ≤ 1

tan (tan^-1x) = x, if ∞ < x < ∞

4.

sin^-1(sin x) = x, if x ∈ \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)

cos ^-1(cos x) = x, if x ∈ [0, π]

tan^-1(tan x) = x, if x ∈ \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)

5.

sin^-1x = cosec^-1\(\left(\frac{1}{x}\right)\) , x≠0

cos^-1x = sec^-1\(\left(\frac{1}{x}\right)\) , x≠0

tan^-1x= cot^-1\(\left(\frac{1}{x}\right)\) , x≠0

cosec^-1x= sin^-1 \(\left(\frac{1}{x}\right)\),(x ≤ -1 or x ≥ 1)

sec^-1x= cos^-1\(\left(\frac{1}{x}\right)\), when (x ≤1 or x ≥ 1)

cot^-1x = tan^-1\(\left(\frac{1}{x}\right)\), when x > 0

6. sin^-1(-x) = -sin^-1x

cos^-1(-x) = 7i- cos^-1x

tan^-1(-x) = -tan^-1x

cot^-1(-x) =n- cot^-1x

sec^-1(-x) =n- sec^-1x

cosec^-1(-x) = -cosec^-1x

7. sin^-1-x + cos^-1-x =\(\frac{\pi}{2}\) -1 ≤ x ≤1

tan^-1-x + cot^-1-x = \(\frac{\pi}{2}\) , x ≠ 0

sec ^-1x + cosec ^-1x = \(\frac{\pi}{2}\),  (x ≤ -1 or x ≥ 1)

8.

1. For 0 <x< 1, we have

sin^-1-x=  \(\cos ^{-1} \sqrt{1-x^2}\)

= \(\tan ^{-1} \frac{x}{\sqrt{1-x^2}}=\cot ^{-1} \frac{\sqrt{1-x^2}}{x}\)

= \(\sec ^{-1} \frac{1}{\sqrt{1-x^2}}\)

= cosec^-1 \(\frac{1}{x}\)

2. Cos^-1-x= \(\sin ^{-1} \sqrt{1-x^2}\)

= \(\tan ^{-1} \frac{\sqrt{1-x^2}}{x}\)

= \(\cot ^{-1} \frac{x}{\sqrt{1-x^2}}\)

= \(\sec ^{-1} \frac{1}{x}\)

= cosec^-1 \(\frac{1}{\sqrt{1-x^2}}\)

3. For x>0, We Have

tan^-1x= \(\sin ^{-1} \frac{x}{\sqrt{1+x^2}}\)

= \(\cos ^{-1} \frac{1}{\sqrt{1+x^2}}=\cot ^{-1} \frac{1}{x}\)

= \(\sec ^{-1} \sqrt{1+x^2}\)

= cosec^-1 \(\frac{\sqrt{1+x^2}}{x}\)

9. 

2sin^-1x = sin^-1(\(2 x \sqrt{1-x^2}\)) = cos^-1 (1- 2x²)

2cos^-1x = cos^-1(2x²– 1) = sin ^-1\(\left(2 x \sqrt{1-x^2}\right.\))

2tan^-1x= tan^-1\(\left(\frac{2 x}{1-x^2}\right)\)  = cos^-1\(\left(\frac{1-x^2}{1+x^2}\right)\)

10.

3sin^-1x = sin^-1(3x- 4x³)

3cos^-1x = cos^-1(4x³– 3x)

3tan^-1x = tan^-1\(\left(\frac{3 x-x^3}{1-3 x^2}\right)\)

11.

When x > 0, y > 0 and xy < 1, we have

tan^-1x + tan y^-1 =  tan^-1\(\left(\frac{x+y}{1-x y}\right)\)

When x > 0, y > 0 and xy > 1, we have

tan^-1x + tan y^-1 = π + tan^-1\(\left(\frac{x+y}{1-x y}\right)\)

12.

sin^–1x + sin^–1y = sin^–1(\(x \sqrt{1-y^2}+y \sqrt{1-x^2}\))

sin^–1x – sin^–1y = sin^–1(\(x \sqrt{1-y^2}-y \sqrt{1-x^2}\))

cos^–1x + cos^–1y= cos^–1(\(x y-\sqrt{1-x^2} \cdot \sqrt{1-y^2}\))

cos^–1x – cos^–1y = cos^–1(\(x y+\sqrt{1-x^2} \cdot \sqrt{1-y^2}\))

cot ^–1x+ cot ^–1x= cot^–1\(\left(\frac{x y-1}{x+y}\right)\)

cot ^–1x- cot ^–1y = cot^–1\(\left(\frac{x y+1}{x-y}\right)\)

Relations And Functions Exercise 1C Multiple Choice Questions

Question 1. The principal value of \(\cos ^{-1}\left(\frac{\sqrt{3}}{2}\right)\)

1. \(\frac{\pi}{6}\frac{\pi}{6}\)
2. \(\frac{5 \pi}{6}\)
3. \(\frac{7 \pi}{6}\)
4. None of these

Answer: 1. \(\frac{\pi}{6}\frac{\pi}{6}\)

1. Let cos^-1\(\left(\frac{\sqrt{3}}{2}\right)\), where x ∈ [0, π].

Then, cos x=\(\frac{\sqrt{3}}{2}\)= cos\(\frac{\pi}{6}\)

⇒  x= \(\frac{\pi}{6}\)

Question 2. The principal value of cosec^-1(2) is

1. \(\frac{\pi}{3}\)
2. \(\frac{\pi}{6}\)
3. \(\frac{2 \pi}{3}\)
4. \(\frac{5 \pi}{6}\)

Answer: 2. \(\frac{\pi}{6}\)

Let cosec^-1(2) = x, where x ∈ \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)-{0}

Then, cosec x= 2cosec \(\frac{\pi}{6}\)

⇒ x= \(\frac{\pi}{6}\)

Question 3. The principal value of \(\cos ^{-1}\left(\frac{-1}{\sqrt{2}}\right)\)

1. \(\frac{-\pi}{4}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{3 \pi}{4}\)
4. \(\frac{5 \pi}{4}\)

Answer: 3. \(\frac{3 \pi}{4}\)

Let cos^-1 \(\left(\frac{-1}{\sqrt{2}}\right)\) = x, where x∈ [0,π]

Then,cos x = \(\frac{-1}{\sqrt{2}}\)

=  \(-\cos \frac{\pi}{4}=\cos \left(\pi-\frac{\pi}{4}\right)\)

= \(\cos \frac{3 \pi}{4} \Rightarrow x=\frac{3 \pi}{4}\)

Question 4. The principal value of \(\sin ^{-1}\left(\frac{-1}{2}\right)\) is

1. \(\frac{-\pi}{6}\)
2. \(\frac{5 \pi}{6}\)
3. \(\frac{7 \pi}{6}\)
4. None of these

Answer: 1. \(\frac{-\pi}{6}\)

Let sin^-1 \(\left(\frac{-1}{2}\right)\) = x where x∈\(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)

Then, sin x  = \(\frac1{-1}{2}\)

= \(-\sin \frac{\pi}{6}=\sin \left(\frac{-\pi}{6}\right)\)

⇒ x= \(frac{-\pi}{6}\)

Question 5. The principal value of \(\cos ^{-1}\left(\frac{-1}{2}\right)\) is

1. \(\frac{-\pi}{3}\)
2. \(\frac{2 \pi}{3}\)
3. \(\frac{4 \pi}{3}\)
4. \(\frac{\pi}{3}\)

Answer: 2. \(\frac{2 \pi}{3}\)

Let cos^-1 \(\frac{-1}{2}\) = x where x∈[0,π]

Then, cos x  = \(\frac{-1}{2}\)

= \(-\cos \frac{\pi}{3}\)

= \(\cos \left(\pi-\frac{\pi}{3}\right)=\cos \frac{2 \pi}{3}\)

x = \(\frac{2 \pi}{3}\)

Question 6. The principal value of tan \(\tan ^{-1}(-\sqrt{3})\)

1. \(\frac{2 \pi}{3}\)
2. \(\frac{4 \pi}{3}\)
3. \(\frac{-\pi}{3}\)
4. None of these

Answer: 3. \(\frac{-\pi}{3}\)

Let tan^-1\(-\sqrt{3}\)= x, where x ∈\(\left(\frac{-\pi}{2},\frac{\pi}{2}\right)\)

Then, tan x= \(-\sqrt{3}\)

= \(-\tan \frac{\pi}{3}=\tan \left(\frac{-\pi}{3}\right)\)

⇒ \(x=\frac{-\pi}{3}\)

Question 7. The principal value of cot^-1(-1) is

1. \(\frac{-\pi}{4}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{5 \pi}{4}\)
4. \(\frac{3 \pi}{4}\)

Answer: 4. \(\frac{3 \pi}{4}\)

Let co^-1(-1) = x, where x ∈ [0, π].

Then, cot x=-1

= \(-\cot \frac{\pi}{4}=\cot \left(\pi-\frac{\pi}{4}\right)\)

= \(\cot \frac{3 \pi}{4}\)

⇒ x= \(\frac{-\pi}{3}\)

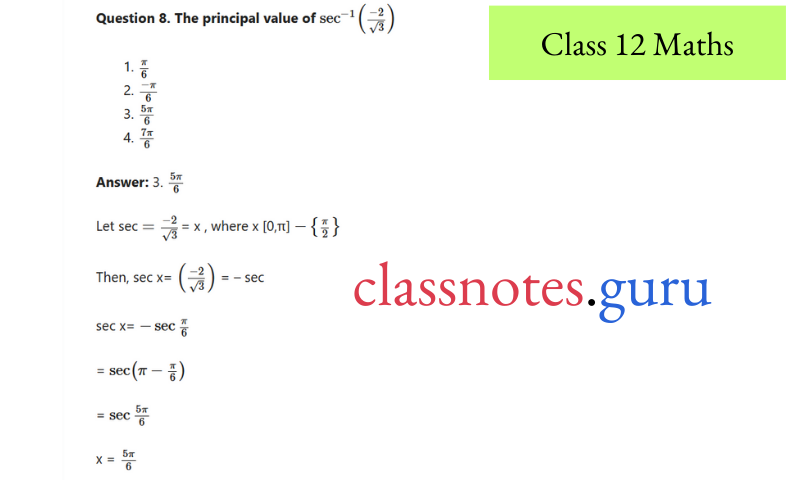
Question 9. The principal value of cosec^-1 \((-\sqrt{2})\) is

1. \(\frac{-\pi}{4}\)
2. \(\frac{3 \pi}{4}\)
3. \(\frac{5 \pi}{4}\)
4. None of these

Answer: 1. \(\frac{-\pi}{4}\)

Let cosec\((-\sqrt{2})\)= x, where x ∈\(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)– {0}

Then , cosec x \(-\sqrt{2}\)

= cosec \(\frac{-\pi}{4}\)

= cosec \(\frac{-\pi}{4}\)

x = \(\frac{-\pi}{4}\)

Question 10. The principal value of \(\cot ^{-1}(-\sqrt{3})\)

1. \(\frac{-\pi}{6}\)
2. \(\frac{-\pi}{6}\)
3. \(\frac{7 \pi}{6}\)
4. \(\frac{5 \pi}{6}\)

Answer: 4. \(\frac{5 \pi}{6}\)

Let cot\((-\sqrt{3})\)= x, where x [0,π]

Then , cot x \(-\sqrt{3}\)

= -cot \(\frac{\pi}{6}\)

\(=\cot \left(\pi-\frac{\pi}{6}\right)=\cot \frac{5 \pi}{6}\)

x= \(\frac{5 \pi}{6}\)

Question 11. The value of \(\sin ^{-1}\left(\sin \frac{2 \pi}{3}\right)\)

1. \(\frac{2 \pi}{3}\)
2. \(\frac{5 \pi}{3}\)
3. \(\frac{\pi}{3}\)
4. None of these

Answer: 3. \(\frac{\pi}{3}\)

Let sin^-1\(\left(\sin \frac{2 \pi}{3}\right)\) = x , where x ∈ \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)

Then,sin x = \(\sin \frac{2 \pi}{3}=\sin \left(\pi-\frac{\pi}{3}\right)=\sin \frac{\pi}{3}\)

x= \(\frac{\pi}{3}\)

Question 12. The value of \(\cos ^{-1}\left(\cos \frac{13 \pi}{6}\right)\)

1. \(\frac{13 \pi}{6}\)
2. \(\frac{7 \pi}{6}\)
3. \(\frac{5 \pi}{6}\)
4. \(\frac{\pi}{6}\)

Answer: 4. \(\frac{\pi}{6}\)

Let cos \(\left(\cos \frac{13 \pi}{6}\right)\)= x, where x [0,]

Then,cos x = \(\cos \frac{13 \pi}{6}=\cos \left(2 \pi+\frac{\pi}{6}\right)\)

x= \(\frac{\pi}{6}\)

Question 13. The value of \(\tan ^{-1}\left(\tan \frac{7 \pi}{6}\right)\)

1. \(\frac{7 \pi}{6}\)
2. \(\frac{5 \pi}{6}\)
3. \(\frac{\pi}{6}\)
4. None of these

Answer: 3. \(\frac{\pi}{6}\)

Let tan^-1\(\left(\tan \frac{7 \pi}{6}\right)\) = x,where x ∈ \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

Then, tan x = tan \(\left(\tan \frac{7 \pi}{6}\right)\)

= tan \(\left(\pi+\frac{\pi}{6}\right)=\tan \frac{\pi}{6}\)

x=  \(\frac{\pi}{6}\)

Question 14. The value of \(\cot ^{-1}\left(\cot \frac{5 \pi}{4}\right)\)

1. \(\frac{\pi}{4}\)
2. \(\frac{-\pi}{4}\)
3. \(\frac{3 \pi}{4}\)
4. None of these

Answer: 1. \(\frac{\pi}{4}\)

Let cos^-1\(\left(\cot \frac{5 \pi}{4}\right)\) = x, where x [0,π]

Then ,cot x = cot \(\frac{5 \pi}{4}\)

= \(\cot \frac{5 \pi}{4}=\cot \left(\pi+\frac{\pi}{4}\right)\)

x\(=\frac{\pi}{4}\)

Question 15. The value of \(\sec ^{-1}\left(\sec \frac{8 \pi}{5}\right)\)

1. \(\frac{2 \pi}{5}\)
2. \(\frac{3 \pi}{5}\)
3. \(\frac{8 \pi}{5}\)
4. None of these

Answer: 1. \(\frac{2 \pi}{5}\)

Let sec^-1\(\left(\sec \frac{8 \pi}{5}\right)\)= x where x∈[0,π]- \(\left\{\frac{\pi}{2}\right\}\)

Then, sec x \(\frac{8 \pi}{5}\)

= sec \(\sec \left(2 \pi-\frac{2 \pi}{5}\right)=\sec \frac{2 \pi}{5}\)

x = \(\frac{2 \pi}{5}\)

Question 16. The value of cosec^-1 (cosec\(\frac{4 \pi}{3}\)) is

1. \(\frac{\pi}{3}\)
2. \(\frac{-\pi}{3}\)
3. \(\frac{2 \pi}{3}\)
4. None of these

Answer: 2. \(\frac{-\pi}{3}\)

Let cosec^-1 \(\frac{4 \pi}{3}\)= x where x ∈ \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]-\{0\}\)

Then,cosec x= cosec \(\frac{4 \pi}{3}\)

= cosec\(\left(\pi+\frac{\pi}{3}\right)\)

=- cosec \(\frac{\pi}{3}\)

=cosec \(\frac{-\pi}{3}\)

x =\(\frac{-\pi}{3}\)

Question 17. The value of \(\tan ^{-1}\left(\tan \frac{3 \pi}{4}\right)\)

1. \(\frac{3 \pi}{4}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{-\pi}{4}\)
4. None of these

Answer: 3. \(\frac{-\pi}{4}\)

Let tan^-1\(\left(\tan \frac{3 \pi}{4}\right)\)= x, where x ∈ \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

Then,tan x= \(\tan \frac{3 \pi}{4}\)

= tan\(\left(\pi-\frac{\pi}{4}\right)=\tan \left(\frac{-\pi}{4}\right)\)

x = \(\frac{-\pi}{4}\)

Question 18. \(\frac{\pi}{3}-\sin ^{-1}\left(\frac{-1}{2}\right)\)

1. 0
2. \(\frac{2 \pi}{3}\)
3. \(\frac{\pi}{2}\)
4. π

Answer: 3. \(\frac{\pi}{2}\)

Let ,sin^-1 x= \(\left(\frac{-1}{2}\right)\)= x, where x ∈ \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)

Then , sin x = \(-\frac{1}{2}\)

-sin = \(\frac{\pi}{6}=\sin \left(\frac{-\pi}{6}\right)\)

x= \(\frac{-\pi}{6}\)

Given example = \(\frac{\pi}{3}-\left(\frac{-\pi}{6}\right)=\left(\frac{\pi}{3}+\frac{\pi}{6}\right)Question \)

= \(\frac{3 \pi}{6}=\frac{\pi}{2}\)

Question 19. The value of sin \(\left(\sin ^{-1} \frac{1}{2}+\cos ^{-1} \frac{1}{2}\right)\)

1. 0
2. 1
3. -1
4. None of these

Answer: 2. 1

⇒ \(\sin ^{-1} \frac{1}{2}+\cos ^{-1} \frac{1}{2}=\frac{\pi}{2}\)

⇒ \(\sin \left(\sin ^{-1} \frac{1}{2}+\cos ^{-1} \frac{1}{2}\right)=\sin \frac{\pi}{2}\)

= 1

20. If x ≠ 0, then cos (tan^-1x+ cot^-1x)

1. -1
2. 1
3. 0
4. None of these

Answer: 3. 0

⇒ \(\cos \left(\tan ^{-1}+\cot ^{-1} x\right)\)

= \(\cos \frac{\pi}{2}\)

= 0

Question 21. The value of sin \(\sin \left(\cos ^{-1} \frac{3}{5}\right)\)

1. \(\frac{2}{5}\)
2. \(\frac{4}{5}\)
3. \(\frac{-2}{5}\)
4. None of these

Answer: 2. \(\frac{4}{5}\)

cos^-1 x \(=\sin ^{-1} \sqrt{1-x^2}\)

= \(\cos ^{-1} \frac{3}{5}=\sin ^{-1} \sqrt{1-\frac{9}{25}}\)

= \(\sin ^{-1} \frac{4}{5}\)

∴  \(\sin \left(\cos ^{-1} \frac{3}{5}\right)=\sin \left(\sin ^{-1} \frac{4}{5}\right)=\frac{4}{5}\)

Question 22. \(\cos ^{-1}\left(\cos \frac{2 \pi}{3}\right)+\sin ^{-1}\left(\sin \frac{2 \pi}{3}\right)\)

1. \(\frac{4 \pi}{3}\)
2. \(\frac{\pi}{2}\)
3. \(\frac{3 \pi}{4}\)
4. π

Answer: 4. \(\frac{3 \pi}{4}\)

cos^-1 \(\left(\cos \frac{2 \pi}{3}\right)=\sin ^{-1}\left(\sin \frac{2 \pi}{3}\right)\)

= \(=\cos ^{-1}\left\{\cos \left(\pi-\frac{\pi}{3}\right)\right\}+\sin ^{-1}\left\{\sin \left(\pi-\frac{\pi}{3}\right)\right\}\)

= \(\cos ^{-1}\left(-\cos \frac{\pi}{3}\right)+\sin ^{-1}\left\{\sin \frac{\pi}{3}\right\}=\cos ^{-1}\left(\frac{-1}{2}\right)+\frac{\pi}{3}\)

= \(\left(\frac{2 \pi}{3}+\frac{\pi}{3}\right)=\pi\)

Question 23. \(\tan ^{-1}(\sqrt{3})-\sec ^{-1}(-2)\)

1. \(\frac{\pi}{3}\)
2. Question \(\frac{-\pi}{3}\)
3. \(\frac{5 \pi}{3}\)
4. None of these

Answer: 2. \(\frac{-\pi}{3}\)

⇒ \(\tan ^{-1} \sqrt{3}-\sec ^{-1}(-2)\)

= \(\tan ^{-1} \sqrt{3}-\left(\pi-\sec ^{-1} 2\right)\)

= \(\frac{\pi}{3}-\pi+\frac{\pi}{3}=\frac{-\pi}{3}\)

24. \(\cos ^{-1} \frac{1}{2}+2 \sin ^{-1} \frac{1}{2}\)

1. \(\frac{2 \pi}{3}\)
2. \(\frac{3 \pi}{2}\)
3. 2π
4. None of these

Answer: 1. \(\frac{2 \pi}{3}\)

= \(\cos ^{-1} \frac{1}{2}+2 \sin ^{-1} \frac{1}{2}\)

= \(\frac{\pi}{3}+\left(2 \times \frac{\pi}{6}\right)=\left(\frac{\pi}{3}+\frac{\pi}{3}\right)\)

= \(\frac{2 \pi}{3}\)

Question 25. \(\tan ^{-1} 1+\cos ^{-1}\left(\frac{-1}{2}\right)+\sin ^{-1}\left(\frac{-1}{2}\right)\)

1. π
2. \(\frac{2 \pi}{3}\frac{2 \pi}{3}\)
3. \(\frac{3 \pi}{4}\)
4. \(\frac{\pi}{2}\)

Answer: 3. \(\frac{3 \pi}{4}\)

⇒ \(\tan ^{-1} 1+\cos ^{-1}\left(\frac{-1}{2}\right)+\sin ^{-1}\left(\frac{-1}{2}\right)\)

= \(\frac{\pi}{4}+\left(\pi-\cos ^{-1} \frac{1}{2}\right)-\sin ^{-1} \frac{1}{2}\)

= \(\left(\frac{\pi}{4}+\pi-\frac{\pi}{3}-\frac{\pi}{6}\right)\)

= \(\frac{3 \pi}{4}\)

Question 26. tan[2 tan^-1\(\frac{1}{5}-\frac{\pi}{4}\)]= ?

1. \(\frac{7}{17}\)
2. \(\frac{-7}{17}\)
3. \(\frac{7}{12}\)
4. \(\frac{-7}{12}\)

Answer: 2. \(\frac{-7}{17}\)

Let 2\(\tan ^{-1} \frac{1}{5}=\theta\) .

Then \(\tan ^{-1} \frac{1}{5}=\frac{1}{2} \theta \Rightarrow \tan \frac{1}{2} \theta=\frac{1}{5}\)

∴ \(\tan \theta=\frac{2 \tan \frac{1}{2} \theta}{1-\tan ^2 \frac{1}{2} \theta}=\frac{\left(2 \times \frac{1}{5}\right)}{\left(1-\frac{1}{25}\right)}\)

= \(\left(\frac{2}{5} \times \frac{25}{24}\right)=\frac{5}{12}\)

∴ \(\tan \left[2 \tan ^{-1} \frac{1}{5}-\frac{\pi}{4}\right]=\tan \left(\theta-\frac{\pi}{4}\right)=\frac{\tan \theta-\tan \frac{\pi}{4}}{1+\tan \theta \cdot \tan \frac{\pi}{4}}\)

= \(\frac{\left(\frac{5}{12}-1\right)}{\left(1+\frac{5}{12} \times 1\right)}=\frac{\left(\frac{-7}{12}\right)}{\left(\frac{17}{12}\right)}=\frac{-7}{17}\)

Question 27. \(\tan \frac{1}{2}\left(\cos ^{-1} \frac{\sqrt{5}}{3}\right)\)= ?

1. \(\frac{(3-\sqrt{5})}{2}\)
2. \(\frac{(3+\sqrt{5})}{2}\)
3. \(\frac{(5-\sqrt{3})}{2}\)
4. \(\frac{(5+\sqrt{3})}{2}\)

Answer: 1. \(\frac{(3+\sqrt{5})}{2}\)

Let \(\cos ^{-1} \frac{\sqrt{5}}{3}=\theta\).Then,cos \(\cos \theta=\frac{\sqrt{5}}{3}\)

⇒ \(\tan \frac{1}{2}\left(\cos ^{-1} \frac{\sqrt{5}}{3}\right)=\tan \frac{1}{2} \theta=\frac{\sin (\theta / 2)}{\cos (\theta / 2)}\)

⇒ \(\sqrt{\frac{1-\cos \theta}{1+\cos \theta}}=\left\{\frac{\left(1-\frac{\sqrt{5}}{3}\right)}{\left(1+\frac{\sqrt{5}}{3}\right)}\right\}^{1 / 2}\)

= \(\left(\frac{3-\sqrt{5}}{3+\sqrt{5}}\right)^{1 / 2}=\left\{3-\frac{\sqrt{5}}{3+\sqrt{5}} \times \frac{3-\sqrt{5}}{3+\sqrt{5}}\right\}^{1 / 2}\)

= \(\frac{(3-\sqrt{5})}{2}\)

Question 28. \(\sin \left(\cos ^{-1} \frac{3}{5}\right)\)

1. \(\frac{3}{4}\)
2. \(\frac{4}{5}\)
3. \(\frac{3}{5}\)
4. None of these

Answer: 2. \(\frac{4}{5}\)

Let cos^-1 \(\frac{3}{5}\)= x ∈ where [0,π] .Then ,cos x= \(\frac{3}{5}\)

∴ Since x[0,π],sinx > 0

∴ Since x\(\sqrt{1-\frac{9}{25}}\)

= \(\sqrt{\frac{16}{25}}=\frac{4}{5}\)

⇒ \(\sin \left(\cos ^{-1} \frac{3}{5}\right)=\frac{4}{5}\)

Question 29. \(\cos \left(\tan ^{-1} \frac{3}{4}\right)\)

1. \(\frac{3}{5}\)
2. \(\frac{4}{5}\)
3. \(\frac{4}{9}\)
4. None of these

Answer: 2. \(\frac{4}{5}\)

Let \(\tan ^{-1} \frac{3}{4}\) = x where x ∈ \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

∴tan x= \(\frac{3}{4}\) and since x ∈ \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\),we have cos x>0

∴cos x = \(\frac{1}{\sec x}=\frac{1}{\sqrt{1+\tan ^2 x}}\)

= \(\frac{1}{\sqrt{1+\frac{9}{16}}}=\frac{4}{5}\)

= \(\cos \left(\tan ^{-1} \frac{3}{4}\right)\)

cos x=\(\frac{4}{5}\)

Question 30. \(\sin \left\{\frac{\pi}{3}-\sin ^{-1}\left(\frac{-1}{2}\right)\right\}\)

1. 1
2. 0
3. \(\frac{-1}{2}\)
4. None of these

Answer: 1. 1

⇒ \(\sin \left\{\frac{\pi}{3}-\sin ^{-3}\left(\frac{-1}{2}\right)\right\}=\sin \left\{\frac{\pi}{3}+\sin ^{-1} \frac{1}{2}\right\}\)

sin = \( \left(\frac{\pi}{3}+\frac{\pi}{6}\right)=\sin \frac{\pi}{2}\)

= 1

Question 31. \(\sin \left(\frac{1}{2} \cos ^{-1} \frac{4}{5}\right)\)

1. \(\frac{1}{\sqrt{5}}\)
2. \(\frac{2}{\sqrt{5}}\)
3. \(\frac{1}{\sqrt{10}}\)
4. \(\frac{2}{\sqrt{10}}\)

Answer: 3. \(\frac{1}{\sqrt{10}}\)

Let \(\cos ^{-1} \frac{4}{5}=\) = x where x∈ [0,π].

Then cos x= \(\frac{4}{5}\)

Since x∈ [0,π] ⇒ \(\frac{1}{2} x \in\left[0, \frac{\pi}{2}\right] \Rightarrow \sin \frac{1}{2} x>0\)

sin = \( \left(\frac{1}{2} \cos ^{-1} \frac{4}{5}\right)=\sin \frac{1}{2} x\)

= \(\sqrt{\frac{1-\cos x}{2}}=\sqrt{\frac{\left(1-\frac{4}{5}\right)}{2}}=\)

= \(\frac{1}{\sqrt{10}}\)

Question 32. \(\tan ^{-1}\left\{2 \cos \left(2 \sin ^{-1} \frac{1}{2}\right)\right\}\)

1. \(\frac{\pi}{3}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{3 \pi}{4}\)
4. \(\frac{2 \pi}{3}\)

Answer: 2. \(\frac{\pi}{4}\)

⇒ \(\tan ^{-1}\left\{2 \cos \left(2 \sin ^{-1} \frac{1}{2}\right)\right\}\)

= \(\tan ^{-1}\left\{2 \cos \left(2 \times \frac{\pi}{6}\right)\right\}\)

=  \(\tan ^{-1}\left\{2 \times \frac{1}{2}\right\}\)

tan^-1 1= \(\frac{\pi}{4}\)

Question 33. \(\text { If } \cot ^{-1}\left(\frac{-1}{5}\right)=x, \text { then } \sin x=?\)

1. \(\frac{1}{\sqrt{26}}\)
2. \(\frac{5}{\sqrt{26}}\)
3. \(\frac{1}{\sqrt{24}}\)
4. None of these

Answer: 2. \(\frac{5}{\sqrt{26}}\)

cot^-1\(\left(\frac{-1}{5}\right)\)

cot x= \(\left(\frac{-1}{5}\right)\),where x∈ (0,π)

sin x>0 in (0,π)

sin x= 1/ cosecx

= \(\frac{1}{\sqrt{1+\cot ^2 x}}\)

⇒\(\frac{1}{\sqrt{1+\frac{1}{25}}}=\frac{5}{\sqrt{26}}\)

Question 34. \(\sin ^{-1}\left(\frac{-1}{2}\right)+2 \cos ^{-1}\left(\frac{-\sqrt{3}}{2}\right)=?\)

1. \(\frac{\pi}{2}\)
2. π
3. \(\frac{3 \pi}{2}\)
4. None of these

Answer: 3. \(\frac{3 \pi}{2}\)

Range of \(\cos ^{-1} \text { is }\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

cos^-1= \(\left(\frac{-\sqrt{3}}{2}\right)\)

Question x = \(\frac{-\sqrt{3}}{2}\)

– cos = \(\frac{\pi}{6}=\cos \left(\pi-\frac{\pi}{6}\right)=\cos \frac{5 \pi}{6}\)

x= \(\frac{5 \pi}{6}\)

sin^-1= \(\left(\frac{-1}{2}\right)+2 \cos ^{-1}\left(\frac{-\sqrt{3}}{2}\right)\)=

= \(-\sin ^{-1} \frac{1}{2}+2 \times \frac{5 \pi}{6}\)

= \(-\frac{\pi}{6}+\frac{5 \pi}{3}=\frac{4 \pi}{6}\)

=\(\frac{3 \pi}{2}\)

35. \(\tan ^{-1}(-1)+\cos ^{-1}\left(\frac{-1}{\sqrt{ } 2}\right)=?\)

1. \(\frac{\pi}{2}\)
2. π
3. \(\frac{3 \pi}{2}\)
4. \(\frac{2 \pi}{2}\)

Answer: 1. \(\frac{\pi}{2}\)

Range of \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

⇒ \(\tan ^{-1}(-1)=x \Rightarrow \tan x=-1\)

= – \(\tan \frac{\pi}{4}=\tan \left(\frac{-\pi}{4}\right) \Rightarrow\)

= \(x\frac{-\pi}{4}\)

The range of cos^-1 is [0,π]

= \(\cos ^{-1}\left(\frac{-1}{\sqrt{2}}\right)\) = y

cos y = \(\frac{-1}{\sqrt{2}}\)

= \(-\cos \frac{\pi}{4}=\cos \left(\pi-\frac{\pi}{4}\right)=\cos \frac{3 \pi}{4}\)

y = \(\frac{3 \pi}{4}\)

Given example = \(-\frac{\pi}{4}+\frac{3 \pi}{4}=\frac{2 \pi}{4}=\frac{\pi}{2}\)

Question 36. \(\cot \left(\tan ^{-1} x+\cot ^{-1} x\right)=?\)

1. 1
2. \(\frac{1}{2}\)
3. 0
4. None of these

Answer: 3. 0

Given example = \(\cot \frac{\pi}{2}\)= 0

Question 37. \(\tan ^{-1} 1+\tan ^{-1} \frac{1}{3}=?\)

1. \(\tan ^{-1} \frac{4}{3}\)
2. \(\tan ^{-1} \frac{2}{3}\)
3. \(\tan ^{-1} 2\)
4. \(\tan ^{-1} 3\)

Answer: 3. \(\tan ^{-1} 2\)

⇒ \(\tan ^{-1} 1+\tan ^{-1} \frac{1}{3}\)

\(=\tan ^{-1}\left\{\frac{1+\frac{1}{3}}{1-\frac{1}{3}}\right\}\)

= \(\tan ^{-1} 2\)

Question 38. \(\tan ^{-1} \frac{1}{2}+\tan ^{-1} \frac{1}{3}=?\)

1. \(\frac{\pi}{3}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{\pi}{2}\)
4. \(\frac{2 \pi}{3}\)

Answer: 2. \(\frac{\pi}{4}\)

⇒ \(\tan ^{-1} \frac{1}{2}+\tan ^{-1} \frac{1}{3}\)

tan^-1\(\left\{\frac{\frac{1}{2}+\frac{1}{3}}{1-\frac{1}{6}}\right\}\)

tan^-1\(=\frac{\pi}{4}\)

Question 39. \(2 \tan ^{-1} \frac{1}{3}=?\)

1. \(\tan ^{-1} \frac{3}{2}\)
2. \(\tan ^{-1} \frac{3}{4}\)
3. \(\tan ^{-1} \frac{4}{3}\)
4. None of these

Answer: 2. \(\tan ^{-1} \frac{3}{4}\)

Use \(2 \tan ^{-1} x=\tan ^{-1}\left(\frac{2 x}{1-x^2}\right)\)

Question 40. \(\cos \left(2 \tan ^{-1} \frac{1}{2}\right)=?\)

1. \(\frac{3}{5}\)
2. \(\frac{4\frac{3}{5}[}{5}\)
3. \(\frac{7}{8}\)
4. [None of these

Answer: 1. \(\frac{3}{5}\)

Use \(2 \tan ^{-1} x=\cos ^{-1}\left(\frac{1-x^2}{1+x^2}\right)\)

Question 41. \(\sin \left[2 \sin ^{-1} \frac{4}{5}\right]\)

1. \(\frac{12}{25}\)
2. \(\frac{80}{89}\)
3. \(\frac{75}{128}\)
4. None of these

Answer: 2. \(\frac{80}{89}\)

Use \(2 \tan ^{-1} x=\sin ^{-1}\left(\frac{2 x}{1+x^2}\right)\)

Question 42. \(\sin \left[2 \sin ^{-1} \frac{4}{5}\right]\)

1. \(\frac{2}{25}\)
2. \(\frac{16}{25}\)
3. \(\frac{24}{25}\)
4. None of these

Answer: 3. \(\frac{16}{25}\)

Use \(2 \sin ^{-1} x=\sin ^{-1}\left[2 x \sqrt{1-x^2}\right]\)

Question 43. \(\text { If } \tan ^{-1} x=\frac{\pi}{4}-\tan ^{-1} \frac{1}{3} \text {, then } x=?\)

1. \(\frac{1}{2}\)
2. \(\frac{1}{4}\)
3. \(\frac{1}{6}\)
4. None of these

Answer: 1. \(\frac{1}{2}\)

\(\frac{\pi}{4}-\tan ^{-1} \frac{1}{3}=\tan ^{-1} 1-\tan ^1 \frac{1}{3}\) \(\tan ^{-1}\left\{\frac{\left(1-\frac{1}{3}\right)}{\left(1+\frac{1}{3}\right)}\right\}=\tan ^{-1} \frac{1}{2}\)

= \(\frac{1}{2}\)

Question 44. \(\text { If } \tan ^{-1}(1+x)+\tan ^{-1}(1-x)=\frac{\pi}{2} \text {, then } x=\text { ? }\)

1. 1
2. -1
3. 0
4. \(\frac{1}{2}\)

Answer: 3. 0

We know that \(\tan ^{-1} x+\cot ^{-1} x=\frac{\pi}{2}\)

= \(\tan ^{-1} x+\tan ^{-1} \frac{1}{x}=\frac{\pi}{2}\)

= \(\tan ^{-1}(1+x)+\tan ^{-1}(1-x)=\frac{\pi}{2}\)

(1-x)= \(\frac{1}{(1+x)}\)(1-x) = 1

x= 0

Question 45. \(\text { If } \sin ^{-1} x+\sin ^{-1} y=\frac{2 \pi}{3}, \text { then }\left(\cos ^{-1} x+\cos ^{-1} y\right)=?\)

1. \(\frac{\pi}{6}\)
2. \(\frac{\pi}{3}\)
3. \(\pi\)
4. \(\frac{2 \pi}{3}\)

Answer: 2. \(\frac{\pi}{3}\)

⇒ \(\sin ^{-1} x+\sin ^{-1} y=\frac{2 \pi}{3}\)

∴ \(\left(\frac{\pi}{2}-\cos ^{-1} x\right)+\left(\frac{\pi}{2}-\cos ^{-1} y\right)=\frac{2 \pi}{3}\)

⇒ \(\cos ^{-1} x+\cos ^{-1} y=\left(\pi-\frac{2 \pi}{3}\right)=\frac{\pi}{3}\)

Question 46. \(\left(\tan ^{-1} 2+\tan ^{-1} 3\right)=?\)

1. \(\frac{-\pi}{4}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{3 \pi}{4}\)
4. \(\pi\)

Answer: 3. \(\frac{3 \pi}{4}\)

( x = 2,y = 3) = xy>1

∴  \(\pi+\tan ^{-1}\left(\frac{2+3}{1-2 \times 3}\right)=\pi+\tan ^{-1}(-1)\)

= \(\pi-\tan (1)=\left(\pi-\frac{\pi}{4}\right)\)

= \(\frac{3 \pi}{4}\)

Question 47. \(\text { If } \tan ^{-1} x+\tan ^{-1} 3=\tan ^{-1} 8, \text { then } x=\text { ? }\)

1. \(\frac{1}{3}\)
2. \(\frac{1}{5}\)
3. 3
4. 5

Answer: 2. \(\frac{1}{5}\)

⇒ \(\tan ^{-1} x+\tan ^{-1} 3=\tan ^{-1} 8\)

= \(\frac{3+x}{1-3 x}\)= 8

3+x = 8-24x

x= \(\frac{1}{5}\)

Question 48. \(\text { If } \tan ^{-1} 3 x+\tan ^{-1} 2 x=\frac{\pi}{4} \text {, then } x=\text { ? }\)

1. \(\frac{1}{2} \text { or }-2\)
2. \(\frac{1}{3} \text { or }-3\)
3. \(\frac{1}{4} \text { or }-2\)
4. \(\frac{1}{6} \text { or }-1\)

Answer: 4. \(\frac{1}{6} \text { or }-1\)

⇒ \(\tan ^{-1}\left(\frac{3 x+2 x}{1-6 x^2}\right)=\frac{\pi}{4}\)

∴ \(\frac{5 x}{1-6 x^2}=\tan \frac{\pi}{4}=1\)

= \(\frac{5 x}{1-6 x^2}=\tan \frac{\pi}{4}\)= 1

⇒ 6x²+5x-1 = 0

(x+1)(6x-1)= 0

x= -1 or x= \(\frac{1}{6}\)

Question 49. \(\tan \left\{\cos ^{-1} \frac{4}{5}+\tan ^{-1} \frac{2}{3}\right\}=?\)

1. \(\frac{13}{6}\)
2. \(\frac{17}{6}\)
3. \(\frac{19}{6}\)
4. \(\frac{23}{6}\)

Answer: 2. \(\frac{17}{6}\)

cos^-1 x= \(\frac{\sqrt{1-x^2}}{x}\)

cos^-1\(\frac{4}{5}\) = tan^-1\(\frac{\sqrt{1-\frac{16}{25}}}{(4 / 5)}\)

tan^-1 = \(\frac{3}{4}\)

∴ \(\cos ^{-1} \frac{4}{5}+\tan ^{-1} \frac{2}{3}=\tan ^{-1} \frac{3}{4}+\tan ^{-1} \frac{2}{3}\)

tan^-1 = \(\frac{\left(\frac{3}{4}+\frac{2}{3}\right)}{\left(1-\frac{3}{4} \times \frac{2}{3}\right)}\)

tan^-1 = \(\frac{17}{6}\)

\(\cos ^{-1} \frac{4}{5}=\tan ^{-1} \frac{\sqrt{1-\frac{16}{25}}}{(4 / 5)}=\tan ^{-1} \frac{3}{4 \)

∴ Given example = \(\tan \left\{\tan ^{-1} \frac{17}{6}\right\}=\frac{17}{6}\)

Question 50. cot^-1 + cosec^-1\(\frac{\sqrt{41}}{4}\)

1. \(\frac{\pi}{6}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{\pi}{3}\)
4. \(\frac{3 \pi}{4}\)

Answer: 2. \(\frac{\pi}{4}\)

cosec x^-1 = \(\cot ^{-1} \sqrt{x^2-1}\)

cosec x^-1 = \(\frac{\sqrt{41}}{4}\)

cot^-1 = \(\frac{\sqrt{41}}{4}\) -1

cot^-1 = \(\frac{5}{4}\)

= \(\cot ^{-1} 9+\cot ^{-1} \frac{5}{4}\)

= \(\tan ^{-1} \frac{1}{9}+\tan ^{-1} \frac{4}{5}\)

Question 51. Range of sin^-1x is

1. \(\left[0, \frac{\pi}{2}\right]\)
2. [0, π]
3. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)
4. None of these

Answer: 3. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)

Question 52. The range of cos^-1x is

1. [0, π]
2. \(\left[0, \frac{\pi}{2}\right]\)
3. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)
4. None of these

 Answer: 1. [0, π]

Question 53. The range of tan^-1x is

1. \(\left(0, \frac{\pi}{2}\right)\)
2. \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)
3. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)
4. None of these

Answer: 2. \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)

Question 54. The range of sec^-1x is

1. \(\left[0, \frac{\pi}{2}\right]\)
2. [0, π]
3. \([0, \pi]-\left\{\frac{\pi}{2}\right\}\)
4. None of these

Answer: 3. \([0, \pi]-\left\{\frac{\pi}{2}\right\}\)

Question 55. Range of cosec^-1x is

1. \(\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)\)
2. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]\)
3. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]-\{0\}\)
4. None of these

Answer: 3. \(\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]-\{0\}\)

Question 56. Domain of cos^-1x is

1. [0,1]
2. [-1,1]
3. [-1,0]
4. None of these

Answer: 2. [-1,1]

Question 57. Domain of sec^-1 x is

1. [-1,1]
2. R- {0}
3. R- [-1,1]
4. R- {-1,1}

Answer: 3. R- [-1,1]

Binomial Distribution

Success And Failure In An Experiment: There are certain kinds of experiments that have two possible outcomes. One of these two outcomes is called a success, while the other is called a failure.

For example, in tossing a coin, we get either a head or a tail. If getting head is taken as a success then getting a tail is a failure.

Bernoulli’s Theorem Let there be n independent trials in an experiment and let the random variable X denote the number of successes in these trials.

Let the probability of getting a success in a single trial be p and that of getting a failure be q so that p + q = 1. Then,

P(X =r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}.\)

Proof: Let us denote a success by S and a failure by F.

Read and Learn More WBCHSE Solutions For Class 12 Maths

A number of ways of getting r successes in n trials = ⁿC_r.

∴ P(X=r) = \({ }^n C_r\) • {P(S) • P(S) …r times} x {P(F) • P(F)… (n – r) times}

= \({ }^n C_r\) (p • p • p …r times) x[q • q • q … (n-r) times]

= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}\)

Hence, P(X = r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}\)

Remark: We have

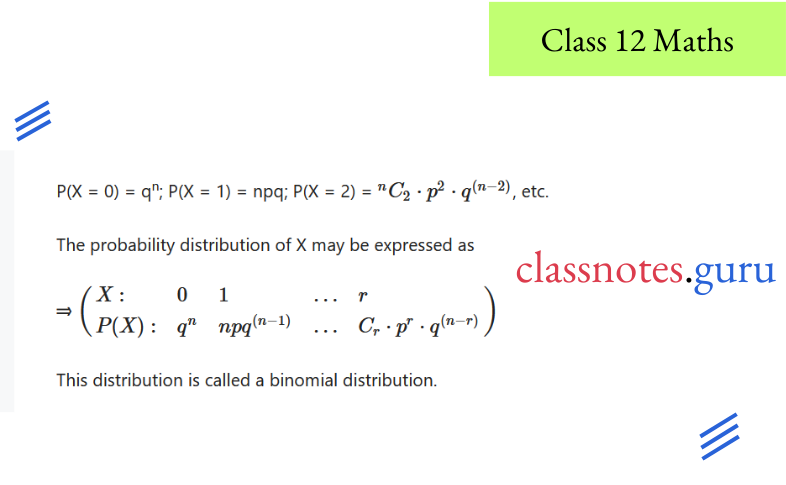Conditions for the Applicability of a Binomial Distribution

1. The experiment is performed for a finite and fixed number of trials.
2. Each trial must give either a success or a failure.
3. The probability of success in each trial is the same.

Binomial Distribution Solved Examples

Example 1. A coin is tossed 4 times. If X is the number of heads observed, find the probability distribution of X.
Solution:

When a coin is tossed, we have S = {H, T}.

P(getting a head) = 1/2, and

P(not getting a head) = (1-1/2) = 1/2

Let X be the random variable denoting the number of heads.

In 4 trials, we may get 0 or 1 or 2 or 3 or 4 heads.

So, X may assume the values 0,1, 2, 3, 4.

P(X = 0) = \({ }^4 C_0 \cdot\left(\frac{1}{2}\right)^0 \cdot\left(\frac{1}{2}\right)^{(4-0)}=\frac{1}{16}\)

P(X = 1) = \({ }^4 C_1 \cdot\left(\frac{1}{2}\right)^1 \cdot\left(\frac{1}{2}\right)^{(4-1)}=\frac{1}{4}\)

P(X = 2) = \({ }^4 C_2 \cdot\left(\frac{1}{2}\right)^2 \cdot\left(\frac{1}{2}\right)^{(4-2)}=\frac{3}{8}\)

P(X = 3) = \({ }^4 C_3 \cdot\left(\frac{1}{2}\right)^3 \cdot\left(\frac{1}{2}\right)^{(4-3)}=\frac{1}{4}\)

P(X = 4) = \({ }^4 C_4 \cdot\left(\frac{1}{2}\right)^4 \cdot\left(\frac{1}{2}\right)^{(4-4)}=\frac{1}{16}\)

Hence, the required probability distribution is given by

⇒ \(\left(\begin{array}{llllll}
X: & 0 & 1 & 2 & 3 & 4 \\
P(X): & \frac{1}{16} & \frac{1}{4} & \frac{3}{8} & \frac{1}{4} & \frac{1}{16}
\end{array}\right)\)

Example 2. Find the probability distribution of the number of suffixes in three tosses of a die.
Solution:

When a die is tossed, we have S = {1, 2, 3, 4, 5, 6}.

∴ P(getting a six) = 1/6 and P(not getting a six) = (1-1/6) = 5/6

Let X be the random variable denoting the number of sixes.

In 3 trials, the number of sixes may be 0 or 1 or 2 or 3.

So, X may assume the values 0, 1, 2, 3.

P(X=0) = \({ }^3 C_0 \cdot\left(\frac{1}{6}\right)^0 \cdot\left(\frac{5}{6}\right)^{(3-0)}=\frac{125}{216}\)

P(X=1 )= \({ }^3 C_1 \cdot\left(\frac{1}{6}\right)^1 \cdot\left(\frac{5}{6}\right)^{(3-1)}=\frac{25}{72}\)

P(X=2) = \({ }^3 C_2 \cdot\left(\frac{1}{6}\right)^2 \cdot\left(\frac{5}{6}\right)^{(3-2)}=\frac{5}{72}\)

P(X=3) = \({ }^3 C_3 \cdot\left(\frac{1}{6}\right)^3 \cdot\left(\frac{5}{6}\right)^{(3-3)}=\frac{1}{216}\)

The required probability distribution of X is given below:

⇒ \(\left(\begin{array}{lcccc}
X: & 0 & 1 & 2 & 3 \\
P(X): & \frac{125}{216} & \frac{25}{72} & \frac{5}{72} & \frac{1}{216}
\end{array}\right) .\)

Example 3. Find the probability distribution of the number of doublets in four throws of a pair of dice.
Solution:

When a pair of dice is thrown, there are 36 possible outcomes.

∴ n(S)= 36.

All possible doublets are (1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6).

P(getting a doublet) = \(\frac{6}{36}\) = \(\frac{1}{6}\), and

P(not getting a doublet) = (1-\(\frac{1}{6}\)) = \(\frac{5}{6}\)

Let X denote the number of doublets.

In 4 throws, we can have 0 or1 2 or 3, or 4 doublets

P(X=0) = \({ }^4 C_0 \cdot\left(\frac{1}{6}\right)^0 \cdot\left(\frac{5}{6}\right)^4=\frac{625}{1296}\)

P(X=1) = \({ }^4 C_1 \cdot\left(\frac{1}{6}\right)^1 \cdot\left(\frac{5}{6}\right)^3=\frac{125}{324}\)

P(X=2) = \({ }^4 C_2 \cdot\left(\frac{1}{6}\right)^2 \cdot\left(\frac{5}{6}\right)^2=\frac{25}{216}\)

P(X=3) = \({ }^4 C_3 \cdot\left(\frac{1}{6}\right)^3 \cdot\left(\frac{5}{6}\right)^1=\frac{5}{324}\)

P(X=4) = \({ }^4 C_4 \cdot\left(\frac{1}{6}\right)^4 \cdot\left(\frac{5}{6}\right)^0=\frac{1}{1296}\)

The required probability distribution is given below:

⇒ \(\left(\begin{array}{lccccc}
X: & 0 & 1 & 2 & 3 & 4 \\
P(X): & \frac{625}{1296} & \frac{125}{324} & \frac{25}{216} & \frac{5}{324} & \frac{1}{1296}
\end{array}\right) .\)

Example 4. An unbiased coin is tossed 6 times. Find, using the binomial distribution, the probability of getting at least 5 heads.
Solution:

In a single throw of a coin, we have S = {H, T}.

P(getting a head) = \(\frac{1}{2}\) and P(not getting a head) = (1-\(\frac{1}{2}\)) = \(\frac{1}{2}\)

∴ p = \(\frac{1}{2}\) and q = \(\frac{1}{2}\)

P(X=r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^6 C_r \cdot\left(\frac{1}{2}\right)^r \cdot\left(\frac{1}{2}\right)^{(6-r)}={ }^6 C_r \cdot\left(\frac{1}{2}\right)^6\)

∴ P(getting at least 5 heads) = P(X≥5)

= P(X = 5) + P(X = 6)

= \({ }^6 C_5 \cdot\left(\frac{1}{2}\right)^6+{ }^6 C_6 \cdot\left(\frac{1}{2}\right)^6=\left(\frac{3}{32}+\frac{1}{64}\right)=\frac{7}{64}\)

Hence, the required probability is \(\frac{7}{64}\)

Example 5. An unbiased coin is tossed 8 times. Find, by using binomial distribution, the probability of getting at least 3 heads.
Solution:

In a single throw of a coin, we have S = {H, T}.

P(getting a head) = \(\frac{1}{2}\) and P(not getting a head) = (1-\(\frac{1}{2}\)) = \(\frac{1}{2}\)

∴ p = \(\frac{1}{2}\) and \(\frac{1}{2}\)

P(X=r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^8 C_r \cdot\left(\frac{1}{2}\right)^r \cdot\left(\frac{1}{2}\right)^{(8-r)}={ }^8 C_r\left(\frac{1}{2}\right)^8\)

∴ P(getting at least 3 heads) = P(X≥3)

= 1 – [P(X = 0) + P(X = 1) + P(X = 2)]

= \(1-\left[{ }^8 C_0 \cdot\left(\frac{1}{2}\right)^8+{ }^8 C_1 \cdot\left(\frac{1}{2}\right)^8+{ }^8 C_2 \cdot\left(\frac{1}{2}\right)^8\right]\)

= \(1-\frac{1}{256} \cdot(1+8+28)=\left(1-\frac{37}{256}\right)=\frac{219}{256}\)

Hence, the required probability is \(\frac{219}{256}\).

Example 6. Six coins are tossed simultaneously. Find the probability of getting

1. 3 Heads
2. No head
3. At least one head
4. Not more than 3 heads.

Solution:

The experiment may be taken as throwing a single coin 6 times.

In a single throw of a coin, we have S = {H, T}.

P(getting a head) = \(\frac{1}{2}\) and P(not getting a head) = (1-\(\frac{1}{2}\)) = \(\frac{1}{2}\)

Let X be the random variable showing the number of heads.

P(X = r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^6 C_r \cdot\left(\frac{1}{2}\right)^r \cdot\left(\frac{1}{2}\right)^{6-r}={ }^6 C_r \cdot\left(\frac{1}{2}\right)^6\)

1. P(getting 3 heads) = P(X = 3) = \(={ }^6 C_3 \cdot\left(\frac{1}{2}\right)^6=\frac{5}{16}\)

2. P(getting no head) = P(X = 0) = \({ }^6 C_0 \cdot\left(\frac{1}{2}\right)^6=\frac{1}{64}\)

3. P(getting at least 1 head)

= \(1-P(X=0)=1-\left[{ }^6 C_0 \cdot\left(\frac{1}{2}\right)^6\right]\)

= \(\left(1-\frac{1}{64}\right)=\frac{63}{64}\)

4. P(getting not more than 3 heads) = P(no head or 1 head or 2 heads or 3 heads)

= P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3)

= \({ }^6 C_0 \cdot\left(\frac{1}{2}\right)^6+{ }^6 C_1 \cdot\left(\frac{1}{2}\right)^6+{ }^6 C_2 \cdot\left(\frac{1}{2}\right)^6+{ }^6 C_3 \cdot\left(\frac{1}{2}\right)^6\)

= \(\left(\frac{1}{2}\right)^6 \cdot(1+6+15+20)=\left(\frac{1}{64} \times 42\right)=\frac{21}{32}\)

Example 7. A die is thrown 5 times. If getting an odd number is a success, find the probability of getting at least 4 successes.
Solution:

When a die is thrown, we have S = {1,2,3,4,5,6}.

∴ P(getting an odd number) = \(\frac{3}{6}\) = \(\frac{1}{2}\)

∴ P(a success) = \(\frac{1}{2}\), and P(not a success) = (1-\(\frac{1}{2}\)) = \(\frac{1}{2}\)

Let X be the random variable showing the number of successes.

P(X=r) = \(={ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^5 C_r \cdot\left(\frac{1}{2}\right)^r \cdot\left(\frac{1}{2}\right)^{(5-r)}={ }^5 C_r \cdot\left(\frac{1}{2}\right)^5\)

P(at least 4 successes) = P(4 successes or 5 successes)

= P(X = 4) + P(X = 5)

= \({ }^5 C_4 \cdot\left(\frac{1}{2}\right)^5+{ }^5 C_5 \cdot\left(\frac{1}{2}\right)^5=\left(\frac{5}{32}+\frac{1}{32}\right)=\frac{6}{32}=\frac{3}{16}\)

Example 8. In 4 throws with a pair of dice, what is the probability of throwing doublets at least twice?
Solution:

In a single throw of a pair of dice, the number of all possible outcomes is 36.

All doublets are (1,1), (2,2), (3,3), (4,4), (5,5), (6,6).

P(getting a doublet) = \(\frac{6}{36}\) = \(\frac{1}{6}\) and,

P(not getting a doublet) = (1-\(\frac{1}{6}\)) = \(\frac{5}{6}\)

Let X be the random variable denoting the number of doublets.

Then, P(X = r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^4 C_r \cdot\left(\frac{1}{6}\right)^r \cdot\left(\frac{5}{6}\right)^{(4-r)} .\)

P(at least 2 doublets) = P(X = 2) + P(X = 3) + P(X = 4)

= \({ }^4 C_2 \cdot\left(\frac{1}{6}\right)^2 \cdot\left(\frac{5}{6}\right)^{(4-2)}+{ }^4 C_3 \cdot\left(\frac{1}{6}\right)^3 \cdot\left(\frac{5}{6}\right)^{(4-3)}+{ }^4 C_4 \cdot\left(\frac{1}{6}\right)^4 \cdot\left(\frac{5}{6}\right)^{(4-4)}\)

= \(6 \cdot\left(\frac{1}{6}\right)^2 \cdot\left(\frac{5}{6}\right)^2+4 \cdot\left(\frac{1}{6}\right)^3 \cdot\left(\frac{5}{6}\right)^1+\left(\frac{1}{6}\right)^4 \cdot\left(\frac{5}{6}\right)^0\)

= \(\left(\frac{25}{216}+\frac{5}{324}+\frac{1}{1296}\right)=\frac{171}{1296}\)

Example 9. The bulbs produced in a factory are supposed to contain 5% defective bulbs. What is the probability that a sample of 10 bulbs will contain no more than 2 defective bulbs?
Solution:

P(getting a defective bulb) = \(\frac{5}{100}\) = \(\frac{1}{20}\), and

P(getting a non-defective bulb) = (1-\(\frac{1}{20}\)) = \(\frac{19}{20}\), and

Then, p = \(\frac{1}{20}\) and q = \(\frac{19}{20}\)

Let X denote the number of defective bulbs.

P(X=r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}\)=\({ }^{10} C_r \cdot\left(\frac{1}{20}\right)^r \cdot\left(\frac{19}{20}\right)^{(10-r)}\)

P(getting not more than 2 defective bulbs)

= P(X = 0 or X = 1 or X = 2)

= P(X = 0) + P(X = 1) + P(X = 2)

= \({ }^{10} C_0 \cdot\left(\frac{1}{20}\right)^0 \cdot\left(\frac{19}{20}\right)^{10}+{ }^{10} C_1 \cdot\left(\frac{1}{20}\right)^1 \cdot\left(\frac{19}{20}\right)^9+{ }^{10} C_2 \cdot\left(\frac{1}{20}\right)^2 \cdot\left(\frac{19}{20}\right)^8\)

= \(\left(\frac{19}{20}\right)^{10}+\frac{1}{2} \cdot\left(\frac{19}{20}\right)^9+\frac{9}{80} \cdot\left(\frac{19}{20}\right)^8=\left(\frac{19}{20}\right)^8 \cdot\left(\frac{149}{100}\right)\)

Let A = \(\left(\frac{19}{20}\right)^8 \cdot\left(\frac{149}{100}\right) \cdot\) Then,

log A = 8(log 19 – log 20) + log 149 – log 100

= 8(1.2788 – 1.3010) + 2.1732 – 2 = -0.0044 = 1.9956.

∴ A = antilog (1.9956) = 0.99.

Hence, the required probability = \(\frac{99}{100}\)

Example 10. If on average, out of 10 ships, one gets drowned then what is the probability that out of 5 ships at least 4 reach the shore safely?
Solution:

Probability of a ship to reach the shore safely = \(\frac{9}{10}\)

Probability that a ship gets drowned = (1-\(\frac{9}{10}\)) = \(\frac{1}{10}\)

Let X be the random variable showing the number of ships reaching the shore safely.

∴ P(at least 4 reaching safely)

= P(4 reaching safely or 5 reaching safely)

= P(4 reaching safely) + P(5 reaching safely)

= \({ }^5 C_4 \cdot\left(\frac{9}{10}\right)^4 \cdot\left(\frac{1}{10}\right)^{(5-4)}+{ }^5 C_5 \cdot\left(\frac{9}{10}\right)^5 \cdot\left(\frac{1}{10}\right)^0\)

= \(\frac{1}{2} \cdot\left(\frac{9}{10}\right)^4+\left(\frac{9}{10}\right)^5=\left(\frac{9}{10}\right)^4\left(\frac{1}{2}+\frac{9}{10}\right)=\frac{7}{5} \cdot\left(\frac{9}{10}\right)^4\)

A = \(\frac{7}{5} \cdot\left(\frac{9}{10}\right)^4=\frac{7 \times(9)^4}{5 \times(10)^4}\)

⇒ logA = log7 + 4xlog9-log5-4xloglO = (0.8451 + 4 x 0.9542 – 0.6990- 4) = -0.0371 = 1.9629

⇒ A = antilog (1.9629) = 0.9181.

Hence, the required probability is 0.9181.

Mean and Variance of a Binomial Distribution

Mean: If a random variable X assumes the values x₁, x₂,… ,x_n with probabilities p₁, p₂, ……., p_n respectively then the mean of X is defined by

∴ \(\mu=\sum_{i=1}^n x_i p_i .\)

To Find the Mean of a Binomial Distribution

For the binomial distribution P(X = r) = P(r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}\), where r = 0,1,2,… ,n the mean, p, is given by

⇒ \(\mu =\sum_{r=0}^n r \cdot p(r)=\sum_{r=0}^n r \cdot{ }^n C_r \cdot p^r \cdot q^{(n-r)}\)

= \({ }^n C_1 \cdot p \cdot q^{n-1}+2 \cdot{ }^n C_2 \cdot p^2 \cdot q^{(n-2)}+\ldots+n \cdot{ }^n C_n \cdot p^n q^0\)

= \(1 \cdot n p \cdot q^{n-1}+n(n-1) \cdot p^2 \cdot q^{(n-2)}+\ldots+n p^n\)

= \(n p \cdot\left[{ }^{(n-1)} C_0 \cdot p^0 \cdot q^{(n-1)}+{ }^{(n-1)} C_1 \cdot p^1 \cdot q^{(n-2)}+\ldots+{ }^{(n-1)} C_{(n-1)} \cdot p^{n-1} \cdot q^0\right]\)

= \((n p) \cdot(q+p)^{n-1}=(n p)\) [because q+p=1]

Hence, the mean is given by μ= np.

The variance =σ² is given by

⇒ \(\sigma^2=\sum_{r=0}^n r^2 \cdot p(r)-(\text { mean })^2\)

= \(\sum_{r=0}^n r^2 \cdot{ }^n C_r \cdot p^r q^{(n-r)}-(n p)^2\) [because mean=np]

= \(\sum_{r=0}^n\{r+r(r-1)\} \cdot{ }^n C_r p^r q^{(n-r)}-(n p)^2\)

= \(\sum_{r=0}^n r \cdot{ }^n C_r p^r q^{(n-r)}+\sum_{r=0}^n r(r-1) \cdot{ }^n C_r \cdot p^r \cdot q^{(n-r)}-(n p)^2\)

= \(n p+\sum_{r=2}^n r(r-1) \cdot \frac{n(n-1)}{r(r-1)} \cdot{ }^{(n-2)} C_{(r-2)} \cdot p^2 \cdot p^{(r-2)} \cdot q^{(n-r)}-(n p)^2\)

= \(n p+n(n-1) \cdot p^2 \cdot\left(\sum_{r=2}^n \sum_{r=0}^n r \cdot{ }^n C_r \cdot p^r \cdot q^{(n-r)}=\text { mean }=n p\right]\)

= \(n p+n(n-1) p^2(q+p)^{(n-2)}-n^2 p^2\)

= \(n p+n(n-1) p^2-n^2 p^2\) [because q+p=1]

= \(n p-n p^2=n p(1-p)=n p q\).

Hence, variance = npq.

∴ standard deviation = √npq.

Recurrence Relation for a Binomial Distribution

We have

P(r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)} \text { and } P(r+1)={ }^n C_{(r+1)} \cdot p^{(r+1)} \cdot q^{n-r-1} \text {. }\)

∴ \(\frac{P(r+1)}{P(r)}=\frac{{ }^n C_{(r+1)} \cdot p^{(r+1)} \cdot q^{n-r-1}}{{ }^n C_r \cdot p^r \cdot q^{(n-r)}}\)

= \(\frac{(n !)}{(r+1) ! \cdot(n-r-1) !} \cdot \frac{(r) ! \cdot(n-r) !}{(n) !} \cdot \frac{p}{q}=\frac{(n-r)}{(r+1)} \cdot \frac{p}{q}\)

∴ P(r+1) = \(\frac{(n-r)}{(r+1)} \cdot \frac{p}{q} \cdot P(r)\)

Example 11. If X follows a binomial distribution with mean 3 and variance (3/2), find

1. P(X≥1)
2. P(X≤5).

Solution:

We know that mean = np and variance = npq.

∴ np = 3 and npq = \(\frac{3}{2}\)

⇒ 3q = \(\frac{3}{2}\)

⇒ q = \(\frac{1}{2}\)

Now, np = 3 and p = \(\frac{1}{2}\)

⇒ n X \(\frac{1}{2}\) = 3

⇒ n = 6.

So, the binomial distribution is given by

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^6 C_r \cdot\left(\frac{1}{2}\right)^r \cdot\left(\frac{1}{2}\right)^{(6-r)}={ }^6 C_r\left(\frac{1}{2}\right)^6 \cdot\)

1. P(X≥1) =1-P(X=0)

= \(1-{ }^6 C_0 \cdot\left(\frac{1}{2}\right)^6=\left(1-\frac{1}{64}\right)=\frac{63}{64}\)

2. P(X≤5) =1-P(X= 0)

= \(1-{ }^6 C_6\left(\frac{1}{2}\right)^6=\left(1-\frac{1}{64}\right)=\frac{63}{64}\)

Example 12. If X follows a binomial distribution with mean 4 and variance 2, find P(X≥5).
Solution:

We know that mean = np and variance = npq.

∴ np = 4 and npq = 2.

Now, np = 4 and npq= 2

⇒ 4q = 2 ⇒ q = \(\frac{1}{2}\)

Now,np = 4 and p = \(\frac{1}{2}\)

⇒ \(\frac{1}{2}\) n = 4 ⇒ n = 8.

So, the binomial distribution is given by

P(X= r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^8 C_r \cdot\left(\frac{1}{2}\right)^r \cdot\left(\frac{1}{2}\right)^{(8-r)}={ }^8 C_r \cdot\left(\frac{1}{2}\right)^8\)

∴ P(X > 5) = P(X = 5) + P(X = 6) + P(X = 7) + P(X= 8)

= \({ }^8 C_5 \cdot\left(\frac{1}{2}\right)^8+{ }^8 C_6 \cdot\left(\frac{1}{2}\right)^8+{ }^8 C_7 \cdot\left(\frac{1}{2}\right)^8+{ }^8 C_8 \cdot\left(\frac{1}{2}\right)^8\)

= \(\left[{ }^8 C_3+{ }^8 C_2+{ }^8 C_1+1\right]\left(\frac{1}{2}\right)^8\)

= \((56+28+8+1) \cdot \frac{1}{256}=\frac{93}{256}\)

Example 13. Find the binomial distribution for which the mean and variance are 12 and 3 respectively.
Solution:

Let X be a binomial variate for which mean = 12 and variance = 3.

Then, np =12 and npq = 3

⇔ 12xq = 3 ⇒ q = \(\frac{1}{4}\)

∴ p = (1-q) = (1-\(\frac{1}{4}\)) = \(\frac{3}{4}\)

And, np =12 ⇔ n = \(\frac{12}{p}\) = 12 x \(\frac{4}{3}\) = 16.

Thus, n =16, p = \(\frac{3}{4}\) and q = \(\frac{1}{4}\)

Hence, the binomial distribution is given by

P(X=r) = \({ }^{16} C_r \cdot\left(\frac{3}{4}\right)^r \cdot\left(\frac{1}{4}\right)^{(16-r)}\) where r = 0, 1, 2, 3, …,15.

Example 14. If the sum of the mean and variance of a binomial distribution for 5 trials is 1.8, find the distribution.
Solution:

We know that

mean = np and variance = npq.

It is being given that n = 5 and mean + variance = 1.8.

∴ np + npq = 1.8, where n = 5 +

⇔ 5p + 5pq = 1.8

⇔ p + p(1 -p) = 0.36 [q = (1-p)]

⇔ p²-2p + 0.36 = 0

⇔100p² – 200p + 36 = 0

⇔ 25p² – 50p + 9 = 0

⇔ 25p² -45p -5p + 9 = 0

⇔ 5p(5p – 9) – (5p – 9) = 0

⇔ (5p – 9)(5p -1) = 0 1

⇔ p = \(\frac{1}{5}\) = 0.2 [p cannot exceed 1].

Thus, n = 5, p = 0.2, and q = (1-p) = (1- 0.2) = 0.8.

Let X denote the binomial variate. Then, the required distribution is

P(X = r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^5 C_r \cdot(0.2)^r \cdot(0.8)^{(5-r)} \text {, }\) where r = 0,1,2,3,4,5.

Example 15. The sum and the product of the mean and variance of a binomial distribution are 24 and 128 respectively. Find the distribution.
Solution:

We have np + npq – 24 and np x npq = 128

⇔ (np)( 1 + q) = 24 and n²p² x q = 128

⇔ n²p² = \(\frac{576}{(1+q)^2}\) and n²p² x q =128

⇔ \(\frac{576}{(1+q)^2}=\frac{128}{q}\)

⇔ 2(1 + q² + 2q) = 9q

⇔ 2q²-5q + 2 = 0

⇔ (2q – 1)(q – 2) = 0

⇔ q = \(\frac{1}{2}\) ⇔ q = 2

∴ p = (1-q) = (1-\(\frac{1}{2}\)) = \(\frac{1}{2}\)

Now, np(1+q) = 24

⇔ n x \(\frac{1}{2}\)(1+\(\frac{1}{2}\)) = 24

⇔ n = 32

Hence, the requied probability distribution is given by P(X=r) = \({ }^{32} C_r \cdot\left(\frac{1}{2}\right)^{32}\)

Example 16. In a binomial distribution, prove that mean > variance.
Solution:

Let X be a binomial variate with parameters n and p.

Then, mean = np and variance = npq.

∴ (mean)- (variance) = (np- npq) = np(1 -q) = np²> 0 [(1- q) = p and np²>0 as n∈N]

⇒ [(mean)- (variance)] > 0

⇒ mean > variance.

Hence, mean > variance.

Example 17. A die is tossed thrice. Getting an even number is considered a success. What is the variance of the binomial distribution?
Solution:

Here, n = 3.

Let p = probability of getting an even number in a single throw

⇒ p = \(\frac{3}{6}\) = \(\frac{1}{2}\)

⇒ q = (1-p) = (1-\(\frac{1}{2}\)) = \(\frac{1}{2}\)

∴ variance = npq = (3x\(\frac{1}{2}\)x\(\frac{1}{2}\)0 = \(\frac{3}{4}\)

Example 18. A die is rolled 20 times. Getting a number greater than 4 is a success. Find the mean and variance of the number of successes.
Solution:

In a single throw of a die, we have

p = probability of getting a number greater than 4 = \(\frac{2}{6}\) = \(\frac{1}{3}\)

∴ q = (1-p) = (1-\(\frac{1}{3}\)) = \(\frac{2}{3}\)

Also, n = 20 (given).

∴ mean = np =(20x\(\frac{1}{3}\))= 6.67, and

variance = npq =(20x\(\frac{1}{3}\)x\(\frac{2}{3}\))= 4.44.

Example 19. A die is tossed 180 times. Find the expected number (μ) of times the face with the number 5 will appear. Also, find the standard deviation (σ), and variance (σ²).
Solution:

In a single throw of a die, S = {1, 2, 3, 4, 5 6}.

p = (probability of getting the number 5) = \(\frac{1}{6}\)

∴ q = (1-p) = (1-\(\frac{1}{6}\)) = \(\frac{5}{6}\)

Thus, n = 180, p = \(\frac{1}{6}\) and q = \(\frac{5}{6}\)

∴ μ = np = (180x\(\frac{1}{6}\))=30.

Variance =  σ² = npq = (l80x\(\frac{1}{6}\)x\(\frac{5}{6}\)) = 25

Standard deviation = σ = √25 = 5

Binomial Distribution Exercise

Question 1. A coin is tossed 6 times. Find the probability of getting at least 3 heads.
Solution: 21/32

Hint: In a single toss, P(H) 1/2 and P(not H) = 1/2

⊂ p = 1/2, q = 1/2 and n = 6.

Let X show the number of heads. Then,

P(X= r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^6 C_r\left(\frac{1}{2}\right)^6 .\)

Required probability = P(X= 3) +P(X= 4) +P(X= 5) +P(X= 6).

Question 2. A coin is tossed 5 times. What is the probability that a head appears an even number of times?
Solution: 1/2

Hint: Here,p = 1/2, q = 1/2 and n = 5

Let X show the number of heads. Then,

P(X=r) = \({ }^n C_r \cdot p^r \cdot q^{(n-n)}={ }^5 C_r\left(\frac{1}{2}\right)^5\).

Required probability=P(X= 0) + P(X= 2) + P(X= 4)

Question 3. 7 coins are tossed simultaneously. What is the probability that a tail appears an odd number of times?
Solution: 1/2

Hint: 7 coins being tossed simultaneously is the same as one coin being tossed 7 times.

∴ p = 1/2, q = 1/2 and n = 7.

Let X show the number of tails. Then,

P(X= r) = \({ }^n C_r \cdot p^r \cdot q^{n-r}={ }^7 C_r \cdot\left(\frac{1}{2}\right)^7\)

Required probability=P(X= 1) +P(X= 3) + P(X= 5) + P(X= 7).

Question 4. A coin is tossed 6 times. Find the probability of getting

1. Exactly 4 heads
2. At least 1 head
3. At most 4 heads.

Solution:

1. 15/64
2. 63/64
3. 57/64

Hint: P(a head) = 1/2 and P(not a head) = 1/2

∴ p = 1/2, q=2 and n = 6.

∴ P(X =r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^6 C_r \cdot\left(\frac{1}{2}\right)^6 .\)

1. P(exactly 4 heads) = \({ }^6 C_4 \cdot\left(\frac{1}{2}\right)^6\)

2. P(at least1 head) =1- P(no head)

=1- P(0 head) = \(1-P(0 \text { head })=\left[1-{ }^6 C_0 \cdot\left(\frac{1}{2}\right)^6\right]\)

3. P(at the most 4 heads) = P(4 or less than 4 heads)

= 1-P[5 heads or 6 heads] = \(1-\left[\left({ }^6 C_5+{ }^6 C_6\right)\left(\frac{1}{2}\right)^6\right] .\)

Question 5. 10 coins are tossed simultaneously. Find the probability of getting

1. Exactly 3 heads
2. Not more than 4 heads
3. At least 4 heads.

Solution:

1. 15/128
2. 193/512
3. 53/64

Hint: 10 coins being tossed simultaneously is the same as one coin being tossed 10 times.

P(X=r) = \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^{10} C_r \cdot\left(\frac{1}{2}\right)^{10}\)

1. P(exactly 3 heads) = \({ }^{10} C_3 \cdot\left(\frac{1}{2}\right)^{10} .\)

2. P(not more than 4 heads)

= P(X ≤ 4)

= P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4)

= \(\left({ }^{10} C_0+{ }^{10} C_1+{ }^{10} C_2+{ }^{10} C_3+{ }^{10} C_4\right)\left(\frac{1}{2}\right)^{10}\)

3. P(at least 4 heads)

= P(4 heads or 5 heads or … or 10 heads)

= 1- P(0 head or 1 head or 2 heads or 3 heads)

= 1- [P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3)]

= \(1-\left({ }^{10} C_0+{ }^{10} C_1+{ }^{10} C_2+{ }^{10} C_3\right)\left(\frac{1}{2}\right)^{10}\)

Question 6. A die is thrown 6 times. If getting an even number is a success, find the probability of getting

1. Exactly 5 successes
2. At least 5 successes
3. At most 5 successes.

Solution:

1. 3/32
2. 7/64
3. 63/64

Hint: p = \(\frac{3}{6}=\frac{1}{2}, q=\left(1-\frac{1}{2}\right)=\frac{1}{2} \text { and } n=6 . \)

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{n-r}={ }^6 C_5\left(\frac{1}{2}\right)^6\)

1. P(exactly 5 successes) = \({ }^n C_r \cdot p^r \cdot q^{n-r}={ }^6 C_5\left(\frac{1}{2}\right)^6\)

2. P(at least 5 successes) = P[(5 successes) or (6 successes)]

\(\left({ }^6 C_5+{ }^6 C_6\right)\left(\frac{1}{2}\right)^6\)

3. P(at most 5 successes) = P[0 or 1 or 2 or 3 or 5 successes]

= 1- P(6 successes) = \(\left[1-{ }^6 C_6 \cdot\left(\frac{1}{2}\right)^6\right]\)

Question 7. A die is thrown 4 times. “Getting a 1 or a 6” is considered a success. Find the probability of getting

1. Exactly 3 successes
2. At least 2 successes
3. At most 2 successes.

Solution:

1. 8/81
2. 11/27
3. 8/9

Hint: p= \(\frac{2}{6}=\frac{1}{3}, q=\left(1-\frac{1}{3}\right)=\frac{2}{3}\) and n=4.

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^4 C_r \cdot\left(\frac{1}{3}\right)^r \cdot\left(\frac{2}{3}\right)^{(4-r)}\).

1. P(exactly 3 successes)= \({ }^4 C_3 \cdot\left(\frac{1}{3}\right)^3 \cdot\left(\frac{2}{3}\right)^1\)

2. P(at least 2 successes) = [P(X = 2) or P(X = 3) or P(X = 4)] =1-[P(X = 0) + P(X=1)].

3. P(at most 2 successes) = P[(X = 0) or (X = l) or (X = 2)].

Question 8. Find the probability of a 4 turning up at least once in two tosses of a fair die.
Solution: 11/36

Hint: p= \(\frac{1}{6}, q=\left(1-\frac{1}{6}\right)=\frac{5}{6}\) and n=2.

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-n)}={ }^2 C_r \cdot\left(\frac{1}{6}\right)^r \cdot\left(\frac{5}{6}\right)^{(2-r)}\).

P[at least one 4] = P(X=1 or X=2) = P(X =1) + P(X = 2)

Question 9. A pair of dice is thrown 4 times. If “getting a doublet’ is considered a success, find the probability of getting 2 successes.
Solution: 25/216

Hint: p= \(\frac{6}{36}=\frac{1}{6}, q=\left(1-\frac{1}{6}\right)=\frac{5}{6} \text { and } n=4 . \)

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^4 C_r \cdot\left(\frac{1}{6}\right)^r \cdot\left(\frac{5}{6}\right)^{(4-r)}\)

∴ P(X=2)= \({ }^4 C_2 \cdot\left(\frac{1}{6}\right)^2 \cdot\left(\frac{5}{6}\right)^2\).

Question 10. A pair of dice is thrown 7 times. If ‘getting a total of 7’ is considered a success, find the probability of getting

1. No success
2. Exactly 6 successes
3. At least 6 successes
4. At most 6 successes.

Solution:

1. (5/6)²
2. 35/6⁷
3. 1/6⁵
4. (1-1/6⁷)

Hint: Let E={(1,6),(2,5),(3,4),(4,3),(5,2),(6,1)}.

∴ p= \(\frac{6}{36}=\frac{1}{6}, q=\left(1-\frac{1}{6}\right)=\frac{5}{6}\), n=7

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^7 C_r \cdot\left(\frac{1}{6}\right)^r \cdot\left(\frac{5}{6}\right)^{(7-r)}\)

1. P(X=0)= \({ }^7 C_0 \cdot\left(\frac{5}{6}\right)^7\).

2. P(X=6)= \({ }^7 C_6 \cdot\left(\frac{1}{6}\right)^6 \cdot\left(\frac{5}{6}\right)\).

3. P(at least 6 successes)=P(6 successes or 7 successes)

= P(X=6)+P(X=7)

= \({ }^7 C_6 \cdot\left(\frac{1}{6}\right)^6 \cdot\left(\frac{5}{6}\right)+{ }^7 C_7 \cdot\left(\frac{1}{6}\right)^7\)

4. P(at most 6 successes)= P(X \≤ 6)

=[1-P(X=7)]= \(1-{ }^7 C_7 \cdot\left(\frac{1}{6}\right)^7\)

Question 11. There are 6% defective items in a large bulk of items. Find the probability that a sample of 8 items will include not more than one defective item.
Solution: (47/50)⁷x(71/50)

Hint: p= \(\frac{6}{100}=\frac{3}{50}, q=\left(1-\frac{3}{50}\right)=\frac{47}{50} \text { and } n=8 \text {. }\)

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^8 C_r \cdot\left(\frac{3}{50}\right)^r \cdot\left(\frac{47}{50}\right)^{(8-r)}\)

Required probability = P(0 defective or 1 defective)

=P(X=0)+P(X=1) = \({ }^8 C_0 \cdot\left(\frac{47}{50}\right)^8+{ }^8 C_1 \cdot\left(\frac{3}{50}\right)\left(\frac{47}{50}\right)^7 . \)

Question 12. In a box containing 60 bulbs, 6 are defective. What is the probability that out of a sample of 5 bulbs

1. None is defective,
2. Exactly 2 are defective?

Solution:

1. (9/10)⁵
2. 729/10000

Hint: p = \(\frac{6}{60}=\frac{1}{10}, q=\left(1-\frac{1}{10}\right)=\frac{9}{10}\) and n=5

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{n-r}={ }^5 C_r \cdot\left(\frac{1}{10}\right)^r \cdot\left(\frac{9}{10}\right)^{(5-r)}\)

1. P(none is defective)= \(P(X=0)={ }^5 C_0 \cdot\left(\frac{9}{10}\right)^5\).

2. P(exactly 2 are defective)= \(P(X=2)={ }^5 C_2 \cdot\left(\frac{1}{10}\right)^2 \cdot\left(\frac{9}{10}\right)^3\).

Question 13. The probability that a bulb produced by a factory will fuse after 6 months of use is 0.05. Find the probability that out of 5 such bulbs

1. None will fuse after 6 months of use
2. At least one will fuse after 6 months of use
3. Not more than one will fuse after 6 months of use.

Solution:

1. (19/20)⁵
2. 1-(19/20)⁵
3. (19/20)⁴x(6/5)

Hint: p= \(\frac{1}{20}, q=\left(1-\frac{1}{20}\right)=\frac{19}{20}\) and n=5.

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-n)}={ }^5 C_r \cdot\left(\frac{1}{20}\right)^r \cdot\left(\frac{19}{20}\right)^{(5-r)}\)

1. P(X=0)= \({ }^5 C_0 \cdot\left(\frac{19}{20}\right)^5\).

2. \(P(X≥1)=1-P(X<1)=1-P(X=0)\)

= \(1-{ }^5 C_0 \cdot\left(\frac{19}{20}\right)^5\) \text

3. \(P(X \leq 1)=P(X=0)+P(X=1)\)

= \({ }^5 C_0 \cdot\left(\frac{19}{20}\right)^5+{ }^5 C_1 \cdot\left(\frac{1}{20}\right)\left(\frac{19}{20}\right)^4\)

Question 14. In the items produced by a factory, there are 10% defective items. A sample of 6 items is randomly chosen. Find the probability that this sample contains

1. Exactly 2 defective items,
2. Not more than 2 defective items,
3. At least 3 defective items.

Solution:

1. 3/20 x (9/10)⁴
2. 3/2 x (19/20)⁴
3. 1-[3/2x(19/20)⁴]

Hint: p= \(\frac{10}{100}\)=\(\frac{1}{10}\), q= \(\left(1-\frac{1}{10}\right)\)=\(\frac{9}{10}\) and n=6

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^6 C_r \cdot\left(\frac{1}{10}\right)^r \cdot\left(\frac{9}{10}\right)^{(6-n)}\)

1. P(X=2)= \({ }^6 C_2 \cdot\left(\frac{1}{10}\right)^2 \cdot\left(\frac{9}{10}\right)^4\).

2. \(P(X \leq 2)={ }^6 C_0 \cdot\left(\frac{9}{10}\right)^6+{ }^6 C_1 \cdot \frac{1}{10} \times\left(\frac{9}{10}\right)^5+{ }^6 C_2 \cdot \frac{1}{100} \times\left(\frac{9}{10}\right)^4\).

3. P(X≥3)=1-P(X<3)=1-P(X≤2)

Question 15. Assume that on average one telephone number out of 15, called between 3 p.m. and 4 p.m. on weekdays, will be busy. What is the probability that if six randomly selected telephone numbers are called, at least 3 of them will be busy?
Solution: 1-(14/15)⁴ .(59/45)

Hint: p= \(\frac{1}{15}, q=\left(1-\frac{1}{15}\right)=\frac{14}{15} \text { and } n=6\)

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-n}={ }^6 C_r \cdot\left(\frac{1}{15}\right)^r \cdot\left(\frac{14}{15}\right)^{(6-n)} \text {. }\)

P(X≥3)=1-P(X<3)=1-[P(X=0)+P(X=1)+P(X=2)]

= \(1-\left[{ }^6 C_0 \cdot\left(\frac{14}{15}\right)^6+{ }^6 C_1 \cdot\left(\frac{1}{15}\right) \cdot\left(\frac{14}{15}\right)^5+{ }^6 C_2 \cdot\left(\frac{1}{15}\right)^2 \cdot\left(\frac{14}{15}\right)^4\right]\)

= \(1-\left(\frac{14}{15}\right)^4\left(\frac{59}{45}\right)\)

Question 16. Three cars participate in a race. The probability that any one of them has an accident is 0.1. Find the probability that all the cars reach the finishing line without any accident.
Solution: 729/1000

Hint: p= \(\frac{1}{10}, q=\left(1-\frac{1}{10}\right)=\frac{9}{10} \text { and } n=3 \)

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-n)}={ }^3 C_r \cdot\left(\frac{1}{10}\right)^r \cdot\left(\frac{9}{10}\right)^{\beta-n}\)

P(X=0)= \({ }^3 C_0 \cdot\left(\frac{9}{10}\right)^3\)

Question 17. Past records show that 80% of the operations performed by a certain doctor were successful. If he performs 4 operations in a day, what is the probability that at least 3 operations will be successful?
Solution: 512/625

Hint: p= \(\frac{80}{100}=\frac{4}{5}, q=\left(1-\frac{4}{5}\right)=\frac{1}{5} \text { and } n=4 \text {. }\)

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-n)}={ }^4 C_r \cdot\left(\frac{4}{5}\right)^r \cdot\left(\frac{1}{5}\right)^{(4-n)} \text {. }\)

P(X≥3)=P(X=3)+P(X=4)

= \(\left[{ }^4 C_3 \cdot\left(\frac{4}{5}\right)^3 \cdot\left(\frac{1}{5}\right)+{ }^4 C_4 \cdot\left(\frac{4}{5}\right)^4\right]\)

Question 18. The probability of a man hitting a target is (1/4). If he fires 7 times, what is the probability of his hitting the target at least twice?
Solution: 4547/8192

Hint: p=\(\frac{1}{4}, q=\left(1-\frac{1}{4}\right)=\frac{3}{4}\) and n=7

P(X=r)=\({ }^n C_r \cdot p^r \cdot q^{(n-n}={ }^7 C_r \cdot\left(\frac{1}{4}\right)^r \cdot\left(\frac{3}{4}\right)^{(n-n)}\)

P(X≥2)=1-P(X<2) =1-P[(X = 0) or (X=1)] =1-[P(X = 0) + P(X=1)].

Question 19. In a hurdles race, a player has to cross 10 hurdles. The probability that he will clear each hurdle is (5/6). What is the probability that he will knock down fewer than 2 hurdles?
Solution: \(\frac{5^{10}}{\left(2 \times 6^9\right)}\)

Hint: p=P(knocking down 1 hurdle)= \(\left(1-\frac{5}{6}\right)=\frac{1}{6}\), q=\(\frac{5}{6}\) and n=10.

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^{10} C_r \cdot\left(\frac{1}{6}\right)^r \cdot\left(\frac{5}{6}\right)^{(10-r)}\)

P(X<2)=P(X=0)+P(X=1)

= \(\left[{ }^{10} C_0 \cdot\left(\frac{5}{6}\right)^{10}+{ }^{10} C_1 \cdot\left(\frac{1}{6}\right)^1 \cdot\left(\frac{5}{9}\right)^9\right]\)

Question 20. A man can hit a bird, once in 3 shots. On this assumption he fires 3 shots. What is the chance that at least one bird is hit?
Solution: 19/27

Hint: p = \(\frac{1}{3}\), q= \(\left(1-\frac{1}{3}\right)=\frac{2}{3}\) and n=3.

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^3 C_r \cdot\left(\frac{1}{3}\right)^r \cdot\left(\frac{2}{3}\right)^{(3-r)} \cdot\)

⇒ \(P(X \geq 1) =P(X=1)+P(X=2)+P(X=3)\)

= \(\left[{ }^3 C_1 \times \frac{1}{3} \times\left(\frac{2}{3}\right)^2\right]+\left[{ }^3 C_2 \times\left(\frac{1}{3}\right)^2 \times \frac{2}{3}\right]+\left[{ }^3 C_3 \times\left(\frac{1}{3}\right)^3\right]\)

= \(\left(\frac{4}{9}+\frac{2}{9}+\frac{1}{9}\right)=\frac{7}{9}\)

Question 21. If the probability that a man aged 60 will live to be 70 is 0.65, what is the probability that out of 10 men, now 60, at least 8 will live to be 70?
Solution: 0.2615

Hint: \(P(X \geq 8)=P(X=8)+P(X=9)+P(X=10)\)

= \(\left[{ }^{10} C_8 \cdot\left(\frac{13}{20}\right)^8 \cdot\left(\frac{7}{20}\right)^2+{ }^{10} C_9 \cdot\left(\frac{13}{20}\right)^9 \cdot\left(\frac{7}{20}\right)+{ }^{10} C_{10} \cdot\left(\frac{13}{20}\right)^{10}\right]\)

= \(\left[{ }^{10} C_2 \cdot\left(\frac{13}{20}\right)^8 \cdot \frac{49}{400}+{ }^{10} C_1 \cdot\left(\frac{13}{20}\right)^9 \cdot \frac{7}{20}+\left(\frac{13}{20}\right)^{10}\right] \text {. }\)

∴ P=\(\left(\frac{13}{20}\right)^8 \cdot\left[\frac{441}{80}+\frac{91}{40}+\frac{169}{400}\right]=\left[\left(\frac{13}{20}\right)^8 \times \frac{821}{100}\right] \text {. }\)

⇒ \(\log P=[8(\log 13-\log 20)+\log 821-\log 100]\)

=[-1.4968+2.9143-2]=-0.5825 = \(\overline{1}\).4175

⇒ P= antilog \((\overline{1} .4175)\)

Question 22. A bag contains 5 white, 7 red, and 8 black balls. If four balls are drawn one by one with replacement, what is the probability that

1. None is white,
2. All are white,
3. At least one is white.

Solution:

1. 81/256
2. 1/256
3. 175/256

Hint:

P(white)=\(\frac{5}{20}=\frac{1}{4}, P(\text { non-white })=\frac{3}{4}\)

∴ p= \(\frac{1}{4}, q=\frac{3}{4} \text { and } n=4\)

P(X=r)=\({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^4 C_r \cdot\left(\frac{1}{4}\right)^r \cdot\left(\frac{3}{4}\right)^{(4-r)}\)

1. P(X=0)= \({ }^4 C_0 \cdot\left(\frac{3}{4}\right)^4\).

2. P(X=4)=\({ }^4 C_4 \cdot\left(\frac{1}{4}\right)^4\).

3. \(P(X \geq 1)=1-P(X<1)=1-P(X=0)\).

Question 23. A policeman fires 6 bullets at a burglar. The probability that the burglar will be hit by a bullet is 0.6. What is the probability that the burglar is still unhurt?
Solution: 0.004096

Hint: p=\(\frac{6}{10}=\frac{3}{5}, q=\left(1-\frac{3}{5}\right)=\frac{2}{5}\) and n=6.

P(X=r)= \({ }^n C_r \cdot p^r \cdot q^{(n-r)}={ }^6 C_r \cdot\left(\frac{3}{5}\right)^r \cdot\left(\frac{2}{5}\right)^{(6-r)}\)

∴ P(X=0)= \({ }^6 C_0 \cdot\left(\frac{2}{5}\right)^6\)

Question 24. A die is tossed thrice. A success is 1 or 6 on a toss. Find the mean and variance of successes.
Solution: μ = 1, σ² = 2/3

Hint: p= \(\frac{2}{6}=\frac{1}{3}, q=\left(1-\frac{1}{3}\right)=\frac{2}{3} \text { and } n=3\)

∴ μ= np and σ²= npq

Question 25. A die is thrown 100 times. Getting an even number is considered a success. Find the mean and variance of sucess.
Solution: μ = 50, σ² = 25

Question 26. Determine the binomial distribution whose mean is 9 and variance is 6.
Solution: \({ }^{27} C_r \cdot\left(\frac{1}{3}\right)^r \cdot\left(\frac{2}{3}\right)^{(27-r)}, \text { where } r=0,1,2,3, \ldots, 27\)

Question 27. Find the binomial distribution whose mean is 5 and variance is 2.5.
Solution: \({ }^{10} C_r \cdot\left(\frac{1}{2}\right)^r \cdot\left(\frac{1}{2}\right)^{(10-r)}, 0 \leq r \leq 10\)

Question 28. The mean and variance of a binomial distribution are 4 and (4/3) respectively. Find P(X≥1).
Solution: 728/729

Hint: \(\left(n p=4 \text { and } n p q=\frac{4}{3}\right) \Rightarrow q=\frac{1}{3}\)

∴ p=(1-q)=\(\left(1-\frac{1}{3}\right)=\frac{2}{3}\)

and \(n=\frac{4}{p}=\left(4 \times \frac{3}{2}\right)=6 \)

∴ P(X=r)=\({ }^n C_r \cdot p^r \cdot q^{(n-r)}\)

= \(P(X \geq 1)=1-P(X=0) \cdot\left(\frac{2}{3}\right)^r \cdot\left(\frac{1}{3}\right)^{(6-r)}\)

= \(1-{ }^6 C_0 \cdot\left(\frac{2}{3}\right)^0 \cdot\left(\frac{1}{3}\right)^6\)

= \(\left(1-\frac{1}{3^6}\right)=\frac{728}{729}\)

Question 29. For a binomial distribution, the mean is 6 and the standard deviation is √2 ‘. Find the probability of getting 5 successes.
Solution: \({ }^9 C_5 \cdot\left(\frac{2}{3}\right)^5 \cdot\left(\frac{1}{3}\right)^4\)

Hint: np = 6 and npq = 2

⇒ q = \(\frac{1}{3}\) and p = \(\frac{2}{3}\)

⇒ \(\left(n \times \frac{2}{3}\right)=6 \Rightarrow n=\left(6 \times \frac{3}{2}\right)=9\)

∴ \(P(5 \text { successes })={ }^9 C_5 \cdot\left(\frac{2}{3}\right)^5 \cdot\left(\frac{1}{3}\right)^4\)

Question 30. In a binomial distribution, the sum and the product of the mean and the variance are (25/3) and (50/3) respectively. Find the distribution.
Solution: \({ }^{15} C_r \cdot\left(\frac{1}{3}\right)^r \cdot\left(\frac{2}{3}\right)^{(15-r)}\)

Question 31. Obtain the binomial distribution whose mean is 10 and the standard deviation is 2√2.
Solution: \({ }^{50} C_r \cdot\left(\frac{1}{5}\right)^r \cdot\left(\frac{4}{5}\right)^{(50-r)}, 0 \leq r \leq 50\)

Question 32. Bring out the fallacy, if any, in the following statement: ‘The mean of a binomial distribution is 6 and its variance is 9’.
Solution: The probability of getting a failure (i.e., q) cannot be greater than1

Hint: np = 6 and npq = 9

⇒ q = \(\frac{n p q}{n p}=\frac{9}{6}=\frac{3}{2}\)

But, q cannot be greater than 1.

 

 

 

 

 

Probability Distribution

Random Variable Let S be the sample space associated with a given random experiment.

A real-valued function X which assigns a unique real number X(w) to each w ∈ S, is called a random variable. A random variable that can assume only a finite number of values is called a discrete random variable.

Example: Suppose that a coin is tossed twice.

Then, sample space S = {TT, HT, TH, HH}

Consider a real-valued function X on S, defined by X: S → R: X(w) = number of heads in w, for all w ∈ S.

Then, X is a random variable such that X(TT) = 0, X(HT) = 1, X(TH) = 1 and X(HH) = 2.

Clearly, range (X) = {0,1, 2}.

Read and Learn More WBCHSE Solutions For Class 12 Maths

Probability Distribution of a Random Variable: A description giving the values of a random variable along with the corresponding probabilities is called the probability distribution of the random variable.

If a random variable X takes the values x₁,x₂, …,x_n with respective probabilities p₁, p₂,……,p_n then the probability distribution of X is given by

 

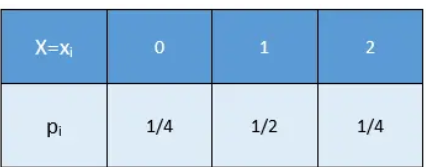
Remark: The above probability distribution of X is defined only when

1. each p_i ≥0
2. \(\sum_{i=1}^n p_i=1\)

Mean and Variance of Random Variables

 

Let a random variable X assume values x₁, x₂,… ,x_n with probabilities p₁, p₂,…..,p_n respectively such that each p_{i ≥} 0 and \(\sum_{i=1}^n p_i=1 .\). Then mean of X, denoted by μ [or expected value of X, denoted by E(X)], is defined as

∴ \(\mu=E(X)=\sum_{i=1}^n x_i p_i\)

And, the variance, denoted by σ², is defined as \(\sigma^2=\left(\Sigma x_i^2 p_i-\mu^2\right)\)

Standard deviation, σ is given by \(\sigma=\sqrt{\text { variance }}.\)

Probability Distribution Solved Examples

Example 1. Find the mean, variance, and standard deviation of the number of tails in two tosses of a coin.
Solution:

 

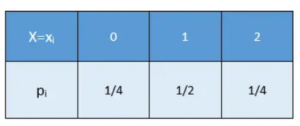
∴ mean, \(\mu =\Sigma x_i p_i=\left(0 \times \frac{1}{4}\right)+\left(1 \times \frac{1}{2}\right)+\left(2 \times \frac{1}{4}\right)=1\)

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2\)

= \(\left[\left(0 \times \frac{1}{4}\right)+\left(1 \times \frac{1}{2}\right)+\left(4 \times \frac{1}{4}\right)\right]-1^2\) = \(\frac{1}{2}\) .

Standard deviation, \(\sigma=\frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}}=\frac{\sqrt{2}}{2}=\frac{1.414}{2}=0.707\).

Example 2. Find the mean, variance, and standard deviation of the number of heads when three coins are tossed.
Solution:

Here, S = [TTT, TTH, THT, HTT, THH, HFH, HHT, HHH).

∴ n(S) = 8.

So, every single outcome has a probability 1/8

Let X = number of heads in tossing three coins.

The number of heads may be 0,1,2, or 3.

So, the possible values of X are 0,1,2,3.

P(X = 0) = P(getting no head) = P(TTT) = \(\frac{1}{8}\)

P(X = 1) = P(getting 1 head) = P(TTH or THT or HTT) = \(\frac{3}{8}\)

P(X = 2) = P( getting 2 heads) = P(THH, HTH, HHT) = \(\frac{3}{8}\)

P(X = 3) = P(getting 3 heads) = P(HHH) = \(\frac{1}{8}\)

Thus, we have the following probability distribution:

 

 

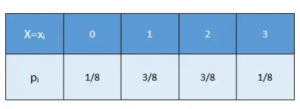
∴ mean \(\mu=\Sigma x_i p_i=\left(0 \times \frac{1}{8}\right)+\left(1 \times \frac{3}{8}\right)+\left(2 \times \frac{3}{8}\right)+\left(3 \times \frac{1}{8}\right)=\frac{3}{2}\)

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2\)

= \(\left[\left(0 \times \frac{1}{8}\right)+\left(1 \times \frac{3}{8}\right)+\left(4 \times \frac{3}{8}\right)+\left(9 \times \frac{1}{8}\right)-\frac{9}{4}\right]=\frac{3}{4}\).

Standard deviation, \(\sigma=\frac{\sqrt{3}}{2}\).

Example 3. A die is tossed once. If the random variable X is defined as X = 1, if the die results in an even number, X = 0, if the die results in an odd number then find the mean and variance of X.
Solution:

In tossing a die once, the sample space is given by S = {1,2,3,4,5,6}.

∴ P(getting an even number) = \(\frac{3}{6}\) = \(\frac{1}{2}\)

P(getting an odd number) = \(\frac{3}{6}\) = \(\frac{1}{2}\)

As given, X takes the value 0 or 1.

P(X = 0) = P(getting an odd number) = \(\frac{1}{2}\)

P(X = 1) = P(getting an even number) = \(\frac{1}{2}\)

Thus, the probability distribution of X is given by

 

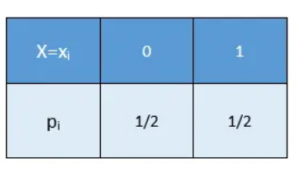
∴ mean, \(\mu=\Sigma x_i p_i=\left(0 \times \frac{1}{2}\right)+\left(1 \times \frac{1}{2}\right)=\frac{1}{2}\)

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2\)

= \(\left(0 \times \frac{1}{2}\right)+\left(1 \times \frac{1}{2}\right)-\left(\frac{1}{2}\right)^2=\left(\frac{1}{2}-\frac{1}{4}\right)=\frac{1}{4}\)

Example 4. Find the mean, variance, and standard deviation of the number of sixes in two tosses of a die.
Solution:

In a single toss, we have a probability of getting a six = \(\frac{1}{6}\), and

Probability of getting a non-six = (1-\(\frac{1}{6}\)) = \(\frac{5}{6}\)

Let X denote the number of sixes in two tosses.

Then, clearly X can assume the value 0,1, or 2.

P(X = 0) = P[(non-six in the 1st draw) and (non-six in the 2nd draw)]

= P(non-six in the 1st draw) x P(non-six in the 2nd draw)

= (\(\frac{5}{6}\)x\(\frac{5}{6}\) = \(\frac{25}{36}\)

P(X=1)= P[(six in the 1st draw and non-six in the 2nd draw) or (non-six in the 1st draw and six in the 2nd draw)]

= P(six in the 1st draw and non-six in the 2nd draw) + P(non-six in the 1st draw and six in the 2nd draw)

= \(\left(\frac{1}{6} \times \frac{5}{6}\right)+\left(\frac{5}{6} \times \frac{1}{6}\right)=\left(\frac{5}{36}+\frac{5}{36}\right)=\frac{10}{36}=\frac{5}{18}\)

P(X = 2) = P[six in the 1st draw and six in the 2nd draw]

= P(six in the 1st draw) x P(six in the 2nd draw)

= \(\left(\frac{1}{6} \times \frac{1}{6}\right)=\frac{1}{36}\)

Hence, the probability distribution is given by

 

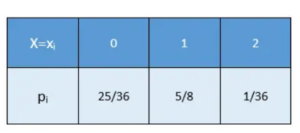
∴ mean, \(\mu=\Sigma x_i p_i=\left(0 \times \frac{25}{36}\right)+\left(1 \times \frac{5}{18}\right)+\left(2 \times \frac{1}{36}\right)=\frac{6}{18}=\frac{1}{3}\).

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2\)

= \(\left[\left(0 \times \frac{25}{36}\right)+\left(1 \times \frac{5}{18}\right)+\left(4 \times \frac{1}{36}\right)-\frac{1}{9}\right]=\frac{5}{18}\).

Standard deviation, \(\sigma=\sqrt{\frac{5}{18}}=\frac{1}{3} \cdot \sqrt{\frac{5}{2}}\).

Example 5. Two cards are drawn successively with replacement from a well-shuffled pack of 52 cards. Find the mean and variance of the number of kings.
Solution:

Let X be the random variable. Then,

X = number of kings obtained in two draws.

Clearly, X can assume the value 0,1 or 2.

P(drawing a king) = \(\frac{4}{52}\) = \(\frac{1}{13}\)

P(not drawing a king) = (1-\(\frac{1}{13}\)) = \(\frac{12}{13}\)

P(X = 0) = P(not a king in the 1st draw and not a king in the 2nd draw)

= \(\left(\frac{12}{13} \times \frac{12}{13}\right)=\frac{144}{169}\).

P(X = 1) = P(a king in the 1st draw and not a king in the 2nd draw)

or P(not a king in the 1st draw and a king in the 2nd
draw)

= \(\left(\frac{1}{13} \times \frac{12}{13}+\frac{12}{13} \times \frac{1}{13}\right)=\frac{24}{169}\)

P(X = 2) = P(a king in the 1st draw and a king in the 2nd draw)

= \(\left(\frac{1}{13} \times \frac{1}{13}\right)=\frac{1}{169}\)

Hence, the probability distribution is given by

 

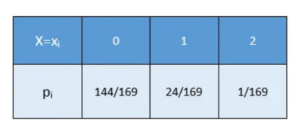
∴ mean, \(\mu=\Sigma x_i p_i=\left(0 \times \frac{144}{169}\right)+\left(1 \times \frac{24}{169}\right)+\left(2 \times \frac{1}{169}\right)=\frac{2}{13} \text {. }\)

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2\)

= \(\left[\left(0 \times \frac{144}{169}\right)+\left(1 \times \frac{24}{169}\right)+\left(4 \times \frac{1}{169}\right)-\frac{4}{169}\right]=\frac{24}{169}\)

Example 6. Two cards are drawn simultaneously (or successively without replacement) from a well-shuffled pack of 52 cards. Find the mean and variance of the number of aces.
Solution:

Let X be the random variable.

Then, X denotes the number of aces in a draw of 2 cards.

∴ X can assume the value 0,1 or 2.

Number of ways of drawing 2 cards out of 52 = C(52,2).

P(X = 0) = P(both non-aces, i.e., 2 non-aces out of 48)

= \(\frac{{ }^{48} C_2}{{ }^{52} C_2}=\left(\frac{48 \times 47}{2 \times 1} \times \frac{2}{52 \times 51}\right)=\frac{188}{221}\)

P(X=1)= \(P[(\text { one ace out of } 4) \text { and (one non-ace out of } 48)]\)

= \(\frac{{ }^4 C_1 \times{ }^{48} C_1}{{ }^{52} C_2}=\left(\frac{4 \times 48}{52 \times 51} \times 2\right)=\frac{32}{221}\)

P(X=2)= \(P(\text { both aces })=\frac{{ }^4 C_2}{{ }^{52} C_2}=\left(\frac{4 \times 3}{2 \times 1} \times \frac{2 \times 1}{52 \times 51}\right)=\frac{1}{221}\)

Thus, we have the following probability distribution:

 

∴ mean, \(\mu=\Sigma x_i p_i=\left(0 \times \frac{188}{221}\right)+\left(1 \times \frac{32}{221}\right)+\left(2 \times \frac{1}{221}\right)=\frac{2}{13}\)

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2
=\left(0 \times \frac{188}{221}\right)+\left(1 \times \frac{32}{221}\right)+\left(4 \times \frac{1}{221}\right)-\frac{4}{169}\)

=\(\left(\frac{36}{221}-\frac{4}{169}\right)=\frac{400}{2873}\)

Example 7. Three defective bulbs are mixed with 7 good ones. Let X be the number of defective bulbs when 3 bulbs are drawn at random. Find the mean and variance of X.
Solution:

Let X denote the random variable showing the number of defective bulbs.

Then, X can take the value 0,1,2 or 3.

∴ P(X = 0) = P(none of the bulbs is defective)

= P(all the 3 bulbs are good ones)

= \(\frac{{ }^7 C_3}{{ }^{10} C_3}\)=\(\left(\frac{7 \times 6 \times 5}{3 \times 2 \times 1} \times \frac{3 \times 2 \times 1}{10 \times 9 \times 8}\right)\)=\(\frac{7}{24}\).

P(X=1)=P(1 defective and 2 non-defective bulbs)

= \(\frac{{ }^3 C_1 \times{ }^7 C_2}{{ }^{10} C_3}=\left(3 \times \frac{7 \times 6}{2 \times 1} \times \frac{3 \times 2 \times 1}{10 \times 9 \times 8}\right)=\frac{21}{40}\).

P(X=2)=P(2 defective and 1 good one)

= \(\frac{{ }^3 C_2 \times{ }^7 C_1}{{ }^{10} C_3}=\left(\frac{3 \times 2}{2 \times 1} \times 7 \times \frac{3 \times 2 \times 1}{10 \times 9 \times 8}\right)=\frac{7}{40}\).

P(X=3)=P(3 defective bulbs)

= \(\frac{{ }^3 C_3}{{ }^{10} C_3}=\left(1 \times \frac{3 \times 2 \times 1}{10 \times 9 \times 8}\right)=\frac{1}{120}\).

Thus, the probability distribution is given by

 

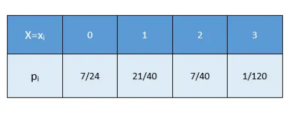
∴ mean, \(\mu=\Sigma x_i p_i=\left(0 \times \frac{7}{24}\right)+\left(1 \times \frac{21}{40}\right)+\left(2 \times \frac{7}{40}\right)+\left(3 \times \frac{1}{120}\right)=\frac{9}{10}\)

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2\)

= \(\left(0 \times \frac{7}{24}\right)+\left(1 \times \frac{21}{40}\right)+\left(4 \times \frac{7}{40}\right)+\left(9 \times \frac{1}{120}\right)-\frac{81}{100}\)

= \(\left(\frac{13}{10}-\frac{81}{100}\right)=\frac{49}{100}\).

Example 8. An urn contains 4 white and 3 red balls. Let X be the number of red balls in a random draw of 3 balls. Find the mean and variance of X.
Solution:

When 3 balls are drawn at random, there may be no red ball, 1 red ball, 2 red balls or 3 red balls.

Let X denote the random variable showing the number of red balls in a draw of 3 balls.

Then, X can take the value 0,1,2 or 3.

P(X=0) =P(getting no red ball)

= P(getting 3 white balls)

= \(\frac{{ }^4 C_3}{{ }^7 C_3}=\left(\frac{4 \times 3 \times 2}{3 \times 2 \times 1} \times \frac{3 \times 2 \times 1}{7 \times 6 \times 5}\right)=\frac{4}{35}\)

P(X=1)=P(getting 1 red and 2 white balls)

= \(\frac{{ }^3 C_1 \times{ }^4 C_2}{{ }^7 C_3}=\left(\frac{3 \times 4 \times 3}{2} \times \frac{3 \times 2 \times 1}{7 \times 6 \times 5}\right)=\frac{18}{35} \)

P(X=2) =P(\text { getting } 2 \text { red and } 1 \text { white ball })

= \(\frac{{ }^3 C_2 \times{ }^4 C_1}{{ }^7 C_3}=\left(\frac{3 \times 2}{2 \times 1} \times 4 \times \frac{3 \times 2 \times 1}{7 \times 6 \times 5}\right)=\frac{12}{35}\)

P(X=3)=P(getting 3 red balls)

= \(\frac{{ }^3 C_3}{{ }^7 C_3}=\frac{1 \times 3 \times 2 \times 1}{7 \times 6 \times 5}=\frac{1}{35}\).

Thus, the probability distribution of X is given below.

Class 12 Maths Probability Distribution Mean And Variance Of An Urn Contains 4 White And 3 red Balls

 

∴ mean, \(\mu=\Sigma x_i p_i=\left(0 \times \frac{4}{35}\right)+\left(1 \times \frac{18}{35}\right)+\left(2 \times \frac{12}{35}\right)+\left(3 \times \frac{1}{35}\right)=\frac{9}{7}\)

Variance, \(\sigma^2=\Sigma x_i^2 p_i-\mu^2\)

= \(\left[\left(0 \times \frac{4}{35}\right)+\left(1 \times \frac{18}{35}\right)+\left(4 \times \frac{12}{35}\right)+\left(9 \times \frac{1}{35}\right)-\frac{81}{49}\right]\)

= \(\left(\frac{15}{7}-\frac{81}{49}\right)=\frac{24}{49}\)

Example 9. In a game, 3 coins are tossed. A person is paid Rs 5 if he gets all heads or all tails and he is supposed to pay Rs 3 if he gets one head or two heads. What can he expect to win on an average per game?
Solution:

In tossing 3 coins, the sample space is given by

S = {HHH, HHT, HTH, THH, HTT, THT, TTH, TTT}.

∴ n(S) = 8.

P(getting all heads or all tails) = \(\frac{2}{8}\)=\(\frac{1}{4}\)

P(getting one head or 2 heads) = \(\frac{6}{8}\)=\(\frac{3}{4}\)

Let X = the number of rupees the person gets.

Then, possible values of X are 5 and -3.

P(X=5) = \(\frac{1}{4}\) and P(X=-3) = \(\frac{3}{4}\)

Thus, we have

 

∴ the required expectations = mean, \(\mu=\Sigma x_i p_i \)

= \(\left(5 \times \frac{1}{4}\right)+(-3) \times \frac{3}{4}=-1\),

i.e., he loses Re 1 per toss.

Probability Distribution Exercise

Question 1. Find the mean (μ), variance (σ²), and standard deviation (σ) for each of the following probability distributions:

 

 

 

 

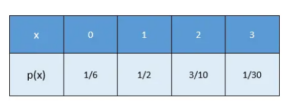
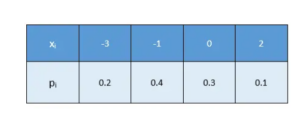
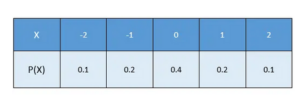
Solution:

Mean =1.5, variance=0.56, SD = 0.74

Mean =2, variance=1, SD =1

Mean = -0.8, variance=2.6, SD = 1.612

Mean = 0, variance=1.2, SD=1.095

Question 2. Find the mean and variance of the number of heads when two coins are tossed simultaneously.
Solution: Mean =1, variance=0.5

Hint: S = {HH, HT, TH, TT}.

Let X be the number of heads. Then, X= 0,1 or 2.

P(X= 0) = P(getting no head) = \(\frac{1}{4}\)

P(X= 1) =P(getting1 head) = \(\frac{2}{4}\) = \(\frac{1}{2}\)

P(X= 2) = P(getting 2 heads) = \(\frac{1}{4}\)

 

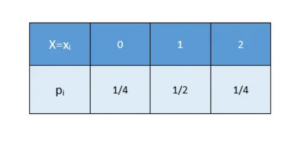
Question 3. Find the mean and variance of the number of tails when three coins are tossed simultaneously.
Solution: Mean =1.5, variance=0.75

 

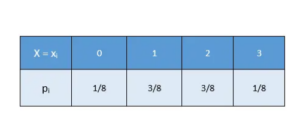
Question 4. A die is tossed twice. ‘Getting an odd number on a toss’ is considered a success. Find the probability distribution of a number of successes. Also, find the mean and variance of the number of successes.
Solution: Mean =1, variance=0.5

Hint: In a single toss,

P(success) = P(getting an odd number) = \(\frac{3}{6}\) = \(\frac{1}{2}\), and

P(non-success) = (1-\(\frac{1}{2}\)) = \(\frac{1}{2}\)

Let X be the number of successes. Then, X= 0,1 or 2.

P(X= 0) = P[(non-successin the 1st toss and non-successin the 2nd)]

= \(\left(\frac{1}{2} \times \frac{1}{2}\right)=\frac{1}{4}\).

P(X=1)- P[(successin the 1st toss and non-success in the 2nd) or (non-success in 1st toss and success in the 2nd)]

= \(\left(\frac{1}{2} \times \frac{1}{2}\right)+\left(\frac{1}{2} \times \frac{1}{2}\right)=\frac{1}{2}\)

P(X=2) =P[success in the 1 st toss and success in the 2nd]

= \(\left(\frac{1}{2} \times \frac{1}{2}\right)=\frac{1}{4}\).

 

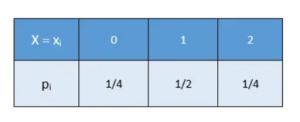
Question 5. A die is tossed twice. ‘Getting a number greater than 4’ is considered a success. Find the probability distribution of a number of successes. Also, find the mean and variance of the number of successes.
Solution: Mean=\(\frac{2}{3}\), variance=\(\frac{4}{9}\)

In a single toss, P(success) = and P(non-success) = (1-\(\frac{1}{3}\)) = \(\frac{2}{3}\)

P(X = 0) = P(non-success in the 1st draw and non-success in the second)

= \(\left(\frac{2}{3} \times \frac{2}{3}\right)=\frac{4}{9} \text {. }\)

P(X=1) = P(success in the ls.t toss and non-success in the 2nd) or ( non-success in the 1st toss and success in the 2nd)]

= \(\left(\frac{1}{3} \times \frac{2}{3}\right)+\left(\frac{2}{3} \times \frac{1}{3}\right)=\frac{4}{9}\)

P(X = 2) = P[(success in the 1st toss and success in the 2nd)]

= \(\left(\frac{1}{3} \times \frac{1}{3}\right)=\frac{1}{9}\).

 

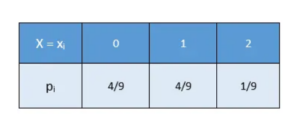
Question 6. A pair of dice is thrown 4 times. If getting a doublet is considered a success, find the probability distribution of a number of successes. Also, find the mean and variance of a number of successes.
Solution: Mean=\(\frac{2}{3}\), variance=\(\frac{5}{9}\)

Hint: In a single throw, P(doublet) = 6/36, 1/6, and P(non-doublet) = (1-\(\frac{1}{6}\)) = \(\frac{5}{6}\)

Let X be the number of doublets. Then, X = 0, 1, 2 or 3.

P(X = 0) = P(non-doublet in each case)

= \(P\left(\bar{D}_1 \bar{D}_2 \bar{D}_3 \bar{D}_4\right)=\left(\frac{5}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{5}{6}\right)=\frac{625}{1296}\)

P(X=1)= P(one doublet)

= \(P\left(D_1 \bar{D}_2 \bar{D}_3 \bar{D}_4\right) \text { or } P\left(\bar{D}_1 D_2 \bar{D}_3 \bar{D}_4\right) \)

or \(P\left(\bar{D}_1 \bar{D}_2 D_3 \bar{D}_4\right) \text { or } P\left(\bar{D}_1 \bar{D}_2 \bar{D}_3 D_4\right) \)

= \(\left(\frac{1}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{5}{6}\right)+\left(\frac{5}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{5}{6}\right)+\left(\frac{5}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{5}{6}\right)+\left(\frac{5}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{1}{6}\right)\)

= \(\left(4 \times \frac{125}{1296}\right)=\frac{125}{324}\)

P(X=2)= P(two doublets)

= \(P\left(D_1 D_2 \bar{D}_3 \bar{D}_4\right) \quad \text { or } P\left(D_1 \bar{D}_2 D_3 \bar{D}_4\right) \text { or } P\left(D_1 \bar{D}_2 \bar{D}_3 D_4\right)\)

or \(P\left(\bar{D}_1 D_2 D_3 \bar{D}_4\right) \text { or } P\left(\bar{D}_1 D_2 \bar{D}_3 D_4\right) \text { or } P\left(\bar{D}_1 \bar{D}_2 D_3 D_4\right)\)

= \(\left(\frac{1}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{5}{6}\right)+\left(\frac{1}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{5}{6}\right)+\left(\frac{1}{6} \times \frac{5}{6} \times \frac{5}{6} \times \frac{1}{6}\right)\)

+ \(\left(\frac{5}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{5}{6}\right)+\left(\frac{5}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{1}{6}\right)+\left(\frac{5}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{1}{6}\right)\)

= \(\left(6 \times \frac{25}{1296}\right)=\frac{25}{216}\)

P(X=3)= P(three doublets)

= \(P\left(D_1 D_2 D_3 \bar{D}_4\right) \text { or } P\left(D_1 D_2 \bar{D}_3 D_4\right)\)

or \(P\left(D_1 \bar{D}_2 D_3 D_4\right) \text { or } P\left(\bar{D}_1 D_2 D_3 D_4\right)\)

= \(\left(\frac{1}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{5}{6}\right)+\left(\frac{1}{6} \times \frac{1}{6} \times \frac{5}{6} \times \frac{1}{6}\right)+\left(\frac{1}{6} \times \frac{5}{6} \times \frac{1}{6} \times \frac{1}{6}\right)+\left(\frac{5}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{1}{6}\right)\)

= \(\left(4 \times \frac{5}{1296}\right)=\frac{5}{324} \)

P(X=4)= P(four doublets)=P\left(D_1 D_2 D_3 D_4\right)[/latex]

= \(\left(\frac{1}{6} \times \frac{1}{6} \times \frac{1}{6} \times \frac{1}{6}\right)=\frac{1}{1296}\)

Thus, we have

 

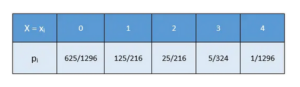
Question 7. A coin is tossed 4 times. Let X denote the number of heads. Find the probability distribution of X. Also, find the mean and variance of X.
Solution: Mean=2, variance=1

In a single throw of a coin, \(P(H)=\frac{1}{2}\) and \(P(\bar{H})=P(T)=\frac{1}{2}\).

Let X show the number of heads. Then, X=0,1,2,3 or 4 .

P(X=0)=P(no head)=\(P\left(\bar{H}_1 \bar{H}_2 \bar{H}_3 \bar{H}_4\right)=\left(\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}\right)=\frac{1}{16} \text {. }\)

P(X=1)=P(one head)= \(P\left(H_1 \bar{H}_2 \bar{H}_3 \bar{H}_4\right) \text { or }\left(\bar{H}_1 H_2 \bar{H}_3 \bar{H}_4\right)\)

or \(P\left(\bar{H}_1 \bar{H}_2 H_3 \bar{H}_4\right) \text { or } P\left(\bar{H}_1 \bar{H}_2 \bar{H}_3 H_4\right) \)

= \(4\left(\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}\right)=\left(4 \times \frac{1}{16}\right)=\frac{1}{4}\)

P(X=2)=P(two heads)= \(P\left(H_1 H_2 \bar{H}_3 \bar{H}_4\right) \text { or } P\left(H_1 \bar{H}_2 H_3 \bar{H}_4\right)\)

or \(P\left(H_1 \bar{H}_2 \bar{H}_3 H_4\right) \text { or } P\left(\bar{H}_1 H_2 H_3 \bar{H}_4\right)\)

or \(P\left(\bar{H}_1 H_2 \bar{H}_3 H_4\right) \text { or } P\left(\bar{H}_1 \bar{H}_2 H_3 H_4\right)\)

= \(\left(6 \times \frac{1}{16}\right)=\frac{3}{8}\)

P(X=3)=P(\text { three heads })=P\left(H_1 H_2 H_3 \bar{H}_4\right) \text { or }\left(H_1 H_2 \bar{H}_3 H_4\right)[/latex]

or \(P\left(H_1 \bar{H}_2 H_3 H_4\right) \text { or } P\left(\bar{H}_1 H_2 H_3 H_4\right)\)

= \(4 \times\left(\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}\right)=\left(4 \times \frac{1}{16}\right)=\frac{1}{4} \text {. }\)

P(X=4)=P(four heads)= \(P\left(H_1 H_2 H_3 H_4\right)=\left(\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}\right)=\frac{1}{16}\).

Thus, we have

 

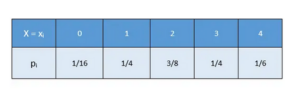
Question 8. Let X denote the number of times ‘a total of 9’ appears in two throws of a pair of dice. Find the probability distribution of X. Also, find the mean, variance, and standard deviation of X.
Solution: Mean=\(\frac{2}{9}\), variance=\(\frac{16}{81}\), SD = \(\frac{4}{9}\)

Hint: Let E={(3,6),(6,3),(4,5),(5,4)}. So, n(E)=4.

∴ P(E)= \(\frac{4}{36}=\frac{1}{9}\), and \(P(\bar{E})=\left(1-\frac{1}{9}\right)=\frac{8}{9}\).

Let X be the number of times ‘a total of 9’ appears in 2 throws Then, X=0, 1 or 2.

P(X=0)= \(=P\left(\bar{E}_1 \bar{E}_2\right)=\left(\frac{8}{9} \times \frac{8}{9}\right)=\frac{64}{81}\)

P(X=1)= \(P\left[\left(E_1 \bar{E}_2\right) \text { or }\left(\bar{E}_1 E_2\right)\right]=P\left(E_1 \bar{E}_2\right)+P\left(\bar{E}_1 E_2\right)\)

= \(\left(\frac{1}{9} \times \frac{8}{9}\right)+\left(\frac{8}{9} \times \frac{1}{9}\right)=\frac{16}{81}\)

P(X=2)= \(P\left(E_1 E_2\right)=P\left(E_1\right) \times P\left(E_2\right)=\left(\frac{1}{9} \times \frac{1}{9}\right)=\frac{1}{81}\)

 

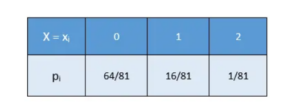
Question 9. There are 5 cards, numbered 1 to 5, with one number on each card. Two cards are drawn at random without replacement. Let X denote the sum of the numbers on the two cards drawn. Find the mean and variance of X.
Solution: Mean=6, variance=3

Hint: S ={(1, 2), (1, 3), (1, 4), (1, 5), (2, 1), (2, 3), (2, 4), (2, 5), (3, 1), (3, 2), (3, 4), (3, 5),
(4, 1), (4, 2), (4, 3), (4, 5), (5, 1), (5, 2), (5, 3), (5, 4)}

Then X = 3, 4, 5, 6, 7, 8 and 9.

P(X=3)=\(\frac{2}{20}\)=\(\frac{1}{10}\);

P(X=4)=\(\frac{2}{10}\)=\(\frac{1}{10}\)

P(X=5)=\(\frac{4}{20}\)=\(\frac{1}{5}\)

P(X=6)=\(\frac{4}{20}\)=\(\frac{1}{5}\)

P(X=7)=\(\frac{4}{20}\)=\(\frac{1}{5}\)

P(X=8)=\(\frac{2}{20}\)=\(\frac{1}{10}\)

P(X=9)=\(\frac{2}{20}\)=\(\frac{1}{10}\)

Thus, we have

 

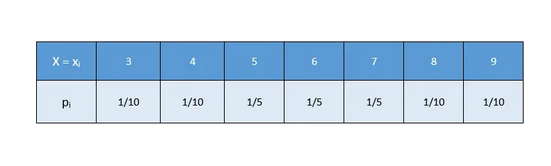
Question 10. Two cards are drawn from a well-shuffled pack of 52 cards. Find the probability distribution of a number of kings. Also, compute the variance for the number of kings.
Solution: Variance=\(\frac{400}{2873}\)

Hint: Let X be the number of kings. Then, X = 0,1 or 2.

P(X = 0) = P(none is a king) = P(both are non-kings)

= \(\frac{{ }^{48} C_2}{{ }^{52} C_2}=\left(\frac{48 \times 47}{2} \times \frac{2}{52 \times 51}\right)=\frac{188}{221} .\)

P(X = 1) = P(one king and one non-king)

= \(\frac{{ }^4 C_1 \times{ }^{48} C_1}{{ }^{52} C_2}=\left(4 \times 48 \times \frac{2}{52 \times 51}\right)=\frac{32}{221} .\)

P(X = 2) = P(both are kings)

= \(\frac{{ }^4 C_2}{{ }^{52} C_2}=\left(\frac{4 \times 3}{2 \times 1} \times \frac{2 \times 1}{52 \times 51}\right)=\frac{1}{221}\)

Thus, we have

 

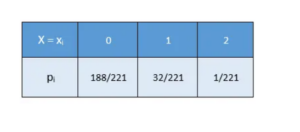
Question 11. A box contains 16 bulbs, out of which 4 bulbs are defective. Three bulbs are drawn at random from the box. Let X be the number of defective bulbs drawn. Find the mean and variance of X.
Solution: Mean=\(\frac{3}{4}\), variance=\(\frac{39}{80}\)

Hint: Three bulbs drawn one by one without replacement is the same as drawing 3 bulbs simultaneously.

Let X = number of defective bulbs in a lot of 3 bulbs drawn.

Then, X = 0, 1, 2 or 3.

P(X = 0) = P(none of the bulbs is defective)

= \(\frac{{ }^{12} C_3}{{ }^{16} C_3}=\left(\frac{12 \times 11 \times 10}{3 \times 2 \times 1} \times \frac{3 \times 2 \times 1}{16 \times 15 \times 14}\right)=\frac{11}{28}\)

P(X=1)=P(1 defective bulb and 2 non-defective bulbs)

= \(\frac{{ }^4 C_1 \times{ }^{12} C_2}{{ }^{16} C_3}=\left(\frac{4 \times 12 \times 11}{2 \times 1} \times \frac{3 \times 2 \times 1}{16 \times 15 \times 14}\right)=\frac{33}{70}\)

P(X=2)=P(2 defective bulbs and 1 non-defective bulb)

= \(\frac{\left({ }^4 C_2 \times{ }^{12} C_1\right)}{{ }^{16} C_3}=\left(\frac{4 \times 3}{2 \times 1} \times 12 \times \frac{3 \times 2 \times 1}{16 \times 15 \times 14}\right)=\frac{9}{70}\).

P(X=3)=P(3 defective bulbs)

= \(\frac{{ }^4 C_3}{{ }^{16} C_3}=\left(\frac{4 \times 3 \times 2}{3 \times 2 \times 1} \times \frac{3 \times 2 \times 1}{16 \times 15 \times 14}\right)=\frac{1}{140}\).

 

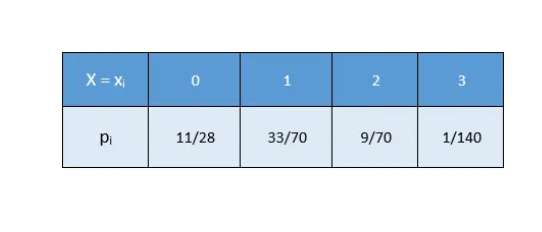
Question 12. 20% of the bulbs produced by a machine are defective. Find the probability distribution of the number of defective bulbs in a sample of 4 bulbs chosen at random.
Solution:

Let there be 100 bulbs in all and let X be the number of defective bulbs. Then,

P(X = 0) = P(none is defective) = \(=\frac{{ }^{80} C_4}{{ }^{100} C_4} .\)

P(X = 1) = P(1 defective and 3 non-defective)

= \(\frac{\left({ }^{20} C_1 \times{ }^{00} C_3\right)}{{ }^{100} C_4}\)

P(X=2)= \(\frac{\left({ }^{20} C_2 \times{ }^{80} C_2\right)}{{ }^{100} C_4} ; P(X=3)=\frac{\left({ }^{20} C_3 \times{ }^{80} C_1\right)}{{ }^{100} C_4} ; P(X=4)=\frac{{ }^{20} C_4}{{ }^{100} C_4}\)

Question 13. Four bad eggs are mixed with 10 good ones. Three eggs are drawn one by one without replacement. Let X be the number of bad eggs drawn. Find the mean and variance of X.
Solution: Mean=\(\frac{6}{7}\), variance=\(\frac{30}{49}\)

Question 14. Four rotten oranges are accidentally mixed with 16 good ones. Three oranges are drawn at random from the mixed lot. Let X be the number of rotten oranges drawn. Find the mean and variance of X.
Solution: Mean=\(\frac{3}{5}\), variance=\(\frac{68}{125}\)

Question 15. Three balls are drawn simultaneously from a bag containing 5 white and 4 red balls. Let X be the number of red balls drawn. Find the mean and variance of X.
Solution: Mean=\(\frac{4}{3}\), variance=\(\frac{5}{9}\)

Hint:

P(X=0)= \(\frac{{ }^5 C_3}{{ }^9 C_3}=\frac{5}{42}, P(X=1)=\frac{\left({ }^4 C_1 \times{ }^5 C_2\right)}{{ }^9 C_3}=\frac{10}{21},\)

P(X=2)=\(\frac{\left({ }^4 C_2 \times{ }^5 C_1\right)}{{ }^9 C_3}=\frac{5}{14}, P(X=3)=\frac{{ }^4 C_3}{{ }^9 C_3}=\frac{1}{21}\).

Question 16. Two cards are drawn without replacement from a well-shuffled deck of 52 cards. Let X be the number of face cards drawn. Find the mean and variance of X.
Solution: Mean=\(\frac{20}{13}\), variance=\(\frac{1000}{2873}\)

Hint: There are 12 face cards (4 kings, 4 queens and 4 jacks).

Clearly, X = 0 or 1 or 2.

P(X = 0) = P(no face card)

= P(drawing 2 cards out of 40 non-face cards)

= \(\frac{{ }^{40} C_2}{{ }^{52} C_2}=\left(\frac{40 \times 39}{2 \times 1} \times \frac{2 \times 1}{52 \times 51}\right)=\frac{10}{17}\)

P(X=1)=P(1 face card and 1 non-face card)

= \(\frac{\left({ }^{12} C_1 \times{ }^{40} C_1\right)}{{ }^{52} C_2}=\left(12 \times 40 \times \frac{2 \times 1}{52 \times 51}\right)=\frac{80}{221}\).

P(X=2)=P(2 face cards)

= \(\frac{{ }^{40} C_2}{{ }^{52} C_2}=\left(\frac{40 \times 39}{2 \times 1} \times \frac{2 \times 1}{52 \times 51}\right)=\frac{10}{17}\)

Thus, we have

 

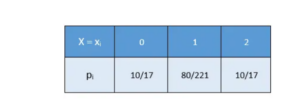
Question 17. Two cards are drawn one by one with replacement from a well-shuffled deck of 52 cards. Find the mean and variance of the number of aces.
Solution: Mean=\(\frac{2}{13}\), variance=\(\frac{24}{169}\)

Question 18. Three cards are drawn successively with replacement from a well-shuffled deck of 52 cards. A random variable X denotes the number of hearts in the three cards drawn. Find the mean and variance of X.
Solution: Mean=\(\frac{3}{4}\), variance=\(\frac{9}{16}\)

Hint: There are 13 hearts and 39 other cards.

Let E = event of drawing a heart.

Then, \(P(E)=\frac{13}{52}=\frac{1}{4} \text { and } P(\bar{E})=\left(1-\frac{1}{4}\right)=\frac{3}{4}\)

Let X = number of hearts in a draw.

Then, X = 0,1, 2 or 3.

P(X=0)=\(P(\bar{E} \bar{E} \bar{E})=P(\bar{E}) \times P(\bar{E}) \times P(\bar{E})=\left(\frac{3}{4} \times \frac{3}{4} \times \frac{3}{4}\right)=\frac{27}{64}\)

P(X=1)=\(P[(E \bar{E} \bar{E}) \text { or }(\bar{E} E \bar{E}) \text { or }(\bar{E} \bar{E} E)]\)

= \(P(E \bar{E} \bar{E})+P(\bar{E} E \bar{E})+P(\bar{E} \bar{E} E)\)

= \(\left(\frac{1}{4} \times \frac{3}{4} \times \frac{3}{4}\right)+\left(\frac{3}{4} \times \frac{1}{4} \times \frac{3}{4}\right)+\left(\frac{3}{4} \times \frac{3}{4} \times \frac{1}{4}\right)=\frac{27}{64}\)

P(X=2)= \(P[(E E \bar{E}) \text { or }(E \bar{E} E) \text { or }(\bar{E} E E)]\)

= \(P(E E \bar{E})+P(E \bar{E} E)+P(\bar{E} E E)\)

= \(\left(\frac{1}{4} \times \frac{1}{4} \times \frac{3}{4}\right)+\left(\frac{1}{4} \times \frac{3}{4} \times \frac{1}{4}\right)+\left(\frac{3}{4} \times \frac{1}{4} \times \frac{1}{4}\right)=\frac{9}{64}\)

P(X=3) = \(P(E E E)=P(E) \times P(E) \times P(E)=\left(\frac{1}{4} \times \frac{1}{4} \times \frac{1}{4}\right)=\frac{1}{64}\)

 

Bayes Theorem and Its Applications

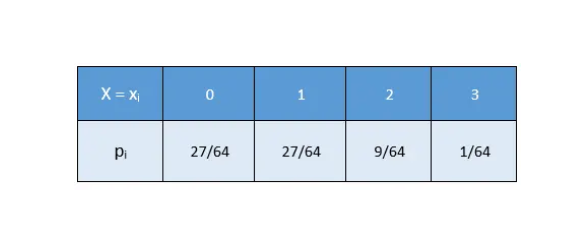
Theorem of Total Probability

Theorem: Let E₁, E₂,..E_n be mutually exclusive and exhaustive events associated with a random experiment and let E be an event that occurs with some E_i. Then, prove that

⇒ P(E) = \(\sum_{i=1}^n P\left(E / E_i\right) \cdot P\left(E_i\right) .\)

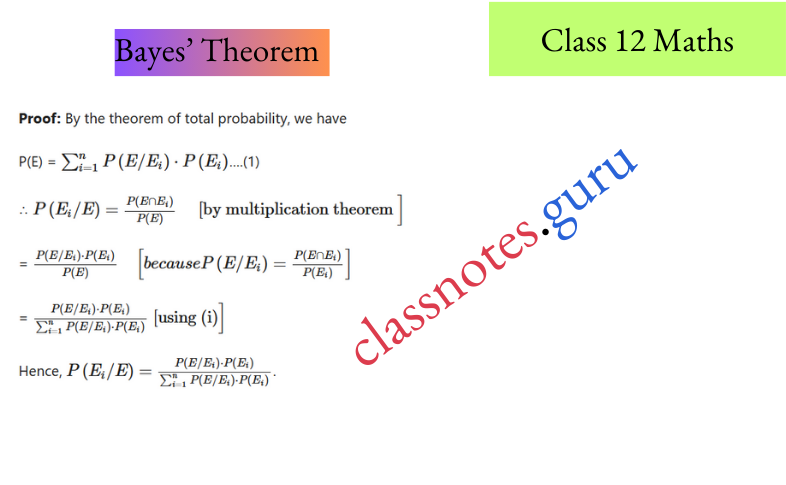
Example: There are three urns containing 3 white and 2 black balls; 2 white and 3 black balls; 1 black and 4 white balls respectively. There is an equal probability of each urn being chosen. One ball is drawn from an urn chosen at random. What is the probability that a white ball is drawn?
Solution:

Read and Learn More WBCHSE Solutions For Class 12 Maths

Let E₁, E_2, and E₃ be the events of choosing the first, second, and third urn respectively.

Then, P(E₁) = P(E₂) = P(E₃) = \(\frac{1}{3}\)

Let E be the event that a white ball is drawn. Then,

P(E/E₁) = \(\frac{3}{5}\), P(E/E₂) = \(\frac{2}{5}\) and P(E/E₃) = \(\frac{4}{5}\)

By the theorem of total probability, we have

P(E) = P(E/E₁) • P(E₁) + P(E/E₂) • P(E₂) + P(E/E₃) • P(E₃)

= \(\left(\frac{3}{5} \times \frac{1}{3}+\frac{2}{5} \times \frac{1}{3}+\frac{4}{5} \times \frac{1}{3}\right)=\left(\frac{1}{5}+\frac{2}{15}+\frac{4}{15}\right)=\frac{9}{15}=\frac{3}{5} .\)

Bayes’ Theorem: Let E₁, E₂…, E_n be mutually exclusive and exhaustive events, associated with a random experiment, and let E be any event that occurs with some Ei. Then,

⇒ \(P\left(E_i / E\right)=\frac{P\left(E / E_i\right) \cdot P\left(E_i\right)}{\sum_{i=1}^n P\left(E / E_i\right) \cdot P\left(E_i\right)} ; i=1,2,3, \ldots, n\)

Proof: By the theorem of total probability, we have

P(E) = \(\sum_{i=1}^n P\left(E / E_i\right) \cdot P\left(E_i\right)\)….(1)

∴ \(P\left(E_i / E\right)= \left.\frac{P\left(E \cap E_i\right)}{P(E)} \quad \text { [by multiplication theorem }\right]\)

= \(\frac{P\left(E / E_i\right) \cdot P\left(E_i\right)}{P(E)} \quad\left[because P\left(E / E_i\right)=\frac{P\left(E \cap E_i\right)}{P\left(E_i\right)}\right]\)

= \(\left.\frac{P\left(E / E_i\right) \cdot P\left(E_i\right)}{\sum_{i=1}^n P\left(E / E_i\right) \cdot P\left(E_i\right)} \text { [using }(\mathrm{i})\right]\)

Hence, \(P\left(E_i / E\right)= \frac{P\left(E / E_i\right) \cdot P\left(E_i\right)}{\sum_{i=1}^n P\left(E / E_i\right) \cdot P\left(E_i\right)}\).

Bayes Theorem Solved Examples

Example 1. A factory has three machines, X, Y, and Z, producing 1000,2000 3000 bolts per day respectively. The machine X produces 1% defective bolts, Y produces 1.5% defective bolts and Z produces 2% defective bolts. At the end of the day, a bolt is drawn at random and it is found to be defective. What is the probability that this defective bolt has been produced by the machine X?
Solution:

Total number of bolts produced in a day = (1000 + 2000 + 3000) = 6000.

Let E₁, E_2, and E₃ be the events of drawing a bolt produced by machines X, Y, and Z respectively.

Then, \(P\left(E_1\right)=\frac{1000}{6000}=\frac{1}{6} ; P\left(E_2\right)=\frac{2000}{6000}=\frac{1}{3} \text { and } P\left(E_3\right)=\frac{3000}{6000}=\frac{1}{2}\)

Let E be the event of drawing a defective bolt.

Then, P(E/E₁) = probability of drawing a defective bolt, given that it is produced by the machine X = \(\frac{1}{100}\)

P(E/E₂) = probability of drawing a defective bolt, given that it is produced by the machine Y = \(\frac{1.5}{100}\)= \(\frac{15}{1000}\) = \(\frac{3}{200}\)

P(E/E₃) = probability of drawing a defective bolt, given that it is produced by the machine Z = \(\frac{2}{100}\) = \(\frac{1}{50}\)

Required probability = P(E₁/E) = probability that the bolt drawn is produced by X, given that it is defective

= \(\frac{P\left(E_1\right) \cdot P\left(E / E_1\right)}{P\left(E_1\right) \cdot P\left(E / E_1\right)+P\left(E_2\right) \cdot P\left(E / E_2\right)+P\left(E_3\right) \cdot P\left(E / E_3\right)}\)

= \(\frac{\left(\frac{1}{6} \times \frac{1}{100}\right)}{\left(\frac{1}{6} \times \frac{1}{100}\right)+\left(\frac{1}{3} \times \frac{3}{200}\right)+\left(\frac{1}{2} \times \frac{1}{50}\right)}\)

= \(\left(\frac{1}{600} \times \frac{600}{10}\right)=\frac{1}{10}=0.1\)

Hence, the required probability is 0.1.

Example 2. In a bolt factory, three machines, A, B, and C, manufacture 25%, 35%, and 40% of the total production respectively. Of their respective outputs, 5%, 4%, and 2% are defective. A bolt is drawn at random from the total product and it is found to be defective. Find the probability that it was manufactured by machine C.
Solution:

Let E₁, E_2, and E₃ be the events of drawing a bolt produced by machines A, B, and C respectively.

Then \(P\left(E_1\right)=\frac{25}{100}=\frac{1}{4}, P\left(E_2\right)=\frac{35}{100}=\frac{7}{20} \text {, and } P\left(E_3\right)=\frac{40}{100}=\frac{2}{5} \text {. }\)

Let E be the event of drawing a defective bolt. Then,

P(E/E₁) = probability of drawing a defective bolt, given that it is produced by the machine A = \(\frac{5}{100}\)=\(\frac{1}{20}\)

P(E/E₂) = probability of drawing a defective bolt, given that it is produced by the machine B = \(\frac{4}{100}\)=\(\frac{1}{25}\)

P(E/E₃) = probability of drawing a defective bolt, given that it is produced by the machine C = \(\frac{2}{100}\)=\(\frac{1}{50}\)

The probability that the bolt drawn is manufactured by C, given that it is defective

= \(P\left(E_3 / E\right)\)

= \(\frac{P\left(E / E_3\right) \cdot P\left(E_3\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)+P\left(E / E_3\right) \cdot P\left(E_3\right)}\) [by Bayes’ theorem]

= \(\frac{\left(\frac{1}{50} \times \frac{2}{5}\right)}{\left(\frac{1}{20} \times \frac{1}{4}\right)+\left(\frac{1}{25} \times \frac{7}{20}\right)+\left(\frac{1}{50} \times \frac{2}{5}\right)}=\left(\frac{1}{125} \times \frac{2000}{69}\right)=\frac{16}{69}\) .

Hence, the required probability is \(\frac{16}{69}\)

Example 3. A company has two plants to manufacture bicycles. The first plant manufactures 60% of the bicycles and the second plant, 40%. Also, 80% of the bicycles are rated of standard quality at the first plant and 90% of standard quality at the second plant. A bicycle is picked up at random and found to be of standard quality. Find the probability that it comes from the second plant.
Solution:

Let E₁ and E₂ be the events of choosing a bicycle from the first plant and the second plant respectively.

Then, \(P\left(E_1\right)=\frac{60}{100}=\frac{3}{5} \text {, and } P\left(E_2\right)=\frac{40}{100}=\frac{2}{5} \text {. }\)

Let E be the event of choosing a bicycle of standard quality. Then,

P(E/E₁) = probability of choosing a bicycle of standard quality, given that it is produced by the first plant = \(\frac{80}{100}\) = \(\frac{4}{5}\)

P(E/E₂) = probability of choosing a bicycle of standard quality, given that it is produced by the second plant = \(\frac{90}{100}\) = \(\frac{9}{10}\)

The required probability

P(E₂/E) = probability of choosing a bicycle from the second plant, given that it is of standard quality

= \(\frac{P\left(E_2\right) \cdot P\left(E / E_2\right)}{P\left(E_1\right) \cdot P\left(E / E_1\right)+P\left(E_2\right) \cdot P\left(E / E_2\right)}\) [by Bayes’ theorem]

= \(\frac{\left(\frac{2}{5} \times \frac{9}{10}\right)}{\left(\frac{3}{5} \times \frac{4}{5}\right)+\left(\frac{2}{5} \times \frac{9}{10}\right)}=\frac{3}{7}\).

Example 4. An insurance company insured 2000 scooter drivers, 4000 car drivers, and 6000 truck drivers. The probability of an accident involving a scooter, a car, and a truck is 1/ 100, 3/100, and 3/20 respectively. One of the insured persons met with an accident. What is the probability that he is a scooter driver?
Solution:

Total number of persons insured = (2000 + 4000 + 6000) = 12000.

Let E₁, E_2, and E₃ be the events of choosing a scooter driver, a car driver, and a truck driver respectively.

Then, \(P\left(E_1\right)=\frac{2000}{12000}=\frac{1}{6}, P\left(E_2\right)=\frac{4000}{12000}=\frac{1}{3} \text {, and } P\left(E_3\right)=\frac{6000}{12000}=\frac{1}{2} \text {. }\)

Let E be the event of an insured person meeting with an accident. Then,

P(E/E₁) = probability that an insured person meets with an accident, given that he is a scooter driver = \(\frac{1}{100}|\)

Similarly, P(E/E₂) = \(\frac{3}{100}|\) and P(E/E₃) = \(\frac{3}{20}|\)

Required probability = P(E₁/E) [by Bayes’ theorem]

= probability of choosing a scooter driver, given that he meets with an accident

= \(\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)+P\left(E / E_3\right) \cdot P\left(E_3\right)}\)

= \(\frac{\left(\frac{1}{100} \times \frac{1}{6}\right)}{\left(\frac{1}{100} \times \frac{1}{6}\right)+\left(\frac{3}{100} \times \frac{1}{3}\right)+\left(\frac{3}{20} \times \frac{1}{2}\right)}=\frac{1}{52}\).

Hence, the required probability is \(\frac{1}{52}\)

Example 5. A doctor to visit a patient. From past experience, it is known that the probabilities that he will come by train, bus, scooter, or by car are respectively \(\frac{3}{100}\), \(\frac{1}{5}\), \(\frac{1}{10}\) and \(\frac{2}{5}\). The probabilities that he will be late are \(\frac{1}{4}\), \(\frac{1}{3}\), and \(\frac{1}{12}\), if he comes by train, bus and scooter respectively; but if he comes by car, he will not be late. When he arrives, he is late. What is the probability that he has come by train?
Solution:

Let E₁, E₂, E₃, and E₄ be the events that the doctor comes by train, bus, scooter, and car respectively.

Then, \(P\left(E_1\right)=\frac{3}{10}, P\left(E_2\right)=\frac{1}{5}, P\left(E_3\right)=\frac{1}{10} \text { and } P\left(E_4\right)=\frac{2}{5} \text {. }\)

Let E be the event that the doctor is late. Then,

P(E/E₁) = probability that the doctor is late, given that he comes by train = \(\frac{1}{4}\)

P(E/E₂) = probability that the doctor is late, given that he comes by bus =\(\frac{1}{3}\)

P(E/E₃) = probability that the doctor is late, given that he comes by scooter = \(\frac{1}{12}\)

P(E/E₄) = probability that the doctor is late, given that he comes by car = 0.

The probability that he comes by train, given that he is late = P(E₁/E)

= \(\frac{P\left(E_1\right) \cdot P\left(E / E_1\right)}{P\left(E_1\right) \cdot P\left(E / E_1\right)+P\left(E_2\right) \cdot P\left(E / E_2\right)+P\left(E_3\right) \cdot P\left(E / E_3\right)+P\left(E_4\right) \cdot P\left(E / E_4\right)}\) [by Bayes’ theorem]

\(\left.=\frac{\left(\frac{3}{10} \times \frac{1}{4}\right)}{\left(\frac{3}{10} \times \frac{1}{4}\right)+\left(\frac{1}{5} \times \frac{1}{3}\right)+\left(\frac{1}{10} \times \frac{1}{12}\right)+\left(\frac{2}{5} \times 0\right)} \times \frac{120}{18}\right)=\frac{1}{2}\).

Hence the required probability is \(\frac{1}{2}\)

Example 6. A man is known to speak the truth 3 out of 4 times. He throws a die and reports that it is a six. Find the probability that it is actually a six.
Solution:

In a throw of a die, let

E₁ = event of getting a six,

E₂ = event of not getting a six, and

E = event that the man. reports that it is a six. Then,

Then, P(E₁) = \(\frac{1}{6}\) , and P(E₂) = (1-\(\frac{1}{6}\) = \(\frac{1}{5}[/latex

P(E/E₁) = probability that tire man reports that six occurs when six has actually occurred

= probability that the man speaks the truth = [latex]\frac{3}{4}\)

P(E/E₂) = probability that the man reports that six occurs when six has not actually occurred

= probability that the man does not speak the truth = (1-\(\frac{3}{4}\)) = \(\frac{1}{4}\)

Probability of getting a six, given that the man reports it to be six

= \(P\left(E_1 / E\right)\)

= \(\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)}\) [by Bayes’ theorem]

= \(\frac{\left(\frac{3}{4} \times \frac{1}{6}\right)}{\left(\frac{3}{4} \times \frac{1}{6}\right)+\left(\frac{1}{4} \times \frac{5}{6}\right)}=\left(\frac{1}{8} \times 3\right)=\frac{3}{8}\) .

Hence, the required probability is \(\frac{3}{8}\).

Example 7. In an examination, an examinee either guesses or copies or knows the answer to a multiple-choice question with four choices. The probability that he makes a guess is (1/3) and the probability that he copies the answer is (1/6). The probability that his answer is correct, given that he copied it, is (1/8). The probability that his answer is correct, given that he guessed it, is (1/4). Find the probability that he him the answer to the question, given that he correctly answered it.
Solution:

Let E₁ = event that the examinee guesses the answer,

E₂ = event that he copies the answer,

E₃ = event that he knows the answer, and

E = event that he answers correctly.

Then, \(P\left(E_1\right)=\frac{1}{3}, P\left(E_2\right)=\frac{1}{6}, \text { and } P\left(E_3\right)=1-\left(\frac{1}{3}+\frac{1}{6}\right)=\frac{1}{2}\)

[E₁, E₂, E₃ are mutually exclusive and exhaustive].

∴ P(E/E₁) = probability that he answers correctly, given that he guesses \(\frac{1}{4}\)

P(E/E₂) = probability that he answers correctly, given that he copies \(\frac{1}{8}\)

P(E/E₃) = probability that he answers correctly, given that he knew the answer = 1.

Required probability = \(P\left(E_3 / E\right)\)

= \(\frac{P\left(E / E_3\right) \cdot P\left(E_3\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)+P\left(E / E_3\right) \cdot P\left(E_3\right)}\) [by Bayes’ theorem]

= \(\frac{\left(1 \times \frac{1}{2}\right)}{\left(\frac{1}{4} \times \frac{1}{3}\right)+\left(\frac{1}{8} \times \frac{1}{6}\right)+\left(1 \times \frac{1}{2}\right)}=\frac{24}{29}\).

Hence, the required probability is \(\frac{24}{29}\).

Example 8. By examining the chest X-ray, the probability that a person is diagnosed with TB when he is actually suffering from it is 0.99. The probability that the doctor incorrectly diagnoses a person to be having TB, on the basis of X-ray reports, is 0.001. In a certain city, 1 in 1000 persons suffers from TB. A person is selected at random and is diagnosed to have TB. What is the chance that he actually has TB?
Solution:

Let E = event that the doctor diagnoses TB,

E₁ = event that the person selected is suffering from TB, and

E₂ = event that the person selected is not suffering from TB.

P(E/E₁) = probability that TB is diagnosed when the person actually has TB = \(\frac{99}{100}\)

P(E/E₂) = probability that TB is diagnosed, when the person has no TB = \(\frac{1}{1000}\)

Using Bayes’ theorem, we have

P(E₁/E) = probability of a person actually having TB, if it is known that he is diagnosed to have TB

= \(\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)}\)

= \(\frac{\left(\frac{99}{100} \times \frac{1}{1000}\right)}{\left(\frac{99}{100} \times \frac{1}{1000}\right)+\left(\frac{1}{1000} \times \frac{999}{1000}\right)}=\frac{110}{221}\).

Hence, the required probability is  \(\frac{110}{221}\).

Example 9. Bag A contains 2 white and 3 red balls, and bag B contains 4 white and 5 red balls. One ball is drawn at random from one of the bags and it is found to be red. Find the probability that it was drawn from bag B.
Solution:

Let E₁ = event of choosing bag A,

E₂ = event of choosing bag B, and E = event of drawing a red ball.

Then, P(E₁) = \(\frac{1}{2}\) and P(E₂) = \(\frac{1}{2}\)

Also, P(E/E₁) = event of drawing a red ball from bag A = \(\frac{3}{5}\) and

P(E/E₂) = event of drawing a red ball from bag B = \(\frac{5}{9}\)

The probability of drawing a ball from B, it is given that it is red = P(E₂/E)

= \(\frac{P\left(E / E_2\right) \cdot P\left(E_2\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)}\) [by Bayes’ theorem]

= \(\frac{\left(\frac{5}{9} \times \frac{1}{2}\right)}{\left(\frac{3}{5} \times \frac{1}{2}\right)+\left(\frac{5}{9} \times \frac{1}{2}\right)}=\frac{25}{52}\).

Hence, the required probability is \(\frac{25}{52}\).

Example 10. There are 5 hags, each containing 5 white balls and 3 black balls. Also, there are 6 bags, each containing 2 white balls and 4 black balls. A white ball is drawn at random. Find the probability that this white ball is from a bag of the first group.
Solution:

Let E₁ = event of selecting a bag from the first group,

E₂ = event of selecting a bag from the second group, and

E = event of drawing a white ball.

Then, P(E₁) = \(\frac{5}{11}\) and P(E₂) = \(\frac{6}{11}\)

P(E/E₁) = probability of getting a white ball, given that it is from a bag of the first group = \(\frac{5}{8}\)

P(E/E₂) = probability of getting a white ball, given that it is from a bag of the second group = \(\frac{2}{6}\)= \(\frac{1}{3}\)

The probability of getting the ball from a bag of the first group, given that it is white = P(E₁/E)

= \(\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)}\) [by Bayes’ theorem]

= \(\frac{\left(\frac{5}{8} \times \frac{5}{11}\right)}{\left(\frac{5}{8} \times \frac{5}{11}\right)+\left(\frac{1}{3} \times \frac{6}{11}\right)}=\frac{75}{123}\).

Example 11. Urn A contains 1 white, 2 black, and 3 red balls; urn B contains 2 white, 1 black, and 1 red ball; and urn C contains 4 white, 5 black, and 3 red balls. One urn is chosen at random and two balls are drawn. These happen to be one white and one red. What is the probability that they come from urn A?
Solution:

Let E₁, E₂, and E₃ be the events that the balls are drawn from urn A, urn B, and urn C respectively, and let E be the event that the balls drawn are one white and one red.

Then, P(E₁) = P(E₂) = P(E₃) = \(\frac{1}{5}\)

P(E/E₁) = probability that the balls drawn are one white and one red, given that the balls are from urn A

= \(\frac{{ }^1 C_1 \times{ }^3 C_1}{{ }^6 C_2}=\frac{3}{15}=\frac{1}{5}\)

P(E/E₂) = probability that the balls drawn are one white and one red, given that the balls are from urn B

= \(\frac{{ }^2 C_1 \times{ }^1 C_1}{{ }^4 C_2}=\frac{2}{6}=\frac{1}{3} .\)

P(E/E₃) = probability that the balls drawn are one white and one red, given that the balls are from urn C

= \(\frac{{ }^4 C_1 \times{ }^3 C_1}{{ }^{12} C_2}=\frac{12}{66}=\frac{2}{11}\)

The probability that the balls drawn are from urn A, it is given that the balls drawn are one white and one red = P(E₁/E)

= \(\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)+P\left(E / E_3\right) \cdot P\left(E_3\right)}\)

[by Bayes’ theorem]

= \(\frac{\left(\frac{1}{5} \times \frac{1}{3}\right)}{\left(\frac{1}{5} \times \frac{1}{3}\right)+\left(\frac{1}{3} \times \frac{1}{3}\right)+\left(\frac{2}{11} \times \frac{1}{3}\right)}\)

= \(\left(\frac{1}{15} \times \frac{495}{118}\right)=\frac{33}{118}\).

Hence, the required probability is \(\frac{33}{118}\)

Example 12. A card from a pack of 52 cards is lost. From the remaining cards of the -pack, two cards are drawn and are found to be both spades. Find the probability of the lost card being a spade.
Solution:

Let E₁, E₂, E_3, and E₄ be the events of losing a card of spades, clubs, hearts, and diamonds respectively.

Then, P(E₁) = P(E₂) = P(E₃) = P(E₄) = \(\frac{13}{52}\) = \(\frac{1}{4}\)

Let E be the event of drawing 2 spades from the remaining 51 cards. Then,

P(E/E₁) = probability of drawing 2 spades, given that a card of spades is missing

= \(\frac{{ }^{12} C_2}{{ }^{51} C_2}=\frac{(12 \times 11)}{2 !} \times \frac{2 !}{(51 \times 50)}=\frac{22}{425}\).

P(E/E₂) = probability of drawing 2 spades, given that a card of clubs is missing

= \(\frac{{ }^{13} C_2}{{ }^{51} C_2}=\frac{(13 \times 12)}{2 !} \times \frac{2 !}{(51 \times 50)}=\frac{26}{425}\).

P(E/E₃) = probability of drawing 2 spades, given that a card of hearts is missing

= \(\frac{{ }^{13} C_2}{{ }^{51} C_2}=\frac{26}{425}\)

P(E/E₄) = probability of drawing 2 spades, given that a card of diamonds is missing

= \(\frac{{ }^{13} C_2}{{ }^{51} C_2}=\frac{26}{425}\)

∴ P(E₁/E) = probability of the lost card being a spade, given that 2 spades are drawn from the remaining 51 cards

= \(\frac{P\left(E_1\right) \cdot P\left(E / E_1\right)}{P\left(E_1\right) \cdot P\left(E / E_1\right)+P\left(E_2\right) \cdot P\left(E / E_2\right)+P\left(E_3\right) \cdot P\left(E / E_3\right)+P\left(E_4\right) \cdot P\left(E / E_4\right)}\)

= \(\frac{\left(\frac{1}{4} \times \frac{22}{425}\right)}{\left(\frac{1}{4} \times \frac{22}{425}\right)+\left(\frac{1}{4} \times \frac{26}{425}\right)+\left(\frac{1}{4} \times \frac{26}{425}\right)+\left(\frac{1}{4} \times \frac{26}{425}\right)}\)

= \(\frac{22}{100}\)=0.22.

Hence, the required probability is 0.22.

Bayes Theorem Exercise

Question 1. In a bulb factory, three machines, A, B, and C, manufacture 60%, 25%, and 15% of the total production respectively. Of their respective outputs, 1%, 2%, and 1% are defective. A bulb is drawn at random from the total product and it is found to be defective. Find the probability that it was manufactured by machine C.
Solution: 2/25

Question 2. A company manufactures scooters at two plants, A and B. Plant A produces 80% and plant B produces 20% of the total product. 85% of the scooters produced at plant A and 65% of the scooters produced at plant B are of standard quality. A scooter produced by the company is selected at random and it is found to be of standard quality. What is the probability that it was manufactured at plant A.
Solution: 68/81

Hint: Let E₁ = event that the selected scooter is produced at plant A, and

E₂ = event that the selected scooter is produced at plant B.

Then, P(E₁) = \(\frac{80}{100}\) =  \(\frac{4}{5}\)and P(E₂) = \(\frac{20}{100}\) = \(\frac{1}{5}\)

Let E be the event of choosing a scooter that is of standard quality.

The probability that the selected scooter’s standard quality

Then, \(P\left(E / E_1\right)=\frac{85}{100}=\frac{17}{20} \text {, and } P\left(E / E_2\right)=\frac{65}{100}=\frac{13}{20}\)

The probability that the selected scooter was produced at plant A, given that is is of standard quality = \(P\left(E_1 / E\right)\)

= \(\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)}\)

Question 3. In a certain college, 4% of boys and 1% of girls are taller than 1.75 metres. Furthermore, 60% of the students are girls. If a student is selected at random and is taller than 1.75 meters, what is the probability that the selected student is a girl?
Solution: 3/11

Hint: Let E₁ and E₂ be the events of selecting a boy and a girl respectively.

Then, P(E₁) = \(\frac{40}{100}\) = \(\frac{2}{5}\), and P(E₂) = \(\frac{60}{100}\) = \(\frac{3}{5}\)

Let E = event that the student selected is taller than 1.75 m.

Then, \(P\left(E / E_1\right)=\frac{4}{100}=\frac{1}{25} \text { and } P\left(E / E_2\right)=\frac{1}{100}\)

The probability that the selected student is a girl, given that she is taller than 1.75 m. = \(P\left(E_2 / E\right)\)

= \(\frac{P\left(E / E_2\right) \cdot P\left(E_2\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)}\)

Question 4. In a class, 5% of the boys and 10% of the girls have an IQ of more than 150. In this class, 60% of the students are boys. If a student is selected at random and found to have an IQ of more than 150, find the probability that the student is a boy.
Solution: 3/7

Hint: Let E₁ and E₂ be the events of selecting a boy and a girl respectively. Then,

⇒ \(P\left(E_1\right)=\frac{60}{100}=\frac{3}{5} \text {, and } P\left(E_2\right)=\frac{40}{100}=\frac{2}{5} \text {. }\)

Let E be the event of selecting a student having an IQ of more than 150.

Then, \(P\left(E / E_1\right)=\frac{5}{100}=\frac{1}{20} \text {, and } P\left(E / E_2\right)=\frac{10}{100}=\frac{1}{10} \text {. }\)

Required probability = P(E₁/E)

= \(=\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)} .\)

Question 5. Suppose 5% of men and 0.25% of women have grey hair. A grey-haired person is selected at random. What is the probability of this person being male? Assume that there are equal numbers of males and females.
Solution: 2/3

Hint: Let there be 1000 males and 1000 females.

Let E₁ and E₂ be the events of choosing a male and a female respectively.

Then, \(P\left(E_1\right)=\frac{1000}{2000}=\frac{1}{2} \text {, and } P\left(E_2\right)=\frac{1000}{2000}=\frac{1}{2} \text {. }\)

Let E be the event of choosing a grey-haired person. Then,

⇒ \(P\left(E / E_1\right)=\frac{50}{1000}=\frac{1}{20} \text {, and } P\left(E / E_2\right)=\frac{25}{1000}=\frac{1}{40} \text {. }\)

The probability of selecting a male person, given that the person selected is a grey-haired = P(E₁/E)

= \(\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)} .\)

Question 6. Two groups are competing for the positions on the board of directors of a corporation. The probabilities that the first and the second groups will win are 0.6 and 0.4 respectively. Further, if the first group wins, the probability of introducing a new product is 0.7 and when the second group wins, the corresponding probability is 0.3. Find the probability that the new product introduced was by the second group.
Solution: 2/9

Hint: Let E₁ = event that the first group wins,

E₂ = event that the second group wins, and

E = event that a new product is introduced.

Then, P(E₁) = 0.6, P(E₂) = 0.4,

P(E/E₁) = 0.7, P(E/E₂) = 0.3.

Required probability = P(E₂/E)

=\(\frac{P\left(E / E_2\right) \cdot P\left(E_2\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)} .\)

Question 7. A bag A contains 1 white and 6 red balls. Another bag contains 4 white and 3red balls. One of the bags is selected at random and a ball is drawn from it, which is found to be white. Find the probability that the ball drawn is from bag A.
Solution: 1/5

Question 8. There are two bags 1 and 2. Bag 1 contains 3 white and 4 black balls, and bag 2 contains 5 white and 6 black balls. One ball is drawn at random from one of the bags and is found to be white. Find the probability that it was drawn from bag 1.
Solution: 33/88

Question 9. A box contains 2 gold and 3 silver coins. Another box contains 3 gold and 3 silver coins. A box is chosen at random, and a coin is drawn from it. If the selected coin is a gold coin, find the probability that it was drawn from the second box.
Solution: 5/9

Question 10. Three urns A, B, and C contain 6 red and 4 white; 2 red and 6 white; and 1 red and 5 white balls respectively. An urn is chosen at random and a ball is drawn. If the ball drawn is found to be red, find the probability that the ball was drawn from the urn A.
Solution: 36/61

Hint: Let E₁, E_2, and E₃ be the events of choosing the urns A, B, and C respectively, and let £ be the event of drawing a red ball. Then,

P(E₁) = P(E₂) = P(E₃) = 1/3

⇒ \(P\left(\frac{E}{E_1}\right)=\frac{6}{10}, P\left(\frac{E}{E_2}\right)= \frac{2}{8} \text {, and } P\left(\frac{E}{E_3}\right)=\frac{1}{6}\)

Required probability = \(P\left(\frac{E_1}{E}\right)\)

= \(\frac{P\left(E_1\right) \times P\left(\frac{E}{E_1}\right)}{P\left(E_1\right) \times P\left(\frac{E}{E_1}\right)+P\left(E_2\right) \times P\left(\frac{E}{E_2}\right)+P\left(E_3\right) \times P\left(\frac{E}{E_3}\right)}\)

= \(\frac{\left(\frac{1}{3} \times \frac{6}{10}\right)}{\left(\frac{1}{3} \times \frac{6}{10}\right)+\left(\frac{1}{3} \times \frac{2}{8}\right)+\left(\frac{1}{3} \times \frac{1}{6}\right)}=\frac{36}{61}\)

Question 11. Three urns contain 2 white and 3 black balls; 3 white and 2 black balls, and 4 white and 1 black ball respectively. One ball is drawn from an urn chosen at random and it was found to be white. Find the probability that it was drawn from the first urn.
Solution: 2/9

Question 12. There are three boxes, the first one containing 1 white, 2 red, and 3 black balls; the second one containing 2 white, 3 red, and 1 black ball and the third one containing 3 white, 1 red, and 2 black balls. A box is chosen at random and from it two balls are drawn at random. One ball is red and the other, white. What is the probability that they come from the second box?
Solution: 6/11

Question 13. Urn A contains 7 white and 3 black balls; urn B contains 4 white and 6 black balls; urn C contains 2 white and 8 black balls. One of these urns is chosen at random with probabilities 0.2, 0.6 and 0.2 respectively. From the chosen urn, two balls are drawn at random without replacement. Both the balls happen to be white. Find the probability that the balls drawn are from the urn C.
Solution: 1/40

Hint: P(A) = 0.2, P(B) = 0.6 and P(Q) = 0.2.

Let E be the event that 2 white balls are drawn. Then,

P(E/A)= \(\frac{{ }^7 C_2}{{ }^{10} C_2} ; P(E / B)=\frac{{ }^4 C_2}{{ }^{10} C_2} ; P(E / C)=\frac{{ }^2 C_2}{{ }^{10} C_2}\)

∴ required probability = P(C/E)

= \(\frac{P(E / C) \cdot P(C)}{P(E / A) \cdot P(A)+P(E / B) \cdot P(B)+P(E / C) \cdot P(C)} \text {. }\)

Question 14. There are 3 bags, each containing 5 white and 3 black balls. Also, there are 2 bags, each containing 2 white and 4 black balls. A white ball is drawn at random. Find the probability that this ball is from a bag of the first group.
Solution: 45/61

Hint: Let E₁ = event of selecting a bag from the first group, and

E₂ = event of selecting a bag from the second group.

Then, P(E₁) = 3/5 and P(E₂) = 2/5

Let E = event that the ball drawn is white. Then,

P(E/E₁) = 5/8, P(E/E₂) = 2/6 = 1/3

⇒ \(P\left(E_1 / E\right)=\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)}\)

Question 15. There are four boxes, A, B, C, and D, containing marbles. A contains 1 red, 6 white, and 3 black marbles; B contains 6 red, 2 white, and 2 black marbles; C contains 8 red, 1 white, and 1 black marbles; and D contains 6 white and 4 black marbles. One of the boxes is selected at random and a single marble is drawn from it. If the marble is red, what is the probability that it was drawn from box A?
Solution: 1/15

Hint: Let E₁, E₂, E₃, E₄ be the events of selecting boxes A, B, C, and D respectively. Then,

P(E₁) = P(E₂) = P(E₃) = P(E₄) = 1/4

Let E = event that the marble drawn is red. Then,

P(E/E₁) = 1/10, P(E/E₂) = 6/10 = 3/5, P(E/E₃) = 8/10, 4/5, P(E/E₄) =0

∴ \(P\left(E_1 / E\right)=\frac{P\left(E / E_1\right) \cdot P\left(E_1\right)}{P\left(E / E_1\right) \cdot P\left(E_1\right)+P\left(E / E_2\right) \cdot P\left(E_2\right)+P\left(E / E_3\right) \cdot P\left(E_3\right)+P\left(E / E_4\right) \cdot P\left(E_4\right)} .\)

Question 16. A car manufacturing factory has two plants X and Y. Plant X manufactures 70% of the cars and Plant Y manufactures 30%. At plant X, 80% of the cars are rated of standard quality and at plant Y, 90% are rated of standard quality. A car is picked up at random and is found to be of standard quality. Find the probability that it has come from plant X.
Solution: 56/83

Hint: Let E₁ and E₂ be the events that the car is manufactured by plants X and Y respectively.

Let E be the event that the car is of standard quality. Then

⇒ \(P\left(E_1\right)=\frac{70}{100}=\frac{7}{10}, P\left(E_2\right)=\frac{30}{100}=\frac{3}{10}\) ;

⇒  \(P\left(E / E_1\right)=\frac{80}{100}=\frac{4}{5}, P\left(E / E_2\right)=\frac{90}{100}=\frac{9}{10}\)

∴ \(P\left(E_1 / E\right)=\frac{P\left(E_1\right) \times P\left(E / E_1\right)}{P\left(E_1\right) \times P\left(E / E_1\right)+P\left(E_2\right) \times P\left(E / E_2\right)}\)

= \(\frac{\left(\frac{7}{10} \times \frac{4}{5}\right)}{\left(\frac{7}{10} \times \frac{4}{5}\right)+\left(\frac{3}{10} \times \frac{9}{10}\right)}=\frac{56}{83}\)

Question 17. An insurance company insured 2000 scooters and 3000 motorcycles. The probability of an accident involving a scooter is 0.01 and that of a motorcycle is 0.02. An insured vehicle met with an accident. Find the probability that the accident vehicle was a motorcycle.
Solution: 3/4

Hint: Let E₁ and E₂ be the events that an insured vehicle is a scooter and a motorcycle respectively.

Let E be the event that the insured vehicle meets an accident.

⇒ \(P\left(E_1\right)=\frac{2000}{(2000+3000)}=\frac{2}{5}, P\left(E_2\right)=\frac{3000}{5000}=\frac{3}{5}\)

⇒ \(P\left(E / E_1\right)=0.01 \text { and } P\left(E / E_2\right)=0.02\)

∴ \(P\left(E_2 / E\right)=\frac{P\left(E_2\right) \cdot P\left(E / E_2\right)}{P\left(E_1\right) \cdot P\left(E / E_1\right)+P\left(E_2\right) \cdot P\left(E / E_2\right)}\)

= \(\frac{\left(\frac{3}{5} \times 0.02\right)}{\left(\frac{2}{5} \times 0.01\right)+\left(\frac{3}{5} \times 0.02\right)}=\frac{3}{4}\)

Question 18. In a bulb factory, machines A, B, and C manufacture 60%, 30%, and 10% of bulbs respectively. Out of these bulbs 1%, 2%, and 3% of the bulbs produced respectively by A, B, and C are found to be defective. A bulb is picked up at random from the total production and found to be defective. Find the probability that this bulb was produced by machine A.
Solution: 2/5

 

Probability

Conditional Probability And Probability Of Independent Events

In this chapter, we shall be dealing with problems based on conditional probability and probability of independent events.

Conditional Probability

Let A and B be two events associated with the same random experiment. Then, the probability of occurrence of A under the condition B has already occurred and P(B) ^ 0, is called conditional probability, denoted by P(A/B).

We define:

⇒ \(P(A / B)=\frac{P(A \cap B)}{P(B)}, \text { where } P(B) \neq 0\)

and \(P(B / A)=\frac{P(B \cap A)}{P(A)}=\frac{P(A \cap B)}{P(A)}, \text { where } P(A) \neq 0\).

Read and Learn More WBCHSE Solutions For Class 12 Maths

Conditional Probability Solved Examples

Example 1. A die is rolled. If the outcome is an odd number, what is the probability that it is prime?
Solution:

When a die is rolled, the sample space is given by S = { 1,2,3,4, 5,6}.

Let A = event of getting a prime number, and

B = event of getting an odd number.

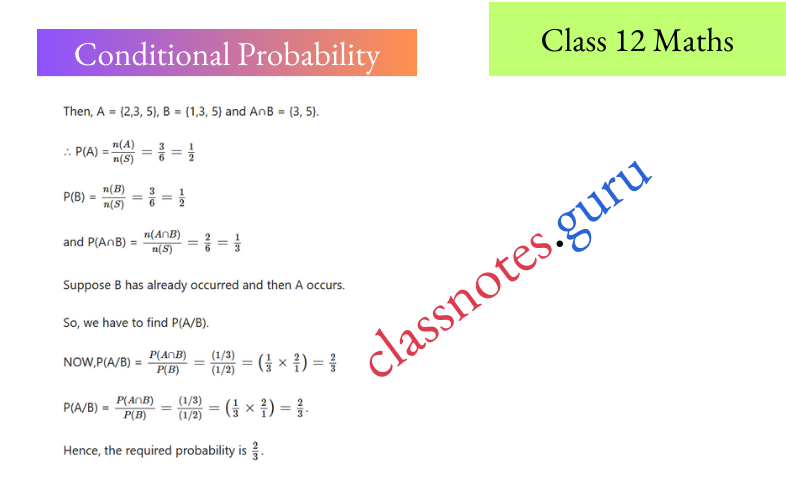
Example 2. Ten cards numbered 1 to 10 are placed in a box, mixed up thoroughly and then one card is drawn randomly. If it is known that the number on the drawn card is more than 3, what is the probability that it is an even number?
Solution:

Clearly, the sample space is S = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}.

Let A =event that the number on the drawn card is even, and
B =event that the number on the drawn card is more than 3.

Then A ={2, 4, 6, 8, 10}, B ={4, 5, 6, 7, 8, 9, 10} and A ∩ B ={4,6, 8, 10}.

∴ P(A) = \(\frac{n(A)}{n(S)}=\frac{5}{10}=\frac{1}{2}\),

P(B)= \(\frac{n(B)}{n(S)}=\frac{7}{10}\) and

P(A∩B)= \(\frac{n(A \cap B)}{n(S)}=\frac{4}{10}=\frac{2}{5}\)

Suppose B has already occurred and then A occurs.

So, we have to find P(A/B).

Now, P(A/B) = \(\frac{P(A \cap B)}{P(B)}=\frac{(2 / 5)}{(7 / 10)}=\left(\frac{2}{5} \times \frac{10}{7}\right)=\frac{4}{7}\).

Hence, the required probability is \(\frac{4}{7}\).

Example 3. A die is thrown twice and the sum of the numbers appearing is observed to be 6. What is the conditional probability that the number 4 has appeared at least once?
Solution:

We know that when a die is thrown twice, then the sample space has 36 possible outcomes.

Let A = event that 4 appears at least once, and
B = event that the sum of the numbers appearing is 6.

Then, A = {(4,1), (4,2), (4,3), (4,4), (4, 5), (4,6), (1,4), (2,4), (3,4), (5,4), (6,4)}

and B = {(1,5), (2,4), (3,3), (4,2), (5,1)}

∴ A∩B = {(2,4), (4,2)}.

So, P(A) = \(\frac{n(A)}{n(S)}=\frac{11}{36}, P(B)=\frac{n(B)}{n(S)}=\frac{5}{36}\)

P(B) = \(\frac{n(B)}{n(S)}=\frac{5}{36}\)

and P(A∩B)= \(\frac{n(A \cap B)}{n(S)}=\frac{2}{36}=\frac{1}{18}\)

Suppose B has already occurred and then A occurs.

So, we have to find P(A/B).

Now, P(A B) = \(\frac{P(A \cap B)}{P(B)}=\frac{(1 / 18)}{(5 / 36)}=\left(\frac{1}{18} \times \frac{36}{5}\right)=\frac{2}{5} \text {. }\)

Hence, the required probability is \(\frac{2}{5}\)

Example 4. Assume that each born child is equally likely to be a boy or a girl. If a family has two children, what is the conditional probability that both are girls given that

1. The youngest child is a girl,
2. At least one of the children is a girl.

Solution:

We may write the sample space as

S = {G₁G₂, G₁B₂, B₁G₂, B₁B₂}, where the youngest child appears later,

1. Let A = event that both the children are girls and B = event that the youngest child is a girl.

Then, A = {G₁G₂}, B = {G₁G₂, B₁G₂} and A∩B = {G₁G₂}.

∴ P(A)= \(\frac{n(A)}{n(S)}=\frac{1}{4}\), \(P(B)=\frac{n(B)}{n(S)}=\frac{2}{4}=\frac{1}{2}\)

and P(A∩B) = \(\frac{n(A \cap B)}{n(S)}=\frac{1}{4}\)

Suppose B has already occurred and then A occurs.

So, we have to find P(A/B).

Now, \(P(A / B)=\frac{P(A \cap B)}{P(B)}=\frac{(1 / 4)}{(1 / 2)}=\left(\frac{1}{4} \times \frac{2}{1}\right)=\frac{1}{2}\).

Hence, the required probability is \(\frac{1}{2}\).

2. Let A = event that both the children are girls, and

E = event that at least one of the children is a girl.

Then, A = {G₁G₂}, E = {G₁B₂, B₁G₂, G₁G₂} and A∩E = {G₁G₂}.

∴ P(A)= \(\frac{n(A)}{n(S)}=\frac{1}{4}\), P(E)= \(\frac{n(E)}{n(S)}=\frac{3}{4}\)

and \(P(A \cap E)=\frac{n(A \cap E)}{n(S)}=\frac{1}{4}\).

Suppose E has already occurred and then A occurs.

So, we have to find P(A/E).

Now, \(P(A / E)=\frac{P(A \cap E)}{P(E)}=\frac{(1 / 4)}{(3 / 4)}=\left(\frac{1}{4} \times \frac{4}{3}\right)=\frac{1}{3}\)

Hence, the required probability is 1/3.

Example 5. An instructor has a question bank consisting of 300 easy true/false questions; 200 difficult true/false questions; 500 easy multiple-choice questions and 400 difficult multiple-choice questions. If a question is selected at random from the question bank, what is the probability that it will be an easy question given that it is a multiple-choice question?
Solution:

Clearly, the sample space consists of 1400 questions.

∴ n(S) = 1400.

Let A = event of selecting an easy question, and

B = event of selecting a multiple-choice question.

Then, A∩B = event of selecting an easy multiple-choice question.

∴ n(A) = (300 +500) = 800, n(B) = (500 +400) = 900 and n(A∩B) = 500.

So, P(A) = \(\frac{n(A)}{n(S)}=\frac{800}{1400}=\frac{4}{7}\), P(B)= \(\frac{n(B)}{n(S)}=\frac{900}{1400}=\frac{9}{14}\)

and \(P(A \cap B)=\frac{n(A \cap B)}{n(S)}=\frac{500}{1400}=\frac{5}{14} \text {. }\)

Hence, the required probability is \(\frac{5}{14}\)

Suppose B has already occurred and then A occurs.

Thus we have to find P(A/B)

Now, P(A/B)= \(\frac{P(A \cap B)}{P(B)}=\frac{(5 / 14)}{(9 / 14)}=\left(\frac{5}{14} \times \frac{14}{9}\right)=\frac{5}{9}\).

Hence, the required probability is \(\frac{5}{9}\).

Example 6. Two numbers are selected at random from the integers 1 through 9. If the sum is even, find the probability that both the numbers are odd.
Solution:

Out of the numbers from 1 to 9, there are 5 odd numbers and 4 even numbers.

Let A = event of choosing two odd numbers, and

B = event of choosing two numbers whose sum is even.

Then, n(A) = number of ways of choosing 2 odd numbers out of = ⁵C₂

n(B) = number of ways of choosing 2 numbers whose sum is even

= (⁴C₂ + ⁵C₂) [2 out of 4 even and 2 out of 5 odd].

n(A∩B) = number of ways of choosing 2 odd numbers out of 5 = ⁵C₂.

Suppose B has already occurred and then A occurs.

Then, we have to find P(A/B).

Now, P(A/B) = \(\frac{P(A \cap B)}{P(B)}=\frac{n(A \cap B)}{n(B)}=\frac{{ }^5 C_2}{{ }^4 C_2+{ }^5 C_2}\)

= \(\frac{5 \times 4}{2} \times \frac{2}{(4 \times 3+5 \times 4)}=\frac{20}{32}=\frac{5}{8}\) .

Hence the required probability is \(\frac{5}{8}\)

Properties Of Conditional Probability

Theorem 1. Let A and B be events of a sample space S of an experiment. Then, prove thatP(S/B) =P(B/B) -1.

Proof: We know that:

P(S/B) = \(\frac{P(S \cap B)}{P(B)}=\frac{P(B)}{P(B)}=1[because S \cap B=B]\)

And, \(P(B / B)=\frac{P(B \cap B)}{P(B)}=\frac{P(B)}{P(B)}=1\).

Hence, P(S/B) -P(B/B) =1.

Theorem 2. Let A and B be two events of a sample space S and let E be an event such that P(E) ≠ 0. Then, prove that P[(A∪B)/E] =P(A/E) +P(B/E) -P[(A∩B)/E].

Proof: We have

P[(A∪B)/E] = \(\frac{P[(A \cup B) \cap E]}{P(E)}\)

= \(\frac{P[(A \cap E) \cup(B \cap E)]}{P(E)}\)

= \(\frac{P(A \cap E)+P(B \cap E)-P[(A \cap E) \cap(B \cap E)]}{P(E)}\)

= \(\frac{P(A \cap E)+P(B \cap E)-P(A \cap B \cap E)}{P(E)}\)

= \(\frac{P(A \cap E)}{P(E)}+\frac{P(B \cap E)}{P(E)}-\frac{P[(A \cap B) \cap E]}{P(E)}\)

= \(P(A / E)+P(B / E)-P[(A \cap B) / E]\).

Hence, \(P(A / E)+P(B / E)-P[(A \cap B) / E]\)

Corollary: If A and B are disjoint events, prove that P[(A∪B)/E] =P(A/E)+P(B/E).

Proof: For any events A and B, we have

P[(A∪B)/E] = P(A/E) +P(B/E) -P[(A∩B)/E].

If A and B are disjoint, then P[(A∩B)/E] = 0.

Hence, in this case, we have P[(A∪B)/E] = P(A/E) +P(B/E).

Theorem 3. For any events A and B of a sample space S, proue Bird P(\(\bar{A}\)/B) = 1 -P(A/B), where \(\bar{A}\) denotes ‘not A’.

Proof: We know that

P(S/B) =1

⇒ P[(Au\(\bar{A}\))/B] =1 [S = \(A \cup \bar{A}]\)]

⇒ P(A/B)+ P(A/B)=1 [A \(\bar{A}\)= ]

⇒ P(\(\bar{A}\)/B) = 1-P(A/B).

Hence, P(\(\bar{A}\)/B) = 1-P(A/B).

Example 1. If A end B are two events such that P(A) = \(\frac{3}{5}\), P(B)= \(\frac{7}{10}\) and P(A∪B) = \(\frac{9}{10}\), then find

1. P(A∩B)
2. P(A/B)
3. P(B/A).

Solution:

1. We know that P(A∩B) =P(A) +P(B) -P(A∪B)

= \(\left(\frac{3}{5}+\frac{7}{10}-\frac{9}{10}\right)=\frac{(6+7-9)}{10}=\frac{4}{10}=\frac{2}{5}\) .

2. \(P(A / B)=\frac{(A \cap B)}{P(B)}=\frac{(2 / 5)}{(7 / 10)}=\left(\frac{2}{5} \times \frac{10}{7}\right)=\frac{4}{7}\).

3. \(P(B / A)=\frac{(B \cap A)}{P(A)}=\frac{P(A \cap B)}{P(A)}=\left(\frac{2 / 5}{3 / 5}\right)=\left(\frac{2}{5} \times \frac{5}{3}\right)=\frac{2}{3}\).

Example 2. Evaluate P(A∪B), if 2P(A) = P(B) = \(\frac{6}{13}\) and P(A/B) = \(\frac{1}{3}\)
Solution:

2P(A) = P(B) = \(\frac{6}{13}\)

⇒ P(A) = \(\frac{3}{13}\) and P(B) = \(\frac{6}{13}\)

∴ P(A/B) = \(\frac{P(A \cap B)}{P(B)}\)

⇒ \(P(A \cap B)=P(A / B) \cdot P(B)=\left(\frac{1}{3} \times \frac{6}{13}\right)=\frac{2}{13}\).

So, P(A∪B) = P(A) + P(B) – P(A∩B)

= \(\left(\frac{3}{13}+\frac{6}{13}-\frac{2}{13}\right)=\frac{(3+6-2)}{13}=\frac{7}{13} .\)

Hence, P(A∪B) = \(\frac{7}{13}\)

Example 3. Let A and B be events such that P(A) = \(\frac{1}{3}\),P(B) = \(\frac{1}{4}\) and P(A∩B) = \(\frac{1}{5}\) Find:

1. P(A/B)
2. P(B/A)
3. P(A∪B)
4. P(B/A)

Solution:

We have:

1. P(A/B) = \(\frac{P(A \cap B)}{P(B)}=\frac{(1 / 5)}{(1 / 4)}=\left(\frac{1}{5} \times \frac{4}{1}\right)=\frac{4}{5}\).

2. P(B/A) = \(\frac{P(B \cap A)}{P(A)}=\frac{P(A \cap B)}{P(A)}=\frac{(1 / 5)}{(1 / 3)}=\left(\frac{1}{5} \times \frac{3}{1}\right)=\frac{3}{5}\)

3. \(P(A \cup B)\) = \(P(A)+P(B)-P(A \cap B)\)

= \(\left(\frac{1}{3}+\frac{1}{4}-\frac{1}{5}\right)=\frac{(20+15-12)}{60}=\frac{23}{60}\)

4. \(P(\bar{B} / \bar{A})=\frac{P(\bar{B} \cap \bar{A})}{P(\bar{A})}=\frac{P(\bar{A} \cap \bar{B})}{P(\bar{A})}=\frac{P(\overline{A \cup B})}{P(\bar{A})}\)

= \(\frac{1-P(A \cup B)}{1-P(A)}=\frac{\left(1-\frac{23}{60}\right)}{\left(1-\frac{1}{3}\right)}=\frac{(37 / 60)}{(2 / 3)}=\left(\frac{37}{60} \times \frac{3}{2}\right)=\frac{37}{40}\).

Conditional Probability Exercise 1A

Question 1. Let A and B be events such that P(A) = \(\frac{7}{13}\), P(B)= \(\frac{9}{13}\) and P(A∩B)= \(\frac{4}{13}\) Find

1. P(A/B)
2. P(B/A)
3. P(A∪B)
4. \(P(\bar{A} / \bar{B}).\)

Solution:

1. 4/9
2. 4/7
3. 12/13
4. 1/6

Question 2. Let A and B be events such that P(A)= \(\frac{5}{11}\) ,P(B)= \(\frac{6}{11}\) and P(A∪B)= \(\frac{7}{11}\). Find

1. P(A∩B)
2. P(A/B)
3. P(B/A)
4. \(P(\bar{A} / \bar{B})\)

Solution:

1. 4/11
2. 2/3
3. 4/5
4. 4/5

Question 3. Let A and B be events such that P(A) = \(\frac{3}{10}\), P(B) = \(\frac{1}{2}\) and P(B/A) = \(\frac{2}{5}\) Find

1. P(A∩B)
2. P(A∪B)
3. P(A/B).

Solution:

1. 3/25
2. 17/25
3. 6/25

Question 4. Let A and B be events such that 2P(A) = P(B) = \(\frac{5}{13}\) and P(A/B) = \(\frac{2}{5}\) Find

1. P(A∩B)
2. P(A∪B)

Solution:

1. 2/13
2. 11/26

Question 5. A die is rolled. If the outcome is an even number, what is the probability that it is a number greater than 2?
Solution: 2/3

Question 6. A coin is tossed twice. If the outcome is at most one tail, what is the probability that both head and tail have appeared?
Solution: 2/3

Question 7. Three coins are tossed simultaneously. Find the probability that all coins show heads if at least one of the coins shows a head.
Solution: 1/7

Question 8. Two unbiased dice are thrown. Find the probability that the sum of the numbers appearing is 8 or greater if 4 appears on the first die.
Solution: 1/2

Hint: Let A =event of getting the sum 8 or greater, and

B = event of getting a 4 on the first die.

∴ A ={(2, 6),(3, 5), (3, 6),(4, 4), (4, 5),(4, 6), (5, 3),(5, 4), (5, 5),(5, 6), (6,2),(6, 3), (6, 4),(6, 5), (6, 6)}

B ={(4,1), (4, 2),(4, 3), (4, 4), (4, 5), (4, 6)}.

(A∩B) ={(4,4), (4, 5), (4, 6)}.

∴ n(A) =15, n(B) =6 and n(A∩B) =3.

Hence, the required probability = P(A/B) = \(\frac{n(A \cap B)}{n(B)}=\frac{3}{6}=\frac{1}{2}\)

Question 9. A die is thrown twice and the sum of the numbers appearing is observed to be 8. What is the conditional probability that the number 5 has appeared at least once?
Solution: 2/5

Question 10. Two dice were thrown and it is known that the numbers which come up were different. Find the probability that the sum of the two numbers was 5.
Solution: 2/15

Hint: Let A =event that the sum of two numbers appeared is 5, and

B =event event that the two dice show different numbers.

Then, A ={(2, 3), (3, 2), (1, 4), (4,1)}.

Thus, n(A) =4, n(B) =30, A∩B=A and so n(A n B) =n(A) =4

∴ the required probability = P(A/B) = \(\frac{n(A \cap B)}{n(B)}=\frac{4}{30}=\frac{2}{15}.\)

Question 11. A coin is tossed and then a die is thrown. Find the probability of obtaining a 6, given that a head came up.
Solution: 1/6

Let A =event that the die shows a 6, and

B =event that a head comes up.

Then, A ={(H, 6), (T, 6)}

and B ={(H,1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6)}.

Then, P(A/B) = \(\frac{n(A \cap B)}{n(B)}=\frac{1}{6}\).

Question 12. A couple has 2 children. Find the probability that both are boys if it is known that

1. One of the children is a boy, and
2. The elder child is a boy.

Solution:

1. 1/3
2. 1/2

Question 13. In a class, 40% of students study mathematics; 25% study biology, and 15% study both mathematics and biology. One student is selected at random. Find the probability that

1. He studies mathematics if it is known that he studies biology
2. He studies biology if it is known that he studies mathematics.

Solution:

1. 3/5
2. 3/8

Hint:

Let M =event of studying mathematics, and

B =event of studying biology.

Then P(M)= \(\frac{40}{100}\) = \(\frac{2}{5}\), P(B)= \(\frac{25}{100}\) = \(\frac{1}{4}\) and P(M nB)= \(\frac{15}{100}\) = \(\frac{3}{20}\)

1. P(M/B) = \(\frac{P(M \cap B)}{P(B)}\)
2. P(B/M) = \(\frac{P(M \cap B)}{P(M)}\)

Question 14. The probability that a student selected at random from a class will pass in Hindi is 4/5 and the probability that he passes in Hindi and English is 1/2. What is the probability that he will pass in English if it is known that he has passed in Hindi?
Solution: 5/8

Hint: Given, P(H)= \(\frac{4}{5}\) and P(H∩E)= \(\frac{1}{2}\)

∴ P(E/H) = \(\frac{P(E \cap H)}{P(H)}=\frac{P(H \cap E)}{P(H)} \text {. }\)

Question 15. The probability that a certain person will buy a shirt is 0.2, the probability that he will buy a coat is 0.3 and the probability that he will buy a shirt given that he buys a coat is 0.4. Find the probability that he will buy both a shirt and a coat.
Solution: 0.12

Hint: P(S) = 02, P(C) = 0.3, P(S/C)= 0.4.

∴ P(S/C) = \(\frac{P(S \cap C)}{P(C)}\)

⇒ P(S∩C)=P(C)·P(S/C).

Question 16. In a hostel, 60% of the students read Hindi newspapers, 40% read English newspapers and 20% read both Hindi and English newspapers. A student is selected at random.

1. Find the probability that he reads neither Hindi nor English newspapers.
2. If he reads Hindi newspaper, what is the probability that he reads English newspaper?
3. If he reads an English newspaper, what is the probability that he reads Hindi newspaper?

Solution:

1. 1/5
2. 1/3
3. 1/2

Question 17. Two integers are selected at random from integers 1 through 11. If the sum is even, find the probability that both the numbers selected are odd.
Solution: 3/5

Hint: Let A =event of choosing both odd numbers,

B =event that sum of chosen numbers is even.

In integers from 1 to 11, there are 5 even and 6 odd integers.

⇒ \(P(A)=\frac{{ }^6 C_2}{{ }^{11} C_2}=\frac{3}{11}, P(B)=\left(\frac{{ }^6 C_2+{ }^5 C_2}{{ }^{11} C_2}\right)=\frac{5}{11}, P(A \cap B)=\frac{{ }^6 C_2}{{ }^{11} C_2}=\frac{3}{11}\)

∴ required probability = P(A/B) = \(\frac{P(A \cap B)}{P(B)}\).

Multiplication Theorem On Probability

Let A and B be two events associated with a sample space S. Then, the simultaneous occurrence of two events A and B is denoted by (A∩B) and also written as AB

Multiplication Theorem: Let A and B be two events associated with a sample space S. Then, prove that

P(AB) = P(A∩B) =P(A)·P(B/A) =P(B)·P(A/B), provided P(A)≠0 and P(B)≠ 0.

Proof: For any events A and B, we have

P(A/B) = \(\frac{P(A \cap B)}{P(B)}\), where P(A)≠0.

∴ P(A∩B)=P(B)·P(A/B)….. (1)

Again, P(B/A) = \(\frac{P(B \cap A)}{P(A)}=\frac{P(A \cap B)}{P(A)}\) where P(B)≠0.

∴ P(A∩B) =P(A)·P(B/A)…….(2)

From (1) and (2), we get

P(A∩B) =P(B)·P(A/B) = P(A)·P(A/B), where P(A)≠0 and P(A)≠0.

Multiplication Rule for Three Events: For any three events A, B, and C of the same sample space, we have P(A∩B∩C) = P(A)·P(B/A)·P[C/(A∩B)]

= P(A)·P(B/A)·(C/AB).

This rule can be extended for four or more events.

Multiplication Theorem On Probability Solved Examples

Example 1. An urn contains 8 white and 4 red balls. Two balls are drawn from the urn one after the other without replacement. What is the probability that both drawn balls are white?
Solution:

Let A and B denote respectively the events that the first and second balls both drawn are white.

Then, we have to find P(A∩B).

Now, P(A) = P (white ball in the first draw) = \(\frac{8}{12}\).

After the occurrence of event A, we are left with 7 white and 4 red balls.

The probability of drawing second white ball, given that the first ball drawn is white, is clearly the conditional probability of occurrence of B, given that A has occurred.

∴ P(B/A) = \(\frac{7}{11}\).

By multiplication rule of probability, we have \(P(A \cap B)=P(A) \cdot P(B / A)=\left(\frac{8}{12} \times \frac{7}{11}\right)=\frac{14}{33}\)

Hence, the required probability is \(\frac{14}{33}\)

Example 2. Three cards are drawn successively without replacement from a pack of 52 well-shuffled cards. What is the probability that first two cards are queens and the third card drawn is a king?
Solution:

Let Q denote the event that the card drawn is a queen and K be the event that the card drawn is a king. Then, we have to find P(QQK).

Probability of drawing first queen is P(Q)= \(\frac{4}{52}\)

Now, there are 3 queens in the remaining 51 cards.

Let P(Q/Q) be the probability of getting the second queen with the condition that one queen has already been drawn.

∴ P(Q/Q)= \(\frac{3}{51}\)

Lastly, P(K/QQ) is the probability of a third drawn card to be a king, with the condition that two queens have already been drawn.

Now, there are 4 kings in remaining 50 cards.

∴ P(K/QQ)= \(\frac{4}{50}\)

By multiplication law of probability, we have P(QQK)=P(Q∩Q∩K)

=P(Q)·P(Q/Q)·P(K/QQ)

= \(\left(\frac{4}{52} \times \frac{3}{51} \times \frac{4}{50}\right)=\left(\frac{1}{13} \times \frac{1}{17} \times \frac{2}{25}\right)=\frac{2}{5525}\)

Hence, the required probability is \(\frac{2}{5525}\)

Independent Events: Two events A and B are said to be independent if

P(A/B) = P(A), where P(B)≠0 and P(B/A) = P(B), where P(A)≠0.

i.e., event A does not depend on the occurence of event B and vice versa.

Condition for Independence of Two Events: For any two events A and B, we have

P(A∩B) = P(A)·P(B/A)…. (1)

If A and B are independent, we have P(B/A) = P(B).

∴ (1) becomes P(A∩B) = P(A) xP(B).

Thus, two events A and B associated with the same random experiment are said to be independent if P(A∩B) =P(A)xP(B).

Note: Two events A and B are said to be dependent if they are not independent, i.e., if P(A∩B)≠P(A)xP(B).

Difference between Two Mutually Exclusive and Independent Events: Two events A and B are said to be mutually exclusive if A∩B = φ and in this case P(A∩B) -P(φ) =0.

Also, we know that two events A and B are independent if P(A nB)= P(A) xP(B).

Clearly, two independent events with nonzero probabilities cannot be mutually exclusive.

Also, two mutually exclusive events with nonzero probabilities cannot be mutually independent.

Three events A, B and C are said to be mutually independent, if

P(A∩B) =P(A) x P(B), P(A∩C) =P(A) x P(C), P(B∩C) =P(B) x P(C) and P(A∩B ∩C) =P(A) x P(B) x P(C).

If at least one of the above is not true for three given events A, B and C, then we say that these events are not independent.

Example 3. Let E₁ and E₂ be two events such that P(E₁) = 0.3, P(\(E_1 \cup E_2\)) =0.4 and P(E₂) = x. Find the value of x such that 

1. E₁ and E₂ are mutually exclusive,
2. E₁ and E₂ are independent.

Solution:

Let E₁ and E₂ be mutually exclusive. Then, E₁ ∩ E₂ = φ.

∴ P(E₁ ∩ E₂)=P(E₁)+P(E₂)

⇒ 0.4 = 0.3+x

⇒ x = 0.1.

Thus, when E₁ and E₂ are mutually exclusive, then x = 0.1.

2. Let E₁ and E₂ be two independent events. Then,

P(E₁ ∩ E₂) =P(E₁) X P(E₂) = 0.3 x x = 0.3x.

∴ P(\(E_1 \cup E_2\)) =P(E₁)+P(E₂)-P(E₁∩E₂)

⇒ 0.4 =0.3+ x-0.3x

⇒ 0.7x = 0.1

⇒ x =\(=\frac{0.1}{0.7}=\frac{1}{7} .\)

Thus, when E₁ and E₂ are independent, then A = \(\frac{1}{7} .\)

Example 4. Let E₁ and E₂ are two independent events such that P(E₁) =0.35 and P(\(E_1 \cup E_2\)) = 0.60,find P(E₂).
Solution:

Let P(E₂)= A.

Then, E₁ and E₂ being independent events, we have

P(E₁∩E₂) =P(E₁) X P(E₂) = 0.35 X x = 0.35x.

Now, P(E₁∪E₂)=P(E₁)+P(E₂)-P(E₁∩E₂)

⇒ 0.60 =0.35 + x – 0.35x

⇒ 0.65A =0.25

⇒ x = \(\frac{0.25}{0.65}=\frac{25}{65}=\frac{5}{13}\) .

Hence, \(P\left(E_2\right)=\frac{5}{13}\).

Example 5. A coin is tossed thrice. Let the event E be ‘the first throw results in a head’, and the event F be ‘the last throw results in a tail’. Find whether the events E and F are independent.
Solution:

When a coin is tossed three times, the sample space is given by S = [HHH, HHT, HTH, THH, TTH, TUT, HTT, TTT}.

Now, E = event that the first throw results in a head.

∴ E = {HHH, HHT, HTH, HTT}.

And, F = event that the last throw results in a tail.

∴ F = {HHT, THT, HTT, TIT}.

So, (E∩F) = {HHT, HIT}.

Clearly, n(E) = 4, n(F) = 4, n(E nF) = 2 and n(S) = 8.

∴ P(E)= \(\frac{n(E)}{n(S)}=\frac{4}{8}=\frac{1}{2}, P(F)=\frac{n(F)}{n(S)}=\frac{4}{8}=\frac{1}{2}\)

and \(P(E \cap F)\)=\(\frac{n(E \cap F)}{n(S)}\)=\(\frac{2}{8}\)=\(\frac{1}{4}\)

Thus, P(E∩F) = P(E )x P(F).

Hence, E and F are independent events.

Example 6. An unbiased die is tossed twice. Find the probability of getting a 4, 5 or 6 on the first toss and a 1, 2,3 or 4 on the second toss.
Solution:

In each case, the sample space is given by S = {1,2,3,4,5,6}.

Let E = event of getting a 4,5 or 6 on the first toss.

And, F = event of getting a 1,2,3 or 4 on the second toss.

Then, P(E) = \(\frac{3}{6}\) = \(\frac{1}{2}\) and P(F)= \(\frac{4}{6}\) = \(\frac{2}{3}\)

Clearly, E and F are independent events.

∴ required probability=P(E∩F) = P(E) x P(F)

[E and F are independent]

= \(\left(\frac{1}{2} \times \frac{2}{3}\right)=\frac{1}{3}\)

Example 7. Ramesh appears for an interview for two posts, A and B, for which the selection is independent. The probability for his selection for Post A is (1/6) and for Post B, it is (1/7). Find the probability that Ramesh is selected for at least one post.
Solution:

Let E₁ = event that Ramesh is selected for tire post A, and

E₂ = event that Ramesh is selected for the post B.

Then, \(P\left(E_1\right)=\frac{1}{6} \text { and } P\left(E_2\right)=\frac{1}{7}\)

Clearly, E₁ and E₂ are independent events.

∴ \(P\left(E_1 \cap E_2\right)=P\left(E_1\right) \times P\left(E_2\right)=\left(\frac{1}{6} \times \frac{1}{7}\right)=\frac{1}{42}\).

∴ P(Ramesh is selected for at least one post) =P(E₁∪E₂)

= P(E₁)+P(E₂)-P(E₁∩E₂)

= \(\left(\frac{1}{6}+\frac{1}{7}-\frac{1}{42}\right)=\frac{12}{42}=\frac{2}{7}\).

Hence, the required probability is \(\frac{2}{7}\)

Example 8. A can solve 90% of the problems given in a book, and B can solve 70%. What is the probability that at least one of them will solve a problem selected at random from the book?
Solution:

Let E₁ =event that A solves the problem,

and E₂ =event that B solves the problem.

Then, \(P\left(E_1\right)=\frac{90}{100}=\frac{9}{10} \text { and } P\left(E_2\right)=\frac{70}{100}=\frac{7}{10}\)

Clearly, E₁ and E₂ are independent events.

∴ \(P\left(E_1 \cap E_2\right)=P\left(E_1\right) \times P\left(E_2\right)=\left(\frac{9}{10} \times \frac{7}{10}\right)=\frac{63}{100}\).

∴ P(at least one of them will solve the problem)

= P(E₁∪E₂)

= P(E₁)+P(E₂)-P(E₁∩E₂)

= \(\left(\frac{9}{10}+\frac{7}{10}-\frac{63}{100}\right)=\frac{(90+70-63)}{100}=\frac{97}{100}\)

Hence, the required probability is 0.97.

Example 9. The probability that A hits a target is (1/3) and the probability that B hits it is (2/5). What is the probability that the target will be hit if both A and B shoot at it?
Solution:

Let E₁ =event that A hits the target,

and E₂ =event that B hits the target.

Then, \(P\left(E_1\right)=\frac{1}{3} \text { and } P\left(E_2\right)=\frac{2}{5} \text {. }\)

Clearly, E₁ and E₂ are independent events.

∴ \(P\left(E_1 \cap E_2\right)=P\left(E_1\right) \times P\left(E_2\right)=\left(\frac{1}{3} \times \frac{2}{5}\right)=\frac{2}{15}\)

∴ P(target is hit) = P(A hits or B hits)

= P(E₁ ∪E₂)

= P(E₁)+P(E₂)-P(E_1∩E₂)

Hence, the required probability is \(\frac{2}{15}\)

Example 10. A and B appear for an interview for two posts. The probability of A’s selection is (1/3) and that of B’s selection is (2/5). Find the probability that only one of them will be selected.
Solution:

Let E₁ = event that A is selected, and E₂ = event that B is selected.

Then, P(E₁)= \(\frac{1}{3}\) and P(E₂) = \(\frac{2}{5}\)

⇒ \(P\left(\bar{E}_1\right)=\left(1-\frac{1}{3}\right)=\frac{2}{3} \text { and } P\left(\bar{E}_2\right)=\left(1-\frac{2}{5}\right)=\frac{3}{5}\)

∴ P(event that only one of them is selected)

=  P[(E₁ and not E₂) or (E₂ and not E₁]

= \(P\left(E_1 \cap \bar{E}_2\right)+P\left(E_2 \cap \bar{E}_1\right) \quad\left[because \quad\left(E_1 \cap \bar{E}_2\right) \cap\left(E_2 \cap \bar{E}_1\right)=\phi\right]\)

= \(P\left(E_1\right) \cdot P\left(\bar{E}_2\right)+P\left(E_2\right) \cdot P\left(\bar{E}_1\right)\)

[because \(E_1 and \bar{E}_2\) are independent, and \(E_1 and \bar{E}_1\) are independent]

= \(\left(\frac{1}{3} \times \frac{3}{5}\right)+\left(\frac{2}{5} \times \frac{2}{3}\right)\)

= \(\left(\frac{1}{5}+\frac{4}{15}\right)=\frac{7}{15}\)

Example 11. A speaks the truth in 60% of the cases, and B m 90% o/ f/zc cases. In what percentage of cases are they likely to contradict each other in stating the same fact?
Solution:

Let E₁ = event that A speaks the truth, and E₂ = event that B speaks the truth.

Then, \(\bar{E}_1\) = event that A tells a lie,

and \(\bar{E}_2\) = event that B tells a lie.

Clearly, E₁ and E₂ are independent events.

Also, (E₁ and \(\bar{E}_2\)) as well as (\(\bar{E}_1\) and E₂) are independent.

Now, \(P\left(E_1\right)=\frac{60}{100}=\frac{3}{5} ; P\left(E_2\right)=\frac{90}{100}=\frac{9}{10} ;\)

⇒ \(P\left(\bar{E}_1\right)=\left(1-\frac{3}{5}\right)=\frac{2}{5} \text { and } P\left(\bar{E}_2\right)=\left(1-\frac{9}{10}\right)=\frac{1}{10}\).

∴ P(A and B contradict each other)

= P[(A speaks the truth and B tells a lie) or (A tells a lie and B speaks the truth)]

= \(P\left[\left(E_1 \cap \bar{E}_2\right) \cup\left(\bar{E}_1 \cap E_2\right)\right]\)

= \(P\left(E_1 \cap \bar{E}_2\right)+P\left(\bar{E}_1 \cap E_2\right) \quad\left[because \quad\left(E_1 \cap \bar{E}_2\right) \cap\left(\bar{E}_1 \cap E_2\right)=\phi\right]\)

= \(\left\{P\left(E_1\right) \times P\left(\bar{E}_2\right)\right\}+\left\{P\left(\bar{E}_1\right) \times P\left(E_2\right)\right\}\)

= \(\left(\frac{3}{5} \times \frac{1}{10}\right)+\left(\frac{2}{5} \times \frac{9}{10}\right)=\left(\frac{3}{50}+\frac{18}{50}\right)=\frac{21}{50}\).

Percentage of cases in which A and B Contradict each other = \(\left(\frac{21}{50} \times 100\right) \%\) = 42%

Example 12. The probabilities of a specific problem being solved independently by A and B are 1/2 and 1/3 respectively. If both try to solve the problem independently, find the probability that

1. The problem is solved
2. Exactly one of them solves the problem.

Solution:

Let E₁ =event that A solves the problem,

and E₂ =event that B solves the problem.

Then, P(E₁) = \(\frac{1}{2}\) and P(E₂) = \(\frac{1}{3}\)

⇒ \(P\left(\bar{E}_1\right)=\left(1-\frac{1}{2}\right)=\frac{1}{2} \text { and } P\left(\bar{E}_2\right)=\left(1-\frac{1}{3}\right)=\frac{2}{3} \text {. }\)

Clearly, E₁ and E₂ are independent events.

⇒ \(P\left(E_1 \cap E_2\right)=P\left(E_1\right) \times P\left(E_2\right)=\left(\frac{1}{2} \times \frac{1}{3}\right)=\frac{1}{6}\).

1. P(the problem is solved)

= P(at least one of A and B solves the problem)

= \(P\left(E_1 \text { or } E_2\right)=P\left(E_1 \cup E_2\right)\)

= \(P\left(E_1\right)+P\left(E_2\right)-P\left(E_1 \cap E_2\right)\)

= \(\left(\frac{1}{2}+\frac{1}{3}-\frac{1}{6}\right)=\frac{4}{6}=\frac{2}{3}\).

2. P(exactly one of them solves the problem)

= \(P\left(E_1 \text { or } E_2\right)=P\left(E_1 \cup E_2\right)\)

= \(P\left(E_1\right)+P\left(E_2\right)-P\left(E_1 \cap E_2\right)\)

= \(=\left(\frac{1}{2} \times \frac{2}{3}\right)+\left(\frac{1}{3} \times \frac{1}{2}\right)=\left(\frac{1}{3}+\frac{1}{6}\right)=\frac{3}{6}=\frac{1}{2}\).

Example 13. Amit and Nisha appear for an interview for two vacancies in a company. The probability of Amit’s selection is 1/5 and that of Nisha’s selection is 1/6. What is the probability that

1. Both of them are selected?
2. Only one of them is selected?
3. None of them is selected?

Solution:

Let E₁ = event that Amit is selected,

and E₂ = event that Nisha is selected.

Then, P(E₁) = \(\frac{1}{5}\) and P(E₂) = \(\frac{1}{6}\)

Clearly, E₁ and E₂ are independent events.

1. P(both are selected) =P(E₁∩E₂)

=P(E₁)xP(E₂) [E₁ and E₂ are independent]

= \(\left(\frac{1}{5} \times \frac{1}{6}\right)=\frac{1}{30}\)

2. P(only one of them is selected)

=P[(E₁ and not E₂) or (E₂ and not E₁]

=P(E₁ and not E₂) +P(E₂ and not E₁)

=P(E₁) -P(not E₂) +P(E₂)-P(not E₁)

=P(E₁)•[1 -P(E₂)] +P(E₂)•[1 -P(E₁)]

= \(\frac{1}{5} \cdot\left(1-\frac{1}{6}\right)+\frac{1}{6} \cdot\left(1-\frac{1}{5}\right)=\left(\frac{1}{5} \times \frac{5}{6}\right)+\left(\frac{1}{6} \times \frac{4}{5}\right)\)

= \(\left(\frac{1}{6}+\frac{2}{15}\right)=\frac{9}{30}=\frac{3}{10}\)

3. P (none of them is selected)

=P(not E₁ and not E₂)

=P(not E₁) and P(not E₂)

=[1-P(E₁)] [1-P(E₂)1]

= \(\left(1-\frac{1}{5}\right) \cdot\left(1-\frac{1}{6}\right)=\left(\frac{4}{5} \times \frac{5}{6}\right)=\frac{2}{3}\)

Example 14. Three groups of children contain 3 girls and 1 boy; 2 girls and 2 boys; and 1 girl and 3 boys. One child is selected at random from each group. Find the chance that the three children selected comprise 1 girl and 2 boys.
Solution:

Let G₁, G₂, and G₃ be the events of selecting a girl from the first, second and third groups respectively, and let B₁, B₂, and B₃ be the events of selecting a boy from the first, second and third groups respectively.

Then,

⇒ \(P\left(G_1\right)=\frac{3}{4} ; P\left(G_2\right)=\frac{2}{4}=\frac{1}{2} ; P\left(G_3\right)=\frac{1}{4}\)

⇒ \(P\left(B_1\right)=\frac{1}{4} ; P\left(B_2\right)=\frac{2}{4}=\frac{1}{2} \text { and } P\left(B_3\right)=\frac{3}{4}\)

∴ P(selecting 1 girl and 2 boys)

= \(P\left[\left(G_1 B_2 B_3\right) \text { or }\left(B_1 G_2 B_3\right) \text { or }\left(B_1 B_2 G_3\right)\right]\)

= \(P\left(G_1 B_2 B_3\right)+P\left(B_1 G_2 B_3\right)+P\left(B_1 B_2 G_3\right)\)

= \(\left\{P\left(G_1\right) \times P\left(B_2\right) \times P\left(B_3\right)\right\}+\left\{P\left(B_1\right) \times P\left(G_2\right) \times P\left(B_3\right)\right\}+\left\{P\left(B_1\right) \times P\left(B_2\right) \times P\left(G_3\right)\right\}\)

= \(\left(\frac{3}{4} \times \frac{1}{2} \times \frac{3}{4}\right)+\left(\frac{1}{4} \times \frac{1}{2} \times \frac{3}{4}\right)+\left(\frac{1}{4} \times \frac{1}{2} \times \frac{1}{4}\right)=\left(\frac{9}{32}+\frac{3}{32}+\frac{1}{32}\right)=\frac{13}{32}\).

Hence, the chances of selecting 1 girl and 2 boys are \(\frac{13}{32}\)

Example 15. A problem is given to three students whose chances of solving it are 1/3, 2/7 and 3/8. What is the probability that the problem will be solved?
Solution:

Let the three students be  A, B, and C respectively. Let E₁/E₂/E₃ be the events that the problem is solved by A, B, and C respectively. Then,

⇒ \(P\left(E_1\right)=\frac{1}{3}, P\left(E_2\right)=\frac{2}{7}, P\left(E_3\right)=\frac{3}{8} .\)

∴ \(P\left(\bar{E}_1\right)=\left(1-\frac{1}{3}\right)=\frac{2}{3} ; P\left(\bar{E}_2\right)=\left(1-\frac{2}{7}\right)=\frac{5}{7} \text { and } P\left(\bar{E}_3\right)=\left(1-\frac{3}{8}\right)=\frac{5}{8}\)

∴ P(none solves the problem)

= P[(not E₁) and (not E₂) and (not E₃)]

= \(P\left(\bar{E}_1 \cap \bar{E}_2 \cap \bar{E}_3\right)\)

= \(P\left(\bar{E}_1\right) \times P\left(\bar{E}_2\right) \times P\left(\bar{E}_3\right) \quad\left[because \bar{E}_1, \bar{E}_2, \bar{E}_3 \text { are independent }\right]\)

= \(\left(\frac{2}{3} \times \frac{5}{7} \times \frac{5}{8}\right)=\frac{25}{84}\).

∴ P(that the problem is solved)

=1-P(none solves the problem)

= \(\left(1-\frac{25}{84}\right)=\frac{59}{84}\) .

Hence, the required probability is \(\frac{59}{84}\).

Example 16. A problem in mathematics is given to three students whose chances of solving it correctly are 1/2, 1/3 and 1/4 respectively. What is the probability that only one of them solves it correctly?
Solution:

Let A, B, and C be the given students and let E₁,E₂ and E₃ be the events that the problem is solved by A, B, and C respectively. Then,

⇒ \(\bar{E}_1, \bar{E}_2 \text { and } \bar{E}_3\)are the events that the given problem is not solved by A, B, C respectively. Then,

⇒ \(P\left(E_1\right)=\frac{1}{2} ; P\left(E_2\right)=\frac{1}{3} ; P\left(E_3\right)=\frac{1}{4} ;\)

⇒ \(P\left(\bar{E}_1\right)=\left(1-\frac{1}{2}\right)=\frac{1}{2} ; P\left(\bar{E}_2\right)=\left(1-\frac{1}{3}\right)=\frac{2}{3} \text { and } P\left(\bar{E}_3\right)=\left(1-\frac{1}{4}\right)=\frac{3}{4}\)

P (exactly one of them solves the problem)

= \(P\left[\left(E_1 \cap \bar{E}_2 \cap \bar{E}_3\right) \text { or }\left(\bar{E}_1 \cap E_2 \cap \bar{E}_3\right) \text { or }\left(\bar{E}_1 \cap \bar{E}_2 \cap E_3\right)\right]\)

= \(P\left(E_1 \cap \bar{E}_2 \cap \bar{E}_3\right)+P\left(\bar{E}_1 \cap E_2 \cap \bar{E}_3\right)+P\left(\bar{E}_1 \cap \bar{E}_2 \cap E_3\right)\)

= \(\left\{P\left(E_1\right) \times P\left(\bar{E}_2\right) \times P\left(\bar{E}_3\right)\right\}+\left\{P\left(\bar{E}_1\right) \times P\left(E_2\right) \times P\left(\bar{E}_3\right)\right\}\quad\)

+ \(\left\{P\left(\bar{E}_1\right) \times P\left(\bar{E}_2\right) \times P\left(E_3\right)\right\}\)

= \(\left(\frac{1}{2} \times \frac{2}{3} \times \frac{3}{4}\right)+\left(\frac{1}{2} \times \frac{1}{3} \times \frac{3}{4}\right)+\left(\frac{1}{2} \times \frac{2}{3} \times \frac{1}{4}\right)\)

= \(\left(\frac{1}{4}+\frac{1}{8}+\frac{1}{12}\right)=\frac{11}{24}\)

Hence, the required probability is \(\frac{11}{24}\)

Example 17. Three critics review a book. For the three critics, the odds in favour of the book are (5:2), (4:3) and (3:4) respectively. Find the probability that the majority is in favour of the book.
Solution:

Let A, B, and C denote the events that the book is favoured by the first, second and third critic respectively. Then,

P(A)= \(\frac{5}{7}\);P(B)= \(\frac{4}{7}\);P(C) = \(\frac{3}{7}\);

⇒ \(P(\bar{A})=\left(1-\frac{5}{7}\right)=\frac{2}{7} ; P(\bar{B})=\left(1-\frac{4}{7}\right)=\frac{3}{7} \text { and } P(\bar{C})=\left(1-\frac{3}{7}\right)=\frac{4}{7}\)

Required probability

= P(2 critics favour the book or 3 critics favour the book)

= P(2 critics favour the book) + P(3 critics favour the book)

= P[{A and B and not C} or A and C and not B or {B and C and not A}] + P(A and B and C)

= \(P(A \cap B \cap \bar{C})+P(A \cap \bar{B} \cap C)+P(\bar{A} \cap B \cap C)+P(A \cap B \cap C)\)

= \(\{P(A) \times P(B) \times P(\bar{C})\}+\{P(A) \times P(\bar{B}) \times P(C)\}+\{P(\bar{A}) \times P(B) \times P(C)\}\)

+ \(\{P(A) \times P(B) \times P(C)\}\)

= \(\left(\frac{5}{7} \times \frac{4}{7} \times \frac{4}{7}\right)\)+\(\left(\frac{5}{7} \times \frac{3}{7} \times \frac{3}{7}\right)\)+\(\left(\frac{2}{7} \times \frac{4}{7} \times \frac{3}{7}\right)\)+\(\left(\frac{5}{7} \times \frac{4}{7} \times \frac{3}{7}\right)\)

= \(\left(\frac{80}{343}+\frac{45}{343}+\frac{24}{343}+\frac{60}{343}\right)=\frac{209}{343}\)

Hence, the required probability is \(\frac{209}{343}\)

Example 18. The odds against a man who is 45 years old, living till he is 70 are 7: 5, and the odds against his wife who is now 36, living till she is 61 are 5 : 3. Find the probability that

1. The couple will be alive 25 years hence
2. At least one of them will be alive 25 years hence.

Solution:

Let E₁ = event that the husband will be alive 25 years hence, and E₂ = event that the wife will be alive 25 years hence.

Then, \(P\left(E_1\right)=\frac{5}{12} and P\left(E_2\right)=\frac{3}{8}\).

∴ \(P\left(\bar{E}_1\right)=\left(1-\frac{5}{12}\right)=\frac{7}{12} \text { and } P\left(\bar{E}_2\right)=\left(1-\frac{3}{8}\right)=\frac{5}{8} \text {. }\)

Clearly, E₁ and E₂ are independent events.

1. P(the couple will be alive 25 years hence) =

= \(P\left(E_1 \text { and } E_2\right)=P\left(E_1 \cap E_2\right)\)

= \(P\left(E_1\right) \cdot P\left(E_2\right)=\left(\frac{5}{12} \times \frac{3}{8}\right)=\frac{5}{32}\).

2. P(at least one of them will be alive 25 years hence)

= 1-P(none will be alive 25 years hence)

= 1-P[(not E₁) and (not E₂)]

= \(1-P\left(\bar{E}_1 \cap \bar{E}_2\right)\)

= \(1-\left[P\left(\bar{E}_1\right) \cdot P\left(\bar{E}_2\right)\right] \quad\left[because \bar{E}_1 \text { and } \bar{E}_2 \text { are independent }\right]\)

= \(1-\left(\frac{7}{12} \times \frac{5}{8}\right)=\left(1-\frac{35}{96}\right)=\frac{61}{96}\)

Example 19. A, B and C shoot to hit a target. If A hits the target 4 times in 5 trials; B hits it 3 times in 4 trials and C hits it 2 times in 3 trials, what is the probability that the target is hit by at least 2 persons?
Solution:

Let E₁, E₂ and E₃ be the events that A hits the target, B hits the target and C hits the target respectively. Then,

⇒ \(P\left(E_1\right)=\frac{4}{5}, P\left(E_2\right)=\frac{3}{4}, P\left(E_3\right)=\frac{2}{3} \)

⇒  \(P\left(\bar{E}_1\right)=\left(1-\frac{4}{5}\right)=\frac{1}{5}, P\left(\bar{E}_2\right)=\left(1-\frac{3}{4}\right)=\frac{1}{4} \text { and } P\left(\bar{E}_3\right)=\left(1-\frac{2}{3}\right)=\frac{1}{3}\)

Case 1 A, B, and C all hit the target

In this case, P(A, B and C) all hit the target)

= \(P\left(E_1\right. and E_2 and \left.E_3\right)\)

= \(P\left(E_1\right) \cdot P\left(E_2\right) \cdot P\left(E_3\right)\) [because \(E_1, E_2, E_3\) are independent]

= \(\left(\frac{4}{5} \times \frac{3}{4} \times \frac{2}{3}\right)=\frac{2}{5}\)

Case 2 A and B hit but not C

In this case, P(A and B hit but not C)

= \(P\left(E_1 \text { and } E_2 \text { and not } E_3\right)\)

= \(P\left(E_1 \cap E_2 \cap \bar{E}_3\right)\)

= \(P\left(E_1\right) \cdot P\left(E_2\right) \cdot P\left(\bar{E}_3\right)\left[because E_1, E_2, \bar{E}_3 \text { are independent }\right]\)

= \(\left(\frac{4}{5} \times \frac{3}{4} \times \frac{1}{3}\right)=\frac{1}{5}\).

Case 3 A and C both hit but not B

In this case, P(A and C hit but not B)

= \(P\left(E_1 \text { and } E_3 \text { and } \bar{E}_2\right)\)

= \(P\left(E_1\right) \cdot P\left(E_3\right) \cdot P\left(\bar{E}_2\right)\left[because E_1, E_3, \bar{E}_2\right. \text { are independent}\)

= \(\left(\frac{4}{5} \times \frac{2}{3} \times \frac{1}{4}\right)=\frac{2}{15}\)

Case 4 B and C both hit but not A

In this case, P(B and C hit but not A)

= \(P\left(E_2 \text { and } E_3 \text { and } E_1\right)\)

= \(P\left(E_2\right) \cdot P\left(E_3\right) \cdot P\left(\bar{E}_1\right)\left[because E_2, E_3, \bar{E}_1 \text { are independent }\right]\)

= \(\left(\frac{3}{4} \times \frac{2}{3} \times \frac{1}{5}\right)=\frac{1}{10}\).

Clearly, all these are mutually exclusive.

Hence, required probability = \(\left(\frac{2}{5}+\frac{1}{5}+\frac{2}{15}+\frac{1}{10}\right)=\frac{5}{6} \text {. }\)

Multiplication Theorem On Probability Excercise 1B

Question 1. A bag contains 17 tickets, numbered from 1 to 17. A ticket is drawn and then another ticket is drawn without replacing the first one. Find the probability that both the tickets may show even numbers.
Solution: 7/34

Question 2. Two marbles are drawn successively from a box containing 3 black and 4 white marbles. Find the probability that both the marbles are black, if the first marble is not replaced before the second draw.
Solution: 1/7

Question 3. A card is drawn from a well-shuffled deck of 52 cards and without replacing this card, a second card is drawn. Find the probability that the first card is a club and the second card is a spade.
Solution: 13/204

Question 4. There is a box containing 30 bulbs of which 5 are defective. If two bulbs are chosen at random from the box in succession without replacing the first, what is the probability that both the bulbs chosen are defective?
Solution: 2/87

Question 5. A bag contains 10 white and 15 black balls. Two balls are drawn in succession without replacement. What is the probability that the first ball is white and the second is black?
Solution:  1/4

Question 6. An urn contains 5 white and 8 black balls. Two successive drawings of 3 balls at a time are made such that the balls drawn in the first draw are not replaced before the second draw. Find the probability that the first draw gives 3 white balls and the second draw gives 3 black balls.
Solution: 7/429

Explanation:

Required Probability = \(\left\{\frac{{ }^5 C_3}{{ }^{13} C_3} \times \frac{{ }^8 C_3}{{ }^{10} C_3}\right\}=\left(\frac{5 \times 4 \times 3}{13 \times 12 \times 11} \times \frac{8 \times 7 \times 6}{10 \times 9 \times 8}\right)\)

Question 7. Let E₁ and E₂ be the events such that P(E₁) = 1/3 and P(E₂) = 3/5 Find:

1. P(E₁∪E₂), when E₁ and E₂ are mutually exclusive,
2. P(E₁∩E₂), when E₁ and E₂ are independent.

Solution:

1. 14/15
2. 1/5

Question 8. If E₁ and E₂ are two events such that P(E₁) =1/4, P(E₂) = 1/3, and P(E₁∪E₂) = 1/2, show that E₁ and E₂ are independent events.
Solution: 4/5

Question 9. If E₁ and E₂ are independent events such that P(E₁) =0.3 and P(E₂) =0.4, find

1. \(P\left(E_1 \cap E_2\right)\)
2. \(P\left(E_1 \cap E_2\right)\)
3. \(P\left(\bar{E}_1 \cap \bar{E}_2\right)\)
4. \(P\left(\bar{E}_1 \cap E_2\right)\).

Solution:

1. 0.12
2. 0.58
3. 0.42
4. 0.28

Question 10. Let A and B be events such that P(A) = 1/2, P(B) = 7/12 and P(not A or not B) = 1/4 State whether A and B are

1. Mutually exclusive,
2. Independent.⇒

Solution:

1. No
2. No

Hint: P({not} A or {not} B) = \(P(\bar{A} \text { or } \bar{B})=P(\bar{A} \cup \bar{B})\)

= \(P(\overline{A \cap B})=1-P(A \cap B)\)

⇒ \(1-P(A \cap B)=\frac{1}{4}\)

⇒ \(P(A \cap B)=\frac{3}{4}\)

1. Since \(P(A \cap B) \neq 0\), so A and B are not mutually exclusive.

2. \(P(A) \times P(B)=\left(\frac{1}{2} \times \frac{7}{12}\right)=\frac{7}{24} \neq P(A \cap B)\)

⇒ A and B are not independent.

Question 11. Kamal and Vimal appeared for an interview for two vacancies. The probability of Kamal’s selection is 1/3 and that of Vimal’s selection is 1/5 Find the probability that only one of them will be selected.
Solution: 2/5

Hint:

Let E₁ = event that kamal is selected,

and E₂ = event that Vimal is selected. Then,

⇒ \(P\left(E_1\right)=\frac{1}{3}, P\left(E_2\right)=\frac{1}{5}, P\left(\bar{E}_1\right)=\left(1-\frac{1}{3}\right)=\frac{2}{3} \text { and } P\left(\bar{E}_2\right)=\left(1-\frac{1}{5}\right)=\frac{4}{5}\)

∴ required probability

= \(P\left[\left(E_1 \text { and } \operatorname{not} E_2\right) \text { or }\left(E_2 \text { and } \operatorname{not} E_1\right)\right]\)

= \(P\left[\left(E_1\right.\right. and \left.\bar{E}_2\right) or \left(E_2\right. and \left.\left.\bar{E}_1\right)\right]\)

= \(P\left(E_1 \cap \bar{E}_2\right)+P\left(E_2 \cap \bar{E}_1\right)\)

= \(\left\{P\left(E_1\right) \times P\left(\bar{E}_2\right)\right\}+\left\{P\left(E_2\right) \times P\left(\bar{E}_1\right)\right\}\).

Question 12. Arun and Ved appeared for an interview for two vacancies. The probability of Aran’s selection is 1/4 and that of Ved’s rejection is 2/3. Find the probability that at least one of them will be selected.
Solution: 1/2

Hint:

Let E₁ =event that Arun is selected,

and E₂ =event that Ved is selected.

Then, \(P\left(E_1\right)=\frac{1}{4} \text { and } P\left(E_2\right)=\left(1-\frac{2}{3}\right)=\frac{1}{3} \text {. }\)

Clearly, E₁ and E₂ are independent events.

∴ \(P\left(E_1 \cap E_2\right)=P\left(E_1\right) \times P\left(E_2\right)=\left(\frac{1}{4} \times \frac{1}{3}\right)=\frac{1}{12}\)

Required probability = \(P\left(E_1 \text { or } E_2\right)=P\left(E_1 \cup E_2\right)\)

= \(P\left(E_1\right)+P\left(E_2\right)-P\left(E_1 \cap E_2\right)=\left(\frac{1}{4}+\frac{1}{3}-\frac{1}{12}\right)=\frac{6}{12}=\frac{1}{2}\)

Question 13. A and B appear for an interview for two vacancies in the same post. The probability of A’s selection is 1/6 and that of B’s selection is 1/4. Find the probability that

1. Both of them are selected
2. Only one of them is selected
3. None is selected
4. At least one of them is selected.

Solution:

1. 1/24
2. 1/3
3. 5/8
4. 3/8

Hint:

We have P(A)= \(\frac{1}{6}, P(B)=\frac{1}{4}\).

∴ \(P(\bar{A})=1-P(A)=\left(1-\frac{1}{6}\right)=\frac{5}{6}\) and \(P(\bar{B})=1-P(B)=\left(1-\frac{1}{4}\right)=\frac{3}{4}\).

1. P (both are selected)

= \(P(A \cap B)=P(A) \times P(B) \text {. }\)

2. P(only one of them is selected)

= \(P[(A \text { and not } B) \text { or }(B \text { and not } A)]\)

= \(P(A \cap \bar{B})+P(B \cap \bar{A})\)

= \(\{P(A) \times P(\bar{B})\}+\{P(B) \times P(\bar{A})\}\)

3. P( none is selected )=P(not A and not B)

= \(P(\bar{A} \cap \bar{B})=P(\bar{A}) \times P(\bar{B})\)

4. P (at least one is selected )= 1-P (none is selected).

Question 14. Given the probability that A can solve a problem is 2/3, and the probability that B can solve the same problem is 3/5, find the probability that

1. At least one of a and b will solve the problem
2. None of the two will solve the problem.

Solution:

1. 13/15
2. 2/15

Hint:

Let E₁ =event that A can solve the problem

and E₂ =event that B can solve the problem.

Then, E₁ and E₂ are clearly independent events.

∴ \(P\left(E_1 \cap E_2\right)=P\left(E_1\right) \times P\left(E_2\right)=\left(\frac{2}{3} \times \frac{3}{5}\right)=\frac{2}{5} \text {. }\)

1. P(at least one of A and B can solve the problem)

= \(P\left(E_1 \cup E_2\right)=P\left(E_1\right)+P\left(E_2\right)-P\left(E_1 \cap E_2\right)=\left(\frac{2}{3}+\frac{3}{5}-\frac{2}{5}\right)=\frac{13}{15}\)

2. P(none can solve the problem)

=1-P(at least one can solve the problem)

= \(\left(1-\frac{13}{15}\right)=\frac{2}{15}\)

Question 15. A problem is given to three students whose chances of solving it are 1/4, 1/5, and 1/6 respectively. Find the probability that the problem is solved.
Solution: 1/2

Hint: Let A, B, and C be the events of solving the problem by the 1st, 2nd, and 3rd student respectively. Then,

P(A)= \(\frac{1}{4}\), P(B)= \(\frac{1}{5}\) and P(C)= \(\frac{1}{6}\)

⇒ \(P(\bar{A})=\left(1-\frac{1}{4}\right)=\frac{3}{4}  P(\bar{B})=\left(1-\frac{1}{5}\right)=\frac{4}{5} \text { and } P(\bar{C})=\left(1-\frac{1}{6}\right)=\frac{5}{6}\)

∴ P(none solves the problem)= P({not} A)and{not} B) and }({not} C))

= \(P(\bar{A} \text { and } \bar{B} \text { and } \bar{C})=P(\bar{A}) \times P(\bar{B}) \times P(\bar{C})\)

Question 16. The probabilities of A, B, and C solving a problem are 1/3, 1/4, and 1/6 respectively. If all three try to solve the problem simultaneously, find the probability that exactly one of them will solve it.
Solution: 31/72

Hint:

Given P(A)= \(\frac{1}{3}\), P(B)=\(\frac{1}{4}\) and P(C)= \(\frac{1}{6}\)

⇒ \(P(\bar{A})=\left(1-\frac{1}{3}\right)=\frac{2}{3}, P(\bar{B})=\left(1-\frac{1}{4}\right)=\frac{3}{4} \text { and } P(\bar{C})=\left(1-\frac{1}{6}\right)=\frac{5}{6}\)

∴ required probability

= P(exactly one of them solves the problem)

= \(P[(A \cap \bar{B} \cap \bar{C}) \text { or }(\bar{A} \cap B \cap \bar{C}) \text { or }(\bar{A} \cap \bar{B} \cap C)]\)

= \(P(A \cap \bar{B} \cap \bar{C})+P(\bar{A} \cap B \cap \bar{C})+P(\bar{A} \cap \bar{B} \cap C)\)

= \(\{P(A) \times P(\bar{B}) \times P(\bar{C})\}+\{P(\bar{A}) \times P(B) \times P(\bar{C})\}+\{P(\bar{A}) \times P(\bar{B}) \times P(C)\}\)

Question 17. A can hit a target 4 times in 5 shots, B can hit 3 times in 4 shots, and C can hit 2 times in 3 shots. Calculate the probability that

1. A, B, and C all hit the target
2. B and C hit and A does not hit the target.

Solution:

1. 2/5
2. 1/10

Hint: P(A hits)= 4/5, P(B hits)= 3/4 and P(C hits)= 2/3

1. P(A hits and B hits and C hits) = P(A) x P(B) x P(C).
2. P(B hits C hits and A does not hit) = P(B)xP(C)xP\((\overline{\mathrm{A}})\)

Question 18. Neelam has offered physics, chemistry, and mathematics in Class 12. She estimates that her probabilities of receiving a grade A in these courses are 0.2, 0.3, and 0.9 respectively. Find the probabilities that Neelam receives

1. All a grades
2. No a grade
3. Exactly 2 grades.

Solution:

1. 0.054
2. 0.056
3. 0.348

Hint:

1. P(getting all A grades) = \(P\left(A_1 A_2 A_3\right)=P\left(A_1\right) \times P\left(A_2\right) \times P\left(A_3\right)\)

2. P(getting no A grade) = \(P\left(\bar{A}_1 \bar{A}_2 \bar{A}_3\right)=P\left(\bar{A}_1\right) \times P\left(\bar{A}_2\right) \times P\left(\bar{A}_3\right)\) .

3. P(getting exactly two A grades)

= \(P\left[\left(\bar{A}_1 A_2 A_3\right) \text { or }\left(A_1 \bar{A}_2 A_3\right) {or}\left(A_1 A_2 \bar{A}_3\right)\right]\)

= \(P\left(\bar{A}_1 A_2 A_3\right)+P\left(A_1 \bar{A}_2 A_3\right)+P\left(A_1 A_2 \bar{A}_3\right)\)

= \(\left\{P\left(\bar{A}_1\right) \times P\left(A_2\right) \times P\left(A_3\right)\right\}+\left\{P\left(A_1\right) \times P\left(\bar{A}_2\right) \times P\left(A_3\right)\right\}+\left\{P\left(A_1\right) \times P\left(A_2\right) \times P\left(\bar{A}_3\right)\right\}\)

Question 19. An article manufactured by a company consists of two parts X and Y. In the process of manufacture of part X, 8 out of 100 parts may be defective. Similarly, 5 out of 100 parts of Y may be defective. Calculate the probability that the assembled product will not be defective.
Solution: 437/500

Hint: P(X is defective) = \(\frac{8}{100}\) = \(\frac{2}{25}\)

P(Y is defective) = \(\frac{5}{100}\) = \(\frac{1}{20}\)

P(X is not defective) = (1-\(\frac{2}{25}\)) = \(\frac{23}{25}\)

P(Y is not defective = (1-\(\frac{2}{20}\)) = \(\frac{19}{20}\)

Required probability =P(assembled part is not defective)

= P(X is not defective and Y is not defective)

= \(\left(\frac{23}{25} \times \frac{19}{20}\right)=\frac{437}{500}\).

Question 20. A town has two fire-extinguishing engines, functioning independently. The probability of availability of each engine when needed is 0.95. What is the probability that

1. Neither of them is available when needed.
2. An engine is available when needed?

Solution:

1. 1/400
2. 19/200

Hint: Let E₁ =event of availability of the first engine.

And, E₂ =event of availability of the second engine.

Then, \(P\left(E_1\right)=P\left(E_2\right)=0.95 and P\left(\bar{E}_1\right)=P\left(\bar{E}_2\right)=(1-0.95)=0.05\).

1. P(neither of them is available when needed)

= \(P\left(\bar{E}_1 \text { and } \bar{E}_2\right)=P\left(\bar{E}_1\right) \times P\left(\bar{E}_2\right) \text {. }\)

2. P(an engine is available when needed)

= \(P\left[\left(E_1 \text { and not } E_2\right) \text { or }\left(E_2 \text { and not } E_1\right)\right]\)

= \(P\left[\left(E_1 \text { and } \bar{E}_2\right) \text { or }\left(E_2 \text { and } \bar{E}_1\right)\right] \)

= \(P\left(E_1 \cap \bar{E}_2\right)+P\left(E_2 \cap \bar{E}_1\right)\)

= \(\left\{P\left(E_1\right) \times P\left(\bar{E}_2\right)\right\}+\left\{P\left(E_2\right) \times P\left(\bar{E}_1\right)\right\}\)

Question 21. A machine operates only when all of its three components function. The probabilities of the failures of the first, second, and third components are 0.14, 0.10, and 0.05 respectively. What is the probability that the machine will fail?
Solution: 0.02647

Hint: Let E₁, E₂, and E₃ be the respective events that the 1st, 2nd, and 3rd components function. Then,

⇒ \(P\left(\bar{E}_1\right)=0.14, P\left(\bar{E}_2\right)=0.10 \text { and } P\left(\bar{E}_3\right)=0.05\)

P(E₁) =(1- 0.14) = 0.86, P(E₂) =(1- 0.10) = 0.90 and P(E₃) =(1- 0.05) = 0.95

P(machine fails) =1 -P(machine functions)

=1-P[(E₁ and E₂ and E₃)]

=1-P(E₁)X P(E₂)X P(E₃)].

Question 22. An anti-aircraft gun can take a maximum of 4 shots at an enemy plane moving away from it. The probabilities of hitting the plane at the first, second, third, and fourth shots are 0.4,0.3,0.2 and 0.1 respectively. What is the probability that at least one shot hits the plane?
Solution: 0.6976

Hint: Let E₁, E₂, E₃, and E₄ be the respective events that the plane is hit in the 1st, 2nd, 3rd, and 4th shots. Then,

P(E₁) = 0.4, P(E₂) = 0.3, P(E₃) = 0.2 and P(E₄) = 0.1

∴ \(P\left(\bar{E}_1\right)=(1-0.4)=0.6, P\left(\bar{E}_2\right)=(1-0.3)=0.7,\)

∴ \(P\left(\bar{E}_3\right)=(1-0.2)=0.8, P\left(\bar{E}_4\right)=(1-0.1)=0.9\)

∴ P(at least one shot hits the plane) = \(1-P\left(\bar{E}_1 \text { and } \bar{E}_2 \text { and } \bar{E}_3 \text { and } \bar{E}_4\right)\)

= \(1-\left\{P\left(\bar{E}_1\right) \times P\left(\bar{E}_2\right) \times P\left(\bar{E}_3\right) \times P\left(\bar{E}_4\right)\right\}\)

Question 23. Let S₁ and S₂ be two switches and let their probabilities of working be given by P(S₁) = 4/5 and P(S₂) = 9/10. Find the probability that the current flows from terminal A to terminal B when S₁ and S₂ are installed in series, shown as follows:
Solution: 18/25

Hint: P(current flows from A to B)

=P(S₁ is closed and S₂ is closed)

=P(S₁ and S₂)=P(S₁) x P(S₂).

Question 24. Let S₁ and S₂ be two switches and let their probabilities of working be given by P(S₁) = 4/5 and P(S₂) = 3/4. Find the probability that the current flows from terminal A to terminal B, when S₁ and S₂ are installed in parallel, as shown below:
Solution: 11/12

Hint: P(the current flows) =P(S₁ or S₂ )

=P(S₁∪S₂)=P(S₁)+P(S₂)-P(S₁∩S₂)

=P(S₁)+P(S₂)-P(S₁)xP(S₂).

Question 25. A coin is tossed. If a head comes up, a die is thrown but if a tail comes up, the coin is tossed again. Find the probability of obtaining

1. Two tails
2. A head and the number 6
3. A head and an even number.

Solution:

1. 1/8
2. 1/8
3. 1/3

Hint:

S =[H1, HI, H3, HA, H5, H6, TT, TH) → n(S) = 8.

1. P(two tails) = 1/8
2. P(head and the number 6) = 1/8
3. P(head and an even number) = 3/8