NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Introduction

In daily life, there are so many examples which follow a certain pattern.

1. In a fixed deposit scheme, the amount becomes. 1 item of itself after every year. The maturity amount of ₹10,000 after 1, 2, 3 and 4 years will be ₹11,000, ₹12,100, ₹13,310,14.641
2. When a person is offered a job with a monthly salary of ₹18,000 and with an annual increment of ₹400, His salary for the succeeding years will be ₹18,000, ₹18,400, ₹ 18,800 per mon.
   • In the first example, the succeeding terms are obtained by multiplying with 1.1. In the second example, the succeeding terms are obtained by adding 400.
   • Now, we will discuss all those patterns in which the succeeding terms are obtained by adding a fixed number to the preceding terms.

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Sequence

A number of things that come one after another form a sequence. It may be possible that we do not have a formula to find the nth term of the sequence, but still, we know about the next term.

Read and Learn More Class 10 Maths Solutions Exemplar

Sequence For example: 2, 3, 5, 7, 11,13, 17, 19, …is a sequence of prime numbers.

We all know about the next number of the sequence but we do not have any formula to calculate the next number.

• The numbers present in the sequence are called the terms of the sequence.
• The nth term of the sequence can be represented by T_n or a_n. It is called the general term.
• A sequence which has finite terms is called a finite sequence.

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Progression

Those sequences whose terms follow certain patterns are called progressions. In progression, each term (except the first) is obtained from the previous one by some rule.

The difference between a progression and a sequence is that a progression has a specific formula to calculate its nth term, whereas a sequence can be based on a logical rule like a group of prime numbers, which does not have any formula associated with it.

The numbers 2, 4, 6, 8, 10, 12 … form a progression as its nth term T_n =2n while 2,3,5,7,11,13,17,19,23,29,31,… is a sequence of numbers, as there is no formula to find the next number. It is a group of prime numbers.

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Series

If T₁, T₂, T₃,…, T_n form a progression, then T₁, T₂, T₃,…, T_n is called its corresponding series. In other words, if all the terms of a progression are connected by ‘+’ started

Series For example:1-2 + 3- 4+5-6 ……… is a series. The first term is 1, the second term is -2, the third term is 3, the fourth is -4 and so on. Its nth is n(-1) n+1

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Series Solved Examples

Question 1. The nth of a sequence is an = 2n+1. Find its first four terms.
Solution:

Given

The nth of a sequence is an = 2n+1.

a_n=2n+1

put n= 1,2,3,4, we get

a₁ = 2×1+3 = 5

a₂ = 2×2+3 = 7

a₃ = 2×3+3 = 9

a₄ = 2×4+3 = 11

∴ The first four terms of the sequence are 5,7,9,11.

Question 2. The nth term of a sequence is an=n2+5. Find its first terms.
Solution:

Given

The nth term of a sequence is an=n²+5

a_n=n²+5

put n=1,2,3, we get

a₁ = 1² + 5 = 6

a₂ = 2² + 5 = 9

a₃ = 3² + 5 = 14

∴ The first four terms of the sequence are 6,9,14.

Question 3. Fibonacci sequence is defined as follows: a₁ = a₂ = 1 and a_n = a_n-2 + a_n-1 , where n > 2. Find third, fourth and fifth terms
Solution:

Given

Fibonacci sequence is defined as follows: a₁ = a₂ = 1 and a_n = a_n-2 + a_n-1 , where n > 2.

a₁ = a₁ = 1

⇒ \(a_n=a_{n-2}+a_{n-1}, n>2\)

put n = 3, we get

⇒ \(a_3=a_1+a_2=1+1=2\)

put n = 4, we get

⇒ \(a_4=a_2+a_3=1+2=3\)

put it = 5, we get

⇒ \(a_5=a_3+a_4=2+3=5\)

Question 4. A sequence is defined as follows: a₁ = 3, a_n = 2a_n-1 + 1, where n > 1. Find \(\frac{a_{n+1}}{a_n}\) for n=1,2,3.
Solution:

Given

A sequence is defined as follows: a₁ = 3, a_n = 2a_n-1 + 1, where n > 1.

a₁=3

⇒ \(a_n=2 a_{n-1}+1 \quad \text { where } n>1\)

put n= 2, we get

⇒ \(a_2=2 a_1+1=2 \times 3+1=7\)

put n = 3, we get

⇒ \(a_3=2 a_2+1=2 \times 7+1=15\)

put n = 4, we get

\(a_4=2 a_3+1=2 \times 15+1=31\)

Now, for n = 1

⇒ \(\frac{a_{n+1}}{a_n}=\frac{a_2}{a_1}=\frac{7}{3}\)

For n = 2,

⇒ \(\frac{a_{n+1}}{a_n}=\frac{a_3}{a_2}=\frac{15}{7}\)

For n = 3,

⇒ \(\frac{a_{n+1}}{a_n}=\frac{a_4}{a_3}=\frac{31}{15}\)

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression

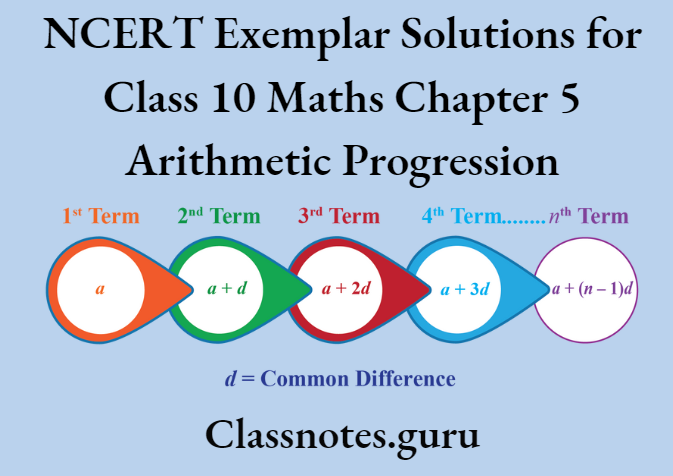
An arithmetic progression (A.P.) is a list of numbers in which each term is obtained by adding a fixed number to the preceding term, except the first term.

or

If the difference of any two consecutive terms of a progression is the same (constant), it is called arithmetic progression.

For example: a, a + d, a + 2d, a + 3d, ….

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression – Common Difference

The difference between two consecutive terms (i.c., any term — preceding term) of an arithmetic progression is called a common difference.

It is denoted by ‘d’

∴ d = a₂-a₁ = a₃-a₂= … = a_n-a_n-1.

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 General Term Of Arithmetic Progression

Let the first term and common difference of an arithmetic progression be ‘a’ and ‘b’ respectively.

∴ Arithmetic progression is a, a +d, a + 2d, a + 3d,…..

Here, first term = a = a+(1-1)d

Second term = a+d = a+(2-1)d

Third term = a+2d = a+(3-1)d
.
.
.
.

nth term = a+(n-1)d

∴ a_n=a+(n-1)d

nth term of a progression is called its general term.

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression nth Term From The End Of An Arithmetic Progression

Let the first term, common difference and last term of an arithmetic progression be a, d and / respectively.

Arithmetic progression is a, a + d, a + 2d,…….l – 2d, l-d,l

Here, the First term from the end -l = l – (1 -1)d

Second term from the end = l – d =l-(2-l)d

Third term from the end = l – 2d = l – (3 – 1 )d

.

.

.

nth term from the end =l-(n- 1 )d.

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Solved Examples

Question 1. For the following A.P., write the first term and common difference: 3, 1,-1,-3, …
Solution:

3, 1,-1,-3,…

Here, first term a = 3

Common difference = 1 – 3 = -2

Question 2. Write the first four terms of the A.P., when the first term V and the common difference ‘d’ are given as follows:

a = 10, d = 5

Solution:

a = 10, d = 5

a₁ = 10

a₂ =10 + 5=15

a₃ =15 + 5 = 20

a₄ = 20 + 5 = 25.

∴ First 4 terms are 10, 15, 20, 25.

Question 3. Find the 18th term of the A.P. 4, 7, 10, …
Solution:

Given

A.P. 4, 7, 10

Here, a = 4,

d = 7 – 4 = 10-7 = 3,

n = 18

∴ a_n = a + (n – 1 )d

a₁₈ = 4 + (18 – 1) × 3 =4 + 51=55

∴ 18th term of the given A.P. = 55.

Question 4. What is the common difference of an A.P. in which a₂₁-a₇ = 84?
Solution:

Given

a₂₁-a₇ = 84

Let the first term of A.P. be ‘a’ and common difference be ‘d’.

Since, a₂₁-a₇ =84

∴ (a+20d) -(a-6d) = 84

⇒ 14d = 84

⇒ d= 6

Hence, the common difference is 6.

Question 5. Which term of the A.P. 3, 8, 13, 18,….is 88?
Solution:

Given

A.P. 3, 8, 13, 18,….is 88

Here, a = 3,

A = 8-3 = 13 -8 = 5

Let a_n = 88

⇒ 3 + (n – 1)5 = 88

⇒ 3 + 5K – 5 = 88

⇒ 5n – 2 = 88

⇒ 5n = 90

⇒ n= 18

The 18th term of the given A.P. is 88.

Question 6. Which term of the A.P. 90, 87, 84,…is zero?
Solution:

Given

90, 87, 84,…

Here, a = 90,

d = 87 – 90 = -3

Let a_n = 0

⇒ 90 + (n – 1) (-3) = 0

⇒ 90 – 3n + 3 = 0

⇒ -3n = -93

⇒ n= 31

∴ 31st term of the given A.P. is zero.

Question 7. If 2, a, b, c, d, e,f and 65 form an A.P., find the value of e.
Solution:

Given

2, a, b, c, d, e,f and 65 form an A.P.

Here, T₁ = 2, T₈ = 65, T₆ = e

Let the common difference be D

T₈ = 65 ⇒  T₁ + (8 – 1)D = 65

⇒ 2+7D = 65  ⇒ D = 9

∴ e = T₆ = T₁ + (6 – 1 )D = 2 + 5(9) = 47

Hence, the value of e is 47.

Question 8. Which term of the A.P. \(10,9 \frac{1}{3}, 8 \frac{2}{3}\) ….. is the first negative term?
Solution:

Here, a = 10,

⇒ \(d=9 \frac{1}{3}-10=8 \frac{2}{3}-9 \frac{1}{3}=-\frac{2}{3}\)

Let a_n<0

⇒ \(10+(n-1)\left(-\frac{2}{3}\right)<0\)

⇒ \(\frac{30-2 n+2}{3}<0\)

⇒ 32-2n<0

⇒  32 < 2n

⇒ 2n>32

⇒ n>16

⇒ n = 17, 18, 19, (all are negative terms)

∴ First negative term = 17th term

Now, a₁₇=a+(17-1) d

⇒ \(10+16\left(-\frac{2}{3}\right)=10-\frac{32}{3}=\frac{-2}{3}\)

Thus, 17th term which is \(-\frac{2}{3}\) is the first negative term.

Question 9. The 18th term of an A.P. exceeds its 10th term by 8. Find the common difference.
Solution:

The 18th term of an A.P. exceeds its 10th term by 8.

Let the first term and the common difference of the A.P. be ‘a’ and ‘d’ respectively.

Given that,

⇒ a₁₈ = a₁₀ + 8

⇒ a₁₈-a₁₀= 8

⇒ {a +(18-1)d}-{a + (10- 1)d} = 8

⇒ [a + 17d)-{a + 9d) = 8

⇒ a + 17d-a-9d = 8

⇒ 8d = 8

⇒ d = 1

∴ Common difference = 1.

Example 10. The 26th, 11th and last term of an A.P. are 0, 3 and \(-\frac{1}{5}\) respectively. Find the common difference and the number of terms.
Solution:

The 26th, 11th and last term of an A.P. are 0, 3 and \(-\frac{1}{5}\) respectively.

Let the first term, common difference and number of terms in the A.P. be a, d and n respectively.

Given that

⇒ a₂₆ = 0

⇒ a+25d = 0 ……(1)

⇒ a = -25d

⇒ a₁₁ = 3 a+10d = 3

⇒ -25d+10d = 3 [from (1)]

⇒ -15d = 3

⇒ d = \(-\frac{1}{5}\)

put d = \(-\frac{1}{5}\) in equation (1) we get

⇒ \(a=-25\left(-\frac{1}{5}\right)=5\) and \(a_n=-\frac{1}{5}\)

⇒ \(5+(n-1)\left(-\frac{1}{5}\right)=-\frac{1}{5}\)

⇒ \((n-1)\left(-\frac{1}{5}\right)=-\frac{1}{5}-5=-\frac{26}{5}\)

⇒ n- 1=26

⇒ n= 27

No. of terms in the A.P. = 27.

Question 11. If the 9th term of an A.P. is zero, prove that its 29th term is twice its 19th term.
Solution:

The 9th term of an A.P. is zero

Let the first term and the common difference of the A.P. be ‘a’ and ‘d’ respectively.

Given that,

a₉ = 0

a + 8d = 0

a = -8d

Now, a₂₉ = a+28d = -8d+28d

= 20d

T₁₉ = a+18d = -8d + 18d

= 10d

⇒ 2T₁₉ = 20d

∴ T₂₉ = 2T₁₉

Question 12. The nth term of a sequence is 3n + 5. Show that it is an A.P.
Solution:

Given

The nth term of a sequence is 3n + 5.

Here, a_n = 3n+5

a_n-1 = 3(n-1) +5

3n-3+5 = 3n+2

Now, a_n-a_n-1 = (3n+5)-(3n+2) = 3

which does not depend on n i.e., it is constant.

∴ Given sequence is in A.P.

Question 13. For what value of k will k + 9, 2k- 1 and 2k +7 are the consecutive terms of an A.P?
Solution:

Since k + 9, 2k- 1 and 2k + 7are in A.P.

So, there must be a common difference.

i.e., (2k- 1) – (k + 9) = (2k + 7) – (2k- 1)

⇒ k- 10 = 8

⇒ k= 18

The value of k= 18.

Question 14. Find how many integers between 200 and 500 are divisible by 8.
Solution:

Integers between 200 and 500 which are divisible by 8 are as follows :

∴ 208, 216, 224, 232, …, 496

This forms an A.P. whose first term = 208, common difference = 8 and last term = 496. Let there be n terms.

∴ a_n = 496

⇒ a+ (n -1)<d = 496

⇒ 208 + (n- 1)8 = 496

⇒ (n- 1)8 = 496-208 = 288

⇒ \(n-1=\frac{288}{8}\)

∴ n-1 = 36

∴ n = 37

Hence, 37 integers are between 200 to 500 which are divisible by 8.

Question 15. If m times the with term of an A.P. is equal to n times the mth term and m ≠ n, show that its (m + n)th term is zero.
Solution:

Let the first term and the common difference of the A.P. be ‘a and ‘d’ respectively.

Given that

m.a_m = n.a_n

⇒ \( m\{a+(m-1) d\}=n\{a+(n-1) d\}\)

⇒ \(a m+\left(m^2-m\right) d=a n+\left(n^2-n\right) d\)

⇒ \(a(m-n)+\left\{\left(m^2-n^2\right)-m+n\right\} d=0\)

⇒ \(a(m-n)+\{(m-n)(m+n)-1(m-n\} d=0\)

⇒ \(a(m-n)+(m-n)(m+n-1) d=0\)

⇒ \((m-n)\{a+(m+n-1) d\}=0\)

⇒ \(a+(m+n-1) d=0\) (…m=n)

⇒ \(a_{m+n}=0\)

∴ (m + n)th term of the given A.P. is zero.

Question 16. If the term of an A.P. is \(\frac{1}{n}\) and its nth term be \(\frac{1}{m}\) then shows that its (nm)th term is 1.
Solution:

Let the first term and the common difference of the A.P. be ‘a’ and ‘d’ respectively

∴ \(a_m=\frac{1}{n} \quad \Rightarrow \quad a+(m-1) d=\frac{1}{n}\) ….(1)

and \(a_n=\frac{1}{m} \quad \Rightarrow \quad a+(n-1) d=\frac{1}{m}\)

Subtract equation (2) from equation (1), we get

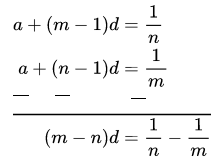
⇒ \((m-n) d=\frac{m-n}{n m}\)

⇒ \(d=\frac{1}{m n}\)

put \(d=\frac{1}{m n}\)

⇒ \( a+(m-1) \cdot \frac{1}{m n}=\frac{1}{n}\)

⇒ \(a+\frac{1}{n}-\frac{1}{m n}=\frac{1}{n}\)

⇒ \(a=\frac{1}{m n}\)

⇒ \(a_{m n}=a+(m n-1) d\)

⇒ \(\frac{1}{m n}+(m n-1) \cdot \frac{1}{m n}=\frac{1}{m n}+1-\frac{1}{m n}=1\)

∴ (mn)th term of the A.P. = 1

Question 17. Find the 7th term from the end of the A.P. : 3, 8, 13, 18,….98
Solution:

Here, l = 98,

d = 8-3 = 13-8 = 5,

n = 7

∴ 7th term from the end =l- (7 – 1)d

= 98 – 6 x 5

= 98 – 30

= 68

Alternative Method :

Write the A.P. in reverse order 98, …18, 13,8,3

Here, a = 98

d = 3-8 = 8- 13 = -5

a₇ = a + (7 – 1)d

= 98 + 6 x (-5) = 98- 30 = 68

which is the 7th term from the end of the given A.P.

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Sum Of n Terms Of An A.P.

Let the first term and common difference of an A.P. be ‘a’ and ‘d’          respectively. Let the A.P. contain ‘n’ terms and the last term be ‘l’.

∴ l = a + (n-1)d ….(1)

Now. a sum of n terms

S_n = a + (a + d) + + (1-d)+l …..(2)

In reverse order

S_n = l + (l + d) + + (a-d)+a …..(3)

Adding equations (2) and (3), we get

2S_n = (a + l) + (a + l) + …… + (a+l) + (a +l) (no.of terms = n)

2S_n = n(a+l)

⇒ \(S_n=\frac{n}{2}(a+l)\)

⇒ \(S_n=\frac{n}{2}[a+a+(n-1) d]\)

From eq.(1)

⇒ \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Sum Of n Terms Solved Examples

Question 1. Find the sum of n terms of the series

⇒ \(\left(4-\frac{1}{n}\right)+\left(4-\frac{2}{n}\right)+\left(4-\frac{3}{n}\right)+\ldots\)

Solution:

We are given the series

⇒ \(S=\left(4-\frac{1}{n}\right)+\left(4-\frac{2}{n}\right)+\left(4-\frac{3}{n}\right)+\ldots \text { to } n \text { terms }\)

⇒ \((4+4+4+\ldots \text { to } n \text { terms })-\left(\frac{1}{n}+\frac{2}{n}+\frac{3}{n}+\ldots \text { to } n \text { terms }\right)\)

⇒ \(4 n-\frac{1}{n}(1+2+3+\ldots \text { to } n \text { terms })\)

⇒ \(4 n-\frac{1}{n}\left[\frac{n}{2}\{2 \times 1+(n-1) \times 1\}\right] \quad( a=1, d=1)\)

⇒ \(4 n-\frac{1}{n}\left[\frac{n}{2}(n+1)\right]=4 n-\frac{n+1}{2}=\frac{8 n-n-1}{2}=\frac{1}{2}(7n-1)\)

Hence, the required sum of n terms is \(\frac{1}{2}(7 n-1) \text {.}\)

Question 2. Find the sum of the following series : 5 + (-41) + 9 +(-39) + 13 + (-37)+ 1 7 + … + (-5) + 81 + (-3)
Solution:

Let 5 = 5 + (-41) + 9 + (-39)+ 13 + (-37) + 17 + … + (-5) + 81 + (-3)

= (5 + 9+ 13 + 17 + … + 81) – (41 + 39 + 37 + … + 3)

= S₁-S₂

where, ,S₁ = 5 + 9+ I3 + 17 + … + 81

It is an A.P. with a₁ = 5,d = 9- 5 = 4

Let there be n terms.

∴ a_n = 81

⇒ a₁ + (n- 1)d = 81

⇒ 5 + (n – 1)4 = 81

⇒ (n-1)4 = 76

⇒ n-1= 19

⇒ n = 20

∴ S₁ = Sum of 20 terms with first term 5 and last term 81

⇒ \(\frac{20}{2}[5+81]=10 \times 86\)

=860

and S₂ = 41 + 39 + 37 + … + 3

It is also an A.P. with a1 = 41, d = 39 – 41 = 2

Let there be n terms.

∴ a_n = 3

⇒ a₁ + (n- 1)d = 3

⇒ 41 + (n – 1)(-2) = 3

⇒ (n- 1)(-2)=-38

⇒ n- 1 = 19

⇒ n=20

∴ S₂ = Sum of 20 terms with first term 41 and last term 3

⇒ \(\frac{20}{2}[41+3]\)

⇒ 10×44 = 440

From equation (1),

S = S₁ – S₂

⇒ S = 860- 440 = 420

Question 3. The first term of an A.P. is 5, the last term is 45 and the sum of all its terms is 150.

Find the number of terms and the common difference of the A.P.

Solution:

Given

The first term of an A.P. is 5, the last term is 45 and the sum of all its terms is 150.

Let a number of terms = n, the first term is a = 5 and the last term is l = 45.

We have, S_n = 150

⇒ \(\frac{n}{2}[a+l]=150\)

⇒ \(\frac{n}{2}(5+45)=150\)

n = 6

Now, l = 45 i.e., nth term = 45

⇒ a + (n-1)d = 45

⇒ 5 + (6- 1)d = 45

⇒ 5d = 40

⇒ \(d=\frac{40}{5}\)

= 8

Hence, number of terms = 6 and common difference = 8

Question 4. Solve for x: 5+ 13 + 21 +……. +x = 2139
Solution:

Given

5+ 13 + 21 +……. +x = 2139

Here, a = 5,d= 13-5 = 8

Let T_n=x

∴ S_n = 2139

∴ \(\frac{n}{2}[2 \times 5+(n-1) 8]=2139\)

⇒ n(5 + 4n – 4)= 2139

⇒ 4n² + n- 2139 = 0

⇒ 4n² – 92n + 93n -2139 = 0

⇒ (n – 23) (4n + 93) = 0

∴ n = 23 or \(n=-\frac{93}{4}\)

since n > 0, we have n = 23

x = T_n = a + (n- 1 )d = 5 + (23 – 1 )(8)

x = 181

Question 5. How many terms of an A.P. -6, \(\frac{-11}{2}\),-5 are needed to give the sum -25? Explain the double answer.
Solution:

Here, a= -6, \(d=\frac{-11}{2}-(-6)=\frac{1}{2}\)

Let -25 be the sum of it terms of this A.P. (n ∈ N)

Using \(S_n=\frac{n}{2}[2 n+(n-1) d]\)

⇒ \(-25=\frac{n}{2}\left[2(-6)+(n-1)\left(\frac{1}{2}\right)\right]\)

⇒ \(-50=n\left(-12+\frac{n-1}{2}\right)\)

⇒ \(-50=n\left(\frac{n-25}{2}\right)\)

⇒ -100 = n²– 25n

⇒ n²-25n+ 100 = 0

⇒ (n – 5) (n – 20) = 0

∴ n = 5, 20

Both the values of n are natural and therefore, admissible.

Explanation of Double Answer:

S₂₀ = \( -6-\frac{11}{2}-5-\frac{9}{2}-4-\frac{7}{2}-3-\frac{5}{2}-2-\frac{3}{2}-1-\frac{1}{2} +0+\frac{1}{2}+1+\frac{3}{2}+2+\frac{5}{2}+3+\frac{7}{2} \)

⇒ \(-6-\frac{11}{2}-5-\frac{9}{2}-4\)

⇒ S₅

Question 6. Find the sum of all odd numbers lying between 100 and 200.
Solution:

The series formed by odd numbers lying between 100 and 200 is 101 + 103 + 105 + … + 199

Here, a = 101,

d= 103- 101 = 105- 103 = 2

Let, a_n= 199

⇒ 101 + (n- 1)²= 199

⇒ (n- 1)² = 98

⇒ n-1 = 49

⇒ n = 50

Now, \(S_{50}=\frac{50}{2}(101+199)\)

=7500

The sum of all odd numbers lying between 100 and 200 =7500

Question 7. If a_n = 3 – 4n, show that a₁,a₂,a₃, … form an A.P. Also find S₂₀.
Solution:

Given

a_n = 3 – 4n

a_n = 3 – 4n

a_n-1 = 3-4(n-1)

= 3-4n+4=7-4n

∴ a_n-a_n-1 = (3-4n)-(7-4n) = -4n

Which does not depend on ‘n’ i.e., the difference of two consecutive terms is constant.

∴ Given sequence is in A.P.

Now, a₁ = a = 3 – 4(1 ) = -1 ,

d = -4

∴ \( S_{20}=\frac{20}{2}[2 a+(20-1) d]\)

⇒ \(=10[2(-1)+19(-4)]\)

=-780

Question 8. If the sum of the first 6 terms of an A.P. is 36 and that of the first 1 6 terms is 256, find the sum of the first 10 terms.
Solution:

Given

The sum of the first 6 terms of an A.P. is 36 and that of the first 1 6 terms is 256

Let the first term and common difference of A.P. be ‘a’ and ‘d’ respectively.

S₆ = 36

⇒ \(\frac{6}{2}[2 a+(6-1) d]=36\)

2a + 5d= 12 ……(1)

5,6 = 256

⇒ \(\frac{16}{2}[2 a+(16-1) d]=256\)

2a + 15d = 32 …..(2)

Subtract eq. (1) from eq. (2), we get

⇒ \(\begin{array}{r}
2 a+15 d=32 \\
2 a+5 d=12 \\
-\quad-\quad- \\
\hline 10 d=20
\end{array}\)

d = 2

put d = 2 in eq. (1), we get

2a + 5(2) = 12

⇒ 2a = 2

⇒ a = 1

Now, the sum of first 10 terms 510 = \(\frac{10}{2}[2 a+(10-1) d]=5[2(1)+9(2)]\)

= 100

The sum of first 10 terms = 100

Question 9. The sum of the first V terms of an A.P. whose first term is 8 and the common difference is 20, is equal to the sum of the first 2, i terms of another A.P. whose first term is -30 and the common difference is 8. Find ‘n’.
Solution:

Given

The sum of the first V terms of an A.P. whose first term is 8 and the common difference is 20, is equal to the sum of the first 2, i terms of another A.P. whose first term is -30 and the common difference is 8.

For first A.P., a = 8, d = 20

∴ \( S_n=\frac{n}{2}[2(8)+(n-1)(20)]\)

⇒ \(n(8+10 n-10)=10 n^2-2 n \)

For second A.P., a = -30, d = 8

⇒ \(S_{2 n}=\frac{2 n}{2}[2(-30)+(2 n-1)(8)]\)

⇒ \(n(-60+16 n-8)=16 n^2-68n\)

Question 10. If the pth term of an A.P. is x and the qth term is y. Show that the sum of first (p + q) terms is \(\frac{p+q}{2}\left\{x+y+\frac{x-y}{p-q}\right\}\)
Solution:

Given

The pth term of an A.P. is x and the qth term is y.

Let first term = n and common difference = d

Now, T_p = x a + (p- 1)d = x …(1)

and T_q + a(q- 1)d =y …(2)

Subtracting eq. (2) from eq. (1), we get

(p – q)d = x- y

⇒ \(d=\frac{x-y}{p-q}\)

Now, if you put the value of d in eq. (1 ) or (2), and find a then it will be a very tedious job. So, don’t find ‘a we will simplify especially:

⇒ \(S_{p+q}=\frac{p+q}{2}[2 a+(p+q-1) d]\)

Bifurcate the terms inside the bracket as

⇒ \(S_{p+q}=\frac{p+q}{2}[\{a+(p-1) d\}+\{a+(q-1) d\}+d]\)

⇒ \(S_{p+q}=\frac{p+q}{2}\left[x+y+\frac{x-y}{p-q}\right]\) [From (1) ,(2) and (3)]

Question 11. The sum of the first six terms of an A.P. is 42. The ratio of its 10th term to 30th term is 1 : 3. Calculate the first term and 1 3th term of the A.P.
Solution:

Given

The sum of the first six terms of an A.P. is 42. The ratio of its 10th term to 30th term is 1 : 3.

Let the first term and common difference of A.P. be V and ‘d’ respectively.

Given that,

⇒ \(\frac{a_{10}}{a_{30}}=\frac{1}{3}\)

⇒ \(\frac{a+9 d}{a+29 d}=\frac{1}{3}\)

⇒ 3a + 27d = a + 29d

⇒ 2a = 2d

⇒ a=d

and, S₆ = 42

⇒ \(\frac{6}{2}(2 a+5 d)=42\)

3(2 d+5 d)=42

d = 2

a = 2

a₁₃ = a+12d

= 2 + 12 × 2

= 26

The first term and 13th term of the A.P is 2 and 26.

Question 12. The ratio between the sum of fird=st n terms of two A.P.’s are in the ratio (7n-5): (5n+17). Find the ratio of their 10th terms.
Solution:

Given

The ratio between the sum of fird=st n terms of two A.P.’s are in the ratio (7n-5): (5n+17).

Let a₁ and d₁, be the first term and common difference of first A.P and let a₂ and d₂ be the first term and common difference of other A.P.

∴ \( \frac{\frac{n}{2}\left[2 a_1+(n-1) d_1\right]}{\frac{n}{2}\left[2 a_2+(n-1) d_2\right]}=\frac{7 n-5}{5 n+17}\)

⇒ \(\frac{2 a_1+(n-1) d_1}{2 a_2+(n-1) d_2} =\frac{7 n-5}{5 n+17}\)

⇒ \(\frac{a_1+\left(\frac{n-1}{2}\right) d_1}{a_2+\left(\frac{n-1}{2}\right) d_2}=\frac{7 n-5}{5 n+17}\)

Replace \(\frac{n-1}{2}\) by 9

⇒ \(\frac{n-1}{2}\)

⇒ n = 19

∴ From eq. (1)

⇒ \(\frac{a_1+9 d_1}{a_2+9 d_2}=\frac{7(19)-5}{5(19)+17}\)

⇒ \(\frac{T_{10}}{T_{10}^*}=\frac{128}{112}=\frac{8}{7}\)

∴ \(T_{10}: T_{10}^*=8: 7\)

Question 13. If the sum of the first ‘m’ terms of an A.P. be V and the sum of its first ‘n’ terms is ‘m’. then show that the sum of its first (m + n) terms is -(m +n).
Solution:

Given

The sum of the first ‘m’ terms of an A.P. be V and the sum of its first ‘n’ terms is ‘m’.

Let the first term and common difference of the A.P. be ‘a’ and ‘d’ respectively.

Given that,

S_m = n

⇒ \(\frac{m}{2}[2 a+(m-1) d] =n \)

⇒ \(2 a m+\left(m^2-m\right) d =2 n\) ….(1)

and S_n =m

⇒ \(\frac{n}{2}[2 a+(n-1) d] =m\)

⇒ \(2 a n+\left(n^2-n\right)d =2 m\) …..(2)

Subtract eq. (2) from eq. (1), we get

⇒ 2a(m – n) + {(m2 – m2) – (m – n))d = 2(n – m)

⇒ 2a(m – n) + {(m – n) (m + n) – (m – n)}d = -2(m – n)

⇒ 2d(m – n) + (m – n) (m + n – 1)d = -2(m – n)

⇒ 2a + (m + n – l)d = -2 …..(3)

Now, \(S_{m+n}=\frac{m+n}{2}\{2 a+(m+n-1) d\}\)

⇒ \(=\frac{m+n}{2}(-2)=-(m+n)\)

Hence proved.

Question 14. If the sum of first n, 2n and 3n terms of an A.P. be S1, S2 and S3 respectively, then prove that: S₃ = 3(S₂ – S₁)
Solution:

Given

The sum of first n, 2n and 3n terms of an A.P. be S1, S2 and S3 respectively,

Let the first term and common difference of the A.P. be V and ld’ respectively.

S₁ = sum of first ‘n’ terms

⇒ \(S_1=\frac{n}{2}[2 a+(n-1) d]\) ….(1)

S₂ = sum of first ‘2n terms

⇒ \(\frac{2 n}{2}[2 a+(2 n-1) d]\)

⇒ \(S_2=\frac{n}{2}[4 a+(4 n-2) d]\) ….(2)

and S₃ = sum of first ‘3n’ terms

⇒ \(S_3=\frac{3 n}{2}[2 a+(3 n-1) d]\) …..(3)

⇒ \(S_2-S_1=\frac{n}{2}[4 a+(4 n-2) d]-\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(\frac{n}{2}[4 a+(4 n-2) d-2 a-(n-1) d]=\frac{n}{2}[2 a+(3 n-1) d]\)

⇒ \(3\left(S_2-S_1\right)=\frac{3 n}{2}[2 a+(3 n-1) d]=S_3\)

Hence proved

Question 15. If the ratio of the sum of the first m and n terms of an A.P. is m²:n², show that the ratio of its mth and nth terms is (2m – 1) : (2n – 1).
Solution:

Given

The ratio of the sum of the first m and n terms of an A.P. is m²:n²

⇒ \(\frac{S_m}{S_n}=\frac{\frac{m}{2}[2 a+(m-1) d]}{\frac{n}{2}[2 a+(n-1) d]}=\frac{m^2}{n^2} \)

⇒ \(\frac{2 a+(m-1) d}{2 a+(n-1) d}=\frac{m}{n}\)

⇒ \(\frac{a+\left(\frac{m-1}{2}\right) d}{a+\left(\frac{n-1}{2}\right) d}=\frac{m}{n} \ldots\)

Caution

Some students write as :

put \(\frac{m-1}{2}=m-1 \quad \Rightarrow \quad m-1=2 m-2\)

m-2m = -2+1

m = 1

which is wrong.

Here has mixed up the m’s of \(\frac{m-1}{2} \text { and } m-1\)

We want, \(\frac{T_m}{T_n} \text { i.e., } \frac{a+(m-1) d}{a+(n-1) d}\)

So, we replace \(\frac{m-1}{2}\) as m-1

i.e., m-1 as 2(m – 1)

⇒ m – 1 as 2m – 2

⇒ m as 2m -2+1

i.e., replace m by 2m – 1

Similarly, replace n by 2n – 1 in eq. (1), we get

∴ \( \frac{a+(m-1) d}{a+(\dot{n}-1) d}=\frac{2 m-1}{2 n-1}\)

i.e., \(\frac{T_m}{T_n}=\frac{2 m-1}{2 n-1}\)

Question 16. An A.P. consists of 37 terms. The sum of the three middlemost terms is 225 and the sum of the last three terms is 429. Find the A.P.
Solution:

Given

An A.P. consists of 37 terms. The sum of the three middlemost terms is 225 and the sum of the last three terms is 429.

Total number of terms (n) = 37, which is odd

∴ Middle term = \(\frac{37+1}{2}\) th term = 19th term

So, 3 middle most terms are 18th, 19th and 20th

∴ T₁₈ +T₁₉ + = 225

⇒ a + 17d + a+ 18d + a+ 19d- 225

⇒ 3a + 54d = 225

⇒ a=18d = 75 ……(1)

Also, a sum of the last 3 terms = 429

T₃₅ + T₃₆ + T₃₇ = 429

⇒ a + 34d + a + 35d + a + 36d = 429

⇒ 3a + 105d = 429

⇒ a + 35d = 143 ……(2)

Solving, (1) and (2), we get d- 4 and a = 3

∴ Required A.P. is a, a + d, a + 2d, a + 3d,…

i.e., 3, 3 + 4, 3 + 2(4), 3 + 3(4)

i.e., 3, 7, 11, 15, …

The A.p = 3, 7, 11, 15, …

Question 17. 25 trees are planted in a straight line 5 metres apart from each other. To water them the gardner must bring water for each tree separately from a well 10 metres from the first tree in line with trees. Find the distance he will move in order to water all the trees beginning with the first if he starts from the well.
Solution:

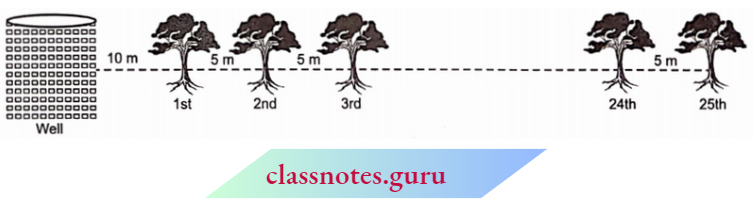
Distance Covered by Gardner from well to well :

⇒ \(\underbrace{10+10}_{\text {well-1-well }}+\underbrace{(10+5)+(10+5)}_{\text {well-2-well }}+\underbrace{(10+2 \times 5)+(10+2 \times 5)}_{\text {well-3-well }}\)

⇒ \(+\underbrace{(10+23 \times 5)+(10+23 \times 5)}_{\text {well-24-well }}+\underbrace{(10+24 \times 5)}_{\text {well to } 25 \text { th tree }}\)

2[10 + (10 + 5) + (10 + 2×5) + ……+(10 + 23×5)] + (10 + 24×5)

⇒ \(2 \underbrace{[10+15+20+\ldots+125]}_{24 \text { terms }}+(10+120)\)

⇒ \(2 \times \frac{24}{2}[10+125]+130=24 \times 135+130=3240+130=3370 \mathrm{~m}\)

Question 18. A child puts one five-rupee coin of her savings in the piggy bank on the first day. She increases her savings by one five-rupee coin daily. If the biggy bank can hold 1 90 coins of five-rupees in all, find the number of days she can continue to put the five-rupee coins into it and find the total money she saved. Write your views on the habit of saving.
Solution:

Child’s savings day-wise are,

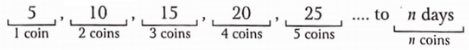
We can have at most 190 coins

i.e., 1+2 + 3+ 4 + 5 + …to n terms =190

⇒ \(\frac{n}{2}[2 \times 1+(n-1) 1]=190\)

⇒ n(n + 1) = 380

⇒ n² +n- 380 = 0

⇒ (n + 20) (n- 19) = 0

⇒ n = -20

or n = 19

But many coins cannot be negative

∴ n = 19

So, number of days =19

Total money she saved = 5+10+15+20 + … upto 19 terms

⇒ \(\frac{19}{2}[2 \times 5+(19-1) 5]\)

⇒ \(\frac{19}{2}[100]=\frac{1900}{2}\)

=950

So, number of days = 19

and total money she saved = ₹950

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Arithmetic Mean Of Two Numbers

If three numbers are in A.P., then the middle term is called the arithmetic mean of the remaining two numbers.

Let A be the arithmetic mean of a and b.

∴ a, A, b are in A.P.

⇒ A – a = b – A

⇒ 2A = a +b

⇒ A= \(\frac{a+b}{2}\)

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Selection Of Continuous Terms Of A.P.

1. Three consecutive terms in A.P. a— d, a, a + d (common difference is d)
2. Four consecutive terms in A.P. a — 3d, a — d, a+ d, a + 3d (common difference is 2d)
3. Five consecutive terms in A.P. a — 2d, a — d, a, a + d, a + 2d (common difference is d)

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Selection Of Continuous Terms Of A.P. Solved Examples

Question 1. Find the value of x for which (x + 2), 2x, (2x + 3) are three consecutive terms of A.P. 
Solution:

Given

(x + 2), 2x, (2x + 3) are three consecutive terms of A.P.

∴ 2x = \(\frac{(x+2)+(2 x+3)}{2}\)

⇒ 4x = 3x + 5

x = 5

The value of x = 5.

Question 2. Split 207 into three parts such that these are in A.P. and the product of the two smaller parts is 4623.
Solution:

Let three parts be a- d, a, a + d.

∴ a-d + a+ a+ d = 207

⇒ 3a = 207

⇒ a = 69

and (a-d)-a = 4623

⇒ (69-d)69 = 4623

⇒ 69 – d = 67

⇒ d = 2

∴ a-d = 69 – 2 = 67

a= 69

a+d = 692 = 71

⇒ three parts are 67,69,71

Question 3. The angles of a triangle are in A.P. The greatest angle is twice the least. Find all the angles of the triangle.
Solution:

Given

The angles of a triangle are in A.P. The greatest angle is twice the least.

Let angles of the triangle be a – d, a, a + d

∴ a – d+a+a + d = 180°

⇒ 3a = 1 80°

⇒ a = 60°

and a + d = 2(a – d)

⇒ 60° + W = 2(60°- d)

⇒ 60° + d = 120° -2d

⇒ 3d = 60°

⇒ d = 20°

∴ a- d = 60°- 20° = 40°

a +d = 60° + 20° = 80°

∴ Three angles of triangle are 40°, 60°, 80°

Question 4. The angles of a quadrilateral are in A.P. and their common difference is 10°. Find the angles.
Solution:

Given

The angles of a quadrilateral are in A.P. and their common difference is 10°.

Let the angles of the quadrilateral be

a, a + 10°, a + 20°, a + 30°   (∵ common difference is 10°)

∴ a + (a + 10°) + (a + 20°) + (a + 30°) = 360°

⇒ 4a + 60° = 360°

⇒ 4a = 300°

⇒ a = 75°

∴ a+ 10°= 75°+ 10° = 85°

a + 20° = 75° + 20° = 95°

a + 30° = 75° + 30°= 105°

Hence, the angles are 75°, 85°, 95°, 105°.

Alternative Method :

Let the four angles of a quadrilateral are

a – 3d, a – d, a + d and a + 3d

∴ Here the common difference is 2d (remember)

∴ (a – 3d) + (a-d) + (a + d) + (a + 3d) = 360°

4a = 360°

⇒ a = 90°

the common difference is given to be 10°

i.e., 2d = 10°

⇒ d = 5°

Four angles are a – 3d = 90°- 3(5°) = 75°,

a – d 90°- 5° = 85°,

a + d = 90° + 5° = 95°,

a + 3d = 90° + 3(5°) = 105°

Question 5. There are m arithmetic means between 5 and -16 such that the ratio of the 7th mean to the (m -7)th mean is 1: 4. Find the value of m.
Solution:

Given

There are m arithmetic means between 5 and -16 such that the ratio of the 7th mean to the (m -7)th mean is 1: 4

Let A₁, A₂,…….A_m be m arithmetic means between 5 and -16.

∴ 5,A₁, A₂, A₃,…..A_m, -16 are in A.P.

∴ \(T_{m+2}=-16\)

⇒ \(5+(m+1) d=-16\)

⇒ \(d=\frac{-21}{m+1}\)

⇒ \(\frac{A_7}{A_{m-7}}=\frac{1}{4}\)

⇒ \(\frac{T_8}{T_{m-6}}=\frac{1}{4}\)

⇒\(\frac{5+7 d}{5+(m-7) d}=\frac{1}{4}\)

⇒ \(\frac{5+7\left(\frac{-21}{m+1}\right)}{5+(m-7)\left(\frac{-21}{m+1}\right)} \frac{1}{4}\)

⇒ \(20-\frac{588}{m+1}=5-\frac{21(m-7)}{m+7}\)

⇒ 20m + 20- 588 = 5m +5- 21m + 147

⇒ 36m = 720

⇒ m = 20

The value of m = 20.

Question 6. The digits of a positive number of three digits are in A.P. and their sum is 15. The number obtained by reversing the digits is 594 less than the original number. Find the number.
Solution:

Given

The digits of a positive number of three digits are in A.P. and their sum is 15. The number obtained by reversing the digits is 594 less than the original number.

Let the 3 digits in A.P. at units, tens and hundredth places be a – d, a and a + d.

According to the first condition,

(a -d) +a + (a +d) = 15

⇒ 3a = 15

a = 5 …(1)

The number is (a – d) + 10a + 100 (a + d)….(1)

i.e., 111+ 99d …(2)

The number; on reversing the digits is

(a + d) + 1 0a + 100(A — d) i.e., 111+ 99d

∴ According to the 2nd condition,

111a- 99d = (11la + 99d) – 594

⇒ 198d = 594

⇒ d = 3 …(3)

∴ Required number = 111a + 99d [from (2)]

= 111(5) + 99(3) [from (1) and (3)]

= 555 + 297

= 852

Required number = 852

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Exercise 5.1

Question 1. In which of the following situations, does the list of numbers involved make an arithmetic progression, and why?

1. File taxi fare after each km when the fare is ₹15 for the first km and ₹8 for each additional km.
2. The amount of air present in a cylinder when a vacuum pump removes — the
   air remaining in the cylinder at a time.
3. The cost of digging a well after every metre of digging, when it costs ₹150 for the first metre rises by ₹50 for each subsequent metre.
4. The amount of money in the account every year, when ₹10000 is deposited at compound interest at 8% per annum.

Solution :

1. Fare of first kilometre = ₹15 

Fare of 2 kilolnetre = ₹(15 + 8) = ₹23

Fare of 3 kilometre = ₹(23 + 8) = ₹31

Fare of 4 kilometre = ₹(3 1 + 8) = ₹39

Now, a₁ = 15, a₂ = 23, a₃ = 31, a₄ = 39

a₂ -a₁ = 23 – 15 = 8

a₃-a₂ = 31 -23 = 8

a₄ – a₃ = 39 – 31 = 8

∴ The difference between two consecutive terms is constant.

∴ The taxi fare after each kilometre is in A.P

2. Let the initial volume of air in the cylinder = V

In the first pump,

air remove = \(\frac{V}{4}\)

Remaining air = \(V-\frac{V}{4}=\frac{3 V}{4}\)

In the second pump,

air remove = \(\frac{1}{4} \times \frac{3 V}{4}=\frac{3 V}{16}\)

Remaining air = \(\frac{3 V}{4}-\frac{3 V}{16}=\frac{9 V}{16}\)

Now, \(a_1=V, a_2=\frac{3 V}{4}, a_3=\frac{9 V}{16}\)

∴ \(a_2-a_1=\frac{3 V}{4}-V=-\frac{V}{4}\)

and \(a_3-a_2=\frac{9 V}{16}-\frac{3 V}{4}=-\frac{3 V}{16}\)

∵ a₂– a₁ ≠ a₃– a₂

∴ The volumes of air are not in A.P.

3. The cost of digging of first metre = ₹150

The cost of digging of 2 metres = ₹.(150 + 50)

= ₹200

The cost of digging of 3 metres

= ₹ (150 + 50 + 50)

= ₹250

The cost of digging of 4 metres

= ₹(150 + 50 + 50 + 50)

= ₹300

Now, a1=₹150, a2 = 200,a3 = ₹250, a4 = ₹300

∴ a₂-a₁ = ₹200-₹150 = ₹50

a₃-a₂= ₹250 -₹200 = ₹50

a₄-a₃= ₹300- ₹250 = ₹50

∵  The difference between two consecutive terms is constant.

∵ The costs of digging each metre are in A.P.

4. Principal P = ₹10,000;

rate of interest R = ₹8%

Amount after 1 year,

⇒ \(A_1=P\left(1+\frac{R}{100}\right)^1=10,000\left(1+\frac{8}{100}\right)^1\)

⇒ \(=10,000 \times \frac{108}{100}=₹ 10,800\)

Amount after 2 years,

⇒ \(A_2=P\left(1+\frac{R}{100}\right)^2=10,000\left(1+\frac{8}{100}\right)^2\)

⇒ \(=10,000 \times \frac{108}{100} \times \frac{108}{100}=₹ 11664\)

Amount after 3 years

⇒ \(A_3=P\left(1+\frac{R}{100}\right)^3=10,000\left(1+\frac{8}{100}\right)^3\)

⇒ \(=10,000 \times \frac{108}{100} \times \frac{108}{100} \times \frac{108}{100}\)

= ₹12597.12

Now, A₂-A₁ = 11664- 10800 = 864

A₃-A₂= 12597.12- 11664 = 933.12

∵ The difference between two consecutive terms is not the same.

∴ Amounts are not in A.P.

Question 2. Write the first four terms of the A.P., when the first term a and the common difference d are given as follows :

1. a= 10, d= 10
2. a =- 2, d = 0
3. a = 4,d = -3
4. a=-1, d=\(\frac{1}{2}\)

Solution:

1. a = 10, d = 10

First term = a = 10

Second term =a + d= 10 + 10 = 20

Third term = a + 2d = 10 + 2 × 10 = 30

Fourth term = a + 3d = 1 0 + 3 × 1 0 = 40

∴ The first four terms of A.P. are 10, 20, 30, 40

2. a = -2, d = 0

First term = a = -2

Second term = a + d = -2 + 0 = -2

Third term = a + 2d = -2 + 2 × 0 = -2

Fourth term = a + 3d = -2 + 3 × 0=-2

∴ The first four terms of A.P. are -2, -2, -2, -2

3. a= 4, d = -3

First term = a = 4

Second term = a + d = 4 + (-3) = 1

Third term = a + 2d = 4 + 2(-3) = -2

Fourth term = a + 3d = 4 + 3(-3) =-5

∴ The first four terms of A.P are 4, 1, -2, -5

4. a=-1, d = \(\frac{1}{2}\)

First term =a = -1

Second term = a + d = \(-1+\frac{1}{2}=-\frac{1}{2}\)

Third term= a + 2d = \(-1+2 \times \frac{1}{2}=0\)

Fourth term= a + 3d = \(-1+3 \times \frac{1}{2}=\frac{1}{2}\)

∴ First four terms of A.P. are = \(-1,-\frac{1}{2}, 0, \frac{1}{2}\)

5. a =- 1.25, d = -0.25

First term = a = -1.25

Second term = a+d =-1.25 + (-0.25) = -1.50

Third term =a + 2d = -1.25 + 2(-0.25)

= -1.75

Fourth term = a + 3d =- 1.25 + 3(-0.25.)

= -2.00

∴ The first four terms of A.P. are

= -1.25, -1.50, -1.75, -2.00

Question 3. For the following A.Ps., write the first term and the common difference :

1. 3, 1,-1, -3,…
2. -5, -1,3, 7,…
3. \(\frac{1}{3}, \frac{5}{3}, \frac{9}{3}, \frac{13}{3}, \ldots\)
4. 0.6, 17,2.8,3.9,…

Solution:

1. 3, 1, -1, -3,…

First term a = 3

Common difference d = 1-3 = (-1)- 1 = -2

2. -5,-1, 3, 7,…

First term a = -5

Common difference d = -1-(-5) = 3-(-1) = 4

3. \(\frac{1}{3}, \frac{5}{3}, \frac{9}{3}, \frac{13}{3}, \ldots\)

First term a = \(\frac{1}{3}\)

Common difference d = \(\frac{5}{3}-\frac{1}{3}=\frac{9}{3}-\frac{5}{3}=\frac{4}{3}\)

4. 0.6, 17,2.8,3.9,…

First term a = 0.6

Common difference d= 1.7 – 0.6

= 2.8-17=1.1

Question 4. Which of the following are A.P.s? If they form an A.P., find the common difference d and write three more terms.

1. \(2,4,8,16,\)
2. \(2, \frac{5}{2}, 3, \frac{7}{2},\)
3. \(-1.2,-3.2,-5.2,-7.2,\)
4. \(-10,-6,-2,2,\)
5. \(3,3+\sqrt{2}, 3+2 \sqrt{2}, 3+3 \sqrt{2},\)
6. \(0.2,0.22,0.222,0.2222,\)
7. \(0,-4,-8,-12,\)
8. \(-\frac{1}{2},-\frac{1}{2},-\frac{1}{2},-\frac{1}{2},\)
9. \(1,3,9,27,\)
10. \(a, 2 a, 3 a, 4 a,\)
11. \(a, a^2, a^3, a^4,\)
12. \(\sqrt{2}, \sqrt{8}, \sqrt{18}, \sqrt{32},\)
13. \(\sqrt{3}, \sqrt{6}, \sqrt{9}, \sqrt{12},\)
14. \(1^2, 3^2, 5^2, 7^2,\)
15. \(1^2, 5^2, 7^2, 73,\)

Solution:

1. 2,4, 8, 16,…

Here, a₁ = 2, = 4, = 8, = 16

a₂– a₁ = 4- 2 = 2

a₃-a₂ = 8- 4 = 4

∵ a₂-a₁ ≠ a₃-a₂

∴ Given sequence is not an A.P.

2. \(2, \frac{5}{2}, 3, \frac{7}{2},\)

Here, \(a_1=2, a_2=\frac{5}{2}, a_3=3, a_4=\frac{7}{2}\)

⇒ \(a_2-a_1=\frac{5}{2}-2=\frac{1}{2}\)

∴ \(a_3-a_2=3-\frac{5}{2}=\frac{1}{2}\)

⇒ \(a_4-a_3=\frac{7}{2}-3=\frac{1}{2}\)

∵ \(a_2-a_1=a_3-a_2=a_4-a_3=\frac{1}{2}\)

∴ Given sequence is an A.P. and d = \(\frac{1}{2}\)

Now, fifth term \(a_5=a_4+d=\frac{7}{2}+\frac{1}{2}=4\)

Sixth term \(a_6=a_5+d=4+\frac{1}{2}=\frac{9}{2}\)

Seventh term \(a_7=a_6+d=\frac{9}{2}+\frac{1}{2}=5\)

∴ Next three terms of A.P. = 4, \(\frac{9}{2}\),5

3. -1.2, -3.2, -5.2, -7.2, …

Here a₁ = -1.2, a₂ =-3.2, a₃ = -5.2, a₄ =-7.2

∴ a₂-a₁ =(-3.2) -(-1.2) =-3.2+ 1.2= -2

a₃ -a₂ = (-5-2) – (-3.2) =-5.2 + 3.2 =-2

a₄ – a₃ = (-7.2) – (-5.2) = -7.2 + 5.2 =- 2

∵ a₂ – a₁ = a₃ – a₂ = a₄ – a₃ = -2

∴ Given sequence is A.P. and d = -2

Now, fifth term a₅= a₄ + d = -7.2 + (-2) = -9.2

Sixth term a₆ = a₅ + d = -9.2 + (-2) = -1 1 .2

Seventh term a₇ =a₆+ d = -11.2 + (-2) =-13.2

∴ Next three terms of A.P. =- 9.2, -1 1.2, -13.2

4. -10, -6, -2,2,…

Here a₁ = -10, a₂ =-6, a₃ =-2, a₄ = 2

∴ a₂-a₁=(-6)-(-10)=-6+ 10 = 4

a₃ – a₂ = (-2) – (-6) =-2 + 6 = 4

a₄ – a₃ = 2-(-2) = 2 + 2 = 4

∵ a₂-a₁ = a₃ – a₂ = a₄ – a₃ = 4

∴ Given sequence is A.P. and d = 4

Now, fifth term a₅ = a₄ + d = 2 + 4 = 6

Sixth term a₆ = a₅ + d = 6 + 4 = 10

Seventh term a₇ = + d = 1 0 + 4 = 14

∴ Next three-term of A.P. = 6, 10, 14

5. \(3,3+\sqrt{2}, 3+2 \sqrt{2}, 3+3 \sqrt{2},\)

Here, \(a_1=3, a_2=3+\sqrt{2}, a_3=3+2 \sqrt{2},\)

⇒ \(a_4=3+3 \sqrt{2}\)

⇒ \(a_2-a_1=(3+\sqrt{2})-3=\sqrt{2}\)

∴ \(a_3-a_2=(3+2 \sqrt{2})-(3+\sqrt{2})=\sqrt{2}\)

⇒ \(a_4-a_3=(3+3 \sqrt{2})-(3+2 \sqrt{2})=\sqrt{2}\)

∵ \(a_2-a_1=a_3-a_2=a_4-a_3=\sqrt{2}\)

∴ Given sequence is A.P. and d = \(\sqrt{2}\)

Now, fifth term \(a_5=a_4+d\)

⇒ \(3+3 \sqrt{2}+\sqrt{2}=3+4 \sqrt{2}\)

Sixth term \(a^6=a^5+d\)

⇒ \(3+4 \sqrt{2}+\sqrt{2}=3+5 \sqrt{2}\)

Seventh term \(a_7=a_6+d\)

⇒ \(3+5 \sqrt{2}+\sqrt{2}=3+6 \sqrt{2}\)

Next three terms of A.P.

⇒ \(3+4 \sqrt{2}, 3+5 \sqrt{2}, 3+6 \sqrt{2}\)

6. 0.2,0.22,0.222,0.2222, …

Here a₁ = 0.2, a₂ = 0.22, a₃ = 0.222, a₄ = 0.2222

∴ a₂-a₁=0.22-0.2 = 0.02

a₃-a₂ = 0.222 -0.22 = 0.002

∵ a₂ – a₁ = a₃ – a₂

∴ Given sequence is not an A.P.

7. 0, -4, -8, -12, …

Here, a₁ = 0, a₂ = -4, a₃ = -8, a₄ = -12

∴ a₂ – a₁ = -4 – 0 = – 4

a₃-a₂ =-8- (-4) =- 8 + 4 = -4

∵ a₄ – a₃ = -12 – (-8) =-12 + 8 =- 4

a₂ – a₁ = a₃ – a₂ = a₄ – a₃ = -4

∴ Given sequence is A.P. and d =-4

Now, fifth term a₅ =a₄ + d =-12 + (-4) =-16

Sixth term a₆ = a₅ + d = -16 + (-4) = -20

Seventh term a₇ = a6 + d = -20 + (-4) = -24

∴ Next three terms of A.P. =-16, -20, -24

8. \(-\frac{1}{2},-\frac{1}{2},-\frac{1}{2},-\frac{1}{2}, \ldots\)

Here, \( a_1=a_2=a_3=a_4=-\frac{1}{2}\)

∴ \(a_2-a_1=\left(-\frac{1}{2}\right)-\left(-\frac{1}{2}\right)=0\)

⇒ \(a_3-a_2=\left(-\frac{1}{2}\right)-\left(-\frac{1}{2}\right)=0\)

⇒ \(a_4-a_3=\left(-\frac{1}{2}\right)-\left(-\frac{1}{2}\right)=0\)

∴ a₂– 1 = a₃ – a₂ = a₄ – a₃ = 0

∴ Given sequence is A.P. and d = 0

Now, fifth term \(a_5=a_4+d=\frac{-1}{2}+0=-\frac{1}{2}\)

Sixth term \(a_6=a_5+d=\frac{-1}{2}+0=-\frac{1}{2}\)

Seventh term \(a_7=a_6+d=\frac{-1}{2}+0=-\frac{1}{2}\)

∴ Next three terms of A.P. = \(-\frac{1}{2},-\frac{1}{2},-\frac{1}{2}\)

9. 1,3, 9,27,…

Here, a₁ = 1, a₂ = 3, a₃ = 9, a₄ = 27

∴ a₂-a₁ = 3-1=2

a₃– a₂ = 9- 3 = 6

∵ a₂ -a₁ ≠ a₃– a₂

∴ Given sequence is not an A.P.

10. a, 2a, 3a, 4a

Here, a₁ = a, a₂ = 2a, a₂ = 3a, a₄ = 4a

∴ a₂-a₁ =2a – a = a

a₃ – a₂ = 3a – 2a =a

a₄ – a₃= 4a – 3a = a

a₂– a₁ = a₃ – a₂ =a₄– a₃ = a

∴ Given sequence is A.P. and d = a

Now, fifth term a₅ = a₄ + d = 4a + a = 5a

Sixth term a₆ = a₅ + d = 5a + a = 6a

Seventh term a₇ = a₆ + d = 6a + a = 7a

∴ Next three terms of A.P. = 5A, 6a, 7a

11. a, a², a³, a⁴ = ,…

Here, a₁ = a, a₂ = a², a₃ = a³, a₄ = a⁴

∴ a₂-a₁ = a² – a

a₃ – a₂ = a³-a²

a₂– a₁ ≠ a₃– a₂

∴ Given sequence is not an A.P.

12. \(\sqrt{2}, \sqrt{8}, \sqrt{18}, \sqrt{32}, \cdots\)

Here, \(=\sqrt{2}, 2 \sqrt{2}, 3 \sqrt{2}, 4 \sqrt{2}, \cdots\)

∴ \(a_1=\sqrt{2}, a_2=2 \sqrt{2}, a_3=3 \sqrt{2}, a_4=4 \sqrt{2}\)

⇒ \(a_2-a_1=2 \sqrt{2}-\sqrt{2}=\sqrt{2}\)

⇒ \(a_3-a_2=3 \sqrt{2}-2 \sqrt{2}=\sqrt{2}\)

⇒ \(a_4-a_3=4 \sqrt{2}-3 \sqrt{2}=\sqrt{2}\)

⇒ \(a_2-a_1=a_3-a_2=a_4-a_3\)

∴ Given sequence is A.P. and d = \(\sqrt{2}\)

Now, fifth term \(a_5=a_4+d=4 \sqrt{2}+\sqrt{2}=5 \sqrt{2}\)

Sixth term \(a_6=a_5+d=5 \sqrt{2}+\sqrt{2}=6 \sqrt{2}\)

Seventh term \(a_7=a_6+d=6 \sqrt{2}+\sqrt{2}=7 \sqrt{2}\)

∴ Next three terms of A.P. = \(5 \sqrt{2}, 6 \sqrt{2}, 7 \sqrt{2}\)

13. \(\sqrt{3}, \sqrt{6}, \sqrt{9}, \sqrt{12}, \ldots\)

Here, \(\quad a_1=\sqrt{3}, a_2=\sqrt{6}, a_3=\sqrt{9}, a_4=\sqrt{12}\)

⇒ \(a_2-a_1=\sqrt{6}-\sqrt{3}=\sqrt{3}(\sqrt{2}-1)\)

⇒ \(a_3-a_2=\sqrt{9}-\sqrt{6}=\sqrt{3}(\sqrt{3}-\sqrt{2})\)

⇒ \(a_2-a_1 \neq a_3-a_2\)

∴ Given sequence is not an A.P.

14. \(1^2, 3^2, 5^2, 7^2, \ldots\)

Here, \(\quad a_1=1^2, a_2=3^2, a_3=5^2, a_4=7^2\)

∴ \(a_2-a_1=3^2-1^2=9-1=8\)

⇒ \(a_3-a_2=5^2-3^2\)

⇒ \(=25-9=16\)

∵ \(a_2-a_1\neq a_3-a_2\)

∴ Given sequence is not an A.P.

15. \(1^2, 5^2, 7^2, 73, \ldots\)

Here, \(a_1=1^2, a_2=5^2, a_3=7^2, a_4=73\)

∴ \(a_2-a_1=5^2-1^2=25-1=24\)

⇒ \(a_3-a_2=7^2-5^2=49-25=24\)

⇒ \(a_4-a_3=73-7^2=73-49=24\)

∵ \(a_2-a_1=a_3-a_2=a_4-a_3=24\)

∴ Given sequence is not an A.P. and d = 24

Now, fifth term a₅ = a₄ + d = 73 + 24 = 97

Sixth term a₆= a₅+d = 97 + 24

= 121 = 11²

Seventh term a₇=a₆ + d= 121 + 24 = 145

∴ Next three terms of A.P. = 97, 11², 145

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Exercise 5.2

Question 1. Fill in the blanks in the following table given the first term. d the common difference and an nth term of the A.P. :

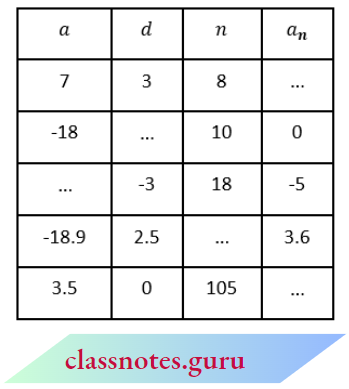
Solution:

1. Here, a = 7, d = 3. n= 8

∴ a_n – a + (n -1)d

= 7 + (8 – 1) 3

= 7 + 7 × 3=28

Therefore, a_n =28

2. a =-18, n = 10, a_n = 0

∴ a + (n – 1)d = a_n

⇒ -18 + (10- 1)d = 0

⇒ 9d = 18

⇒ d = 2

3. d =-3, n= 1 8, a_n =-5

∴ a + (n – 1 )d = a_n

⇒ a + (18 – 1) (-3) =-5

⇒ a-51 =- 5

⇒ a = -5 + 51 =46

4. a = -18.9, d = 2.5, a_n = 3.6

∴ a + (n -1)d = an

-18.9 + (n -1) × 2.5 = 3.6

⇒ (n- 1) × 2.5 =3.6+18.9 = 22.5

⇒ \(n-1=\frac{22.5}{2.5}=9\)

⇒ n = 9+1 = 10

5. a = 3.5, d = 0, n= 105

∴ a_n = a + (n -1 )d

= 3.5 + (105 – 1) × 0

= 3.5

Question 2. Choose the correct choice in the following and justify :

1. 30th term of the A.P.: 10, 7, 4, …, is
   1. 97
   2. 77
   3. -77
   4. -87
2. 11th term of the A.P.: -3, 2 …, is
   1. 28
   2. 22
   3. -38
   4. \(-48 \frac{1}{2}\)

Solution:

1. Given A.P.: 10,7,4…

Mere n= 10,d= 7- 10 = 4- 7 = -3, n =30

∴ a_n =a( n – 1 )d

⇒  a₃₀ = 10 + (30 – 1) (-3) = 10 – 87 – -77

2. Given A.P. : \(-3,-\frac{1}{2}, 2, \ldots\)

Here a = \(a=-3, d=-\frac{1}{2}-(-3)=2-\left(-\frac{1}{2}\right)=\frac{5}{2}\)

n = 11

∴ a_n = a + (n – 1 )d

⇒ \(a_{11}=-3+(11-1) \frac{5}{2}=-3+25=22\)

Question 3. In the following A.Ps., find the missing terms in the boxes :

Solution:

1. Here, first term a = 2

Third term = 26

⇒ a + (3-1)d = 26 2 + 2d = 26

⇒ 2d = 24

⇒ d= 12

∴ Second term = a + d = 2 + 12 = 14

∴ Term of the box = 14

2. Second term =13

⇒ a + (2-1)d = 13 (where a = first term and d = common difference)

⇒ a+d =13 ….(1)

and Fourth term = 3

⇒ a + (4 – 1 )d= 3 ⇒ a + 3d = 3 ….(2)

Subtracting equation (1) from equation (2),

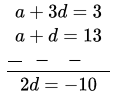
⇒ d = -5

Put the value of d in equation (1)

a + (-5) = 13 ⇒ a = 13+5

= 18

Third term a₂ + d = 13 + (-5) = 8

∴ Term of the boxes = 18. 8 respectively.

3. Here, first term a = 5

Fourth term \(a_4=9 \frac{1}{2}\)

⇒ \(a+3 d=\frac{19}{2}\)

⇒ \(5+3 d=\frac{19}{2}\)

⇒ \(3d=\frac{19}{2}-5=\frac{9}{2} \Rightarrow d=\frac{3}{2}\)

Now, second term \(a_2=a+d=5+\frac{3}{2}=\frac{13}{2}\)

Third term \(a_3=a_2+d=\frac{13}{2}+\frac{3}{2}=\frac{16}{2}=8\)

∴ Term of the boxes = \(\frac{13}{2}, 8\)

4. Here, first term a = -4

Sixth term = 6

⇒ a + 5d = 6 ⇒ -4 + 5d = 6

⇒ 5d = 6+4= 10

⇒ d =2

∴ Second term a₂ = a + d = -4 + 2 = -2

Third term a₃ = a₂ + d = -2 + 2 = 0

Fourth term a₄ = a₃+d = 0 + 2 = 2

Fifth term a₅= a₄ + d = 2 + 2 = A

∴ Term of the boxes = -2, 0, 2, 4 respectively.

5. Here, second term a₂ = 38

⇒ a + d= 38

Sixth term a₅ = -22

⇒ a + 5d = -22

Subtracting equation (1) from (2),

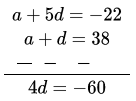
d = -15

Put the value of d in equation (1),

a + (-15) =38

⇒ a = 38+ 15=53

Third term a₃ = a₂ + d = 38 + (-15) = 23

Fourth term a₄ = a₃+d = 23 + (-15) = 8

fifth term a₅ = a₄ + d = 8 + (-15) -7

∴ Term of the boxes = 53, 23, 8, -7 respectively.

Question 4. Which term of the A.P. : 3, 8, 13, 18,… is 78?
Solution:

Given A.P. : 3, 8, 13, 18,…

Here a = 3, d = 8- 3 = 13-8 = 5

Let a_n = 78 ⇒ a + (n – 1 )d = 78

⇒ 3 + (11 – 1)5 = 78

⇒ (n- 1)5 =78-3 = 75

⇒ \(n-1=\frac{75}{5}=15\)

⇒ n= 15+ 1 = 16

∴ The 16th term is 78.

Question 5. Find the number of terms in each of the following A.Ps. :

1. 7, 13, 19,…, 205
2. 18, \(15 \frac{1}{2}\), 13…..-47

Solution:

Given A.P.: 7, 13, 19,…, 205

a=7

d= 13-7= 19- 13 = 6

Let an = 205

⇒ a + (n- l)d = 205

⇒ 7 + (n – 1)6 = 205

⇒ (n- 1)6 = 205-7 = 198

⇒ \(n-1=\frac{198}{6}=33\)

⇒ n = 33 + 1 = 34

∴ Number of terms in given A.P. = 34

2. Given A.P.:18, \(15 \frac{1}{2}\), 13…..-47

Here, a= 18

⇒ \(d=15 \frac{1}{2}-18=13-15 \frac{1}{2}=-2 \frac{1}{2}=\frac{-5}{2}\)

Let \(a_n=-47\)

⇒ \(a+(n-1) d=-47\)

⇒ \(18+(n-1)\left(\frac{-5}{2}\right)=-47\)

⇒ \((n-1)\left(\frac{-5}{2}\right)=-47-18=-65\)

⇒ \(n-1=(-65)\left(-\frac{2}{5}\right)=26\)

⇒ n = 26 + 1 = 27

∴ Number of terms in given A.P. = 27

Question 6. Check whether -150 is a term of the A.P. 1 1,8, 5. 2….
Solution:

Given A.P: 1 1, 8, 5, 2,…

⇒ a= 11, d=8- 11 =5- 8 = -3

Let a_n = -150

⇒ a + (n- 1 )d = -150

⇒ 11 + (n – 1) (-3) = -150

⇒ 11 – 3n + 3 = -150

⇒ 14 + 150 = 3

⇒ \(n=\frac{164}{3}=54 \frac{2}{3}\)

∵ The value of n is not a whole number.

∴ -150 is not a term of a given A.P.

Question 7. Find the 31st term of an A.P. whose 11th term is 38 and the 16th term is 73.
Solution:

Given

In AP 11th term is 38 and the 16th term is 73

Let a be the first term and d the common difference of the A.P.

Now, a₁₁ = 38

⇒ n +(11 – 1)d = 38

⇒ a+ 10 = 38 ….(1)

and a16 = 73

⇒ a+ (16- 1)d = 73

⇒ a+15d=73 …..(2)

Subtracting equation (1) from (2),

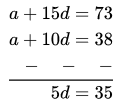
Put the value of d in equation (1),

a+ 10 × 7 = 38

⇒ a=38- 70 =-32

Now, the 31st term of A.P.

a₃₁ = n + (31 – 1)d

=- 32 + 30 × 7

= -32 + 210= 178

The 31st term of an A.P = 178.

Question 8. An A.P. consists of 50 terms of which 3rd term is 12 and the last term is 106. Find the 29th term.
Solution:

Given

An A.P. consists of 50 terms of which 3rd term is 12 and the last term is 106.

Let a be the first term and d the common difference of A.P.

∴ a₃ = 12 ⇒ a + (3 -1)d = 12

⇒ a+2d = 12 ….(1)

and last term= 50th term = 106

⇒ a + (50-1)d = 106

⇒ a + 49 d = 106 …..(2)

Subtracting equation (1) from (2)

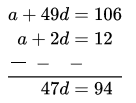
⇒ d = 2

Put the value of d in equation (1),

a + 2 × 2 = 12

a = 12-4 = 8

Now, 29th term = a + (29 – 1)d = 8 + 28 × 2

= 8 + 56 = 64

The 29th term = 64

Question 9. If the 3rd and the 9th terms of an A.P. are 4 and- 8 respectively, which term of this A.P. is zero?
Solution:

Given

The 3rd and the 9th terms of an A.P. are 4 and- 8 respectively,

Let a be the first term and d the common difference of A.P.

a₃ = 4 ⇒ a + (3-1)d = 4

a + 2d = 4…..(1)

a9 = -8 ⇒ a + (9- 1 )d = -8

a + 8d = -8 ……(2)

Subtracting equation (1) from (2),

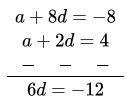
⇒ d = -2

Put the value of d in equation (1),

⇒ a + 2(-2) = 4

⇒ a = 4 + 4 =8

Now, let a_n = 0 a + (n- 1)d =0

⇒ 8 + (n- l)(-2) =0

⇒ 8- 2n + 2 = 0

⇒ -2n =-10

⇒ n =5

∴ 5th term of the progression is zero.

Question 10. The 17th term of an A.P. exceeds its 10th term by 7. Find the common difference.
Solution:

Given

The 17th term of an A.P. exceeds its 10th term by 7.

Let a be the first term and d the common difference of A.P.

∴ a₁₇ =a₁₀ + 7

⇒ a + (17- 1)d = a + (10- 1)d + 7

16d-9d = 7 ⇒ 7d =7

⇒ d=1

Common difference of progression = 1

Question 11. Which term of the A.P. : 3, 15, 27, 39, … will be 132 more than its 54th term?
Solution:

Given A.P. : 3, 15, 27, 39…

a = 3, d=15-3 = 27-15=12

∴ a₅₄ = a+ (54 -1)d = 3 + 53 × 12

= 3 + 636 = 639

Let a_n =a₅₄ + 132

⇒ a + (n – 1)d =639+ 132

⇒ 3 + (n -1)12 =771

⇒ (n- 1)12 =771 -3 = 768

⇒ \((n-1)=\frac{768}{12}=64\)

⇒ n = 64 + 1 = 65

∴ Required term = 65th term.

Question 12. Two A.P.s have the same common difference. The difference between their 100th terms is 1 00, what is the difference between their 1,000th terms?
Solution:

Given

Two A.P.s have the same common difference. The difference between their 100th terms is 1 00

Let the first term be a and the common difference be d of first A.P.

Let the first term be A and the common difference be D of the second A.P.

100th term of first progression = + (100 -1)d

= a + 99d

100th term of the second progression

=A + (100- 1)d

=A + 99d

Difference of 100th terms of two progression

= 100

⇒ (a + 99d) – (A + 99d) = 100

⇒ a + 99d-A-99d = 100

⇒ a-A = 100 ……(1)

Again, the 1000th term of the first progression

= a + (1000-1)d

= a + 999d

1000th term of the second progression

=A + (1000-1)d

=A + 999d

Difference of 1000th terms of two progressions

= (a + 999d) – (A + 999d)

= a + 999d-A-999d

= a – A = 100 [from equation (1)]

Question 13. How many three-digit numbers by 12. are divisible by 7?
Solution:

Three digit numbers: 100, 101 102, …, 999

Three-digit numbers divisible by 7: 105, 112, 119,…, 994

Here, a = 105, d= 1 12 – 105 = 1 19 – 1 12 = 7

Let a_n = 994

⇒ a + (n-1)d =994 ⇒ 105 + (n -1)7 = 994

⇒ (n- 1)7 =994- 105 = 889

⇒ \(n-1=\frac{889}{7}=127\)

⇒ n= 127+ 1 = 128

∴ Number of 3-digit numbers divisible by 7.

= 128

Question 14. How many multiples of 4 lie between 10 and 250?
Solution: The multiples of 4 between 10 and 250 are :

12, 16, 20, …,248

Here a=12,d= 16-12 = 20-16 = 4

a_n =248

⇒ a + (n- 1)d =248

⇒12 + (n- 1)4=248

⇒ (n- 1)4 =248- 12 = 236

⇒ n-1 = 59

⇒ n = 59 + 1 = 60

∴ Multiples of 4 between 10 and 250 = 60

Question 15. For what value of n, are the nth terms of two A.Ps?: 63,65,67,… and 3, 10, 17,… equal?
Solution:

First A.P.: 63, 65, 67…

Here a = 63

d = 65 – 63 = 67- 65 = 2

a_n = a + (n – 1 )d

= 63 + (n – 1)2 = 63 + 2n – 2

= 2n + 61

Second A.P. 3, 10, 17, …

Here A =3,D= 10-3= 17- 10 = 7

A_n =A + (n -1)D = 3 + (n – 1)7

= 3 + 7n-7 = 7n-4

According to the problem, an =An

⇒ 2n + 61 = 7n – 4

⇒ n -7n = -4 – 61

⇒ -5n = -65

⇒ n = 13

∴ The 13th terms of given progressions are equal.

Question 16. Determine the A.P. whose third term is 16 and the 7th term exceeds the 5th term by 12.
Solution:

Let the first term be a and common difference d of the A.P.

According to the problem,

a₇ – a₅ =12

⇒ (a + 6d) – (a + 4d) = 12

⇒ a+6d-a-4d = 12

⇒ 2d = 12

⇒ d = 6

⇒ a₃ = 16

⇒ a + (3-1)d = 16

⇒ a + 2 × 6 = a + (3-1)d = 16

⇒ a + 2 × 6 = 16

⇒ a = 16 – 12 = 4

Now A.P.: 4, 4 + 6, 4 + 2 × 6,…

= 4, 10, 16, …

Question 17. Find the 20th term from the last term of the A.P. : 3, 8, 13, …. 253.
Solution:

Given A.P. : 3, 8, 13,…, 253

Here, last term l = 253, = 8- 3 = 13-8 = 5

20th term from the end =l-(n-1)d

= 253 – (20- 1) × 5

= 253- 19 × 5 = 253-95 = 158

∴ 20th term from the end of the progression

= 158

The 20th term from the last term of the A.P = 158

Question 18. The sum of the 4th and 8th terms of an A.P. is 24 and the sum of the 6th and 10th terms is 44. Find the first three terms of the
Solution :

Given

The sum of the 4th and 8th terms of an A.P. is 24 and the sum of the 6th and 10th terms is 44.

Let the first term be a and common difference d of the A.P.

∴ a₄ + a₈ =24 ⇒ a + 3d + a + 7d = 24

⇒ 2a + 10d =24

⇒ a+2d = 12 …(1)

and a₆ + a₁₀ = 44 ⇒ a + 5d + a + 9d = 44

⇒ 2a+14d =44

⇒ a + 7d =22

Subtracting equation (1) from (2),

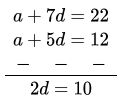
⇒ d = 5

Put the value of d in equation (1),

a + 5 × 5= 12

⇒ a= 12-25 =-13

∴ Second term = a + d = -13 + 5 =-8

Third term-a + 2d =-13+2×5 = -3

So, the first three terms of A.P. are -13, -8,-3.

Question 19. Subba Rao started work in 1995 at an annual salary of ₹5000 and received an increment of ₹200 each year. In which year did his income reach ₹7000?
Solution:

Given

Subba Rao started work in 1995 at an annual salary of ₹5000 and received an increment of ₹200 each year.

Salary in first year = ₹5000 Salary in second year = ₹5000 + ₹200 = ₹5200 Salary in third year = ₹5200 + ₹200 = ₹5400 Progression formed from the salary of each year ₹5000, ₹5200, ₹5400, …

Here, a₂-a₁ = 5200 -5000 = 200

a₃-a₂= 5400 -5200 = 200

a₂ – a₁ = -a₂

⇒ The above progression is an A.P.

a = ₹5000, d = ₹200

Let in the nth year, the salary becomes ₹7000

Let in the nth year, the salary becomes ₹7000.

∴ a_n =7000

⇒ a + (n-1)d =7000

⇒ 5000 + (n- 1)200 =7000

⇒ (n- 1)200 =7000-5000

⇒ (n- 1)200 =2000

⇒ \(n-1=\frac{2000}{200}=10\)

⇒ n= 10+ 1 = 11

∴ In the 11th year, the salary of Subba Rao will be ₹7000.

Question 20. Ramkali saved ₹5 in the first week of the year and then increased her weekly savings by ₹1.75. If in the nth week, her weekly savings become ₹20.75, find n.
Solution:

Given

Ramkali saved ₹5 in the first week of the year and then increased her weekly savings by ₹1.75. If in the nth week, her weekly savings become ₹20.75

Saving of the first week = ₹5

∵ ₹1.75 is increasing in the savings of every week. The saving of every week from an A.P, in which.

a = ₹5 and d = ₹1.75

Let the saving in the nth week = ₹20.75

⇒ a + (n – 1) d = 20.75

⇒ 5 + (w- 1) (1.75) = 20.75

⇒ (n-1) (1.75) =20.75 -5 = 15.75

⇒ \(n-1=\frac{15.75}{1.75}=9\)

⇒ n=9 + 1 = 10

n= 10

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Exercise 5.3

Question 1. Find the sum of the following A.Ps.:

1. 2, 7, 12, …, to 10 terms.
2. -37, -33, -29, …, to 12 terms
3. 0.6, 1.7, 2.8, …, to 100 terms.
4. \(\frac{1}{15}, \frac{1}{12}, \frac{1}{10},\)…, to 11 terms.

Solution:

1. 2, 7, 12…..to 10th term

Here, a = 2,d = 7 -2= 12-7 = 5,n= 10

From the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{10}=\frac{10}{2}[2 \times 2+(10-1) \times 5]\)

= 5(4 + 45) = 5×49 = 245

2. -37, -33, -29,…, to 12 terms

Here a =-37

d = -33- (-37) =-29- (-33) = 4, n = 12

From the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{12}=\frac{12}{2}[2 \times(-37)+(12-1) \times 4]\)

= 6(-74 + 44) = 6 x (-30) = -180

3. 0.6, 1.7, 2.8,…, to 100 terms

Here a = 0.6, d = 1.7 – 0.6 = 2.8 – 1.7 = 1.1 n= 100

From the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{100}=\frac{100}{2}[2 \times 0.6+(100-1) \times 1.1]\)

= 50[1.2 + 108.9] = 50×110.1

= 5505

4. \(\frac{1}{15}, \frac{1}{12}, \frac{1}{10}, \ldots, \text { to } 11 \text { terms }\)

Here, \(a=\frac{1}{15}, d=\frac{1}{12}-\frac{1}{15}=\frac{1}{10}-\frac{1}{12}=\frac{1}{60}, n=11\)

From the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \( S_n=\frac{11}{2}\left[2 \times \frac{1}{15}+(11-1) \times \frac{1}{60}\right]\)

⇒ \(=\frac{11}{2}\left[\frac{8+10}{60}\right]=\frac{11}{2} \times \frac{18}{60}=\frac{33}{20}\)

Question 2. Find the sums given below :

1. 7 +\(10 \frac{1}{2}\) + 14+ … + 84
2. 34 + 32 + 30 + … + 10
3. -5 + (-8) + (-11) + …(-230)

Solution:

1. 7 +\(10 \frac{1}{2}\) + 14+ … + 84

Here a = 7, d = \(10 \frac{1}{2}\) = \(14-10 \frac{1}{2}\)

⇒ \(3 \frac{1}{2}=\frac{7}{2}\)

Let a_n = 84

⇒ a + (n-1)d = 84 => \(7+(n-1) \frac{7}{2}=84\)

⇒ \( (n-1) \frac{7}{2}=84-7=77\)

⇒ \(n-1=77 \times \frac{2}{7}=22\)

⇒ \(n=22+1=23\)

From the formula, \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \( S_{23}=\frac{23}{2}\left[2 \times 7+(23-1) \times \frac{7}{2}\right]\)

⇒ \(\frac{23}{2}[14+77]=\frac{23 \times 91}{2}\)

⇒ \(\frac{2093}{2}\)

⇒ \(1046 \frac{1}{2}\)

2. 34 + 32 + 30 + … + 10

Here a = 34, = 32- 34 = 30 – 32 = -2

Let an = 10 ⇒ a + (n – 1)d = 10

⇒ 34 + (n-1)(-2) =10

⇒ (n- 1) (-2) = 10-34

⇒ (n-1) (-2) =-24

⇒ n-1 =12

⇒ n=12+1 = 13

From the formula, \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{13}=\frac{13}{2}[2 \times 34+(13-1)(-2)]\)

⇒ \(\frac{13}{2}[68-24]\)

⇒ \(\frac{13}{2} \times 44=286 \quad\)

3. -5 + (_8) + (-11)… + (-230)

Here a =-5, d =-8- (-5) =-11 – (-8) =-3

Let an = -230 ⇒ a + (n-1)d = -230

⇒ -5 + (n – 1) (-3) = -230

⇒ (n- 1) (-3) =-230 + 5

⇒ (n – 1)(-3) = -225

⇒ n- 1 = 75

⇒ n = 75 + 1 = 76

From the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{76}=\frac{76}{2}[2 \times(-5)+(76-1)(-3)]\)

= 38(-10 – 225)

= 38 × (-235) = -8930

Question 3. In an A.P. :

1. Given a = 5, d = 3, a_n = 50, find ii and S_n.
2. Given a = 7, a₁₃ = 35, find d and S₁₃.
3. Given a₁₂ = 37, d = 3, find a and S₁₂.
4. Given a₃ = 15, S₁₀ = 125, findd and a₁₀.
5. Given d = 5, S₉ = 75, find a and a₉.
6. Given a = 2, d = 8, S_n = 90, find n and an.
7. Given a = 8, an = 62, S_n = 210, find n and d.
8. Given a_n = 4, n = 2, S_n = -14, find n and a.
9. Given a = 3, n = 8, S = 192, find d.
10. Given l = 28, S = 144, and there are a total 9 terms. Find a

Solution:

1. a = 5,d = 3, a_n = 50

⇒ a + (n -1)d = 50

⇒ 5 + (n-1)3 =50

⇒  (n-1)3 = 45

⇒ a-1= 15

⇒ n = 16

Now, \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(\frac{16}{2}[2 \times 5+(16-1) \times 3]\)

⇒ \(8(10+45)=8 \times 55=440\)

n = 16 and Sn = 440

2. a = 7

a₁₃= 35

⇒ a + (13 – 1)d = 35

⇒ 7 + 12d = 35

⇒ 12d =35-7 = 28

⇒ \(d=\frac{28}{12}=\frac{7}{3}\)

and, from \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{13}=\frac{13}{2}\left[2 \times 7+(13-1) \times \frac{7}{3}\right]\)

⇒ \(\frac{13}{2}[14+28]=\frac{13}{2} \times 42=273\)

∴ \(d=\frac{7}{3} \text { and } S_{13}=273 \quad\)

3. d = 3

a₁₂ = 37

a+(12-1)d = 37

a + 11 × 3 = 37

a = 37 – 33 = 4

and, from \( S_n=\frac{n}{2}[2 n+(n-1) d]\)

⇒ \(S_{12}=\frac{12}{2}[2 \times 4+(12-1) \times 3]\)

⇒ \(6[8+33]=6 \times 41=246\)

∴ \(a=4, S_{12}=246\)

4. Let the first term be a and the common difference is d.

a₃ = 15 ⇒ a + (3 -1)d= 15

⇒ a+2d = 15 …(1)

and S₁₀= 125

⇒ \(\frac{10}{2}[2 a+(10-1) d]=125\)

⇒ 5[2a + 9d] = 125

⇒ 2a + 9d = 25 …(2)

Multiply equation (1) by 2 and subtracting from equation (2),

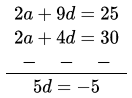
d = -1

Put the value of d in equation (1)

⇒ a + 2 × – 1 = 15

⇒ a-2= 15

⇒ a = 15+2= 17

a₁₀ = a+ (10-1)d

= 17 + 9(-1) = 17-9 = 8

∴ d = -1, a₁₀ = 8

5. d = 5

and S₉ = 75

⇒ \(\frac{9}{2}[2 a+(9-1) \times 5]=75\)

⇒ \( 2 a+40=\frac{75 \times 2}{9}\)

⇒ \(a +20=\frac{75}{9} \Rightarrow a+20=\frac{25}{3}\)

⇒ \(a=\frac{25}{3}-20=\frac{25-60}{3}\)

⇒ \(a=\frac{-35}{3}\)

and \(a_9=a+8 d=\frac{-35}{3}+8 \times 5=\frac{-35}{3}+40\)

⇒ \(\frac{-35+120}{3}=\frac{85}{3}\)

∴ \(a=\frac{-35}{3} \text { and } a_9=\frac{85}{3}\)

6. a = 2, d= 8

S_n = 90

⇒ \( \frac{n}{2}[2 a+(n-1) \cdot d]=90\)

⇒ \(\frac{n}{2}[2 \times 2+(n-1) \cdot 8]=90\)

⇒ \(\frac{4n}{2}[1+(n-1) \cdot 2]=90\)

⇒ \( n(2 n-1)=\frac{90 \times 2}{4}\)

⇒ \(2 n^2-n=45\)

⇒ \(2 n^2-n-45=0\)

⇒ \(2 n^2-10 n+9 n-45=0\)

⇒ \(2 n(n-5)+9(n-5)=0\)

⇒ \((n-5)(2 n+9)=0\)

⇒ \(n-5=0 \quad \text { or } 2 n+9=0\)

⇒ \(n =5 \quad \text { or } \quad n=-\frac{9}{2}\)

⇒ \(n =-\frac{9}{2} \text { is not possible.}\)

∴ n = 5

Now, an = a + (n-1)d

a₅ = 2 + (5 – 1) × 8 = 2 + 32 = 34

n = 5 and a_n = 34

7. a = 8, a_n = 62

S_n= 210

⇒ \(\frac{n}{2}\left(a+a_n\right)=210\)

⇒ \(\frac{n}{2}(8+62)=210\)

⇒ \(n=\frac{210 \times 2}{70}=6\)

⇒ \(a_n=62\)

⇒ \(a+(n-1) d=62\)

⇒ 8 + (6-1)d= 62 => 5d = 62 – 8 = 54

⇒ \(d=\frac{54}{5}\)

∴ \(n=6, d=\frac{54}{5}\)

8. a_n = 4, d = 2 and S_n=-14

a_n = 4

⇒ a + (n -1)d = 4

⇒ a + (n – 1).2 = 4

⇒ a + 2n – 2 =4

⇒ a + 2n = 6

and S_n = -14 …(1)

⇒ \( \frac{n}{2}\left(a+a_n\right)=-14\)

⇒ \(\frac{n}{2}(a+4)=-14\)

⇒ \(\frac{n}{2}(6-2 n+4)=-14 \quad \text { from eqn. (1) }\)

⇒ \(\frac{n}{2}(10-2 n)=-14\)

⇒ \(n(5-n)=-14\)

⇒ \(5 n-n^2=-14\)

⇒ \(0=n^2-5 n-14\)

⇒ \(n^2-7 n+2 n-14=0\)

⇒ \( n(n-7)+2(n-7)=0\)

⇒ \((n-7)(n+2)=0\)

⇒ \(n-7=0 \text { or } n+2=0\)

⇒ \(n=7 \text { or } \quad n=-2\)

⇒ \(n=-2 \text { is not possible.}\)

∴ n=7

From equation (1)

a + 2 × 7 = 6

a = 5

a = 6 – 14= -8

∴ n = 7 and a = -8

9. \(a=3, n=8 \text { and } S=192\)

⇒ \(S=192\)

⇒ \(\frac{n}{2}[2 a+(n-1) d]=192\)

⇒ \(\frac{8}{2}[2 \times 3+(8-1) d]=192\)

⇒ \(4(6+7 d)=192\)

⇒ \(24+28 d=192\)

⇒ \(28 d=192-24=168\)

⇒ \(d=\frac{168}{28}=6\)

∴ d=6

10. l=28, S =144, n=9

S=144

⇒ \(\frac{n}{2}(a+l)=144 \Rightarrow \frac{9}{2}(a+28)=144\)

⇒ \(a+28=\frac{144 \times 2}{9}=32\)

⇒ a=32-28=4

∴ a=4

Question 4. How many terms of the A.P.: 9, 17, 25,… must be taken to give a sum of 636?
Solution:

Given A.P: 9. 17. 25….

Here a = 9,d= 17-9 = 25-17 = 8

Let S_n=636

⇒ \(\frac{n}{2}[2 a+(n-1)] d=636\)

⇒ \(\frac{n}{2}[2 \times 9+(n-1) \cdot 8]=636\).

⇒ \(n[9+(n-1) \cdot 4]=636\)

⇒ \(n(9+4 n-4)=636\)

⇒ \(n(4 n+5)=636\)

⇒ \(4 n^2+5 n-636=0\)

⇒ \(4 n^2+53 n-48 n-636=0\)

⇒ \( n(4 n+53)-12(4 n+53)=0\)

⇒ \((4 n+53)(n-12)=0\)

⇒ \(4 n+53=0 \text { or } n-12=0\)

⇒ \(n=-\frac{53}{4} \text { or } n=12\)

but \(n=-\frac{53}{4}\) is not possible.

∴ n = 12

Therefore, the number of terms = 12

Question 5. The first term of an A.P. is 5, the last term is 45 and the sum is 400. Find the number of terms and the common difference.
Solution:

Here a = 5

Let the number of terms = n

⇒ \(a_n=45 \text { and } S_n=400\)

⇒ \(S_n=400 \Rightarrow \frac{n}{2}\left(a+a_n\right)=400\)

⇒ \(\frac{n}{2}(5+45)=400\)

⇒ \(n=\frac{400 \times 2}{50}=16\)

⇒ \(a_n=45\)

⇒ \(a+(n-1) d=45\)

⇒ \(5+(16-1) d=45\)

⇒ \(15 d=45-5=40\)

⇒ \(d=\frac{40}{15}=\frac{8}{3}\)

∴ \(n=16 \text { and } d=\frac{8}{3}\)

The number of terms and the common difference 16 and\(\frac{8}{3}\)

Question 6. The first and the last terms of an A.P. are 17 and 350 respectively. If the common difference is 9, how many terms are there and what is their sum?
Solution:

Given

The first and the last terms of an A.P. are 17 and 350 respectively. If the common difference is 9

Let the number of terms = n

a= 17, d = 9

an = 350 ⇒ a + {n- l)d = 350

⇒ \( 17+(n-1) \cdot 9=350\)

⇒ \((n-1)9=350-17=333\)

⇒ \(n-1=\frac{333}{9}=37\)

⇒ \(n=37+1=38\)

Now, from \( S_n=\frac{n}{2}\left(a+a_n\right)\)

⇒ \(S_{38}=\frac{38}{2}(17+350)\)

⇒ \(19 \times 367=6973\)

∴ n=38 and S_n=6973

Question 7. Find the sum of the first 22 terms of an A.P. in which d = 7 and the 22nd term is 149.
Solution:

d =7

a₂₂=149

⇒ \(a+(22-1) d=149\)

⇒ \(a+21 \times 7=149\)

⇒ \(a+147=149\)

⇒ \(a=149-147=2\)

⇒ \(S_n=\frac{n}{2}\left(a+a_n\right)\)

⇒ \(S_{22}=\frac{22}{2}\left(a+a_{22}\right)=11(2+149)\)

= 11 × 151 = 1661

The sum of the first 22 terms of an A.P. = 1661

Question 8. Find the sum of the first 51 terms of an A.P. whose second and third terms are 14 and 18 respectively.
Solution :

Given

Second and third terms of an A.P are 14 and 18 respectively

Let the first form of A.P. be a and the common difference be d.

Now, \(a_2=14 \quad \Rightarrow \quad a+d=14 \quad \ldots(1)\)

and \(a_3=18 \quad \Rightarrow a+2 d=18 \quad \ldots(2)\).

Subtracting equation (1) from (2)

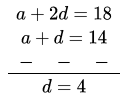
Put the value of d in equation (l),

a+4 = 14

a = 14-4 = 10

Now, from the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{51}=\frac{51}{2}[2 \times 10+(51-1) \times 4]\)

⇒ \(\frac{51}{2}(20+200)\)

⇒ \(\frac{51}{2} \times 220=5610\)

∴ The sum of 51 terms = 5610

Question 9. If the sum of first 7 terms of an A.P is 49 and that of 1 7 terms is 289, find the sum of first n terms.
Solution:

Given

The sum of first 7 terms of an A.P is 49 and that of 1 7 terms is 289

Let the first term of A.P. be a and common difference be d.

S₇ = 49

⇒ \( \frac{7}{2}[2 a+(7-1) d]=49 \Rightarrow \frac{1}{2}[2 a+6 d]=7\)

⇒ \(a+3 d=7\)

⇒ \(S_{17}=289\)

⇒ \(\frac{17}{2}[2 a+(17-1) d]=289\)

⇒ \(\frac{1}{2}[2 a+16 d]=17 \Rightarrow a+8 d=17 \ldots(2)\)

Subtracting equation (1) from (2),

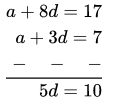
d = 2

Put the value of d in equation (1),

a + 3×2=7

⇒ a + 6=7

⇒ a =7 -6=1

Now, \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(\frac{n}{2}[2 \times 1+(n-1) \cdot 2]\)

⇒\(n(1+n-1)=n^2\)

∴ The sum of n terms of A.P. = n²

Question 10. Show that a₁, a₂, an A.P. where a„ is defined as below :

1. a_n = 3 + 4n
2. a_n = 9 – 5n

Also, find the sum of the first 15 terms in each case.

Solution:

the nth term of the sequence,

a_n=3+4n

Put n = 1\(a_1=3+4 \times 1=3+4=7\)

Put n = 2\(a_2=3+4 \times 2=3+8=11\)

Put n = 3\(a_3=3+4 \times 3=3+12=15\)

Now, \(a_2-a_1=11-7=4\)

⇒ \(a_3-a_2=15-11=4\)

⇒ \( a_2-a_1=a_3-a_2=4\)

∴ The difference between two consecutive terms of the sequence is constant.

So, the sequence is A.P.

Now, d = 4 and a = 7

A sum of first 15 terms

⇒ \(frac{n}{2}[2 a+(n-1) d]\)

⇒ \(\frac{15}{2}[2 \times 7+(15-1) \times 4]\)

⇒ \(\frac{15}{2}[14+56]=\frac{15}{2} \times 70=525\)

2. \(a_n=9-5 n\)

Put n = 1, \(a_1=9-5 \times 1=9-5=4\)

Put n = 2, \(a_2=9-5 \times 2=9-10=-1\)

Put n = 3, \(a_3=9-5 \times 3=9-15=-6\)

Now, \(a_2-a_1=-1-4=-5\)

⇒ \(a_3-a_2=6-(-1)=-6+1=-5\)

⇒ \(a_2-a_1=a_3-a_2=-5\)

∴ The difference between two consecutive terms of the sequence is constant.

So, the sequence is A.P.

Now d =-5,a = 4

∴ The sum of the first 15 terms

⇒ \(\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(\frac{15}{2}[2 \times 4+(15-1)(-5)]\)

⇒ \(\frac{15}{2}(8-70)=\frac{15}{2} \times(-62)=-465\)

Question 11. If the sum of the first n terms of an A.P. is 4n – n², what is the first term (that is S₁)? What is the sum of the first two terms? What is the second term? Similarly, find the 3rd, the 10th and the nth terms.
Solution:

Here S_n = 4n – n²

Put n = 1

⇒ \(S_1=4 \times 1-(1)^2=4-1=3\)

Put n = 2

⇒ \(S_2=4 \times 2-2^2=8-4=4\)

Second term \(a_2=S_2-S_1=4-3=1\)

Put n = 3

⇒ \(S_3=4 \times 3-3^2=12-9=3\)

⇒ \(a_3=S_3-S_2=3-4=-1\)

Put n = 9

⇒ \(S_9=4 \times 9-9^2=36-81=-45\)

Put n = 10

⇒ \( S_{10}=4 \times 10-10^2=40-100=-60\)

⇒ \(a_{10}=S_{10}-S_9=(-60)-(-45)\)

= -60+45 = -15

Replace n by (n – 1)

⇒ \(S_{n-1}=4(n-1)-(n-1)^2\)

⇒ \(4 n-4-\left(n^2-2 n+1\right)\)

⇒ \(4 n-4-n^2+2 n-1=6 n-n^2-5\)

⇒ \(a_n=S_n-S_{n-1}\)

⇒ \(\left(4 n-n^2\right)-\left(6 n-n^2-5\right)\)

⇒ \(4 n-n^2-6 n+n^2+5\)

⇒ 5-2 n

Question 12. Find the sum of the first 40 positive integers divisible by 6.
Solution:

The progression formed the positive integers divisible by 6

6, 12, 1 8, 24, … to 40 terms

Here a = 6, = 6, n = 40

From the formula, \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{40}=\frac{40}{2}[2 \times 6+(40-1) \times 6]\)

= 20(12 + 234) = 20 x 246 = 4920

The sum of 40 terms = 4920

Question 13. Find the sum of the first 15 multiples of 8.
Solution:

The first 15 multiples of 8 are 8, 16, 24, …, to 15 terms

Here, a = 8, d = 16 — 8 = 24 — 16 = 8, n= 15

From the formula \( S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{15}=\frac{15}{2}[2 \times 8+(15-1) \times 8]\)

⇒ \(\frac{15}{2} \times 8[2+14]\)

= 60×16 = 960

The sum of the first 15 multiples of 8 = 960

Question 14. Find the sum of the odd numbers between 0 and 50.
Solution:

Odd numbers between 0 and 50 are 1,3,5…..49.

Here, a= 1,d =3- 1 =5-3=2

Let, an = 49

⇒ 1+(n-1).2 = 49

⇒ 1 + 2n – 2 = 49

⇒ 2n – 2 = 49

⇒ 2n = 49 + 1 = 50

⇒ n = 25

Now, from the formula \(S_n=\frac{n}{2}\left(a+a_n\right)\)

⇒ \(S_{25}=\frac{25}{2}(1+49)\)

⇒ \(\frac{25}{2} \times 50=625\)

The sum of the odd numbers between 0 and 50 is 625.

Question 15. A contract on a construction job specifies a penalty for delay of completion beyond a certain date as follows: ₹200 for the first day, ₹250 for the second day, ₹300 for the third day, etc., the penalty for each succeeding day being? 50 more than for the preceding day. How much money does the contractor have to pay as a penalty if he has delayed the work by 30 days?
Solution:

Penalty for delay of first day = ₹200

Penalty for delay of second day = ₹250

Penalty for delay of third day = ₹300

This progression is an A.P.

Here a = 200, d = 250 – 200 = 300 – 250 = 50, n = 30

From the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \( S_{30}=\frac{30}{2}[2 \times 200+(30-1) \times 50]\)

=15(400+1450)

=15×1850=27750

The contractor will pay the penalty of ₹27750.

Question 16. A sum of ₹700 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is ₹20 less than its preceding prize, find the value of each of the prizes.
Solution:

Let first prize = ₹a

Common difference d =- 20, n = 7

Given, S₇ = 700

⇒ \(\frac{7}{2}[2 a+(7-1)(-20)]=700\)

⇒ \({[2 a-120]=200}\)

⇒ 2a =200+ 120

⇒ 2a =320

⇒ a = 160

∴ a₂ =a + d= 160-20= 140

⇒ \(a_3=a_2+d=140-20=120\)

⇒ \(a_4=a_3+d=120-20=100\)

⇒ \(a_5=a_4+d=100-20=80\)

⇒ \(a_6=a_5+d=80-20=60\)

⇒ \(a_7=a_6+d=60-20=40\)

Prizes are ₹160, ₹140, ₹120, ₹100, ₹80, ₹ 60 and ₹40.

Question 17. In a school, students thought of planting trees in and around the school to reduce air pollution. It was decided that the number of trees, that each section of each class will plant, would be the same as the class, in which they are studying, There are three sections of each class. How many trees will be planted by the students?
Solution:

The sequence so formed : 3, 6, 9, … is an A.P.

Here, a = 3,d = 6- 3 = 9- 6 = 3

and n= 12

Now, from the formula \(S_n=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(S_{12}=\frac{12}{2}[2 \times 3+(12-1) \times 3]\)

= 6(6 + 33) = 6 x 39 = 234

Total trees planted = 234

Question 18. A spiral is made up of successive semicircles, with centres alternately at A and B, starting with the centre at A, of radii 0.5 cm, 1.0 cm, 1.5 cm, 2.0 cm,…. What is the total length of such a spiral made up of thirteen consecutive semicircles? ( Take \(\pi=\frac{22}{7}\))

Solution:

The radius of first semicircle r₁ = 0.5 cm.

The radius of second semicircle r₂ =1.0 cm

The radius of third semicircle r₃ = 1.5 cm

.
.
.
.
.
.
.

This sequence is an A.P.

Here a = 0.5 and d = 1.0 – 0.5 = 0.5, n = 13

Now, the length of the spiral is made of 1 3 consecutive semicircles.

⇒ \(\pi r_1+\pi r_2+\pi r_3+\ldots \text { to } 13 \text { terms }\)

⇒ \(\pi\left[r_1+r_2+r_3+\ldots 13\right.\)

⇒ \(\frac{22}{7} \times \frac{13}{2}[2 a+(13-1) \cdot d]\)

⇒ \(\frac{143}{7}[2 \times 0.5+12 \times 0.5]\)

⇒ \(\frac{143}{7} \times 7=143 \mathrm{~cm}\)

The total length of such a spiral made up of thirteen consecutive semicircle 143cm.

Question 19. 200 logs are stacked in the following manner: 20 logs in the bottom row, 1 9 in the next row, 1 8 in the row next to it and so on (see figure). In how many rows are the 200 logs placed and how many logs are in the top row?

Solution:

No. of logs in lowest row = 20.

Starting from the bottom.

Logs in first row = 20

Logs in the second row =19

Logs in the third row = 18

This sequence is an A.P. in which

a = 20, d = 19-20 =-l

Let no. of rows = n

∴ S_n=200

⇒ \(\frac{n}{2}[2 a+(n-1) \cdot d]=200\)

⇒ \(\frac{n}{2}[2 \times 20+(n-1) \cdot(-1)]=200\)

⇒ \(n(40-n+1)=400\)

⇒ n(41-n)=400

⇒ \(41 n-n^2=400\)

⇒ \(0=n^2-41 n+400\)

⇒ \(n^2-25 n-16 n+400=0\)

⇒ \(n(n-25)-16(n-25)=0\)

⇒ \((n-25)(n-16)=0\)

⇒ \(n-25=0 \text { or } n-16=0\)

⇒ \(n=25 \text { or } n=16\)

∴ n = 25th

a₂₅ =n + (25-1)d = 20 + 24(-l)

= -4 which is not possible.

∴ n= 16

n₁₆ =n + (16-1)d = 20 + 1 5(-1)

= 20-15 = 5

So total rows = 1 6

and no. of logs in upper row = 5

Question 20. In a potato race, a bucket is placed at the starting point, which is 5 m from the first potato, and the other potatoes are placed 3 m apart in a straight line. There are ten potatoes in the line.

A competitor starts from the bucket, picks up the nearest potato, runs back with it, drops it in the bucket, runs back to pick up the next potato, runs to the bucket drops it In, and continues in the same way until all the potatoes arc in the bucket. What is the total distance the competitor has to run?
Solution:

Distance of the first potato from the first bucket = 5 m

Distance of the second potato from a bucket

= 5 + 3 = 8 m

Distance of the third potato from the bucket

= 8 + 3 = 11 cm

Once start from a bucket, pick up a potato and run back with it, drop it in the bucket.

Distance covered to drop the potatoes in the bucket.

= 2×5m,2×8m,2×11m, …

= 10 m, 16 m, 22 m,

Here a = 10, d = 16 – 10 = 22 – 16 = 6, n = 10

Distance covered to drop n potatoes in a bucket

⇒ \(\frac{n}{2}[2 a+(n-1) d]\)

Distance covered to drop 10 potatoes in a bucket

⇒ \(\frac{10}{2}[2 a+(10-1) d]\)

⇒ \(5[2 \times 10+9 \times 6]=5(20+54)\)

⇒ \(5 \times 74=370 \mathrm{~m}\)

The total distance the competitor has to run 370 meters,

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Exercise 5.4 (Optional)

Question 1. Which term of the A.P.: 121, 117, 113…..is its first negative term?

[Hint: Find n for an < 0]

Solution:

Given, A.P.: 121, 117, 113, …

Here a= 121,d= 117- 121 = 113- 117 = -4

Let \(a_n<0 \quad \Rightarrow a+(n-1) d<0\)

⇒ \(121+(n-1)(-4)<0\)

⇒ \(121-4 n+4<0\)

⇒ \(-4 n<-125\)

⇒ \(n>\frac{125}{4} \quad \Rightarrow \quad n>31 \frac{1}{4}\)

n = 32, 33, 34…

∴ The first negative term = 32nd term.

Question 2. The sum of the third and the seventh terms of an A.P. is 6 and their product is 8. Find the sum of the first sixteen terms of the A.P.
Solution:

Let the first term of A.P. be a and the common difference be d.

∴ Third term a3 = a + 2d

and Seventh term a7 = a + 6d

According to the problem,

⇒ \( a_3+a_7=6\)

⇒ \(a_3 a_7=8\)

⇒ \(a_3\left(6-a_3\right)=8 \text { [from equation }(1)]\)

⇒ \(6 a_3-a_3^2=8\)

⇒ \(0=a_3^2-6 a_3+8\)

⇒ \(a_3^2-4 a_3-2 a_3+8=0\)

⇒ \(a_3\left(a_3-4\right)-2\left(a_3-4\right)=0\)

⇒ \(\left(a_3-4\right)\left(a_3-2\right) =0\)

⇒ \(a_3-4=0 \text { or } \quad a_3-2=0\)

⇒ \(a_3=4 \text { or } \quad a_3=2\)

If a₃ = 4 then from equation (1)

Now, \( a_7=6-4=2\)

a+2 d=4 ….(2)

a+6 d=2 ….(3)

On subtracting

-4d = 2

⇒ \(d=-\frac{1}{2}\)

Put the value of d in equation (2),

⇒ \(a+2\left(-\frac{1}{2}\right)=4\)

a-1=4 ⇒ a = 4+1=5

⇒ \(S_{16}=\frac{16}{2}[2 a+(16-1) d]\)

⇒ \(8\left[2 \times 5+15\left(-\frac{1}{2}\right)\right]\)

⇒ \(8\left(10-\frac{15}{2}\right)\)

⇒ \(8 \times \frac{5}{2}=20\)

If a₃ = 2 then from equation (1), a₇ = 4

Now, a + 2d = 2 …..(4)

a + 6d = 4 …..(5)

On subtracting

-4d =-2

d = \(\frac{1}{2}\)

Put the value of d in equation (4)

⇒ \(a+2 \times \frac{1}{2}=2 \Rightarrow a=2-1=1\)

⇒ \(S_{16}=\frac{16}{2}[2 a+(16-1) d]\)

⇒ \(8\left[2 \times 1+15 \times \frac{1}{2}\right]\)

⇒ \(8\left(2+\frac{15}{2}\right)\)

⇒ \(8 \times \frac{19}{2}=76\)

The sum of the first sixteen terms of the A.P = 76.

Question 3. A ladder has rungs 25 cm apart. (see figure). The rungs decrease uniformly in length from 45cm at the bottom to 25cm at the top. If the top and the bottom rungs are 2\(\frac{1}{2}\) m apart, what is the length of the wood required for the rungs?

[Hint : Number of rungs = \(\frac{250}{25}+1\)]

Solution:

Horizontal distance between first and last rung = 2\(\frac{1}{2}\) m = 250cm

and distance between two consecutive rungs

Number of rungs in ladder \(=\frac{250}{25}+1=10+1=11\)

Now, the length of the first rung a = 25 cm

Length of last rung l = 45 cm

Length of wood used in 11 rungs

⇒ \(\frac{11}{2}(a+l)=\frac{11}{2}(25+45)\)

⇒ \(11 \times 35=385 \mathrm{~cm}\)

The length of the wood required for the rungs 385cm.

Questionfrom4. The1 tohouses49. Showofa row that is numbered there is a value of x such that the sum of the numbers of the houses preceding the house numbered x is equal to the sum of the numbers of the houses following it. Find this value of x.

Hint: \(S_{x-1}=S_{49}-S_x\)

Solution:

Numbers mark on houses: 1, 2, 3, …47, 48, 49

x is a number such that Sum of the numbers before x = sum of the numbers after x

1 +2 + 3 + …+(x-1)

= (x+ 1) + (x + 2) + … + 49

⇒ \(S_{x-1}=S_{49}-S_x\)

⇒ \( \frac{x-1}{2}[1+x-1]=\frac{49}{2}[1+49]-\frac{x}{2}(1+x)\)

⇒ \(\frac{x^2-x}{2}=1225-\frac{x^2+x}{2}\)

⇒ \(\frac{x^2-x}{2}+\frac{x^2+x}{2}=1225\)

⇒ \(\frac{x^2-x+x^2+x}{2}=1225\)

⇒ \(x^2=(35)^2\)

x=35

The value of x =35.

Question 5. A small terrace at a football ground comprises of 1 5 steps each of which is 50 m long and built of solid concrete.

Each step has a rise of \(\frac{1}{4}\)m and a tread of \(\frac{1}{2}\)m (see figure). Calculate the total volume of concrete required to build the terrace.

[Hint: Volume of concrete required to build first step = \(\frac{1}{4} \times \frac{1}{2} \times 50 \mathrm{~m}^3\)]

Solution:

Given, the length of each step=50m and breadth is \(\frac{1}{2}\)m

The number of steps are 15 and the height of each step from the ground from an A.P. is as follows:

⇒ \(\frac{1}{4}, \frac{2}{4}, \frac{3}{4}, \frac{4}{4}, \frac{5}{4}, \frac{6}{4}, \frac{15}{4}\)

So, the volume of concrete used in the first step

⇒ \(50 \times \frac{1}{2} \times \frac{1}{4}=\frac{50}{8} \mathrm{~m}^3\)

The volume of concrete used in the second step

⇒ \(50 \times \frac{1}{2} \times \frac{2}{4}=\frac{100}{8} \mathrm{~m}^3\)

The volume of concrete used in the third step

⇒ \(50 \times \frac{1}{2} \times \frac{3}{4}=\frac{150}{8} \mathrm{~m}^3\)

The volume of concrete used in the fourth step

⇒ \(50 \times \frac{1}{2} \times \frac{4}{4}=\frac{200}{8} \mathrm{~m}^3\)

So, the volume of total concrete

⇒ \(\frac{50}{8}+\frac{100}{8}+\frac{150}{8}+\frac{200}{8}+\ldots+\text { to } 15 \text { term }\)

⇒ \(a=\frac{50}{8}\)

⇒ \(d=\frac{100}{8}-\frac{50}{8}=\frac{50}{8} \text { and } n=15\)

Therefore, the total volume of concrete

⇒ \(V=\frac{n}{2}[2 a+(n-1) d]\)

⇒ \(\frac{15}{2}\left[2 \times \frac{50}{8}+(15-1) \frac{50}{8}\right]\)

⇒ \(\frac{15}{2} \times \frac{50}{8}[2+15-1]\)

⇒ \(\frac{15}{2} \times \frac{50}{8} \times 16=15 \times 50=750 \mathrm{~m}^3\)

Therefore, the volume of concrete used in the terrace = 750 m3

NCERT Exemplar Solutions for Class 10 Maths Chapter 5 Arithmetic Progression Multiple Choice Questions

Question 1. The sum of the first 6 multiples of 3 is :

1. 55
2. 60
3. 63
4. 65

Answer: 3. 63

Question 2. The sum of 10 terms of the progression 5, 11, 17, … is :

1. 300
2. 320
3. 280
4. 240

Answer: 2. 320

Question 3. 8 times the 8th term of an A.P. is equal to 12 times the 12th term. Its 20th term is

1. 20
2. 0
3. -20
4. None of these

Answer: 2. 0

Question 4. The first two terms of an A.P. are 2 and 7. Its 18th term is :

1. 87
2. 92
3. 82
4. None of these

Answer: 1. 87

Question 5. How many terms are there in A.P. 42, 63, 84 … 210?

1. 7
2. 8
3. 10
4. 9

Answer: 4. 9

Question 6. In an A.P., d =-4,n = 7,an = 7, then the value of a is:

1. 6
2. 7
3. 28
4. 30

Answer: 3. 28

Question 7. The common difference between the two arithmetic progressions are same. If their first terms are 2 and 10 respectively, then the difference between their 5th terms is :

1. 8
2. 2
3. 10
4. 6

Answer: 1. 8