Important Questions For CBSE Class 12 Maths Chapter 6 Applications Of Derivatives

CBSE Class 12 Maths Chapter 6 Applications Of Derivatives Important Questions

Question 1. A function f: R → R is defined as f(x) = x³ + 1. Then the function has

1. No minimum value
2. No maximum value
3. Both maximum and minimum values
4. Neither maximum value nor minimum value

Solution: 4. Neither maximum value nor minimum value

Read and Learn More CBSE Class 12 Maths Important Question and Answers

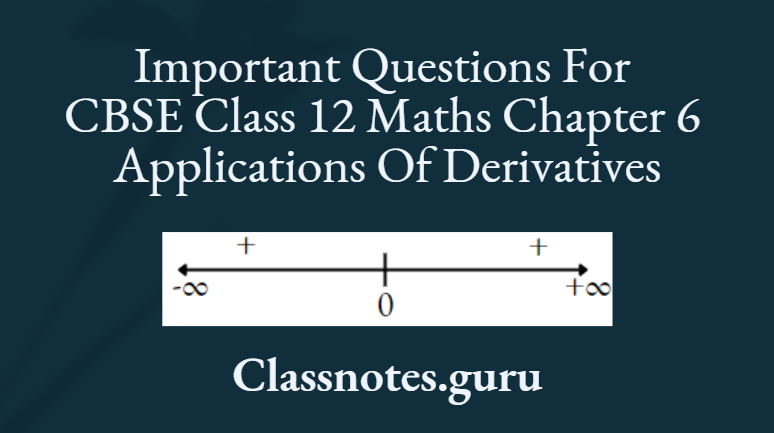
Given ; f(x) = x³ + 1, f: R →  R

f(x) = 3x² = 0

⇒ x = 0

In (0, ∞); f (x) > 0 and In (-∞, 0); f'(x) > 0

Hence; the function has neither maximum nor minimum value.

Question 2. The function y = x²e^-x is decreasing in the interval :

1. (0,2)
2. (2,  ∞)
3. (-∞, 0)
4. (-∞, 0) ∪ (2,  ∞)

Solution: 4. (-∞, 0) ∪ (2,  ∞)

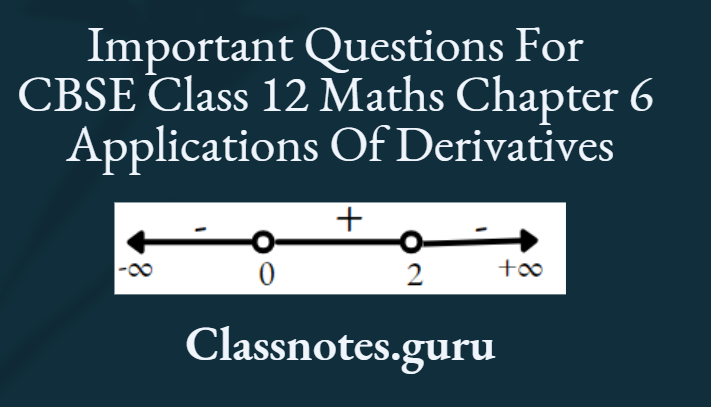
Given, y = x² e^-x

⇒ y’ = 2x² e^-x+ x² (-e^-x) = e^-x {2x – x²} = e^-x. x(2 – x) < 0

(-∞, 0) ∪ (2, ∞)

Question 3.The function f(x) = 2x³ – 15x² + 36x + 6 is increasing in the interval :

1. (-∞, 2)  ∪ (3, ∞)
2. (-∞, 2)
3. (-∞, 2] ∪ [3, ∞)
4. [3, ∞)

Solution: 3. (-∞, 2] ∪ [3, ∞)

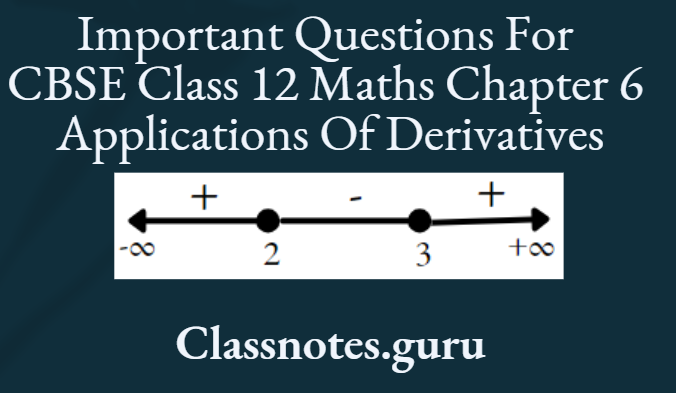
⇒ f(x) = 2X³ – 15x² + 36x +6

⇒ f(x) = 6x² – 30x + 36 = 6(x – 2) (x – 3) > 0

Hence; f(x) is increasing in (-∞, 2] ∪ [3, ∞) [f'(x)≥0]

Question 4. The maxiumu value of \({\frac{1}{x}^x}\) is:

1. \(e^{1 / e}\)
2. \(\mathrm{e}\)
3. \(\left(\frac{1}{e}\right)^{1 / e}\)
4. \(e^e\)

Solution:

Let \(y=\left(\frac{1}{x}\right)^x \Rightarrow \log y=x \log \left(\frac{1}{x}\right)\)

⇒ \(\frac{1}{y} \frac{d y}{d x}=x \times \frac{1}{1 / x} \times\left(\frac{-1}{x^2}\right)+\log \left(\frac{1}{x}\right) \cdot 1=-1+\log \left(\frac{1}{x}\right)\)….(1)

⇒ \(\frac{d y}{d x}=\left(\frac{1}{x}\right)^x\left[-1+\log \left(\frac{1}{x}\right)\right]\)

If \(\frac{d y}{d x}=0 \Rightarrow \log \left(\frac{1}{x}\right)=1=\log e\)

(because \(\left(\frac{1}{x}\right)^x \neq 0\))

⇒ x = \(\frac{1}{e}\)

Now differentiating equation (1) with respect to x, we get \(\frac{1}{y} \frac{d^2 y}{d x^2}-\frac{1}{y^2}\left(\frac{d y}{d x}\right)^2=x \times\left(-\frac{1}{x^2}\right)\)

⇒ \(\frac{1}{y} \frac{d^2 y}{d x^2}-\frac{1}{y^2}\left(\frac{d y}{d x}\right)^2=-\frac{1}{x}\)

⇒ \(\frac{1}{y} \frac{d^2 y}{d x^2}=\left(\log \left(\frac{1}{x}\right)-1\right)^2-\frac{1}{x}\)

⇒ \(\frac{d^2 y}{d x^2}=\left(\frac{1}{x}\right)^x\left\{\left(\log \left(\frac{1}{x}\right)-1\right)^2-\frac{1}{x}\right\}\)

because \(\frac{d^2 y}{d x^2} \text { at } x=\frac{1}{e}<0\)

hence x = \(\frac{1}{e}\) is a point of maxima.

⇒ Maximum value of \(\left(\frac{1}{x}\right)^x=(e)^{1 / e}\)

Question 5. The absolute maximum value of the function f(x) = 4x – 1/2 x² in the interval (-2, 9/2) is:

1. 8
2. 9
3. 6
4. 10

Solution:

f(x) = \(4 x-\frac{1}{2} x^2 \text { in }\left[-2, \frac{9}{2}\right]\)

⇒ \(f^{\prime}(x)=4-\frac{2 x}{2}=4-x\)

Put \(f^{\prime}(x)=0 \Rightarrow x=4\)

Now; \(f(-2)=4 \times(-2)-\frac{1}{2} \times 4=-8-2=-10 \text {, }\)

f(4) = \(4 \times 4-\frac{1}{2} \times 16=16-8=8\)

and \(f\left(\frac{9}{2}\right)=4 \times \frac{9}{2}-\frac{1}{2} \times\left(\frac{9}{2}\right)^2\)

= \(\frac{36}{2}-\frac{81}{8}=\frac{144-81}{8}=\frac{63}{8}\)

Hence, absolute maximum value of f(x) = 8 at x = 4

Question 6. In a sphere of radius r, a right circular cone of height h having maximum curved surface area is inscribed. The expression for the square of the curved surface of the cone is:

1. \(2 \pi^2 r h\left(2 r h+h^2\right)\)
2. \(\pi^2 \mathrm{rh}\left(2 \mathrm{rh}+\mathrm{h}^2\right)\)
3. \(2 \pi^2 r\left(2 h^2-h^3\right)\)
4. \(2 \pi^2 \mathrm{r}^2\left(2 \mathrm{rh}-\mathrm{h}^2\right)\)

Solution: 3. \(2 \pi^2 r\left(2 h^2-h^3\right)\)

As per the given figures,

r² = R² + (h – r)²

R² = r²- (h – r)² = h(2r – h)….(1)

h² + R² = 2rh….(2)

Now; the curved surface area of a cone is S = πRl

S² = π²R²l²

Or, S² = π²[r²-(h-r)²] (R² + h²) (from (1))

= π²h(2r-h) (2rh) (from (2))

= 2π²r(2rh² – h³)

Question 7. Show that the function f(x) = 3/x + 7 is strictly decreasing for x ∈ R-{0}.
Solution:

Given, \(f(x)=\frac{3}{x}+7 \Rightarrow f^{\prime}(x)=-\frac{3}{x^2}\)

∵ \(\mathrm{f}^{\prime}(\mathrm{x})<0\)  For all x ∈ R-{0}

Hence, The given functions strictly decreasing.

Question 8. The interval in which the function f given by f(x) = x² e^-x is strictly increasing is

1. (-∞,∞)
2. (-∞,0)
3. (2,∞)
4. (0,2)

Solution: 4. (0,2)

f(x) = \(x^2 \cdot e^{-x}\) (Differentiating with respect to x)

⇒ \(f^{\prime}(x)=-x^2 e^x+2 x e^{-x}\)

⇒ \(\mathrm{f}^{\prime}(\mathrm{x})>0\) (because \(\mathrm{f}(\mathrm{x})\) is strictly increasing)

⇒ \(-\mathrm{x}^2 \cdot \mathrm{e}^{-\mathrm{x}}+2 \mathrm{x} \cdot \mathrm{e}^{-\mathrm{x}}>0\)

⇒ \(\mathrm{e}^{-\mathrm{x}}\left(2 \mathrm{x}-\mathrm{x}^2\right)>0\)

⇒ \(e^{-x} x(2-x)>0\)

(because \(\mathrm{e}^{-x} \neq 0\) as \(\mathrm{e}^{-\mathrm{x}}\) is always positive for all x in R)

Now,

when x ∈ (0, 2), f'(x) > 0

So, f(x) is strictly increasing function interval (0, 2)

Question 9. If the radius of the circle is increasing at the rate of 0.5 cm/sec, then the rate of increase of its circumference is
Solution:

At any time t let r be the radius and c be the circumference of the circle \(\frac{dr}{dt}\) = 0.5 cm/sec (given)

∵ c = 2πr

⇒ \(\frac{dc}{dt}\) = 2π(0.5) (differentiating with respect to t)

⇒ \(\frac{dc}{dt}\) = π cm /sec

The rate of increase of its circumference is cm/sec.

Question 10. In a residential society comprising 100 houses, there were 60 children between the ages of 10-15 years. Their teachers inspired them to start composting to ensure that biodegradable waste is recycled. For this purpose, instead of each child doing it for only his/her house, children convinced the Residents Welfare Association to do it as a society initiative. For this, they identified a square area in the local park. Local authorities charged an amount of Rs. 50 per square metre for space so that there is no misuse of the space and the Resident welfare association takes it seriously. The association hired a labourer for digging and takes it seriously. The association hired a labourer to dig out 250 m³ and he charged Rs.400 x (depth)². The association would like to have a minimum cost.

1. Let the side of the square plot be x m and its depth is h metres, then cost c for the pit is

1. \(\frac{50}{h}+400 h^2\)
2. \(\frac{12500}{h}+400 h^2\)
3. \(\frac{250}{h}+h^2\)
4. \(\frac{250}{h}+400 h^2\)

Solution: 2. \(\frac{12500}{h}+400 h^2\)

Since the depth of the plot is h metres and the side is x metres,

⇒ Volume of plot is x² x h ⇒ 250 = x² x h

⇒ x² = 250/h = Area of plot

∵ The charge of space is ₹ 50 per m

So, cost of plot is \(\frac{50 \times 250}{h}=₹ \frac{12500}{h}\)

and the charge of digging is 400 x h

So total cost is c = 12500/h + 400 h² ….(1)

2. Value of h(in m) for which = \(\frac{dc}{dh}\) = 0 is

1. 1.5
2. 2
3. 2.5
4. 3

Solution: 3. 2.5

From equation (1); we have

c = \(\frac{12500}{h}+400 h^2\)

Differentiating with respect to ‘h’, \(\frac{\mathrm{dc}}{\mathrm{dh}}=\frac{-12500}{\mathrm{~h}^2}+800 \mathrm{~h}=0\)

⇒ \(\frac{12500}{\mathrm{~h}^2}=800 \mathrm{~h} \Rightarrow \mathrm{h}^3=\frac{125}{8} \Rightarrow \mathrm{h}=\frac{5}{2}=2.5 \mathrm{~m}\)

3. \(\frac{\mathrm{d}^2 \mathrm{c}}{\mathrm{dh}^2}\) is given by

1. \(\frac{25000}{h^3}+800\)
2. \(\frac{500}{h^3}+800\)
3. \(\frac{100}{h^3}+800\)
4. \(\frac{500}{h^3}+2\)

Solution: 1. \(\frac{25000}{h^3}+800\)

∵ \(\frac{\mathrm{dc}}{\mathrm{dh}}=\frac{-12500}{\mathrm{~h}^2}+800 \mathrm{~h}\)

Again differentiating with respect to h; we get \(\frac{\mathrm{d}^2 \mathrm{c}}{\mathrm{dh}^2}=\frac{25000}{\mathrm{~h}^3}+800\)

4. The value of x (in m) for minimum cost is:

1. 5
2. \(10 \sqrt{\frac{5}{3}}\)
3. \(5 \sqrt{5}\)
4. 10

Solution: 4. 10

∵ h = 2.5

⇒ \(\frac{\mathrm{d}^2 \mathrm{c}}{\mathrm{dh}^2}\) at \(\mathrm{h}=2.5\) is >0

So h=2.5 is the point of minima.

because \(x^2=\frac{250}{h}\)

⇒ \(x^2=\frac{250}{2.5} \Rightarrow x^2=100 \Rightarrow x=10 \mathrm{~m}\)

5. Total minimum cost of digging the pit (in Rupees) is:

1. 4,100
2. 7,500
3. 7,850
4. 3, 220

Solution: 2. 7,500

c = \(\frac{12500}{h}+400 h^2 \text { and } h=2.5\)

c = \(\frac{12500}{2.5}+400 \times(2.5)^2\)

c = \(\frac{12500}{25}+\left(400 \times \frac{25}{4}\right)\)

c = 5000+2500 = ₹7500

Question 11. A factory makes an open cardboard box for a jewellery shop from a square sheet of side 18 cm by cutting off squares from each corner and folding up the flaps

Based on the above information answer any four of the following five questions, if x is the length of each square cut from corners.

Solution:

Let the length of the side of the cutting square be x cm, then the length and the breadth of the box will be (18 – 2x) cm each and the height of the box is x cm.

Let V be the volume of the open box formed by folding up the flaps, then V = x(18 – 2x) (18 – 2x) = 4x(9 – x)²= 4(x³ – 18x² + 81 x)

Now, differentiating with respect to x, we get \(\frac{d V}{d x}=4\left(3 x^2-36 x+81\right)=12\left(x^2-12 x+27\right) \text { and } \frac{d^2 V}{d x}=12(2 x-12)=24(x-6)\)

For maxima or minima \(\frac{dV}{dx}\) = 0

⇒ 12(x -12x+27) = 0 or (x-3)(x-9) = 0

x = 3, 9 (but x = 9 is not possible) therefore x = 3

∴ \(\left(\frac{d^2 V}{d x}\right)_{x=3}=24(3-6)=-72<0 \text { (Maxima) }\)

1. The volume of the open box is

1. 4x(x²- 18x + 81)
2. 2x(2x² + 36x + 162)
3. 2x(2x² + 36x – 162)
4. 4x(x²+ 18x + 81)

Answer: 1. 4x(x- 18x + 81)

The volume of the open box is

V = x(18 – 2x) (18 – 2x) = 4x(x² – 18x + 81) = 432 cm

2. The condition for the volume (V) to be maximum is

1. \(4 x\left(x^2-18 x+81\right)\)
2. \(2 x\left(2 x^2+36 x+162\right)\)
3. \(2 x\left(2 x^2+36 x-162\right)\)
4. \(4 x\left(x^2+18 x+81\right)\)

Answer: 1. \(4 x\left(x^2-18 x+81\right)\)

⇒ \(\frac{\mathrm{dV}}{\mathrm{dx}}=0 \text { and } \frac{\mathrm{d}^2 \mathrm{~V}}{\mathrm{dx}^2}<0\)

3. What should be the side of the square to be cut off so that the volume is maximum?

1. 6 cm
2. 9 cm
3. 3 cm
4. 4 cm

Answer: 3. 3 cm

4. Maximum volume of the open box is

1. 423 cm³
2. 432 cm³
3. 400 cm³
4. 64 cm³

Answer: 2. 432 cm³

Maximum volume of the open box

V = 4 x 3 (9 – 18 x 3 + 81)= 12 (9 – 54 + 81) = 432 cm³

5. The total area of the removed squares is:

1. 324 cm²
2. 144 cm²
3. 36 cm²
4. 64 cm²

Answer: 3. 36 cm²

Total area of removed square = 4x² = 4 x 9 = 36 cm²

Question 12. Find the intervals in which the function f(x)= \(\frac{x^4}{4}-x^3-5 x^2+24 x+12\) is

1. Strictly increasing
2. Strictly decreasing

Solution:

Given \(f(x)=\frac{x^4}{4}-x^3-5 x^2+24 x+12\)

⇒ \(f^{\prime}(x)=x^3-3 x^2-10 x+24\)

⇒ \(f^{\prime}(x)=(x-2)(x-4)(x+3)\)

therefore f'(x) = 0, gives x = – 3, 2, 4 points divides the real line into four disjoint intervals.

namely (-∞, -3), (-3, 2). (2, 4) and (4,∞).

f'(K) > 0. when x∈(-3, 2)∪(4,∞) and f'(x) < 0. when x ∈ (-∞, -3)∪(2, 4)

Hence f(x) is strictly increasing in (-3, 2) ∪ (4, ∞) and strictly decreasing in (-∞, -3) ∪ (2, 4)

Question 13. An open tank with a square base and vertical sides is to be constructed from a metal sheet to hold a given quantity of water. Show that the cost of material will be least when the depth of the tank is half of its width. If the cost is to be borne by nearby settled lower-income families, for whom water will be provided?
Solution:

Let v and A be the volume and area of the tank, x be the side of a square base and y be the height of the tank, then v = x²y….(1)

To show (minimum)A = x² + 4xy at y = x/2

⇒ A = \(x^2+4 x y \quad \Rightarrow A=x^2+4 x\left(\frac{v}{x^2}\right) \Rightarrow A=x^2+\frac{4 v}{x}\)

⇒ \(\frac{d A}{d x}=2 x-\frac{4 v}{x^2}\)

for maxima or minima \(\frac{\mathrm{dA}}{\mathrm{dx}}=0\)

⇒ \(2 \mathrm{x}-\frac{4 \mathrm{v}}{\mathrm{x}^2}=0 \Rightarrow 2 \mathrm{x}=\frac{4 \mathrm{v}}{\mathrm{x}^2} \Rightarrow 2 \mathrm{v}=\mathrm{x}^3\)

From equation (1) \(v=x^2 y \Rightarrow 2 x^2 y=x^3 \Rightarrow y=\frac{x}{2}\)

Now \(\frac{\mathrm{d}^2 \mathrm{~A}}{\mathrm{dx}^2}=2+\frac{8 \mathrm{v}}{\mathrm{x}^3}\)

⇒ \(\left(\frac{\mathrm{d}^2 \mathrm{~A}}{\mathrm{dx}^2}\right)>0\) when \(\mathrm{x}^3=2 \mathrm{v}\)

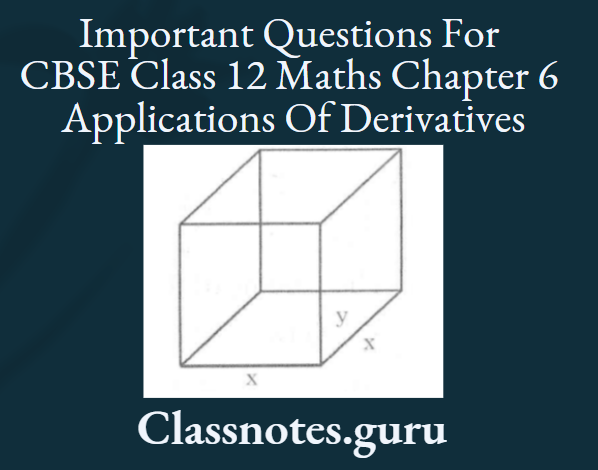
Hence cost of material will be least at y=x/2

Question 14. Amongst all open (from the top) right circular cylindrical boxes of volume 1 25TC cm-1. find the dimensions of the box which has the least surface area.
Solution:

Let r be the radius, h be the height and V be the volume of the cylindrical box respectively

∵ V = 125 π cm³ (given)

∴ \(\pi \mathrm{r}^2 \mathrm{~h}=125 \pi \Rightarrow \mathrm{h}=\frac{125}{\mathrm{r}^2}\)….(1)

Let S be the surface area of the cylindrical box

∴ S = \(2 \pi r h+\pi r^2=\pi r(2 h+r)\)

⇒ S = \(\pi r\left(\frac{250}{r^2}+r\right)\) [from equation(1)]

S = \(\frac{250 \pi}{r}+\pi r^2\)

⇒ \(\frac{\mathrm{dS}}{\mathrm{dr}}=-\frac{250 \pi}{\mathrm{r}^2}+2 \pi r\) and \(\frac{\mathrm{d}^2 \mathrm{~S}}{\mathrm{dr}^2}=\frac{500 \pi}{\mathrm{r}^3}+2 \pi\)

for maxima or minima \(\frac{\mathrm{dS}}{\mathrm{dr}}=0\)

⇒ \(2 \pi r=\frac{250 \pi}{r^2} \Rightarrow r^3=125 \Rightarrow r=5 \Rightarrow\left(\frac{d^2 S}{d^2}\right)_{r=5}>0\)

So the surface area S of the cylinder is least at r = 5

Hence, dimensions of box are r = 5 cm and h = 5 cm

Question 15. A tank with a rectangular base and rectangular sides, open at the top is to be constructed so that its depth is 2 m and volume is 8 m³. If the building of the tank costs Rs. 70 per sq metre for the base and Rs 45 per square metre for the sides. What is the cost of the least expensive tank?
Solution:

Given: A tank with rectangular base and rectangular sides, open at the top and Depth of tank = 2 m

Let x m be the length and y m be the breadth of the base of the tank.

Volume of tank (= /bh) = x.y.2 = 8 m³ (given)

∴ y = \(\frac{8}{2 x}=\frac{4}{x}\)……(1)

Now cost of building the* base of the tank at the given rate of Rs 70 per square metre is Rs 70 xy……(2)

Again the cost of building the four sides (walls), of the tank at the rate of Rs 45 per square metre.

= 45(x.2 + x.2 + y.2 + y.2) = 45(4x + 4y)

= Rs (1 80x + 180y)….(3)

Let z denote the total cost of building the tank.

Adding (2) and (3), z = 70xy + 180x + 180y

Putting y = 4/x from (1), z = 70x. 4/x + 180x + 180. 4/x

or z = 280 + 180x + 720/x…..(4)

∴ \(\frac{\mathrm{dz}}{\mathrm{dx}}=0+180-\frac{720}{\mathrm{x}^2} \text { and } \frac{\mathrm{d}^2 \mathrm{z}}{\mathrm{dx}^2}=\frac{1440}{\mathrm{x}^3}\)

Putting \(\frac{\mathrm{dz}}{\mathrm{dx}}\) = 0 to find turning points, we have

180 \(-\frac{720}{x^2}=0\)

180 = \(\frac{720}{x^2}\)

180 \( x^2=720\)

⇒ \(x^2=\frac{720}{180}=4 \Rightarrow x=2\)(because x being length can’t negative )

At x=2, \(\frac{\mathrm{d}^2 \mathrm{z}}{\mathrm{dx}^2}=\frac{1440}{\mathrm{x}^3}=\frac{1440}{8}=180(+\mathrm{ve})\)

∴ z is minimum at x=2

Putting x=2 in (4), minimum cost

z = \(280+180(2)+\frac{720}{2}=280+360+360=280+720=\text { Rs. } 1000 .\).